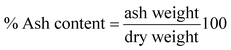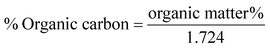Open Access Article
Open Access ArticleUtilization of Euryale ferox Salisbury seed shell for removal of basic fuchsin dye from water: equilibrium and kinetics investigation†
S. Kalitaa,
M. Pathaka,
G. Devia,
H. P. Sarmab,
K. G. Bhattacharyya c,
A. Sarmad and
A. Devi
c,
A. Sarmad and
A. Devi *a
*a
aEnvironmental Chemistry Laboratory, Resource Management and Environment Section, Life Science Division, Institute of Advanced Study in Science and Technology, Guwahati, Assam, India. E-mail: deviarundh2@yahoo.co.in; Fax: +91 3612273062; Tel: +91 3612912075 ext. 118
bDepartment of Environmental Science, Gauhati University, Guwahati, Assam, India
cDepartment of Chemistry, Gauhati University, Guwahati, Assam, India
dDepartment of Chemistry, Morigaon College, Morigaon 782105, Assam, India
First published on 26th May 2017
Abstract
Euryale ferox Salisbury (E. ferox) is an environmentally and economically important wetland macrophyte. This paper investigates the adsorption of a carcinogenic dye, basic fuchsin in the aqueous phase onto the hard shell of Euryale ferox seeds so as to establish the thrown away residue as a novel, efficient, bio-friendly and economically low-cost alternative adsorbent against other expensive adsorbents. Characterization of the bioadsorbent was carried out by TGA, SEM, FTIR and Zetasizer analyses. Zeta potential analysis showed good stability of the biomaterial around neutral pH. The operating variables such as adsorbent amount, adsorbate concentration, contact time, pH and temperature were optimized in a batch system. The maximum biosorption capacity of E. ferox was found to be 19.48 mg g−1 which could remove as much as 97.4% of the dye from an aqueous solution of concentration 40 mg L−1 at 298 K. Isothermal and kinetic data fitted best to Freundlich and pseudo second order models respectively. The thermodynamic study revealed the exothermic and spontaneous nature of the adsorption process.
1 Introduction
Dyes have always been an integral part of human civilization, whether for dyeing cloth, leather, and hair or as a coloring agent for food. In the last few decades with the surge in human population and economic development, there has been a tremendous development of the synthetic dye industries for meeting the demand for higher supply and better quality of dye. This expansion has resulted in a simultaneous increase in the liquid effluent generated from the synthetic dye manufacturing and user industries. It is estimated that annually more than 1.5 × 108 m3 of colored effluents is discharged as waste water.1The discharged wastewater enters the local water bodies and disturbs the aquatic ecosystem by influencing the physicochemical parameters of water which are known for contributing to the maintenance of the ecosystem, essential for the dwelling aquatic organisms. Since most of the dyes are suspected to be carcinogenic and mutagenic in nature, they have the potential to bring toxicity in human beings, fish species and microorganisms.2,3
Basic fuchsin (MF: C20H20CIN3, MW: 337.85) a cationic dye consisting of the salts (hydrochloride or acetate) of rosaniline and pararosaniline with triaminotriphenylmethane as its chemical structural body is widely used as a coloring agent for textile and leather industries. It is also used as a biological stain for nucleus, tissues, and muscles and as a tracking dye for certain proteins.4,5 On direct contact with skin or when ingested or inhaled the dye may cause severe health hazards like irritation to skin or respiratory or gastrointestinal tract.5 The suspected carcinogenic, mutagenic and target organ effects of this dye on humans gives the reason to develop a competent and efficient material or a method for tackling and efficiently removing this pollutant. Several conventional techniques such microbiological or enzymatic decomposition, oxidation, coagulation, flocculation, chemical degradation, electroplating, membrane filtration, etc.6,7 have been developed and used till now. But among these available techniques, the biosorption technology has emerged as a more attractive and greener alternative to the typical treatment tactics employed for decolourization of effluents due to its modest design, smooth operation and most importantly its potential cost-effectiveness. For removal of basic fuchsin, only a few adsorbents have been developed till now namely peat-resin particle,8 Aspergillus niger dead biomass,9 bottom ash and deoiled soya,5 KMnO4-modified activated carbon from Typha orientalis,10 mussel shell11 etc. The present study aims to utilize a novel waste material capable of removing dye material as a model experiment so as to boost the emerging field of bioremediation through the adsorption strategy. The prepared material is the seed coat of Euryale ferox Salisbury (Nymphaeaceae family), locally famous as “Makhana”. It was reported that E. ferox known for its nutritional and medicinal properties is well distributed in the tropical and subtropical regions of Southeast and East Asia.12 In India, it is found in the shallow water bodies of North Bihar and lower Assam regions of India.12 E. ferox seed cover was chosen as a dye removal bioadsorbent because it is an easily available and unused bio-refuse of the edible part of the plant. Even though other biomaterials have been used for removal of basic fuchsin, this macrophytic bio-waste has remained unexplored as a source for remediating wetland water bodies polluted with colored effluents. Relevant kinetic and adsorption isotherm models were employed for a better understanding of this unique adsorbent performance. As the material is sufficiently found to be flourishing in the wetland, there is a greater chance of using this adsorbent for remediating a wetland polluted with anthropogenic activities.
The concentrations of dyes and pigments in industrial effluents are not well documented and the concentrations vary depending on the nature of the industry, the amount of dyes used, the efficiency of the effluent treatment procedures, etc. The present experiments can be considered as a kind of model in which a comparatively wide range of dye concentrations was used with a view to having results of general utility. The adsorbent amounts were similarly selected to achieve optimum adsorption and removal of the dyes.
2 Experimental
2.1 Collection and preparation of the adsorbent and adsorbate
Fresh fruits of E. ferox were collected from the wetland Deepor Beel (a Ramsar site) of Assam, India. The separated seeds from the harvested fruits were washed and shade dried. The seed coat to be utilized as an adsorbent was then removed from the dried seeds and was again washed, air-dried and ground into a powdered form. It was further sieved in between the fraction range of 75–200 μm and was repeatedly washed with Millipore water to obtain color and turbidity-free washings. The washed seed powder fractions were first dried at room temperature for 48 hours and then oven dried at 50 °C temperature to a constant weight. It was then made moisture free in a desiccator until its further use. The basic fuchsin dye taken for the experiment purpose was of analytical grade purchased from Sigma-Aldrich. A stock solution of 500 ppm was prepared by dissolving weighed amount of powdered dye in distilled water. A calibration curve was obtained by measuring the optical density of different known dye concentrations at 542 nm using a UV-Vis spectrophotometer (Shimadzu 1601, Japan).13 Chemdraw Ultra 8.0 software was used for the construction of the chemical structure of the dye. The chemical structure of the dye is as follows:2.2 Characterization of the adsorbent biomaterial
Gravimetric analysis for the determination of moisture content, organic matter and organic carbon of the biomaterial was done in a muffle furnace following the AOAC 2000 protocol14 and were calculated using the following equations:The biomass was treated prior to the determination of its lignocellulosic components following the TAPPI method.15,16 The treated biomass is considered to comprise of cellulose, hemicellulose, lignin and extractives. Thermal gravimetric analysis (TGA) was carried out using Perkin Elmer TGA 4000 at a heating rate of 10 °C per minute with N2 flow rate of 20 mL min−1. CHNS analyser (EuroEA3000 Elemental Analyser) and Atomic Absorption Spectrophotometer (Shimadzu AA 7000) was used for ultimate and element analysis respectively. The surface topography of the biomaterial was analyzed by using Field Emission Scanning Electron Microscope i.e. FE-SEM (Zeiss, Σ-Sigma, Germany). The Fourier Transform Infrared Spectrophotometer (NICOLET 6700, USA) was used to record the spectra in transmission mode within the range of 4000–500 cm−1 to investigate about the functional groups of the adsorbent by employing the KBr pellet method. The surface charge of the adsorbent solution was determined by recording zeta potential measurements of the solution using the Malvern Zetasizer Nano series, Nano-ZS90. Same instrumental analyses were performed for evaluating the potential changes occurred in the biomaterial after the adsorption of the subject dye.
2.3 Bioadsorption procedure
The adsorption experiments were carried out in a series of the batch to evaluate the percentage removal and adsorption capacity of the material. The parameter variation experiments were operated in 100 mL Erlenmeyer flasks with a 50 mL test dye solution placed in a thermostatic shaker at 120 rpm for agitation. The effect of the adsorbent amount, adsorbate strength, contact time and temperature were inspected by varying each parameter value at the natural pH of the working solution. The pH of the BF aqueous solution did not change much with dilution and was found to be between 6.0–6.5. Therefore, the effect of the pH variation on the adsorption of test solution of varying concentration was studied after the optimization of other parameters. The pH was adjusted using 0.1 N NaOH and 0.1 N HCl solutions. The equilibrium reached a concentration of BF solution was centrifuged and then analysed at 542 nm. Compared to inorganic heavy materials, the biosorbent settles down slowly, but complete settlement could be achieved without much difficulty. The obtained results were used to calculate the equilibrium adsorption capacity qe (mg g−1) and the percentage removal by applying the following equations:| qe = {(Co − Ce)V}/M | (1) |
| Dye removal (%) = {Co − Ce/Co} × 100 | (2) |
In the present study, the adsorption data were subjected to different isotherm and kinetic models for examining and understanding the mechanistic steps and surface chemistry involved in the adsorption process. The thermodynamic behaviour was also extensively studied. The details of the models and equations used are given in the appendix. All the experiments were performed in triplicates and the standard error (SE) values are mentioned in the figures and tables.
3 Results and discussion
3.1 Characterization of E. ferox
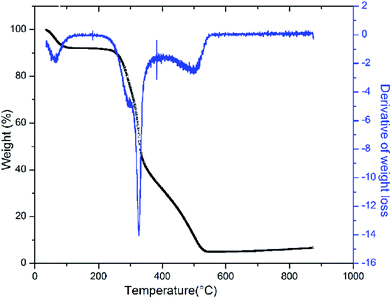
The TGA result regarding the presence of different lignocellulosic constituents was confirmed by gravimetric analysis. The cellulose, hemicellulose lignin and extractives amount was determined in percentage as 14.4%, 38.9%, 42.5% and 4.2%. The presence of high lignin content in the shell is responsible for the protection of interior portion of the seed. On an average nutshells tend to contain more lignin content than the other lignocellulosic constituents.20 The amount of lignin found in this work is the higher when compared to cellulose and hemicellulose in coconut shell (46%) as reported by Cagnon et al.21 respectively. Apart from investigating the thermal behaviour of the adsorbent, the TGA technique could also be used for proximate analysis of the material. The results of proximate analysis and ultimate analysis are given in Table 1. The differences in the ash content analysis using simple gravimetric analysis (0.4%) in a muffle furnace and by TGA (5%) arose because of the loss in fly ash and other volatile matter during the open heating of the sample in the muffle furnace.
| Proximate content (in %) | Ultimate content (%) | ||
|---|---|---|---|
| Moisture content | 7 ± 0.03 | Carbon | 43.82 ± 0.31 |
| Ash content | 5 ± 0.41 | Hydrogen | 4.1 ± 0.01 |
| Volatile matter | 69 ± 0.01 | Nitrogen | 0.81 ± 0.01 |
| Fixed carbon | 19 ± 0.02 | Sulphur | 0.06 ± 0.07 |
| Oxygen | 51.21 ± 0.49 |
The proximate and ultimate analysis result were in accordance to the reported literature by Kumar and Jena.22 The determined values of trace elements like potassium, magnesium, zinc, copper, manganese, sodium, calcium and phosphorus are given in Table 2. According to Ekpete and Horsfall,23 the low ash content and low moisture is an indicative that the raw material can be used as a bioadsorbent. Therefore, E. ferox is an excellent raw material to be put into use as a bioadsorbent.
| Elemental analysis (in mg kg−1) | |
|---|---|
| Potassium | 299.43 ± 4.21 |
| Calcium | 461.20 ± 3.21 |
| Magnesium | 87.425 ± 1.03 |
| Sodium | 278.62 ± 2.03 |
| Zinc | 47.55 ± 0.10 |
| Manganese | 14.857 ± 0.02 |
| Copper | 6.9 ± 0.08 |
| Phosphorus | 652 ± 0.08 |
![[double bond splayed left]](https://www.rsc.org/images/entities/char_e009.gif) NH) appearing at 3309.0 and 3159.3 cm−1 as observed in the FTIR spectra of the dye, which were likely to have been masked by the OH band.
NH) appearing at 3309.0 and 3159.3 cm−1 as observed in the FTIR spectra of the dye, which were likely to have been masked by the OH band.
This might be an indication that E. ferox and BF interactions take place through these functional groups. The symmetric and asymmetric vibrational modes of methyl (–CH3) and methylene (![[double bond splayed left]](https://www.rsc.org/images/entities/char_e009.gif) CH2) groups showed a small shift from 2939.5 to 2935.5 cm−1 after adsorption of the dye which might suggest participation of these groups also in taking up the dye molecules.26 The bands at 1448.2 and 1371.3 cm−1 in the spectra of the biomaterial can be attributed to C–O stretching of carboxylic or carbonate group and the bending vibrations of C–H or OH groups respectively.25 After adsorption of the dye, a shift was recorded in these wave numbers from 1448.2 to 1454.5 cm−1 and 1371.3 to 1366.7 cm−1 confirming the involvement of those functional groups in binding the dye to E. ferox surface. The FTIR results suggest that the binding of the BF dye to the bioadsorbent surface may have occurred mainly due to interactions between OH groups of E. ferox and NH/NH2 groups of the dye. The presence of hydroxyl and carboxyl groups in the FT-IR spectra, further, establishes the anionic nature of the bioadsorbent. Therefore, these groups are likely to serve as the binding sites for the cationic dye.3 Similar results were previously observed for adsorption of dyes on cupuassu shell.27
CH2) groups showed a small shift from 2939.5 to 2935.5 cm−1 after adsorption of the dye which might suggest participation of these groups also in taking up the dye molecules.26 The bands at 1448.2 and 1371.3 cm−1 in the spectra of the biomaterial can be attributed to C–O stretching of carboxylic or carbonate group and the bending vibrations of C–H or OH groups respectively.25 After adsorption of the dye, a shift was recorded in these wave numbers from 1448.2 to 1454.5 cm−1 and 1371.3 to 1366.7 cm−1 confirming the involvement of those functional groups in binding the dye to E. ferox surface. The FTIR results suggest that the binding of the BF dye to the bioadsorbent surface may have occurred mainly due to interactions between OH groups of E. ferox and NH/NH2 groups of the dye. The presence of hydroxyl and carboxyl groups in the FT-IR spectra, further, establishes the anionic nature of the bioadsorbent. Therefore, these groups are likely to serve as the binding sites for the cationic dye.3 Similar results were previously observed for adsorption of dyes on cupuassu shell.27
3.2 Effects of various parameters on adsorption
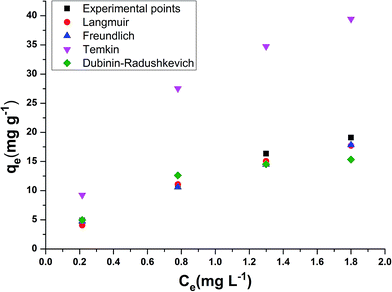
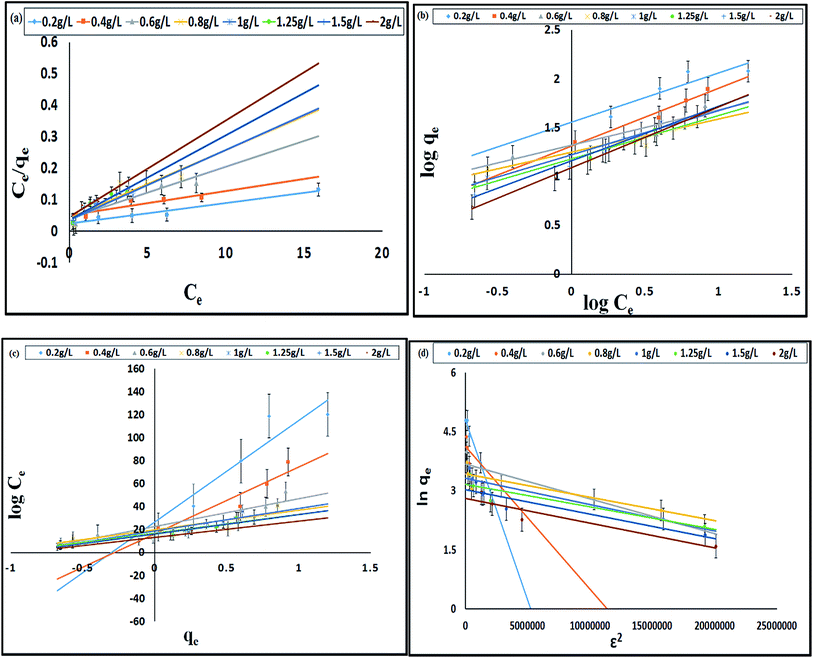
The models which were applied to fit the equilibrium data are Langmuir, Freundlich, Temkin and Dubinin–Radushkevich whose plots at a temperature of 308 K are presented in Fig. 5a–d respectively. Table 3 shows the comparison of adsorption capacity of various bioadsorbents along with the present work. Most of the adsorbents are either modified or in activated carbon form, thereby, having higher qe than E. ferox. The values obtained for the different isotherm parameters along with the correlation coefficient are listed in Table 4. The r2 values of Freundlich isotherm in comparison to other two models were found to be more close to 1 suggesting that the Freundlich model anticipates the adsorption behaviour of the BF dye better on E. ferox.
| Bioadsorbent | Experimental conditions | Adsorption capacity | References |
|---|---|---|---|
| KMnO4 modified Typha orientalis activated carbon | Dye concentration-100 ppm, adsorbent-750 mg , volume-100 mL, temperature-17 °C, time-2.5 h | 145 mg g−1 | 10 |
| Tea dust activated carbon | Dye concentration-100 ppm, adsorbent-100 mg, volume-100 mL, temperature-30 °C, time-90 min | 73.2 mg g−1 | 37 |
| Modified peat-resin particle | Dye concentration-100 ppm, adsorbent-0.5 g, volume-100 mL, temperature-25 °C, time-700 min | 280 mg g−1 | 8 |
| Calcined mussel shell | Dye concentration-50 ppm, adsorbent-500 mg, volume-100 mL, temperature-25 °C, time-240 min | 141.65 mg g−1 | 11 |
| E. ferox | Dye concentration-40 ppm, adsorbent-2 g, volume-50 mL, temperature-30 °C, time-120 min | 19.1 mg g−1 | This work |
| Isotherm models & parameter | E. ferox amount (g L−1) | |||||||
|---|---|---|---|---|---|---|---|---|
| 0.2 | 0.4 | 0.6 | 0.8 | 1 | 1.2 | 1.5 | 2 | |
| Langmuir coefficient | ||||||||
| qm (mg g−1) | 153.84 ± 3.360 | 129.87 ± 0.800 | 60.97 ± 0.001 | 46.29 ± 0.040 | 44.84 ± 0.060 | 37.03 ± 0.5200 | 37.45 ± 0.040 | 32.67 ± 0.046 |
| b (L mg−1) | 0.26 ± 0.010 | 0.15 ± 0.001 | 0.39 ± 0.001 | 0.50 ± 0.030 | 0.61 ± 0.001 | 0.79 ± 0.030 | 0.70 ± 0.001 | 0.65 ± 0.010 |
| RL | 0.98 ± 0.020 | 0.97 ± 0.001 | 0.95 ± 0.001 | 0.93 ± 0.02 | 0.94 ± 0.01 | 0.93 ± 0.02 | 0.95 ± 0.002 | 0.95 ± 0.01 |
| r2 | 0.950 | 0.770 | 0.780 | 0.790 | 0.820 | 0.870 | 0.810 | 0.820 |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) |
||||||||
| Freundlich coefficient | ||||||||
| n | 0.50 ± 0.070 | 0.60 ± 0.100 | 0.40 ± 0.010 | 0.33 ± 0.010 | 0.50 ± 0.010 | 0.40 ± 0.850 | 0.60 ± 0.010 | 0.62 ± 0.170 |
| Kf (L mg−1) | 35.96 ± 0.310 | 20.53 ± 0.200 | 21.04 ± 0.060 | 17.88 ± 0.500 | 16.77 ± 0.01 | 15.22 ± 0.250 | 14.54 ± 1.250 | 12.39 ± 0.020 |
| r2 | 0.790 | 0.960 | 0.890 | 0.890 | 0.950 | 0.970 | 0.980 | 0.990 |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) |
||||||||
| Temkin coefficient | ||||||||
| A (L g−1) | 2.01 ± 0.310 | 1.92 ± 0.310 | 8.95 ± 0.310 | 14.43 ± 0.310 | 9.33 ± 0.310 | 11.53 ± 0.310 | 9.20 ± 0.310 | 8.91 ± 0.310 |
| b (kJ mol−1) | 28.99 ± 0.310 | 44.05 ± 0.520 | 106.06 ± 3.250 | 148.41 ± 1.250 | 130.6 ± 1.960 | 157.35 ± 4.170 | 151.94 ± 0.820 | 180.24 ± 0.160 |
| B (J mol−1) | 88.32 ± 0.870 | 58.12 ± 0.130 | 24.14 ± 0.090 | 17.25 ± 0.610 | 19.6 ± 0.190 | 16.27 ± 0.018 | 16.85 ± 0.440 | 14.20 ± 0.260 |
| r2 | 0.820 | 0.880 | 0.770 | 0.770 | 0.840 | 0.880 | 0.880 | 0.900 |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) |
||||||||
| Dubinin–Radushkevich | ||||||||
| E (kJ mol−1) | 0.74 ± 0.870 | 1.12 ± 0.870 | 2.36 ± 0.870 | 2.89 ± 0.870 | 2.68 ± 0.870 | 2.89 ± 0.870 | 2.89 ± 0.870 | 2.89 ± 0.870 |
| qs (mg g−1) | 122.48 ± 4.830 | 62.17 ± 2.870 | 39.44 ± 0.008 | 30.53 ± 0.630 | 27.6 ± 0.130 | 23.52 ± 0.470 | 20.69 ± 0.005 | 16.56 ± 0.030 |
| r2 | 0.950 | 0.890 | 0.890 | 0.930 | 0.920 | 0.950 | 0.970 | 0.950 |
The Langmuir isotherm model assumption is about monolayer coverage of the liquid phase concentration of the dye on a solid surface of the adsorbent in such a way that all the binding sites for the adsorption are equivalent and the surface is uniform with no interactions between adsorbed molecules.29 The monolayer biosorption capacity (qm) was calculated to be 32.67–153.84 mg g−1 over the entire range of adsorbent dosage from the linear plot (Fig. 5a). Another Langmuir constant, the energy of adsorption (b) determines the affinity between adsorbate and adsorbent. The values of b varying from 0.15–0.79 L mg−1 indicates a good affinity between the dye and the adsorbent. This result supports the earlier report of adsorption of copper ions by Azadirachta indica leaf powder.30 In the Langmuir isotherm model the Hall separation factor, RL, determines the suitability of the biosorbent for a favorable adsorption process when its values lie in between 0 and 1.25 In the present study, the RL values obtained in the range of 0.93–0.98 within the given adsorption favorable dimension confirms the suitability of the E. ferox as a bioadsorbent for the Langmuir-type adsorption process.
The Freundlich linear plots (Fig. 5b) with a coefficient of determination, r2 values ranging 0.79–0.99 indicate the occurrence of a multilayer, energetically non-uniform, and heterogeneous adsorption of basic fuchsin on the surface of E. ferox.2,11 The calculated adsorption capacity (Kf) of the adsorbent was found to be in the range 12.39–35.96 L mg−1. Further, the display of adsorption intensity (n) values between 0.33–0.62 corresponding to the condition 0 < 1/n < 1 by the adsorbent implies a favorable heterogeneous adsorption process.11,25 If the heterogeneity factor draws closer to 1 it means the surface has more heterogeneity. Therefore, heterogeneity factor with an average value of 0.49 indicates an intermediate range of heterogeneity for the adsorbent surface.28 Similarly, Freundlich isotherm was found as a better isotherm fit model for studies of dye adsorption studies onto fly ash,31 Pseudomonas putida32 and Pinus sylvestris.33
The Temkin model takes into account the effects of the interaction of the adsorbate and the adsorbing species.34 The correlation coefficient (r2) ranging from 0.77–0.9 was obtained from the linear plots of qe against log![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) Ce (Fig. 5c). The equilibrium binding constant (A) and Temkin constant b varied from 1.92–14.43 L g−1 and 28.99–180.24 kJ mol−1 respectively. This model assumes that the heat of adsorption of all of the molecules in the layer would decrease linearly rather than logarithmically with coverage due to adsorbate–adsorbent interactions.35 Table 4 shows that the estimated B values decreases with increase coverage of the adsorbent. B values being higher than 1 proposes the occurrence of electrostatic interaction between the dye and heterogeneous surface of E. ferox.19
Ce (Fig. 5c). The equilibrium binding constant (A) and Temkin constant b varied from 1.92–14.43 L g−1 and 28.99–180.24 kJ mol−1 respectively. This model assumes that the heat of adsorption of all of the molecules in the layer would decrease linearly rather than logarithmically with coverage due to adsorbate–adsorbent interactions.35 Table 4 shows that the estimated B values decreases with increase coverage of the adsorbent. B values being higher than 1 proposes the occurrence of electrostatic interaction between the dye and heterogeneous surface of E. ferox.19
Dubinin and Radushkevich model is used for distinguishing an adsorption process on a heterogeneous surface by plotting ln![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) qe against ε2.36 The plots (Fig. 5d) obtained were linear (r2 = 0.73–0.96) with calculated D–R adsorption capacity (qs) values ranging from 16.56 to 122.48 mg g−1. The mean free energy of adsorption (E) has small values in the range of 0.74 to 2.89 kJ mol−1 (Table 4) indicating that the adsorption process is nearly spontaneous.11 Also, the Kad values below 1 implies the surface heterogeneity of E. ferox.19
qe against ε2.36 The plots (Fig. 5d) obtained were linear (r2 = 0.73–0.96) with calculated D–R adsorption capacity (qs) values ranging from 16.56 to 122.48 mg g−1. The mean free energy of adsorption (E) has small values in the range of 0.74 to 2.89 kJ mol−1 (Table 4) indicating that the adsorption process is nearly spontaneous.11 Also, the Kad values below 1 implies the surface heterogeneity of E. ferox.19
| Kinetic models & parameter | E. ferox amount (g L−1) | |||||||
|---|---|---|---|---|---|---|---|---|
| 0.2 | 0.4 | 0.6 | 0.8 | 1 | 1.2 | 1.5 | 2 | |
| Pseudo-first order kinetic | ||||||||
| qe (mg g−1) | 78.6 ± 0.21 | 53.41 ± 0.8 | 35.63 ± 0.07 | 36.57 ± 0.13 | 36.08 ± 0.5 | 33.87 ± 0.87 | 16.71 ± 0.27 | 11.35 ± 0.007 |
| k1 (min−1) | 0.014 ± 0.31 | 0.009 ± 0.001 | 0.01 ± 0.001 | 0.019 ± 0.04 | 0.021 ± 0.001 | 0.03 ± 0.003 | 0.02 ± 0.001 | 0.021 ± 0.001 |
| r2 | 0.950 | 0.960 | 0.940 | 0.910 | 0.870 | 0.940 | 0.950 | 0.950 |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) |
||||||||
| Pseudo-second order kinetic | ||||||||
| qe (mg g−1) | 123.45 ± 0.009 | 77.51 ± 0.010 | 53.19 ± 0.640 | 43.29 ± 0.290 | 37.87 ± 0.170 | 32.25 ± 0.310 | 26.04 ± 0.110 | 19.68 ± 0.300 |
| k2 (g−1 mg−1 min−1) | 0.0005 ± 0.001 | 0.0005 ± 0.001 | 0.0008 ± 0.000 | 0.0011 ± 0.001 | 0.0012 ± 0.000 | 0.0019 ± 0.010 | 0.0003 ± 0.003 | 0.005 ± 0.001 |
| r2 | 0.93 | 0.860 | 0.880 | 0.890 | 0.890 | 0.940 | 0.960 | 0.970 |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) |
||||||||
| Elovich | ||||||||
| α (mg g−1 min−1) | 0.012 ± 0.001 | 0.014 ± 0.004 | 0.017 ± 0.001 | 0.021 ± 0.002 | 0.036 ± 0.001 | 0.046 ± 0.001 | 0.111 ± 0.009 | 0.356 ± 0.090 |
| β (g mg−1) | 29.37 ± 0.090 | 21.28 ± 0.010 | 13.77 ± 0.030 | 11.25 ± 0.080 | 10.04 ± 0.040 | 7.79 ± 0.009 | 5.61 ± 0.050 | 3.67 ± 0.010 |
| r2 | 0.890 | 0.810 | 0.800 | 0.850 | 0.840 | 0.890 | 0.920 | 0.900 |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) |
||||||||
| Intra-particle diffusion model | ||||||||
| Intercept | 23.46 ± 0.440 | 3.27 ± 0.010 | 4.69 ± 0.005 | 3.95 ± 0.010 | 2.8 ± 0.002 | 6.06 ± 0.010 | 7.49 ± 0.100 | 7.6 ± 0.120 |
| kp (mg g−1 min−0.5) | 8.38 ± 0.250 | 6.14 ± 1.010 | 3.99 ± 0.010 | 3.24 ± 0.170 | 2.89 ± 0.310 | 2.21 ± 0.250 | 1.58 ± 0.100 | 1.03 ± 0.001 |
| r2 | 0.950 | 0.890 | 0.890 | 0.930 | 0.920 | 0.950 | 0.970 | 0.950 |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) |
||||||||
| Film diffusion model | ||||||||
| Intercept | −0.34 ± 0.010 | −0.32 ± 0.010 | −0.33 ± 0.001 | −0.07 ± 0.004 | 0.009 ± 0.090 | 0.2 ± 0.010 | −0.308 ± 0.060 | −0.43 ± 0.010 |
| kfd (min−1) | 0.01 ± 0.001 | 0.01 ± 0.010 | 0.1 ± 0.010 | 0.02 ± 0.001 | 0.02 ± 0.010 | 0.03 ± 0.010 | 0.02 ± 0.010 | 0.02 ± 0.001 |
| r2 | 0.960 | 0.980 | 0.950 | 0.860 | 0.800 | 0.790 | 0.940 | 0.920 |
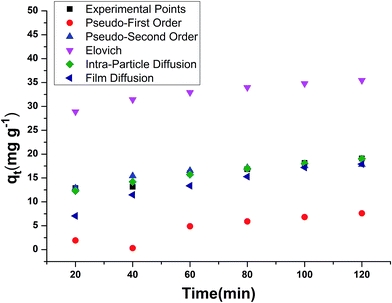
In the pseudo first order reaction model, the computation of the adsorption rate constant k1 was done by plotting log(qe − q) against t for a constant concentration of BF (40 mg L−1) with different E. ferox amount as shown in Fig. 7a. The values of the experimentally obtained adsorption capacity (qe) and that of rate constant k1 are given in Table 5. Even though all the plots showed good linearity with correlation coefficient (r2) values ranging from 0.87–0.96, the experimental ‘qe’ values at equilibrium did not agree with the calculated values of adsorption capacity. The disagreement between the experimental and calculated qe value indicates the sorption kinetics involved in adsorption of BF dye on to E. ferox do not follow pseudo-first order kinetic model.3,11 Therefore, pseudo second order model was applied by plotting t/qt versus t (Fig. 7b). Table 5 shows that unlike the pseudo first order model, the calculated ‘qe’ values from the pseudo second order model were similar to the experimental values. The r2 values (0.86–0.97) were closer to unity showing the predominance of this model in the adsorption process. The result indicates that the adsorption of dye on E. ferox surface follows the pseudo second order kinetic model with chemisorption adsorption mechanism.11,39
The plots qt versus ln![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) t (Fig. 7c) were plotted to verify the applicability of the Elovich equation to kinetic sorption study of E. ferox. This model is utilized for studying the chemisorption processes on a heterogeneous surface.35 Therefore, the linear plots with correlation coefficients varying from 0.8–0.92 suggest that this model can be used to describe chemisorption adsorption of BF dye on the heterogeneous surface of E. ferox. The desorption constant (β) varied from 3.67–29.37 g mg−1. The initial adsorption rate (α) was found to elevate from 0.012–0.356 mg g−1 min−1 on increasing the E. ferox amount (0.2–2 g L−1). The increase in initial adsorption rate due to increase in adsorbent amount can be attributed to the availability of larger surface area for adsorption.28
t (Fig. 7c) were plotted to verify the applicability of the Elovich equation to kinetic sorption study of E. ferox. This model is utilized for studying the chemisorption processes on a heterogeneous surface.35 Therefore, the linear plots with correlation coefficients varying from 0.8–0.92 suggest that this model can be used to describe chemisorption adsorption of BF dye on the heterogeneous surface of E. ferox. The desorption constant (β) varied from 3.67–29.37 g mg−1. The initial adsorption rate (α) was found to elevate from 0.012–0.356 mg g−1 min−1 on increasing the E. ferox amount (0.2–2 g L−1). The increase in initial adsorption rate due to increase in adsorbent amount can be attributed to the availability of larger surface area for adsorption.28
Investigation of the diffusion process and to verify the influence of mass transfer resistance on the binding of basic fuchsin to E. ferox, the intraparticle diffusion model was applied. The intra-particle diffusion constant kp (mg g−1 min−0.5) was obtained from the linear plots of qt (mg g−1) versus square root of time. The values of kp varied from 1.03–8.38 mg g−1 min−1/2. The plots failed to pass through the origin (Fig. 7d) and had significant intercept values (Table 5) implying that the model would not solely limit the overall adsorption kinetics and intraparticle diffusion might have little role to play in the overall kinetics.
The liquid film diffusion model was used in this study to investigate the role of the transport of dye cations from the liquid phase up to the solid phase boundary of E. ferox. The liquid film diffusion constant, kfd, was in the range of 0.01–0.03 min−1 (Table 5). The linear plots of −ln(1 − F) vs. t (Fig. 7e) had very small intercepts and since the intercept values were related to the thickness of the boundary layer, it was likely that the dye cations had to pass through a comparatively thin surface layer of the liquid phase before being adsorbed on the biosorbent surface.28,40 It would imply that the kinetics of the sorption process could have been influenced appreciably by diffusion of the dye cations across the liquid film on the particle surfaces.41
The thermodynamic parameters were computed from the plots of log![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) qe/Ce against 1/T to evaluate the thermodynamics of adsorption and to work out the feasibility of E. Ferox as a biosorbent for the dye. Table 6 shows that the ΔG values decreased with an increase in temperature for a constant and varied adsorbate concentration.
qe/Ce against 1/T to evaluate the thermodynamics of adsorption and to work out the feasibility of E. Ferox as a biosorbent for the dye. Table 6 shows that the ΔG values decreased with an increase in temperature for a constant and varied adsorbate concentration.
| BF (mg L−1) | −ΔH (kJ mol−1) | −ΔS (J mol−1 L−1) | −ΔG (kJ mol−1) | |||
|---|---|---|---|---|---|---|
| 298 K | 303 K | 308 K | 313 K | |||
| 10 | −14.39 | −20.25 | −8.36 | −8 | −7.9 | −7.26 |
| 20 | −48.57 | −136.11 | −8.26 | −7.32 | −7.2 | −6.76 |
| 30 | −49.73 | −140.34 | −8.15 | −6.64 | −6.5 | −6.26 |
| 40 | −37.08 | −100.08 | −8.05 | −5.96 | −5.8 | −5.76 |
| Mean | −37.44 | −99.19 | −8.2 | −6.98 | −6.85 | −6.51 |
The decline in ΔG values describes the spontaneity of the adsorption process. The negative ΔG and ΔH values represent the adsorption behaviour of E. ferox particles as a feasible, spontaneous and exothermic process. The decrease in ΔS values from −20.25 to −140.34 J mol−1 L−1 indicates diminishing randomness at the solid–solution interface following adsorption of the dye. Earlier studies on thermodynamic nature of dye adsorption state that under favourable conditions, both physisorption and chemisorption processes may occur simultaneously or alternately.42 Hence, ΔH values ranging from −14.398 to −49.73 kJ mol−1 K−1 suggests the probability of occurrence of both physisorption and chemisorption processes together in the adsorption of BF dye cations on E. ferox.3 Similar results have been reported when phenol adsorption on activated carbon was studied by Humpola et al.43
4 Conclusion
The results have shown that E. ferox residue biomass could be very effective in the removal of cationic dyes like basic fuchsin from aqueous medium. The material was stable in a wide range of pH and the zeta potential measurements indicated that the material develops a negative charge at pH > 1.0 and therefore is suitable for adsorptive removal of cations from water. In the present work, as basic fuchsin is unstable above pH 6.0, the experiments were done below this pH and the results are very promising.The interactions between the biomaterial and the dye cations have been shown to involve –OH groups of the material and NH/NH2 groups of the dye with likely participation of ![[double bond splayed left]](https://www.rsc.org/images/entities/char_e009.gif) CO groups of the E. ferox. The process has been found to be exothermic and spontaneous accompanied by considerable decrease in enthalpy, entropy and Gibbs energy of the process. Of the different kinetic models used, it is found that the interactions conform to a pseudo-second order model indicating that the dye cations may be held to the biomaterial surface through more than one site. The adsorption data fitted both Freundlich and Langmuir isotherms suggesting chemisorption of the dye cations on E. ferox followed by physisorption on the adsorbed layer.
CO groups of the E. ferox. The process has been found to be exothermic and spontaneous accompanied by considerable decrease in enthalpy, entropy and Gibbs energy of the process. Of the different kinetic models used, it is found that the interactions conform to a pseudo-second order model indicating that the dye cations may be held to the biomaterial surface through more than one site. The adsorption data fitted both Freundlich and Langmuir isotherms suggesting chemisorption of the dye cations on E. ferox followed by physisorption on the adsorbed layer.
Abbreviations
| E. ferox | Euryale ferox |
| qe | Adsorption capacity |
| Co | Initial concentration |
| Ce | Final concentration |
| V | Volume of solution |
| M | Mass of the adsorbent |
| qm | Monolayer adsorption capacity |
| b | Langmuir constant |
| RL | Hall constant |
| n | Heterogeneity factor |
| B | Temkin constant |
| qs | D–R monolayer adsorption capacity |
| ε | Polanyi potential |
| k1 | First order constant |
| k2 | Second order constant |
| α | Initial adsorption rate |
| β | Desorption constant |
| kp | Intraparticle diffusion rate constant |
| kfd | Liquid film diffusion constant |
| ΔH | Enthalpy |
| T | Absolute temperature |
| R | Gas constant |
| ΔS | Entropy |
| ΔG | Gibbs energy |
Acknowledgements
The authors are grateful to the Department of Science and Technology, Govt. of India and the Director of the Institute of Advanced Study in Science and Technology (IASST), Guwahati for financial support to execute this work. The authors are also obliged to thank the Physical Sciences Division and the Central Instrumentation facility of IASST for the help in different instrumentation work.References
- A. W. M. Ip, J. P. Barford and G. McKay, Chem. Eng. J., 2010, 157, 434–442 CrossRef CAS.
- A. M. Aljeboree, A. N. Alshirifi and A. F. Alkaim, Arabian J. Chem., 2014 DOI:10.1016/j.arabjc.2014.01.020.
- Y. Feng, F. Yang, Y. Wang, L. Ma, Y. Wu, P. G. Kerr and L. Yang, Bioresour. Technol., 2011, 102, 10280–10285 CrossRef CAS PubMed.
- Y. Tao, W. Z. Jian, J. Kui and L. Q. Jun, Chem. Res. Chin. Univ., 2006, 22, 292–296 CrossRef.
- V. K. Gupta, A. Mittal, V. Gajbe and J. Mittal, J. Colloid Interface Sci., 2008, 319, 30–39 CrossRef CAS PubMed.
- E. Bayram and E. Ayranci, Environ. Sci. Technol., 2010, 44, 6331–6336 CrossRef CAS PubMed.
- G. Crini, Bioresour. Technol., 2006, 97, 1061–1085 CrossRef CAS PubMed.
- Q. Sun and L. Yang, Water Res., 2003, 37, 1535–1544 CrossRef CAS PubMed.
- B. D. Bhole, B. Ganguly, A. Madhuram, D. Deshpande and J. Joshi, Curr. Sci., 2004, 86, 1641 CAS.
- Q. Shi, J. Zhang, C. Zhang, W. Nie, B. Zhang and H. Zhang, J. Colloid Interface Sci., 2010, 343, 188–193 CrossRef CAS PubMed.
- M. E. Haddad, J. Taibah Univ. Sci., 2015 DOI:10.1016/j.jtusci.2015.08.007.
- A. K. Verma, B. K. Banerji, D. Chakrabarty and S. K. Datta, Curr. Sci., 2010, 99, 795–800 CAS.
- R. J. Lan, J. T. Li and B. H. Chen, Int. J. Photoenergy, 2013, 2013, 1–7 CrossRef.
- Official methods of analysis of AOAC International, ed. W. Horwitz, Association of Analytical Communities, Gaithersburg, MD, USA, 17th edn, 1st revision, 2002 Search PubMed.
- A. O. Ayeni, F. K. Hymore, S. N. Mudliar, S. C. Deshmukh, D. B. Satpute, J. A. Omoleye and R. A. Pandey, Fuel, 2012 DOI:10.1016/j.fuel.2012.12.078.
- Technical Association of the Pulp and Paper Industry: Sampling and Preparing Wood for Analysis Technical Association of the Pulp and Paper Industry, TAPPI Standard T222 om-02, 2002.
- F. F. Avelar, M. L. Bianchi, M. Gonçalves and E. G. da Mota, Bioresour. Technol., 2010, 10, 4639–4645 CrossRef PubMed.
- R. Garcia, C. Pizarro, A. G. Lavin and J. L. Bueno, Bioresour. Technol., 2013, 139, 1–4 CrossRef CAS PubMed.
- O. Uner, U. Gecgel and Y. Bayrak, Water, Air, Soil Pollut., 2016, 227, 1–15 CrossRef CAS.
- N. Kohan, G. Machado, C. Jing, A. Nagardeolekar and B. M. Bujanovic, Energies, 2015, 8, 9640–9654 CrossRef.
- B. Cagnon, X. Py, A. Guillot, F. Stoeckli and G. Chambat, Bioresour. Technol., 2009, 100, 292–298 CrossRef CAS PubMed.
- A. Kumar and H. M. Jena, Results Phys., 2016, 6, 651–658 CrossRef.
- O. A. Ekpete and M. Horsfall, Res. J. Chem. Sci., 2011, 1, 10–17 CAS.
- R. Malik, D. S. Ramteke and S. R. Wate, Indian J. Chem. Technol., 2006, 13, 319–328 CAS.
- S. T. Akar, Y. Y. Balk, O. Tuna and T. Akar, Carbohydr. Polym., 2013, 94, 400–408 CrossRef CAS PubMed.
- O. S. Bello, K. A. Adegoke and O. O. Akinyunni, Appl. Water Sci., 2015 DOI:10.1007/s13201-015-0345-4.
- N. F. Cardoso, E. C. Lima, I. S. Pinto, C. V. Amavisca, B. Royer, R. B. Pinto, W. S. Alencar and S. F. P. Pereira, J. Environ. Manage., 2011, 92, 1237 CrossRef CAS PubMed.
- S. Baruah, A. Devi, K. G. Bhattacharyya and A. Sarma, Int. J. Environ. Sci. Technol., 2016 DOI:10.1007/s13762-016-1150-9.
- S. V. Gokhale, K. K. Jyoti and S. S. Lele, Bioresour. Technol., 2008, 99, 3600–3608 CrossRef CAS PubMed.
- K. G. Bhattacharyya, A. Sarma and J. Sarma, Adsorpt. Sci. Technol., 2010, 8 Search PubMed.
- J. X. Lin, S. L. Zhan, M. H. Fang, X. Q. Qian and H. Yang, J. Environ. Manage., 2008, 87, 193–200 CrossRef CAS PubMed.
- A. Arunarani, P. Chandran, B. V. Ranganathan, N. S. Vasanthi and S. S. Khan, Colloids Surf., B, 2013, 102, 379–384 CrossRef CAS PubMed.
- O. Aksakal and H. Ucun, J. Hazard. Mater., 2010, 181, 666–672 CrossRef CAS PubMed.
- M. J. Tempkin and V. Pyzhev, Acta Physicochim. URSS, 1940, 12, 217–222 Search PubMed.
- C. Aharoni and M. Ungarish, J. Chem. Soc., 1977, 73, 456–464 CAS.
- M. M. Dubinin and L. V. Radushkevich, Proceedings of the Academy of Sciences (USSR), 1947, 55, 331–333 Search PubMed.
- K. M. Rani, P. N. Palanisamy, S. Gayathri and S. Tamilselv, International Journal of Innovative Research in Science, Engineering and Technology, 2015, 4, 6845–6853 CrossRef.
- Y. Feng, H. Zhou, G. Liu, G. Qiao, J. Wang, H. Lu, L. Yang and Y. Wu, Bioresour. Technol., 2012, 125, 138–144 CrossRef CAS PubMed.
- S. Lagergren, K. Sven. Vetenskapsakad. Handl., 1898, 24, 1–39 Search PubMed.
- Y. Yao, F. Xu, M. Chen, Z. Xu and Z. Zhu, Bioresour. Technol., 2010, 101, 3040–3046 CrossRef CAS PubMed.
- J. Sarma, A. Sarma and K. G. Bhattacharyya, Ind. Eng. Chem. Res., 2008, 47, 5433–5440 CrossRef CAS.
- A. Dabrowski, Adv. Colloid Interface Sci., 2001, 93, 135–224 CrossRef CAS PubMed.
- P. Humpola, H. Odetti, A. Fertitta and J. Vicente, J. Chil. Chem. Soc., 2013, 58, 1541–1544 CrossRef CAS.
Footnote |
| † Electronic supplementary information (ESI) available. See DOI: 10.1039/c7ra03014b |
| This journal is © The Royal Society of Chemistry 2017 |