Open Access Article
Open Access ArticleDesign of ultrathin hybrid membranes with improved retention efficiency of molecular dyes†
Peng Liua,
Charles Millettoa,
Susanna Monti *b,
Chuantao Zhua and
Aji P. Mathew
*b,
Chuantao Zhua and
Aji P. Mathew *a
*a
aDivision of Materials and Environmental Chemistry, Stockholm University, Stockholm, 10691, Sweden. E-mail: aji.mathew@mmk.su.se
bCNR-ICCOM, Institute of Chemistry of Organometallic Compounds, Via G. Moruzzi 1, I-56124 Pisa, Italy. E-mail: sapeptides@gmail.com
First published on 11th September 2019
Abstract
Ultrathin layers of 2,2,6,6-Tetramethyl-1-piperidinyloxy (TEMPO) Oxidized Cellulose Nanofibers (TOCNF) embedded with Graphene Oxide nanosheets (GOs) in different ratios were built, via the blade coating technique, on a polyvinylidene difluoride (PVDF) substrate to obtain superior membranes for separating water pollutants from aqueous media. Cellulose nanofiber–graphene oxide hybrid materials have shown a great potential for water purification due to their active microporous structure with extended areas rich in negatively charged carboxyl functional groups capable of adsorbing positively charged contaminants efficiently. In contrast to the pristine free-standing TOCNF films, which are completely impermeable, the ultrathin (68 nm thick) hybrid coating with a 100![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1 TOCNF
1 TOCNF![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) GO ratio showed a stable water permeability (816 ± 3.4 L m−2 h−1 bar−1) higher than that of common polymeric membranes, and a very efficient size selectivity during filtration of water contaminated by various types of dyes. The membranes had high retention efficiency (82–99%) for dyes with hydrated radii greater than ≈0.5 nm due to the favorable combination of electrostatic/hydrophobic interactions with the hybrid matrices and steric entrapment controlled by the pore size. This was confirmed by theoretical calculations that revealed both the structure and dynamic behavior of the dyes in the complex environment of the membranes.
GO ratio showed a stable water permeability (816 ± 3.4 L m−2 h−1 bar−1) higher than that of common polymeric membranes, and a very efficient size selectivity during filtration of water contaminated by various types of dyes. The membranes had high retention efficiency (82–99%) for dyes with hydrated radii greater than ≈0.5 nm due to the favorable combination of electrostatic/hydrophobic interactions with the hybrid matrices and steric entrapment controlled by the pore size. This was confirmed by theoretical calculations that revealed both the structure and dynamic behavior of the dyes in the complex environment of the membranes.
1 Introduction
Over the last few decades, concern about environmental protection has led the scientific community to engage in a struggle for nature preservation by resorting to new eco-friendly production processes and damage repairing activities to reverse established eco-devastating trends. The general idea to become “greener” or more environmentally responsible is based mainly on conservation, wherever possible, sustainable use of non-renewable resources and reduction of pollution. In this respect, air and water are the most important targets. Contamination of air and water is jeopardizing the health of the population and represents a high risk hazard for all living organisms. Focusing on water, there are many types of contamination and several techniques have been developed that should still be improved upon to try to remove these poisons from ground and freshwater sources. Water, the universal solvent, should be transparent, odorless, tasteless and colorless. It should not contain toxic dyes. As a matter of fact, dyes are discharged into water and into the environment by various industries working, for example, in the paper, cosmetics, leather, and textiles sectors to name just a few, because dyes are part of the chemicals used to impart special properties to their products.1,2 Dyes are usually classified into anionic, cationic and non-ionic (neutral) molecules, are stable to light and resistant to biological degradation and aerobic digestion. Although chemical, physical, and biological methods are frequently used to remove dyes form industrial effluents, adsorption is considered the cheapest and most effective method compared to the all the available dye removal strategies.3 Adsorbents as activated carbon are efficient in dye removal, but their use is limited because of their extremely high cost.4 Purification based on membranes is another advanced energy proficient technology that allows dye removal from water in continuous operation with efficiency close to 99% under optimised conditions of pH and concentration.5 The major industrial challenges in membrane technology are, however, high cost, fouling and limited flux. According to Hagen–Poiseuille’s equation, the permeation flux is inversely proportional to the total distance of the liquid running through the membrane.6,7 Ideal membranes are, therefore, expected to have very thin film barriers with the appropriate pore size to maximize permeation flux at low pressure and obtain an excellent dye retention. Recently, a new type of thin-film nanocomposite membrane (TFN) containing ultrathin barriers with good separation efficiency have been developed by incorporating suitable nanofillers (e.g. nanofibers, nanoparticles and nanosheets) into the top selective layers of the porous support.8–11 The focus has, indeed, moved on to layered adsorption membranes made of nanoscaled adsorbents as zeolites, MOFs, graphene oxide and other compounds.12–19 Considering, for example, nanocellulose based materials, inspired by the concept of thin-film nanocomposites, both Cao et al.20 and Ma et al.9 introduced into the membranes thin TOCNF layers by casting and impregnating electrospun mats, which were prepared and characterized for ultrafiltration with pore sizes in the 10–100 nm range.9,20TEMPO-oxidised cellulose nanofibers (TOCNF) have been established as highly functional and biobased adsorbents for water purification due to their high specific surface area, abundant functionality, high strength and crystallinity.21–30 Moreover, TOCNF with small diameters (>10 nm) and high aspect ratios (>100) represent an ideal 1D nanomaterial for the fabrication of ultrathin films.21,23,31
In this work, we have combined TOCNF and GO to create thin film membranes with superior performance and here we report the results obtained by investigating the homogeneous deposition of TOCNF–GO ultrathin films (thickness < 100 nm) on a polyvinylidene difluoride (PVDF) microporous substrate (which was widely used for membrane distillation since it is intrinsically hydrophobic32) by the blade coating technique. As already observed, GO nanosheets are potential candidates for membrane barrier layers due to their unique 2D mono-atomic thick asymmetrical structure and the oxygen-functional groups on the edges of the basal plane. In addition, GO nanosheets have a high chemical resistance and can be easily dispersed and processed in liquid dispersions.33–35 Both GO and TOCNF have excellent compatibility and are connected through relatively strong intermolecular interactions.36–38 This was revealed by Zhu and co-workers who successfully prepared self-assembled TOCNF–GO biohybrids made of intermixed GO nanosheets and TOCNF with promising adsorption capacity towards Cu2+ ions.25
The doctor blade coating technique is easily scalable and can be industrially adapted for the fabrication of membranes with a fine control of the coating thickness. The contribution of the adsorption and size exclusion phenomenon by the membranes was studied focusing on selected anionic, cationic and neutral dyes, namely Victoria Blue B (VB), Rhodamine B (RB), Methyl Orange (MO), Rhodamine 6G (R6G), Methyl Blue (MB) and Methylene Blue (MnB) and correlated to membrane charge, composition and morphology. To support and interpret the experimental findings, quantum chemistry calculations combined with a classical conformational search procedure and reactive molecular dynamics simulations in the complex environment of a membrane were performed to disclose the dye structure and dynamics. The characteristics of the dyes that could be correlated with their entrapment, interaction with the matrices and motion in solution were analysed and linked with the experimental evidence.
2 Experimental
2.1 Materials
We prepared 2,2,6,6-tetramethyl-1-piperidinyloxy (TEMPO) mediated oxidised cellulose nanofibers (TOCNF) by dissolving cellulose fibers (Domsjö Fabriker AB, Örnskoldsvik, Sweden) according to the previously reported method by Saito et al.39 The cellulose pulp was firstly mechanically beaten, followed by TEMPO mediated oxidation (10 mmol hypochlorite per gram of pulp) and finally disintegration via homogenization. A water suspension of TEMPO CNF at 0.4 wt% was obtained after multiple-pass homogenization (two passes), using an APV 2000 homogeniser. Graphene oxide (GO) dispersion in water with a concentration of 4 mg mL−1 was purchased from Sigma-Aldrich (Sweden). Copper(II) nitrate hydrate, calcium chloride hydrate, cadmium nitrate hydrate, lead(II) nitrate, Victoria Blue B (VB, positive charge at pH 5.6), Rhodamine B (RB, neutral at pH 4.7), Methyl Orange (MO, negative charge at pH 5.8), Rhodamine 6G (R6G, positive charge at pH 3.9), Methyl Blue (MB, negative charge at pH: 6) and Methylene Blue (MnB, positive charge at pH 7.2) were all purchased from Sigma-Aldrich, Germany and used as received.2.2 Preparation of dispersions containing GO and TOCNF
The dispersions of GO and TOCNF were mixed using sonication (Q500 Sonicator, Qsonica) in deionized water for 5 minutes with different GO![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) TOCNF mass ratios (Table S1, ESI†). The concentration of TOCNF in all dispersions were the same (4 mg L−1), and combined with GO in mass ratios of 1
TOCNF mass ratios (Table S1, ESI†). The concentration of TOCNF in all dispersions were the same (4 mg L−1), and combined with GO in mass ratios of 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 100 (TOCNF–GO1), 5
100 (TOCNF–GO1), 5![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 100 (TOCNF–GO5) and 10
100 (TOCNF–GO5) and 10![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 100 (TOCNF–GO10).
100 (TOCNF–GO10).
2.3 Preparation of TOCNF–GO thin film nanocomposite membranes
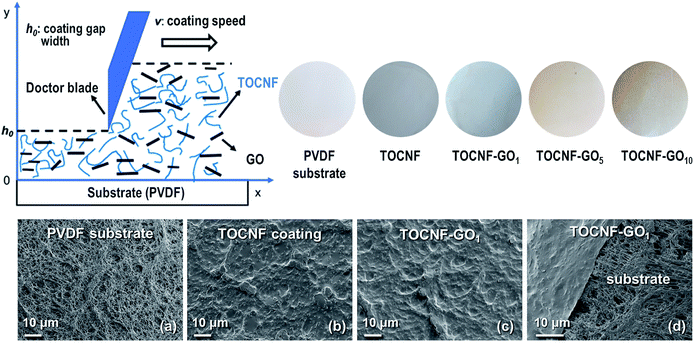
The TOCNF–GO membranes were prepared using a film applicator (Elcometer K4340M101 motorised film applicator, Elcometer Instruments, UK). The doctor blade gap width (h0) could be varied in the 10–200 μm range and the coating processing speed (v0) in the 0.5–10 cm s−1 range. The optimum h0 and v0 for the fabrication of TOCNF–GO membranes were investigated. TOCNF (4 mg L−1) and TOCNF–GO dispersions were coated on the porous polyvinylidene difluoride (PVDF) substrate (pore size: 0.45 μm, HVLP04700, Durapore Membrane Filter). After wet coating, all the membranes were dried overnight at ambient conditions.2.4 Characterisation
2.5 Molecular modeling
3 Results and discussion
3.1 Characterizations of TOCNF and GO
As displayed in Fig. 1-left, the diameters of the oxidised cellulose nanofibers were found to be in the 1–3 nm range and their lengths varied from several hundred nanometers to micrometers. As it can be noticed by inspecting Fig. 1-left, the thickness of the GO layer is rather uniform (around 1 nm), whereas the lateral width is more variable, ranging from hundreds of nanometers to several micrometers, which is significantly larger than the diameter of the TOCNF fibers. Both of these nanoscale components had very high theoretical specific surface areas (SSA), which were in the range 888–2666 m2 g−1 in the case of TOCNF and approximately 2039 m2 g−1 for GO. The zeta potentials of both TOCNF and GO were tendentially negative and remained such in the whole pH range examined in this study. The most negative values were found at neutral pH (around 8), due to the high population of the acidic charged groups (carboxyl groups) that were present on both TOCNF and GO (Fig. 1-right). Inspecting the trends shown in the figure it is apparent that TOCNF had the highest negative zeta potential (−51 mV) at neutral pH, whereas at pH = 10 the zeta potential became less negative (−26 mV) and it was further reduced to −9.7 mV at pH = 2. These sensitive variations were not observed, instead, in the case of GO where the explored zeta potential range was narrower (only 9 mV compared to 41 mV), the minimum and maximum values were found at pH = 10 (−38 mV) and pH = 1.9 (−29 mV), respectively.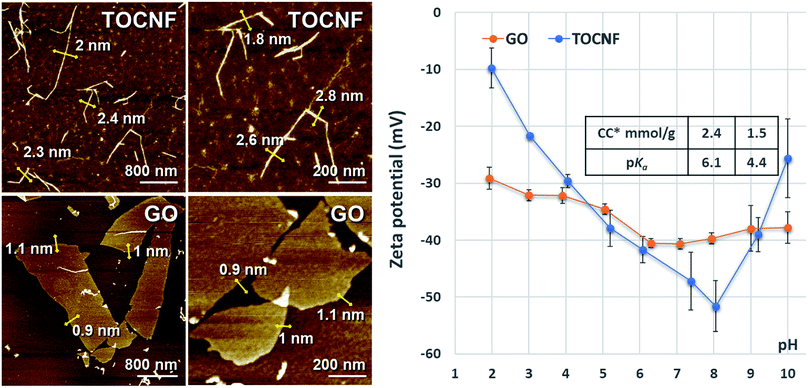 | ||
| Fig. 1 Characterization of TOCNF and GO. (Left) Morphologies detected using AFM; (Right) charge contents (CC), pKa of the acidic functional groups and the zeta potentials as a function of pH. | ||
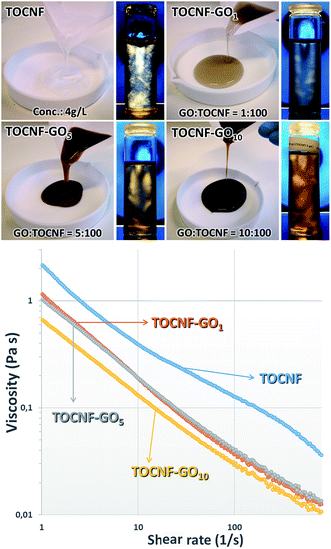
3.2 Rheological characterizations of TOCNF–GO mixed dispersions
Considering that rheological properties of dispersions are crucial when the blade coating technique is used for fabricating the membranes, they were examined in detail and presented qualitatively, as photographs (Fig. 2-top) and quantitatively, through the plot of the shear viscosity as a function of the shear rate (Fig. 2-bottom).The images (Fig. 2-top) clearly suggest that both GO and TOCNF formed stable dispersions at various mass ratios, because of the repulsive interactions resulting from the highly charged surfaces. The flow birefringence photos demonstrate that the dispersions of GO nanosheets and TOCNF nanofibers resulted in stable colloidal systems without any macroscopic aggregates and had a tendency to align in a chiral nematic liquid crystal phase. Indeed, flow birefringence is the double refraction of light that passes through a transparent, molecularly ordered medium and arises from the orientation-dependent differences in the refractive index originated in various regions within the dispersion. As shown in Fig. 2-top, TOCNF (4 g L−1) was a gel-like substance almost transparent, whereas the TOCNF–GO10 dispersion, which contained the highest GO concentration, was dark brown and significantly less viscous than the other samples. It can also be noted that the TOCNF suspension showed the presence of a birefringent glassy phase (remarkable birefringence without flow) with high birefringence intensity (which could be deduced from the whiteness of the areas), whereas the flow birefringence patterns observed in the GT dispersions (especially at higher GO concentrations) displayed some birefringent oriented elliptical domains of a few millimeters with a remarkable distinction direction. However, the different alignments of the individual domains suggest that the global distribution of the orientations is random. Birefringence occurred in the whole area of the dispersions.
As is evident from Fig. 2-bottom, the TOCNF–GO dispersions displayed non-Newtonian shear-thinning (pseudoplastic) behavior, indicating the formation of a network structure between two GO and TOCNF components. This was realized through hydrogen bonding interactions between the hydroxyl, epoxy, carboxyl groups and oxygen species available on both interfaces. The marked decrease in viscosity of the TOCNF–GO dispersions with the increase in the shear rates is consistent with the rheological characteristics of both GO and TOCNF,58,59 whereas the drastic reduction of the shear viscosity with the raise in GO content at all shear rates could be ascribed to a weakening of the interactions between the GO nanosheets and the TOCNF fibers (most probably due to disruption of the intermolecular hydrogen bond network involving the carboxyl groups) and to a heterogeneous distribution of GO in the TOCNF matrices. Indeed, it could be speculated that GO–TOCNF interactions prevailed over GO–GO self-aggregations at low GO content, favouring the formation of GO–TOCNF layered structures uniformly dispersed in the TOCNF matrix, whilst when the percentage of GO increased the GO self-interaction contribution became equally important and stacked GO aggregates could be found in various orientations in relation to the TOCNF fibers. This disorganization of the layers could induce a sort of partial collapse of the compact network structure, reorganization in a more amorphous 3D random arrangement, and thus an increase in flow with a consequential decrease in viscosity.25,60
3.3 Membrane fabrication coating process
The dispersions were coated on the porous substrate using the doctor blade coating technique as presented in the scheme depicted in Fig. S1.† The blade gap width (h0) and the coating speed (v0) are the two most vital processing parameters that have a substantial impact on the structure and, therefore, on the performance of the fabricated membrane. The blade gap width has a positive linear correlation with the coated film thickness and coating grammage. Thus, to investigate the optimal processing parameters, the performance of the TOCNF membrane as a function of h0 and v0 was evaluated by measuring the retention and solution flux when Victoria Blue B (VB), Rhodamine B (RB) and Methyl Orange (MO) dyes were present in solution (feed concentration of 10 mg L−1). The thickness, d, of the dried coated film was calculated from the empirical relationship:61where h0 (μm) is the blade gap width, c is the concentration of the dispersion (g cm−3), and ρ is the material density (the densities of GO and TOCNF are 0.981 g cm−3 and 1.46 g cm−3, respectively62). The optimum parameters identified in the preliminary investigations, namely blade gap width h0 = 50 μm and coating speed v0 = 0.5 cm s−1, were used for the fabrication of the TOCNF–GO membranes. The nomenclature and processing parameters of the thin film nanocomposite membranes are reported in Table S1 (ESI).† Both photographs and SEM images (Fig. 3) revealed the homogeneity of the thin densely coated layer and the surface structure of the microporous substrate beneath it, which is partially visible in the SEM images (Fig. 3(d)). The image highlights the ultralow thickness of the coating and the tight contact between the coated layer and substrate. The surface structure of the TOCNF–GO1 membrane was similar to that of the pristine TOCNF one (Fig. 3(b)). The low grammage (0.1 g m−2) TOCNF–GO1 thin film coatings correspond to a coating thickness of approximately 68 nm.
3.4 Filtration performance
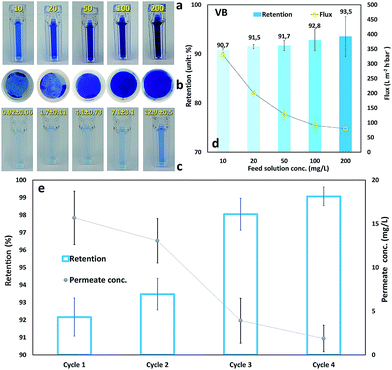
Water permeability of the membranes with different GO dosages was tested through three consecutive filtrations of deionized water and the results are displayed in Fig. 4(a). The permeability of the TOCNF membrane decreased sharply and continuously from 1515 to 571 L m−2 h−1 bar−1.21 Both the increase in film thickness and the narrowing of the water channels, due to nanofiber swelling, lead to the fast decrease in water permeability in line with the studies of Hakalahti and co-workers that justify this behavior considering the hydrophilic nature of the matrix, the abundance of water binding functional groups and the thickness of free-standing TOCNF films.63 On the one hand, TOCNF–GO coated membranes exhibited stable water permeability (Fig. 4), which showed that the added GO nanosheets could function as rigid skeletons inside the TOCNF matrix structure. This prevented swelling and contributed to the structural stability of the pores (Fig. 4(b)). On the other hand, a high amount of GO was detrimental for the permeability of water as they could narrow or even seal some water channels. Indeed, when the GO mass ratio increased from 1 to 10, water permeability dropped sharply from 812 to 82 L m−2 h−1 bar−1. Among the examined membranes, the TOCNF–GO1 one had the best water permeability performance with a good balance between mechanical stability and high water permeation. It exhibited an incomparably high permeability in relation to previous studies as well, due to its extremely low thickness (68 nm) and swelling resistant structure. According to the Hagen–Poiseuille equation, the permeation flux is inversely proportional to the permeation distance, implying that a separation layer should be as thin as possible to maximize the flux.7 Free-standing TOCNF films traditionally prepared through vacuum filtration, or casting, had their thickness in a range of dozens of microns and simply exhibited no water permeability.64 It was reported, in fact, that for a 20 μm thick TOCNF film with excellent gas barrier properties, the oxygen permeability was 1 mL per m2 per day per Pa.21,62 To the best of our knowledge, this is the first study describing the preparation of highly permeable TOCNF based membranes by means of the doctor blade coating technique. In addition, this study also found that the addition of 1 wt% GO could greatly inhibit swelling and packing of the membranes, which is a major improvement in relation to TOCNF membranes. Given these results we opted for the TOCNF–GO1 membrane for further characterizations.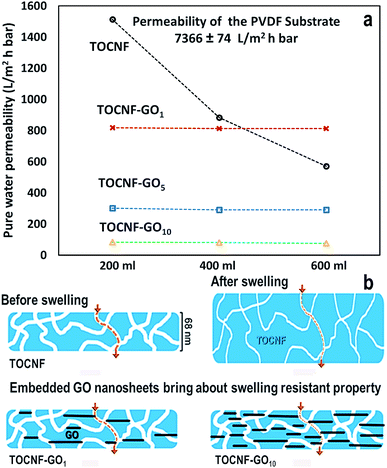 | ||
| Fig. 4 (a) Water permeability derived from three consecutive permeability measurements with the same conditions. (b) Schematic view for percolation through the ultrathin coated film. | ||
3.5 Dye retention performance
 | ||
| Fig. 5 (Top) Retention performance of TOCNF–GO1 membrane as a function of hydrated radius for the probe dyes (feed concentration: 10 mg L−1, each measurement was repeated three times). The hydrated radius of MO, RB, MB, MnB34 and R6G65 was found in the literature. The hydrated radius of VB was estimated from SASA calculations; (Bottom) photos of feed and permeate solutions filtered through TOCNF–GO1 membrane. | ||
A closer inspection of the structure of the dyes (Fig. S1, ESI†) evidenced that the presence of many conjugated rings reduced the flexibility of the molecules, and their whole size had only negligible variation inside the small populations of conformers. A few more considerations regarding the dyes structures in relation to their shape could also be useful to identify possible correlations of these properties with the membrane retention mechanism. To this aim, the radius of gyration (Rgy), which closely reflects the conformation of the dye and hydrated radius (Rh), which reflects the apparent size adopted by the solvated, tumbling, molecule, reported in Table S2 (ESI†) can be considered representative values of each species. In Table S2 (ESI),† the ratio of Rgy and Rh (Rgy/Rh), which could provide shape information is also reported. It was observed, indeed, that globular structures had a characteristic ratio Rgy/Rh around 0.78 (Rgy < Rh),67 whereas in the case of non-spherical or elongated structures the ratio was higher (Rgy > Rh). The ratios in Table S2 (ESI)† suggest that the rhodamines and Victoria blue B have almost spherical shapes, whereas the other three dyes are more elongated, which is consistent with the shapes shown in Fig. S1 (ESI).† These features could be important in the design of the shape of the membrane pores especially in view of the dynamic tumbling of the molecules. To explain further the behaviour of the dyes during their adsorption on the supports, apart from their size, the electrostatic character was taken into account. In Fig. S2 (ESI),† the MEP of R6G, which is a positively charged molecule (+1), is displayed, on the top-right side of the figure, just to show the effect of the molecular charge on the MEP visualization. Thus, to identify the groups of this dye that could be involved in recognition and binding to the surface sites and disclose the nature of the possible interactions, two types of possible neutralization were simulated: (1) deprotonation of one of the nitrogens (top-left image); (2) deprotonation of both of the nitrogens and protonation of the ring oxygen. As can be noted, these two choices influence the whole charge distribution but a few features remain almost unaltered and independent of the modified site. These are the potential of the carbonyl oxygen, which is strongly negative, the negative potential on the planar conjugated rings, and the slightly positive potential of the side chains. Moving to RB, which is the only neutral molecule, similar areas are identified suggesting that the most effective binding mode could be through H-bonding interactions between the carbonyl oxygen (and/or the π-density of the rings) and the hydroxyl groups of the surface complemented with the stacking of the dye and GO sheet rings. Regarding MB (Fig. S3, ESI†), it is apparent that after neutralization the MEP becomes prevalently positive suggesting it favorable adsorption on the negatively charged portion of the TOCNF–GO support. On the contrary, MO shows a remarkable dipolar character with a dominant negative potential that suggests a weaker binding to the substrate, probably perpendicular to its surface. As far as the VB MEP is concerned, it could be speculated that the redistribution of the charge upon adsorption should favor flat orientations and formation of stacked aggregates.
| Coated film mass (mg) | 0.146 | |||
| Cycle 1 | Cycle 2 | Cycle 3 | Cycle 4 | |
| Dye rejection mass (mg) | 1.1 | 2.2 | 3.4 | 4.6 |
| Dye rejection capacity (mg g−1) | 7600 | 15![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 300 300 |
23![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 300 300 |
31![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 500 500 |
3.6 MD simulation results
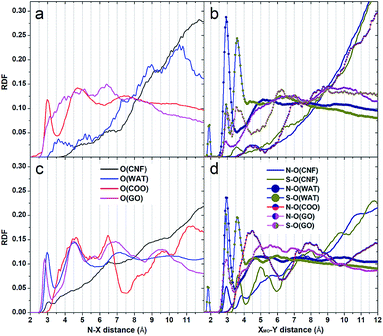
Radial distribution functions. The adsorption of the dyes on the substrate was confirmed by the presence of sharp peaks at short distances in the radial distribution functions between the atoms of the dyes and the oxygen atoms of the supports that were the principal contact points between the adsorbates and the interfaces (Fig. 7). Even though at the beginning of the simulations all the dyes were relatively far from the nanocomposite substrates and quite close to one another, the sampling time was sufficient to observe their migration, adsorption on the interfaces and their marked tendency to self-assemble.
Inspection of the VB RDF nitrogen plots in Fig. 7a–c indicates the involvement of these atoms in hydrogen bonding interactions with the hydroxyl groups of the supports when the GO sheets do not cover completely the fiber interface (Fig. 7c – black peaks centered at around 2.8 Å), whereas when GO molecules laid on the TOCNF fiber, the nitrogens remained far from the cellulose matrix and were instead engaged in hydrogen bonding interactions with the adsorbed GO layers. In contrast, in more porous structures where the sheets were inclined in relation to the fiber, a number of hydrogen bonded structures appeared and included adsorption of the dyes on GO. Indeed, the magenta curve, visible in the RDF graphs, confirms the existence of VB–GO complexes, which were mainly realized through stacking or T-shaped interactions of the rings and hydrogen bonds. The peaks corresponding to the carboxyl groups of TOCNF were found, instead, at longer distances (broad peaks at about 4 Å – Fig. 7c) when the GO sheets were inclined, whereas in the case of the GO flat adsorption a few interactions at short distances with the carboxyl atoms were noticed. As far as the solvation of the dye is concerned, the blue plots indicate water molecules directly hydrogen bonded to the VB nitrogens (peaks at 2.8 Å). These are present in both the simulations but when the structure was more packed, namely GO flat adsorption, the whole molecular arrangement prevented water from being directly connected to the dye. The second water shell was located at about 4.7 Å and the solvent was exchanged between the two shells. Moving to the MO RDFs, it was found that both the N atoms and the SO3 groups were well surrounded by water molecules displaying sharp peaks at around 2.8 and 3.8 Å, respectively, whereas considering the hydroxyl oxygen of TOCNF these distances were slightly longer (2.9 Å for N) and shorter (3.3 Å for S), respectively. Only SO3 was found close to the carboxyl groups (sharp peak at 3.6 Å), but not the nitrogen, which seemed located at around 5.1 Å. When TOCNF was covered with GO, the carboxyl groups were less exposed and the dyes were essentially adsorbed onto the GO planes even though, due to their size and shape, they could reach more easily than VB the hydroxyl groups of the TOCNF substrate. The shape and position of the peaks suggest that MO could adopt most probably vertical orientations relative to the support and be in touch with it just with one of the terminus moieties, primarily the N terminus. This agrees perfectly with the characteristic positive MEP potential of the molecule in that portion (Fig. S4†) and to the typical negatively charged tendency of the TOCNF interface. As far as the location of the Cl− and Na+ counterions are concerned, they were found prevalently close to the nitrogen atoms of VB and coordinated to the SO3 moiety of MO.
Spatial distribution functions. To complement the RDF description, three dimensional spatial distribution functions were also analyzed and used to identify the most populated locations of the nitrogen, sulfur and oxygen atoms of the dyes during the production dynamics. This was very useful to characterize more deeply their adsorption on the cellulose based support (Fig. S5–S8, ESI†). A contour density 1.5 times larger than the average solvent density was chosen to reveal the most visited sites on the GO–TOCNF interfaces considering the last portion (100 structures) of the production trajectory. VB SDFs, shown in Fig. S5 and S6 (ESI),† indicate that in the case of a layer model (where the matrix is more compact – Fig. S5†), the dye molecules were stably adsorbed on the support (N blue contours located close to the supports) in an almost flat orientation and they could interact with both the adsorbed GO molecules and small portions of the TOCNF fibers, remaining essentially bound to specific surface sites because of the entrapping effects at the sheets boundaries. Their rings were found mainly in stacking orientation with respect to the GO planes. Such a behavior could also be due to the redistribution of the negative charge on the substrate that perturbed not only the molecular orientation but also the locations of the counterions. Then, cooperative effects could drive the molecules towards different regions on the surface and also induce the migration of counterions into the solvent (green areas extending into the solvent). Comparison with the more porous model (Fig. S6, ESI†), where the GO sheets were inclined relatively to the fiber surface, revealed that the mobility of the dye, there, was markedly increased, due to their possible reorganization inside the created nanopore structures where solvent molecules are also present. A complete different scenario emerges, instead, from the inspection of the MO SDF profiles (Fig. S7 and S8, ESI†). The main characteristic features of the MO adsorption were a marked tendency to self-assemble and to adopt a great variety of alignments relative to the support. This happened in solution and on the surface. Self-assembly was realized by means of stacking and T-shaped interactions of the rings and interconnection of the SO3 moieties with interposed Na+ ions (mediators). This is clearly visible in Fig. S7 and S8.† The sparse distribution of the densities indicates greater mobility of the dye and a marked tendency to change frequently its orientation in relation to the support. The SO3 moieties could be found almost in touch with the interface when the Na+ counterions were close by. However, the general tendency of MO to interact with the support through its terminus groups adopting different types of arrangement was confirmed. This was easily realized because of the size and shape of the molecule and the dipolar character of its MEP potential (Fig. S4, ESI†). A similar scenario appears in the case of GO sheets in inclined orientations (Fig. S8, ESI†). It is evident that the MO molecules could be entrapped among the sheets and the TOCNF surface; they could self-assemble but also cross-link the TOCNF chains through the mediating actions of the counterions. In summary, the simulations results suggest that the lower retention of MO could be due to a combination of dye properties: first its negatively charged character during the recognition process (driving force at long range when the molecule was far from the interface), and then, its strongly dipolar MEP when it was neutralized by the surrounding environment upon binding (counterions). Size, shape and mobility affected MO binding and retention on GO–TOCNF as well. Indeed, comparison of the MEP of the other dyes, which seemed more stably adsorbed on the composite according to the retention data, suggests that more alternate positive–negative potential regions and the prevalence of positive MEP regions were preferred. In terms of molecular group, size and shape, the data suggested that condensed ring and branched conformations (such as the MB one) were more preferentially entrapped than the other more flexible species and that, besides the charge, these features played an important role in adsorption and retention.
4 Conclusion
In this study, we have successfully demonstrated that an ultrathin (<100 nm) TOCNF barrier film embedded with GO nanosheets can be built on a porous substrate by means of the doctor blade coating technique. Due to its nanoscale thickness and to the presence of GO nanosheets, the nanofiltration membrane exhibited high stability and excellent water permeability (816 ± 3.4 L m−2 h−1 bar−1), which had superior properties compared to previously reported nanocellulose based membranes. The new membrane showed an efficient and effective separation capability (including both high retention and permeation flux) of molecules with MWCO of 0.5 kDa and revealed a stable filtration performance. The rejection mechanism of dyes was governed by size, shape, stacking, charge and concentration of the molecules in water solution, at optimised pH conditions. The TOCNF–GO ultrathin barrier film was capable of adsorbing and storing various types of molecular dyes with different characteristic structure and charge nature. The modeling studies revealed that the molecules could be stably adsorbed on the hybrid supports both through intermolecular hydrogen bond and stacking (or T-shaped) interactions of their rings with the GO planes. Dye self-assembly phenomena in solution and on the supports were also observed and suggested that even though the hydrodynamic radius of some dyes was too small to guarantee their entrapment, their aggregation tendency could disfavor their release in solution. Thus, retention could also be effective due to the formation of aggregate structures. In summary, we have designed a scalable eco-friendly charged membrane with good permeability for water purification that can be further extended to applications involving ion permeation.Conflicts of interest
There are no conflicts to declare.Acknowledgements
We acknowledge the financial support from Swedish Research Council (VR, grant no: 621-2013-5997 and 2017-04254) and Knut and Alice Wallenberg Foundation under Wallenberg Wood Science Centre (WWSC). We thank Fabian Andreas Araoz (Chemical Engineering and Technology, Royal Institute of Technology) for the film applicator (Electrometer 4340). SM is grateful to Adri C. T. van Duin for the standalone version of ReaxFF, for his collaboration and support. SM thanks the SCM experienced team for their technical support during the various stages of this investigation.References
- R. Xiong, H. S. Kim, S. Zhang, S. Kim, V. F. Korolovych, R. Ma, Y. G. Yingling, C. Lu and V. V. Tsukruk, ACS Nano, 2017, 11, 12008–12019 CrossRef CAS.
- X. Yang, Y. Du, X. Zhang, A. He and Z.-K. Xu, Langmuir, 2017, 33, 2318–2324 CrossRef CAS.
- R. V. Kandisa, K. V. N. Saibaba, K. B. Shaik and R. Gopinath, J. Biorem. Biodegrad., 2016, 7, 1000371 Search PubMed.
- V. Katheresan, J. Kansedo and S. Y. Lau, Chem. Eng. J., 2018, 7, 4676–4697 Search PubMed.
- D. Karisma, G. Febrianto and D. Mangindaan, IOP Conf. Ser.: Earth Environ. Sci., 2018, 195, 012057 CrossRef.
- X. Peng, J. Jin, Y. Nakamura, T. Ohno and I. Ichinose, Nat. Nanotechnol., 2009, 4, 353–357 CrossRef CAS.
- S. J. Gao, H. Qin, P. Liu and J. Jin, J. Mater. Chem. A, 2015, 3, 6649–6654 RSC.
- J. Wang, P. Zhang, B. Liang, Y. Liu, T. Xu, L. Wang, B. Cao and K. Pan, ACS Appl. Mater. Interfaces, 2016, 8, 6211–6218 CrossRef CAS.
- H. Ma, C. Burger, B. S. Hsiao and B. Chu, J. Membr. Sci., 2014, 454, 272–282 CrossRef CAS.
- M. J. Penna, M. Mijajlovic and M. J. Biggs, J. Am. Chem. Soc., 2014, 136, 5323–5331 CrossRef CAS.
- H. Wu, B. Tang and P. Wu, J. Membr. Sci., 2013, 428–433, 425 CrossRef.
- H. Ting, H.-Y. Chi, C. H. Lam, K.-Y. Chana and D.-Y. Kang, Environ. Sci.: Nano, 2017, 4, 2205–2214 RSC.
- H.-Y. Chi, S.-H. Hung, M.-Y. Kan, L.-W. Lee, C.-H. Lam, J.-J. Chen and D.-Y. Kang, CrystEngComm, 2018, 20, 5465–5474 RSC.
- L. Yang, Z. Wang and J. Zhang, J. Membr. Sci., 2017, 532, 76–86 CrossRef CAS.
- Q. Xie, S. Zhang, Z. Hong, H. Ma, B. Zeng, X. Gong, W. Shao and Q. Wang, Chem. Eng. J., 2019, 368, 186–201 CrossRef CAS.
- Q. L. Xie, W. Y. Shao, S. S. Zhang, Z. Hong, Q. Q. Wang and B. R. Zeng, RSC Adv., 2017, 7, 54898–54910 RSC.
- G. S. Lai, W. J. Lau, P. S. Goh, A. F. Ismail, N. Yusof and Y. H. Tan, Desalination, 2016, 387, 14–24 CrossRef CAS.
- J. Wang, C. W. Zhao, T. Wang, Z. Wu, X. Li and J. S. Li, RSC Adv., 2016, 6, 82174–82185 RSC.
- E. D. Smith, K. D. Hendren, J. V. HaagIV, E. J. Foster and S. M. Martin, Nanomaterials, 2019, 9, 125 CrossRef.
- X. Cao, M. Huanga, B. Ding, J. Yu and G. Sun, Desalination, 2013, 316, 120–126 CrossRef CAS.
- A. Isogai, T. Saito and H. Fukuzumi, Nanoscale, 2011, 3, 71–85 RSC.
- H. Sehaqui, U. P. de Larraya, P. Liu, N. Pfenninger, A. P. Mathew, T. Zimmermann and P. Tingaut, Cellulose, 2014, 21, 2831–2844 CrossRef CAS.
- H. Ma, C. Burger, B. S. Hsiao and B. Chu, ACS Macro Lett., 2012, 1, 723–726 CrossRef CAS.
- H. Voisin, L. Bergström, P. Liu and A. P. Mathew, Nanomaterials, 2017, 7, 57–76 CrossRef.
- C. Zhu, P. Liu and A. P. Mathew, ACS Appl. Mater. Interfaces, 2017, 9, 21048–21058 CrossRef CAS.
- P. Liu, K. Oksman and A. P. Mathew, J. Colloid Interface Sci., 2016, 464, 175–182 CrossRef CAS.
- A. Mautner, H. A. Maples, H. Sehaqui, T. Zimmermann, U. P. de Larraya, A. P. Mathew, C. Y. Lai, K. Li and A. Bismarck, Environ. Sci.: Water Res. Technol., 2016, 2, 117–124 RSC.
- S. Hokkanen, E. Repo, T. Suopajärvi, H. Liimatainen, J. Niinimaa and M. Sillanpää, Cellulose, 2014, 21, 1471–1487 CrossRef CAS.
- J. Wang and C. Chen, Biotechnol. Adv., 2009, 27, 195–226 CrossRef CAS.
- B. Volesky, Water Res., 2007, 41, 4017–4029 CrossRef CAS PubMed.
- R. Wang, S. Guan, A. Sato, X. Wang, Z. Wang, R. Yang, B. S. Hsiao and B. Chu, J. Membr. Sci., 2013, 446, 376–382 CrossRef CAS.
- K. W. Lawson and D. R. Lloyd, J. Membr. Sci., 1997, 124, 1–25 CrossRef CAS.
- H. Huang, Y. Ying and X. Peng, J. Mater. Chem. A, 2014, 2, 13772–13782 RSC.
- A. Akbari, P. Sheath, S. T. Martin, D. B. Shinde, M. Shaibani, P. C. Banerjee, R. Tkacz, D. Bhattacharyya and M. Majumder, Nat. Commun., 2016, 7, 10891 CrossRef CAS PubMed.
- P. Sun, M. Zhu, K. Wang, M. Zhong, J. Wei, D. Wu, Z. Xu and H. Zhu, ACS Nano, 2013, 7, 428–437 CrossRef CAS.
- X. Wei, T. Huang, J. H. Yang, N. Zhang, Y. Wang and Z. W. Zhou, J. Hazard. Mater., 2017, 335, 28–38 CrossRef CAS.
- Q. Fang, X. Zhou, W. Deng, Z. Zheng and Z. Liu, Sci. Rep., 2016, 6, 33185 CrossRef.
- M. S. Sajab, C. H. Chia, C. H. Chan, S. Zakaria, H. Kaco, S. W. Chook, S. X. China and A. M. Noor, RSC Adv., 2016, 6, 19819–19825 RSC.
- T. Saito, S. Kimura, Y. Nishiyama and A. Isogai, Biomacromolecules, 2007, 8, 2485–2491 CrossRef CAS.
- M. J. Vainio and M. S. Johnson, J. Chem. Inf. Model., 2007, 47, 2462–2474 CrossRef CAS.
- J. S. Puranen, M. J. Vainio and M. S. Johnson, J. Comput. Chem., 2010, 31, 1722–1732 CAS.
- M. J. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuseria, M. A. Robb, J. R. Cheeseman, G. Scalmani, V. Barone, B. Mennucci, G. A. Petersson, H. Nakatsuji, M. Caricato, X. Li, H. P. Hratchian, A. F. Izmaylov, J. Bloino, G. Zheng, J. L. Sonnenberg, M. Hada, M. Ehara, K. Toyota, R. Fukuda, J. Hasegawa, M. Ishida, T. Nakajima, Y. Honda, O. Kitao, H. Nakai, T. Vreven, J. A. Montgomery Jr, J. E. Peralta, F. Ogliaro, M. Bearpark, J. J. Heyd, E. Brothers, K. N. Kudin, V. N. Staroverov, R. Kobayashi, J. Normand, K. Raghavachari, A. Rendell, J. C. Burant, S. S. Iyengar, J. Tomasi, M. Cossi, N. Rega, J. M. Millam, M. Klene, J. E. Knox, J. B. Cross, V. Bakken, C. Adamo, J. Jaramillo, R. Gomperts, R. E. Stratmann, O. Yazyev, A. J. Austin, R. Cammi, C. Pomelli, J. W. Ochterski, R. L. Martin, K. Morokuma, V. G. Zakrzewski, G. A. Voth, P. Salvador, J. J. Dannenberg, S. Dapprich, A. D. Daniels, O. Farkas, J. B. Foresman, J. V. Ortiz, J. Cioslowski and D. J. Fox, Gaussian 09, Revision A.02, Gaussian Inc., Wallingford CT, 2009 Search PubMed.
- A. Akbari, P. Sheath, S. T. Martin, D. B. Shinde, M. Shaibani, P. C. Banerjee, R. Tkacz, D. Bhattacharyya and M. Majumder, Nat. Commun., 2016, 7, 10891 CrossRef CAS.
- J. S. Murray and P. Politzer, Wiley Interdiscip. Rev.: Comput. Mol. Sci., 2011, 1, 153–163 CAS.
- L. Liu, L. Miao, L. Li, F. Li, Y. Lu, Z. Shang and J. Chen, J. Phys. Chem. Lett., 2018, 9, 3573–3579 CrossRef CAS.
- P. Politzer and J. S. Murray, Rev. Comput. Chem., 2007, 2, 273–312 Search PubMed.
- G. S. Remya and C. H. Suresh, Phys. Chem. Chem. Phys., 2016, 18, 20615–20626 RSC.
- Y. Okita, T. Saito and A. Isogai, Biomacromolecules, 2010, 11, 1696–1700 CrossRef CAS.
- A. K. Malde, L. Zuo, M. Breeze, M. Stroet, D. Poger, P. C. Nair, C. Oostenbrink and A. E. Mark, J. Chem. Theory Comput., 2011, 7, 4026–4037 CrossRef CAS.
- Z. Yanwu, M. Shanthi, C. Weiwei, L. Xuesong, J. W. Suk, J. R. Potts and R. S. Ruoff, Adv. Mater., 2010, 22, 3906–3924 CrossRef.
- A. Paajanen and J. Vaari, Cellulose, 2017, 24, 2713–2725 CrossRef CAS.
- L. Yuanyuan, Z. Hongli, Z. Shuze, W. Jiayu, L. Zhen, O. Vaaland, S. Lacey, F. Zhiqiang, D. Hongqi and H. L. L. Teng, NPG Asia Mater., 2015, 7, e150 CrossRef.
- N. V. Medhekar, A. Ramasubramaniam, R. S. Ruoff and V. B. Shenoy, ACS Nano, 2010, 4, 2300–2306 CrossRef CAS.
- J. L. Achtyl, R. R. Unocic, L. Xu, Y. Cai, M. Raju, W. Zhang, R. L. Sacci, I. V. Vlassiouk, P. F. Fulvio, P. Ganesh, D. J. Wesolowski, S. Dai, A. C. T. van Duin, M. Neurock and F. M. Geiger, Nat. Commun., 2015, 6, 6539 CrossRef CAS.
- S. K. Singh, S. G. Srinivasan, M. Neek-Amal, S. Costamagna, A. C. T. van Duin and F. M. Peeters, Phys. Rev. B, 2013, 87, 104114 CrossRef.
- E. C. Neyts, A. C. T. van Duin and A. Bogaerts, Nanoscale, 2013, 5, 7250–7255 RSC.
- SCM, Theoretical Chemistry, Vrije Universiteit, Amsterdam, The Netherlands, http://www.scm.com Search PubMed.
- Z. Xu and C. Gao, ACS Nano, 2011, 5, 2908–2915 CrossRef CAS.
- R. Jalili, S. H. Aboutalebi, D. Esrafilzadeh, R. L. Shepherd, J. Chen, S. Aminorroaya-Yamini, K. Konstantinov, A. I. Minett, J. M. Razal and G. G. Wallace, Adv. Funct. Mater., 2013, 23, 5345–5354 CrossRef CAS.
- B. Wang, W. Lou, X. Wanga and J. Hao, J. Mater. Chem., 2012, 22, 12859–12866 RSC.
- F. C. Krebs, Sol. Energy Mater. Sol. Cells, 2009, 93, 394–412 CrossRef CAS.
- H. Fukuzumi, T. Saito, T. Iwata, Y. Kumamoto and A. Isogai, Biomacromolecules, 2009, 10, 162–165 CrossRef CAS.
- M. Hakalahti, M. Faustini, C. Boissière, E. Kontturi and T. Tammelin, Biomacromolecules, 2017, 18, 2951–2958 CrossRef CAS.
- A. Mautner, K.-. Lee, T. Tammelin, A. P. Mathew, A. J. Nedoma, K. Li and A. Bismarck, React. Funct. Polym., 2015, 86, 209–214 CrossRef CAS.
- I. I. Vlasov, A. A. Shiryaev, T. Rendler, S. Steinert, S.-. Lee, D. Antonov, M. Voros, F. Jelezko, A. Fisenko, L. F. Semjonova, J. Biskupek, U. Kaiser, O. L. Lebedev, I. Sildos, P. R. Hemmer, V. I. Konov, A. Gali and J. Wrachtrup, Nat. Nanotechnol., 2014, 9, 54–58 CrossRef CAS.
- L. Guo and P. H. Santschi, Environmental Colloids and Particles, 2007, pp. 159–221 Search PubMed.
- R. Scherrenberg, B. Coussens, P. van Vliet, G. Edouard, J. Brackman and E. de Brabander, Macromolecules, 1998, 31, 456–461 CrossRef CAS.
- C. Zhu, S. Monti and A. P. Mathew, ACS Nano, 2018, 12, 7028–7038 CrossRef CAS.
Footnote |
| † Electronic supplementary information (ESI) available: Tables S1 and S2; Fig. S1–S6. See DOI: 10.1039/c9ra04435c |
| This journal is © The Royal Society of Chemistry 2019 |