Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Insights from 125Te and 57Fe nuclear resonance vibrational spectroscopy: a [4Fe–4Te] cluster from two points of view†
Florian
Wittkamp‡
a,
Nakul
Mishra‡
 b,
Hongxin
Wang
b,
Hongxin
Wang
 b,
Hans-Christian
Wille
b,
Hans-Christian
Wille
 c,
René
Steinbrügge
c,
René
Steinbrügge
 c,
Martin
Kaupp
c,
Martin
Kaupp
 d,
Stephen P.
Cramer
d,
Stephen P.
Cramer
 *b,
Ulf-Peter
Apfel
*b,
Ulf-Peter
Apfel
 *ae and
Vladimir
Pelmenschikov
*ae and
Vladimir
Pelmenschikov
 *d
*d
aDepartment of Chemistry and Biochemistry, Inorganic Chemistry I, Ruhr-Universität Bochum, Universitätsstraße 150, 44801 Bochum, Germany. E-mail: ulf.apfel@rub.de
bDepartment of Chemistry, University of California, Davis, One Shields Avenue, Davis, California 95616, USA. E-mail: spjcramer@ucdavis.edu
cDeutsches Elektronen-Synchrotron DESY, Notkestraße 85, 22607 Hamburg, Germany
dInstitute of Chemistry, Theoretical Chemistry/Quantum Chemistry, Technical University of Berlin, Sekr. C7, Straße des 17. Juni 135, 10623 Berlin, Germany. E-mail: pelmentschikov@tu-berlin.de
eFraunhofer UMSICHT, Osterfelder Straße 3, 46047 Oberhausen, Germany
First published on 24th June 2019
Abstract
Iron–sulfur clusters are common building blocks for electron transport and active sites of metalloproteins. Their comprehensive investigation is crucial for understanding these enzymes, which play important roles in modern biomimetic catalysis and biotechnology applications. We address this issue by utilizing (Et4N)3[Fe4Te4(SPh)4], a tellurium modified version of a conventional reduced [4Fe–4S]+ cluster, and performed both 57Fe- and 125Te-NRVS to reveal its characteristic vibrational features. Our analysis exposed major differences in the resulting 57Fe spectrum profile as compared to that of the respective [4Fe–4S] cluster, and between the 57Fe and 125Te profiles. DFT calculations are applied to rationalize structural, electronic, vibrational, and redox-dependent properties of the [4Fe–4Te]+ core. We herein highlight the potential of sulfur/tellurium exchange as a method to isolate the iron-only motion in enzymatic systems.
Introduction
Tellurium is a component of many materials of current scientific and technological interest, including thermoelectrics such as PbTe-based materials,1,2 phase change materials for data storage such as Ge2Sb2Te5,3 and superconductors such as Fe1+yTe1−xSex and CdTe solar cells.4,5 Although Te is not an essential trace element in biological systems, certain Te compounds can be bioaccumulated and/or metabolized and some of them have shown or have been proposed to have valuable antibiotic or anticancer applications.6 This contrasts with the lighter chalcogens, S and Se, where S is ubiquitous in most living systems, and Se plays an essential role as selenocysteine.7–9 Only in the late 1980's, storage and transport of semimetal tellurium was observed in biological systems.10 When the tellurium-tolerant fungi Penicillium chrysogenum was fed with sodium tellurite containing media, Te incorporation was observed to afford telluro-cysteine and -methionine.10[4Fe–4S] clusters are one of the most common bioinorganic prosthetic groups, playing crucial roles in many biological processes, such as electron transfer,11,12 O2 and NO-sensing, or even as active sites in radical SAM enzymes.13–17 The role of sulfur atoms in a cofactor is commonly not specifically elucidated, since sulfur spectroscopy of enzymes remains a challenging task due to the sulfur-rich environment of the peptide backbone. Current studies on [4Fe–4S] cluster compounds (or Fe–S interactions in general) are limited to probing the iron sites. Notably, the naturally low abundance of tellurium within an organism makes this element a unique tool to investigate specific processes by following the trace of tellurium, e.g., substituting sulfur(s) at specified locations in biological samples and evaluating the metal–sulfur interaction(s) using difference spectra.
Nuclear resonance vibrational spectroscopy (NRVS),18 also known as nuclear inelastic scattering (NIS), is a relatively new synchrotron-based technique that yields vibrational spectra from nuclear transitions of an appropriate Mössbauer isotope. The key feature of this technique is that it is only sensitive to the motion of the selected isotope along the direction of the incident X-ray beam. For example, in the case of 57Fe-enriched proteins, this allows one to select exclusively the normal modes involving the 57Fe nuclei in a background of thousands of other vibrations involving the protein matrix. 57Fe-NRVS has already been used to investigate a wide variety of Fe-containing proteins, including rubredoxins, ferredoxins,19,20 heme proteins,21 hydrogenases,22,23 dioxygenases,24 NO sensors, and nitrogenase.25
In 2010, NRVS was first applied to 125Te with its nuclear resonance at 35.49 keV.26 Thanks to the development of sapphire backscattering monochromators,27 spectra with 1.1 meV (<9 cm−1) resolution have been obtained for elemental Te,27 Bi2Te3 and Sb2Te3,28–30 as well as GeTe, SnTe, and PbTe.31125Te presents a unique opportunity for bioinorganic NRVS studies thanks to the feasibility of Te for S substitution. Te can replace bridging S in Fe–S clusters, and it can also replace S to yield tellurocysteine and telluromethionine.
Despite growing popularity for materials science, there have been no reports of 125Te-NRVS of biologically relevant complexes to date. In this study we investigate the feasibility of bioinorganic 125Te-NRVS studies using tellurium modification of a well-known [4Fe–4S] cluster, resulting in a 57Fe and 125Te enriched (Et4N)3[Fe4Te4(SPh)4] model compound. Dual isotopic labelling allows for deeper insights into the dynamics of the cluster; since certain modes can be strong in one spectrum and weak or absent in the partner spectrum. Here, the unique ability to combine NRVS measurements of both 57Fe and 125Te in the same sample offers added assessment by density functional theory (DFT) which was applied to analyze the vibrational modes of the [457Fe–4125Te] core in a greater detail.
Herein, we provide a benchmark study to reveal the potential of 125Te-NRVS and a combination of isotopic labelling of both iron and tellurium as a probe in electron transport processes in future in vivo studies within enzymes' Fe–S systems, such as [FeFe]-hydrogenases and CO-dehydrogenases.
Results and discussion
Synthesis
(Et4N)3[57Fe4125Te4(SPh)4] = 1 was synthesized combining the synthetic procedures reported by Midollini32 and Haase,33via the reaction of FeCl2, LiSPh and Li2Te to obtain black needle-shaped crystals (Scheme 1).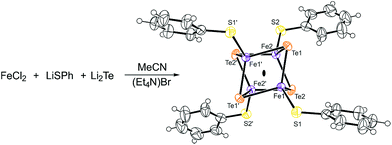 | ||
| Scheme 1 Schematic representation of the synthesis and ORTEP molecular plot of (Et4N)3[Fe4Te4(SPh)4]. The probability level is 50%. Counter ions are omitted for clarity. | ||
The black crystals were analyzed by 1H NMR spectroscopy and X-ray diffractometry. The proton NMR spectrum (Fig. S1†) shows three paramagnetic signals at 13.5 ppm (meta), −3.1 ppm (ortho) and −4.1 ppm (para) in a 2![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2
2![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1 ratio and are assigned to the aromatic protons of the anionic cluster. In addition, two diamagnetic signals at 3.04 ppm and 1.35 ppm in a ratio of 2
1 ratio and are assigned to the aromatic protons of the anionic cluster. In addition, two diamagnetic signals at 3.04 ppm and 1.35 ppm in a ratio of 2![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3 are observed which can be assigned to the tetraethyl ammonium counter ions. The overall spectrum closely resembles the spectrum presented by Midollini et al.32
3 are observed which can be assigned to the tetraethyl ammonium counter ions. The overall spectrum closely resembles the spectrum presented by Midollini et al.32
The obtained crystals were of decent quality (R = 2.6) to perform single crystal X-ray analysis, and the resulting structure confirms the successful synthesis of [Fe4Te4(SPh)4]3−. Compound 1 crystallizes in the space group Fdd2 (Scheme 1, Table S1†). Since the structural features of the cubic cluster are already thoroughly discussed, we herein refer to the literature.32
NRVS
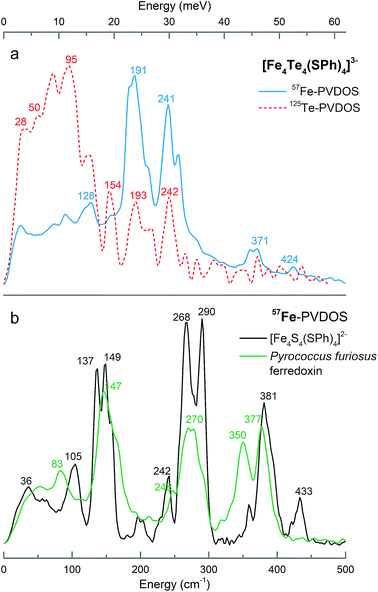
Fig. 1a presents the 125Te and 57Fe partial vibrational density of states (PVDOS) spectra obtained from our NRVS experiments on 1. The 57Fe-PVDOS spectrum shows four main features: a small peak at ∼130 cm−1, a global maximum at ∼190 cm−1, a doublet at ∼240–250 cm−1, and weaker features at ∼360–370 cm−1. An even weaker band is resolved at ∼420 cm−1. At first glance the 57Fe-PVDOS profile of 1 appears to be a completely different spectrum compared to the profiles of [(n-Bu)4N]2[Fe4S4(SPh)4]34 and D14C mutant of ferredoxin from Pyrococcus furiosus (Pf Fd) shown in Fig. 1b.19 There seems little resemblance to the 57Fe-PVDOS of [4Fe–4S] clusters, which have strong in-core Fe–S stretching bands very approximately in the ∼270–290 and ∼350–380 cm−1 regions (Fig. 1b). However, a simple binary harmonic oscillator model for 57Fe–125Te vs.57Fe–32S vibrations, assuming the same force constant, predicts a frequency downshift factor of 0.72 because of the large 125Te mass. This model yields predicted Fe–Te bands in the ∼190–210 cm−1 and ∼250–270 cm−1 regions, in approximate agreement with the observed 57Fe spectrum of 1 (Fig. 1a). Thus, the primary cause of the spectral changes can be attributed to the dramatic change in the chalcogen mass.The 125Te-PVDOS spectrum of 1 reproduces the two main features of the 57Fe spectrum at ∼190 cm−1 and ∼240 cm−1, but at significantly lower intensity. For 125Te, the spectrum shows a global maximum at ∼100 cm−1 within a broad feature with further local maxima between 20 and 120 cm−1. Qualitatively, this indicates relatively little 125Te motion in these modes, which are the strongest 57Fe features. Even without sophisticated analysis, one can envision the Fe ions moving in a much less mobile matrix of Te ions. In contrast, below 100 cm−1 there are modes that show primarily Te motion.
DFT calculations
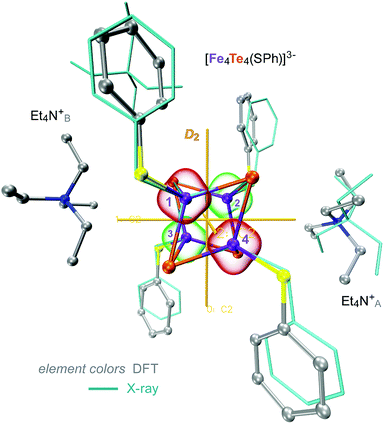 | ||
| Fig. 2 Overlay of the X-ray (wire) and DFT-optimized (ball-and-stick) structures of 1, combined from the [Fe4S4(SPh)4]3− and 2 × Et4N+ molecular fragments. 3 × C2 two-fold axes of the idealized D2 symmetry of the [4Fe–4Te]+ core are shown. Red and green bubbles around the numbered Fe sites correspond to the positive (↑) and negative (↓) 0.01 a.u. point spin density isosurfaces from the representative DFT solution. Hydrogen atoms are omitted for clarity. For extra details, see ESI Fig. S2–S4.† | ||
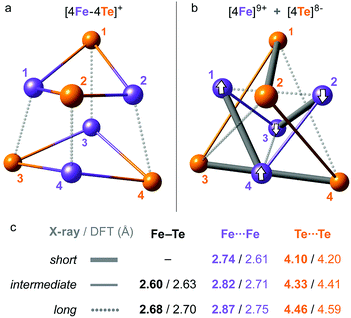 | ||
| Fig. 3 Alternative representations of the iron–tellurium cuboidal core of 1 as (a) an [4Fe–4Te] hexahedron showing 12 × Fe–Te bonding interactions, and (b) a compound of smaller [4Fe] and larger [4Te] tetrahedra showing 6 × Fe⋯Fe and 6 × Te⋯Te nonbonding interactions. The internuclear distances are characterized in subsets of ‘long’, ‘intermediate’, and (optionally) ‘short’, with (c) their mean values in the respective polyhedra given as found in the X-ray/DFT-optimized structures. For a detailed account of these distances, see ESI Table S2.† Broken-symmetry (BS) spin alignment (↑ or ↓) of the [4Fe] sites applied in the DFT solution is shown in (b). | ||
In order to compare the [4Fe–4Te]+ species to its far more common [4Fe–4S]+ counterpart, we likewise analyzed computationally a 1-S species, where the 4 × Te nuclei of 1 were substituted by 4 × S. This analysis included re-optimization of the 1-S model, resulting in a more compressed core structure due to the smaller bridging sulfide dimensions (Fig. S4†). In line with earlier X-ray determinations,32 the mean DFT-optimized S⋯S distance in 1-S is strikingly ∼0.7 Å shorter than the corresponding Te⋯Te distance in 1, and the Fe⋯Fe distances are those least affected (Table S2†). The recognized ‘stereochemical softness’36 of the reduced [4Fe–4X]+ core is manifested here as a modified distribution of the Fe–X distances when X is either Te (1) or S (1-S), yet retaining the matching cuboid elongation depicted vertically in Fig. 3a.
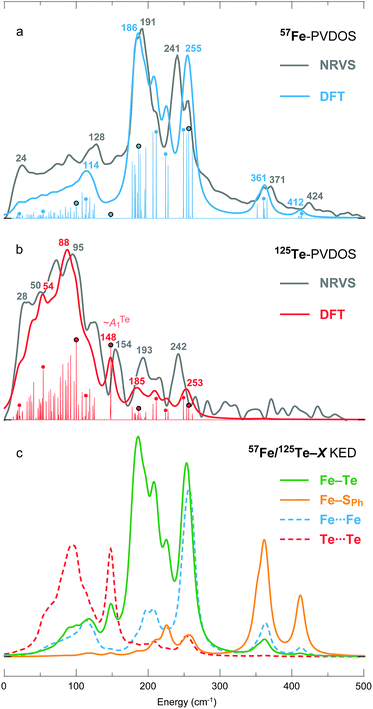 | ||
| Fig. 4 (a) 57Fe- and (b) 125Te-PVDOS spectra of 1 from NRVS measurements and DFT calculations, followed by (c) DFT-based KED spectra of the relative nuclei motion in bonding 12 × Fe–Te and 4 × Fe–SPh, and non-bonding 6 × Fe⋯Fe and 6 × Te⋯Te interactions. Matching NRVS and DFT bands are labelled with their positions. Non-broadened (individual normal mode) DFT PVDOS intensities are additionally provided in a stick-style. For the sticks labelled with dots, corresponding mode animations are available as part of the ESI;† for the four dots circled in black, corresponding modes are depicted in Fig. 5. | ||
A smaller DFT model 1′ = [Fe4Te4(SPh)4]3−, which lacks the Et4N+ counter ions, has been considered additionally. Both the more complete 1 and the size-reduced 1′ models produce qualitatively similar PVDOS spectra as shown in Fig. S5.† Inclusion of the counter ions in 1 (vs. exclusion in 1′), however, provides a more realistic distribution of the intensities in the 180–260 cm−1 range of the 57Fe-PVDOS, and as well a better population of the low-frequency <100 cm−1 portion of the 125Te-PVDOS.
DFT-based kinetic energy distribution (KED) spectra shown in Fig. 4c facilitate analysis of the normal modes in terms of relative displacements in the nuclei pairs. The calculated 57Fe-PVDOS spectrum is largely reproduced by the Fe–Te KED profile, implying that the Fe nuclei vibrate in a predominantly static framework of the Te nuclei. The 125Te-PVDOS is in contrast similar to the ‘Te-only’ Te⋯Te profile, as if the Te nuclei moved independently. 57Fe nuclei vibrations in the stronger Fe–SPh (vs. weaker Fe–Te) bonds produce the two NRVS/DFT high-end bands at 371/361 and 424/412 cm−1, respectively (Fig. 4a).
Breakdown of the calculated PVDOS into contributions from individual normal modes, see Fig. 4a and b and 5, provide further characterization of 1. Of remarkable note is a mode calculated at 148 cm−1 and having prominent 125Te-PVDOS (and Te⋯Te KED) intensity, yet vanishingly weak in 57Fe-PVDOS. Centered in the broad ∼50 cm−1 region devoid of other bands, the 148 cm−1 mode directly corresponds to the experimental 125Te band observed at 154 cm−1. DFT reveals this as a pseudo-A1Te (totally-symmetric) character ‘breathing’ of the [4Te] tetrahedron depicted in Fig. 5b, a type of vibration well-known from studies on [4Fe–4S] and other cubic clusters.19,34,39
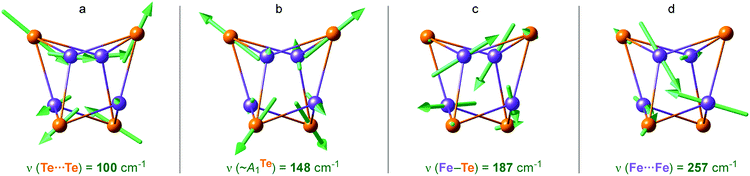 | ||
| Fig. 5 Arrow-style representations of selected calculated normal modes in the [4Fe–4Te]+ core of 1, having (a) Te⋯Te, (b) ‘breathing’ ∼A1Te, (c) Fe–Te, and (d) Fe⋯Fe character. The arrow sizes are proportional to the nuclei displacement amplitudes; actual amplitudes in (b) are respectively ∼0.02/0.005 Å for Te/Fe. Animations for these and several other normal modes are provided as part of the ESI.† | ||
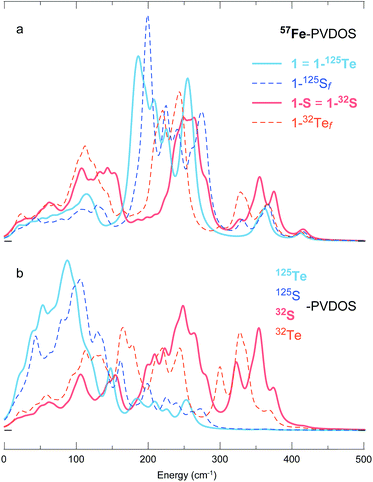
To disentangle the (i) structural/electronic properties and (ii) nuclear masses in the effects determining the PVDOS, we introduce two additional DFT systems 1-32Tef and 1-125Sf having fictitious isotope nuclei with the ‘native’ Te and S masses interchanged, yet their structures correspondingly equal to 1 = 1-125Te and 1-S = 1-32S. As seen from the PVDOS comparison in Fig. 6, the 1-125Te and 1-125Sf systems, despite their elementary (and thus chemically) different content and different structures, display very similar pairs of both 57Fe- and 125Te(125S)-PVDOS profiles; a blue-shift of ∼15–20 cm−1 in the 1-125Sfvs.1-125Te bands is explained by the shorter Fe–S vs. Fe–Te distances (Fig. S4 and Table S2†). In contrast, the variation is more prominent between the 1-32S and 1-32Tef DFT spectra, particularly in the 32S(32Te)-PVDOS. The 57Fe-PVDOS intensities in the 1-32S(32Tef) systems become more evenly distributed over the entire spectral range vs. sharper bands of 1-125Te(125Sf) consolidated around 180–280 cm−1, as seen in Fig. 6a. Displaying similarities to the [4Fe–4S] NRVS spectra observed earlier,19,34 see spectra in Fig. 1b, this behaviour of 1-32S(32Tef) is explained by a stronger vibrational coupling between the motion of Fe and the lighter 32-mass (vs. heavier 125-mass) nuclei in the cubic core. The naturally relevant 1-32S system shows the strongest coupling, producing 57Fe- (Fig. 6a) and 32S-PVDOS (Fig. 6b) profiles that nearly follow each other.
The 57Fe-PVDOS comparison drawn above for the reduced 1vs.1-S DFT models (Fig. 6a) applies as well to their 1e− oxidized [1]+vs. [1-S]+ counterparts (Fig. S6a vs. b†). Both Te/S chalcogenide alternatives display only minor (Te-variant) to moderate (S-variant) redox-dependent alterations in their spectra, where the S-variant behaves similar to the [4Fe–4S]+/2+ cofactor from ferredoxin characterized by 57Fe-NRVS earlier.19,20 The major bands display blue shifts within 10 cm−1 upon oxidation, explained by +0.8/+1.5% larger volume of respectively the [4Fe–4Te/S]1+vs. [4Fe–4Te/S]2+ cores (Table S3†). While these redox-dependent changes can be considered as insignificant, relative trends in the 57Fe-PVDOS and core volume adjustments indicate that the S-to-Te substitution may present a cubic cluster having enhanced proficiency in electron transfer. This expectation is now supported by a significant ∼1.5 ratio (S/Te-variant) in the calculated reorganization energies of structural relaxation following electron transfer for the 1-S/[1-S]+ (17.4 kJ mol−1) vs.1/[1]+ (11.6 kJ mol−1) redox couples (Table S4†). The [4Fe–4Te] core with its volume ∼1.4 times larger than that of [4Fe–4S] (Table S3†) is therefore expected to be more efficient in charge delocalization.
Conclusions
Iron–sulfur clusters play many important roles in biological systems, ranging from purely structural functions to electron transfer, small molecule sensing, and as components of active sites. However, the large amount of sulfur in most biological samples poses a challenge for spectroscopists who want to investigate these clusters from the sulfur point of view.We have shown here that sulfur to tellurium exchange, combined with NRVS measurements from both 57Fe and 125Te points of view, creates a powerful tool for chalcogenide-specific vibrational spectroscopy. Additionally, DFT calculations reproduced and rationalized the obtained structure and spectra. Our results clearly demonstrate corresponding bands in 57Fe- and 125Te-NRVS spectra that could successfully be assigned to the same normal modes. Unlike [4Fe–4S] clusters where the vibrational coupling of the Fe and S motion is tight, a significant difference in the Fe vs. Te nuclei masses and slightly weaker Fe–Te force constants in the [4Fe–4Te] cuboid both lead to divergence in the vibrational signatures of the [4Fe] and [4Te] interposed tetrahedra. Herein, this exchange leads to clearly isolated Fe-only motions displaying sharper bands within the 57Fe spectrum by almost cancelling out the Fe–Te vibrational coupling. Thus, (selective) Te incorporation can be potentially used to simplify complex 57Fe-NRVS spectra of proteins containing multiple Fe–S clusters, and to allow for a more discrete discussion on the properties of such active centers.
This work is the first 125Te-NRVS investigation of an isolated [4Fe–4Te] cluster, and it lays the basis for bioinorganic spectroscopic investigation of Te substitution. Our disclosure of the [4Te]-‘breathing’ mode in 1 is an example of finding by 125Te-NRVS which would evade determination by 57Fe-NRVS which is commonly used nowadays. Se substitution for S in biological [2Fe–2S]40 and [4Fe–4S]41 clusters have been known for decades,42 and Te substitution in various electron-transport proteins as well as enzyme active sites should be feasible. For example, a [4Fe–4Se] cluster was shown to replace the [4Fe–4S]H sub-cluster in [FeFe]-hydrogenase with full retention of activity.43 [4Fe–4Te] clusters could presumably be incorporated into hydrogenase in the same manner and studied by 125Te-NRVS. Since Se can also be incorporated into thiolate positions of the H-cluster azadithiolate ligand,44 comparable experiments using Te and 125Te-NRVS can also be envisaged. In small molecules, Te can be a replacement for terminal thiolate ligands or thioethers, although it remains to be seen whether tellurocysteine or telluromethionine can be inserted into proteins in the manner already established for selenocysteine. Finally, Se has been shown to incorporate into the nitrogenase MoFe cofactor,45 and the analogous experiments with Te might be interesting. Although it has a larger ionic radius and lower electronegativity, tellurium often exhibits chemistry similar to sulfur, and it can replace sulfur in many structures. Our calculations for the [4Fe–4Te] vs. [4Fe–4S] clusters indicate that, while iron–tellurium cofactors are bigger in their size, they have lower reorganization energies favorable during events of electron transfer. These results clearly showcase the potential of 125Te-NRVS in such modified systems.
Conflicts of interest
There are no conflicts of interest to declare.Acknowledgements
U.-P. A. acknowledges funding by the Fonds der Chemischen Industrie (Liebig Grant), the Deutsche Forschungsgemeinschaft (Emmy Noether Grant AP242/2-1), and the Fraunhofer Internal Programs (Grant ATTRACT 097-602175). F. W. thanks the Studienstiftung des Deutschen Volkes for a PhD fellowship. N. M., H. W., and S. P. C. acknowledge support by the National Institutes of Health (grant number GM-65440) and the Einstein Foundation Berlin (grant number EVF-2016-277). V. P. and M. K. acknowledge funding by the Deutsche Forschungsgemeinschaft under Germany's Excellence Strategy – EXC 2008/1 (UniSysCat) – 390540038. We acknowledge DESY (Hamburg, Germany), a member of the Helmholtz Association HGF, for the provision of experimental facilities. The 125Te-NRVS work was carried out at PETRA III and we would like to thank I. Sergeev and O. Leupold for assistance in using the P01 beamline. The 57Fe-NRVS work was performed at SPring-8 under proposals 2018B0141 and 2018A1409.Notes and references
- K. F. Hsu, S. Loo, F. Guo, W. Chen, J. S. Dyck, C. Uher, T. Hogan, E. K. Polychroniadis and M. G. Kanatzidis, Science, 2004, 303, 818–821 CrossRef CAS PubMed.
- Y. Xiao and L.-D. Zhao, npj Quantum Mater., 2018, 3, 55 CrossRef.
- N. Harmgarth, F. Zörner, P. Liebing, E. P. Burte, M. Silinskas, F. Engelhardt, F. T. Edelmann and Z. Für, Z. Anorg. Allg. Chem., 2017, 643, 1150–1166 CrossRef CAS.
- J. Wen, G. Xu, G. Gu, J. M. Tranquada and R. J. Birgeneau, Rep. Prog. Phys., 2011, 74, 124503 CrossRef.
- Z. Xu, J. A. Schneeloch, J. Wen, B. L. Winn, G. E. Granroth, Y. Zhao, G. Gu, I. Zaliznyak, J. M. Tranquada, R. J. Birgeneau and G. Xu, Phys. Rev. B: Condens. Matter Mater. Phys., 2017, 96, 134505 CrossRef.
- L. A. Ba, M. Döring, V. Jamier and C. Jacob, Org. Biomol. Chem., 2010, 8, 4203–4216 RSC.
- T. C. Stadtman, Annu. Rev. Biochem., 1996, 65, 83–100 CrossRef CAS PubMed.
- A. Böck, K. Forchhammer, J. Heider and C. Baron, Trends Biochem. Sci., 1991, 16, 463–467 CrossRef.
- B. L. Cohen, Geochim. Cosmochim. Acta, 1984, 48, 203–205 CrossRef CAS.
- S. E. Ramadan, A. A. Razak, A. M. Ragab and M. El-Meleigy, Biol. Trace Elem. Res., 1989, 20, 225–232 CrossRef CAS PubMed.
- B. B. Buchanan and D. I. Arnon, Adv. Enzymol., 1970, 33, 119–176 CAS.
- W. Lubitz, H. Ogata, O. Rüdiger and E. Reijerse, Chem. Rev., 2014, 114, 4081–4148 CrossRef CAS PubMed.
- J. C. Crack, J. Munnoch, E. L. Dodd, F. Knowles, M. M. Al Bassam, S. Kamali, A. A. Holland, S. P. Cramer, C. J. Hamilton, M. K. Johnson, A. J. Thomson, M. I. Hutchings and N. E. Le Brun, J. Biol. Chem., 2015, 290, 12689–12704 CrossRef CAS PubMed.
- H. Beinert, JBIC, J. Biol. Inorg. Chem., 2000, 5, 2–15 CrossRef CAS PubMed.
- J. B. Broderick, B. R. Duffus, K. S. Duschene and E. M. Shepard, Chem. Rev., 2014, 114, 4229–4317 CrossRef CAS PubMed.
- N. D. Lanz and S. J. Booker, Biochim. Biophys. Acta, Mol. Cell Res., 2015, 1853, 1316–1334 CrossRef CAS PubMed.
- K. Yokoyama and E. A. Lilla, Nat. Prod. Rep., 2018, 35, 660–694 RSC.
- W. Zeng, N. J. Silvernail, W. R. Scheidt and J. T. Sage, in Encyclopedia of Inorganic and Bioinorganic Chemistry, ed. R. A. Scott, John Wiley & Sons, Ltd, Chichester, UK, 2011 Search PubMed.
- D. Mitra, V. Pelmenschikov, Y. Guo, D. A. Case, H. Wang, W. Dong, M.-L. Tan, T. Ichiye, F. E. Jenney, M. W. W. Adams, Y. Yoda, J. Zhao and S. P. Cramer, Biochemistry, 2011, 50, 5220–5235 CrossRef CAS PubMed.
- L. Lauterbach, L. B. Gee, V. Pelmenschikov, F. E. Jenney, S. Kamali, Y. Yoda, M. W. W. Adams and S. P. Cramer, Dalton Trans., 2016, 45, 7215–7219 RSC.
- W. R. Scheidt, J. Li and J. T. Sage, Chem. Rev., 2017, 117, 12532–12563 CrossRef CAS PubMed.
- E. J. Reijerse, C. C. Pham, V. Pelmenschikov, R. Gilbert-Wilson, A. Adamska-Venkatesh, J. F. Siebel, L. B. Gee, Y. Yoda, K. Tamasaku, W. Lubitz, T. B. Rauchfuss and S. P. Cramer, J. Am. Chem. Soc., 2017, 139, 4306–4309 CrossRef CAS PubMed.
- V. Pelmenschikov, J. A. Birrell, C. C. Pham, N. Mishra, H. Wang, C. Sommer, E. Reijerse, C. P. Richers, K. Tamasaku, Y. Yoda, T. B. Rauchfuss, W. Lubitz and S. P. Cramer, J. Am. Chem. Soc., 2017, 139, 16894–16902 CrossRef CAS PubMed.
- K. D. Sutherlin, B. S. Rivard, L. H. Böttger, L. V. Liu, M. S. Rogers, M. Srnec, K. Park, Y. Yoda, S. Kitao, Y. Kobayashi, M. Saito, M. Seto, M. Hu, J. Zhao, J. D. Lipscomb and E. I. Solomon, J. Am. Chem. Soc., 2018, 140, 5544–5559 CrossRef CAS PubMed.
- D. Mitra, S. J. George, Y. Guo, S. Kamali, S. Keable, J. W. Peters, V. Pelmenschikov, D. A. Case and S. P. Cramer, J. Am. Chem. Soc., 2013, 135, 2530–2543 CrossRef CAS PubMed.
- H.-C. Wille, R. P. Hermann, I. Sergueev, U. Pelzer, A. Möchel, T. Claudio, J. Perßon, R. Rüffer, A. Said and Yu. V. Shvyd’ko, EPL, 2010, 91, 62001 CrossRef.
- I. Sergueev, H.-C. Wille, R. P. Hermann, D. Bessas, Yu. V. Shvyd’ko, M. Zając and R. Rüffer, J. Synchrotron Radiat., 2011, 18, 802–810 CrossRef CAS PubMed.
- D. Bessas, I. Sergueev, H.-C. Wille, J. Perßon, D. Ebling and R. P. Hermann, Phys. Rev. B: Condens. Matter Mater. Phys., 2012, 86, 224301 CrossRef.
- D. Bessas, W. Töllner, Z. Aabdin, N. Peranio, I. Sergueev, H.-C. Wille, O. Eibl, K. Nielsch and R. P. Hermann, Nanoscale, 2013, 5, 10629–10635 RSC.
- R. E. Simon, I. Sergueev, I. Kantor, A. Kantor, J. Perßon and R. P. Hermann, Semicond. Sci. Technol., 2014, 29, 124001 CrossRef.
- P. Bauer Pereira, I. Sergueev, S. Gorsse, J. Dadda, E. Müller and R. P. Hermann, Phys. Status Solidi B, 2013, 250, 1300–1307 CrossRef CAS.
- P. Barbaro, A. Bencini, I. Bertini, F. Briganti and S. Midollini, J. Am. Chem. Soc., 1990, 112, 7238–7246 CrossRef CAS.
- H.-O. Stephen, C. Chen, G. Henkel, K. Griesar and W. Haase, J. Chem. Soc., Chem. Commun., 1993, 886–888 RSC.
- Y. Xiao, M. Koutmos, D. A. Case, D. Coucouvanis, H. Wang and S. P. Cramer, Dalton Trans., 2006, 2192–2201 RSC.
- L. Noodleman and D. A. Case, in Advances in Inorganic Chemistry, Elsevier, 1992, vol. 38, pp. 423–470 Search PubMed.
- M. J. Carney, G. C. Papaefthymiou, K. Spartalian, R. B. Frankel and R. H. Holm, J. Am. Chem. Soc., 1988, 110, 6084–6095 CrossRef CAS PubMed.
- P. Venkateswara Rao and R. H. Holm, Chem. Rev., 2004, 104, 527–560 CrossRef CAS PubMed.
- P. W. Anderson and H. Hasegawa, Phys. Rev., 1955, 100, 675–681 CrossRef CAS.
- R. S. Czernuszewicz, K. A. Macor, M. K. Johnson, A. Gewirth and T. G. Spiro, J. Am. Chem. Soc., 1987, 109, 7178–7187 CrossRef CAS.
- J. C. M. Tsibris, M. J. Namtvedt and I. C. Gunsalus, Biochem. Biophys. Res. Commun., 1968, 30, 323–327 CrossRef CAS PubMed.
- J. Meyer and J.-M. Moulis, Biochem. Biophys. Res. Commun., 1981, 103, 667–673 CrossRef CAS PubMed.
- J. Meyer, J.-M. Moulis, J. Gaillard and M. Lutz, in Advances in Inorganic Chemistry, Elsevier, 1992, vol. 38, pp. 73–115 Search PubMed.
- J. Noth, J. Esselborn, J. Güldenhaupt, A. Brünje, A. Sawyer, U.-P. Apfel, K. Gerwert, E. Hofmann, M. Winkler and T. Happe, Angew. Chem., Int. Ed., 2016, 55, 8396–8400 CrossRef CAS PubMed.
- L. Kertess, F. Wittkamp, C. Sommer, J. Esselborn, O. Rüdiger, E. J. Reijerse, E. Hofmann, W. Lubitz, M. Winkler, T. Happe and U.-P. Apfel, Dalton Trans., 2017, 46, 16947–16958 RSC.
- T. Spatzal, K. A. Perez, J. B. Howard and D. C. Rees, eLife, 2015, 4, e11620 CrossRef PubMed.
Footnotes |
| † Electronic supplementary information (ESI) available: Supplementary Tables S1–S4, Fig. S1–S6, Materials and methods, atomic Cartesian coordinates, and animated vibrational modes. CCDC 1908709. For ESI and crystallographic data in CIF or other electronic format see DOI: 10.1039/c9sc02025j |
| ‡ These authors contributed equally. |
| This journal is © The Royal Society of Chemistry 2019 |