Open Access Article
Open Access ArticleBuilding an electrochemical series of metals in pyrrolidinium-based ionic liquids†
Yeojin Jung‡
a,
Bonita Dilasarib,
Wi-Sup Baea,
Hong-In Kim*c and
Kyungjung Kwon *a
*a
aDepartment of Energy, Mineral Resources Engineering, Sejong University, Seoul 05006, Republic of Korea. E-mail: kfromberk@gmail.com
bDepartment of Metallurgical Engineering, Bandung Institute of Technology, Bandung 40132, Indonesia
cConvergence Research Center for Development of Mineral Resources, Korea Institute of Geoscience and Mineral Resources, Daejeon 34132, Republic of Korea. E-mail: hi@kigam.re.kr
First published on 24th June 2020
Abstract
An electrochemical series of pyrrolidinium-based ionic liquids is established by designing a redox system where only one kind of anion is present in the electrolyte and metal ions are supplied by anodic dissolution. This is the first report where an electrochemical series is established in pure ionic liquids.
Ionic liquids, salts in the liquid state, are typically a family of molten salts solely comprised of cations and anions with a low melting point below 100 °C. The unique physicochemical properties of ionic liquids such as low vapor pressure, non-volatility, good thermal stability, high ionic conductivity and a wide electrochemical window make ionic liquids an alternative to conventional organic and aqueous electrolytes.1 Meanwhile, almost unlimited variations of ionic liquids can be synthesized by combining numerous kinds of cations and anions. These features of ionic liquids have enabled the implementation of various technologies, and particularly the redox chemistry of various metals in ionic liquids has been utilized in such applications as electrodeposition, electrowinning, cementation, corrosion, metal-air batteries and redox batteries.2–10 Recently, some unusual findings where a less noble metal is deposited on a more noble metal by electroless deposition, that is, a galvanic displacement reaction, have been reported.11–13 As Lahiri et al. pointed out, these kinds of galvanic displacement reactions could be triggered by forming metal ion complexes with the anions of ionic liquids, making the redox potentials in ionic liquids different to those of metal complexes in aqueous solution.13
An electrochemical series in an aqueous system has been well established, and is available in the form of a standard electrode potential database.14 Standard electrode potential is defined as the measure of the individual potential of reversible electrodes at a standard state with ions at an effective concentration of 1 M at the pressure of 1 atm, and is usually measured or calculated at 25 °C. Similarly, the galvanic series was also established for the determination of the nobility of metals in saltwater.15 In contrast, there is no universal electrochemical series in the case of ionic liquids. Because the combination of metal and ionic liquid is very selective for the specific purpose of the aforementioned applications where the redox chemistry of metals in ionic liquids is utilized,16 Abbott et al.'s electrochemical series for deep eutectic solvents would be the only report of its kind.17 They established an electrochemical series of various redox couples in ethaline that is the 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2 mixture of choline chloride and ethylene glycol. Although their approach was systematic and extensive, there would be some limitations in that ethaline was not a pure compound but a mixture and adopted metal salts consisted of various unspecified anions. Because the metal-ion speciation in this case is complex and inconsistent, it would be desirable to make a redox system as simple as possible. Therefore, we design a redox system where only one kind of anion is present in electrolyte and metal ions are supplied by anodic dissolution for the establishment of an electrochemical series in ionic liquids. In this study, an attempt to build the electrochemical series of various metals is made in pyrrolidinium-based ionic liquids by using a ferrocene/ferrocenium (Fc/Fc+) internal reference at room temperature.
2 mixture of choline chloride and ethylene glycol. Although their approach was systematic and extensive, there would be some limitations in that ethaline was not a pure compound but a mixture and adopted metal salts consisted of various unspecified anions. Because the metal-ion speciation in this case is complex and inconsistent, it would be desirable to make a redox system as simple as possible. Therefore, we design a redox system where only one kind of anion is present in electrolyte and metal ions are supplied by anodic dissolution for the establishment of an electrochemical series in ionic liquids. In this study, an attempt to build the electrochemical series of various metals is made in pyrrolidinium-based ionic liquids by using a ferrocene/ferrocenium (Fc/Fc+) internal reference at room temperature.
The schematic structure of the cation and anions constituting two ionic liquids (1-butyl-1-methylpyrrolidinium bis(trifluoromethylsulfonyl)imide and 1-butyl-1-methylpyrrolidinium bis(fluorosulfonyl)imide) adopted for this study is presented in Scheme 1. 1-Butyl-1-methylpyrrolidinium cation has a few different abbreviations (BMPyr, BMP or Py1,4) in literature. Two kinds of anions (bis(fluorosulfonyl)imide and bis(trifluoromethylsulfonyl)imide) also have various abbreviations where we prefer to use Nf2 (rather than FSA) for bis(fluorosulfonyl)imide, and NTf2 (rather than TFSI, Tf2N, and TFSA) for bis(trifluoromethylsulfonyl)imide.
 | ||
| Scheme 1 Schematic structures of (a) 1-butyl-1-methylpyrrolidinium [BMPyr]+, (b) bis(fluorosulfonyl)imide [Nf2]−, and (c) bis(trifluoromethylsulfonyl)imide [NTf2]−. | ||
Fig. 1 shows a comparison of electrochemical window of [BMPyr] [NTf2] and [BMPyr] [Nf2] ionic liquids in neat condition. The cathodic and anodic electrochemical limit is commonly defined at the voltage where a specific current density is reached. The cutoff current density is usually and arbitrarily selected from 0.1 to 1.0 mA cm−2.18 [BMPyr] [NTf2] exhibits cathodic stability limit and anodic stability limit up to −3.5 and 2.4 V, respectively, and consequently the electrochemical window is 5.9 V. [BMPyr] [Nf2] exhibits cathodic stability limit at −2.5 V and anodic stability limit at 2.8 V, showing the electrochemical window of 5.3 V. No marked reduction or oxidation currents of the electrolytes were detected within this range. All potentials reported herein are displayed on the Fc/Fc+ potential basis, of which stability during storage or cyclic voltammetry in the ionic liquids were confirmed in Fig. S1.† Particularly, [BMPyr] [NTf2] exhibits more negative cathodic stability limit than [BMPyr] [Nf2] whereas [BMPyr] [Nf2] displays a little more extended anodic stability limit than [BMPyr] [NTf2]. In general, the electrochemical window of ionic liquids depends on cation/anion combination, water content, and other factors. The decomposition of corresponding cations and anions of ionic liquids affects cathodic and anodic limits, respectively.19–21 Considering that [BMPyr] [NTf2] and [BMPyr] [Nf2] have the same cation, it is surprising that there is a definite difference in cathodic stability. However, Ong et al. have demonstrated that the cathodic stability is influenced not by cation alone but by the combination of cation and anion.18
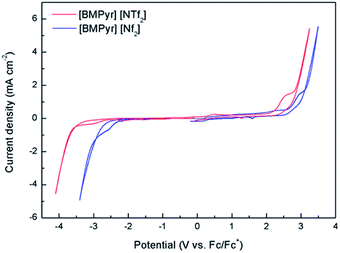 | ||
| Fig. 1 Electrochemical window of [BMPyr] [NTf2] and [BMPyr] [Nf2] (gold working electrode in neat condition at a scan rate of 10 mV s−1 at room temperature). | ||
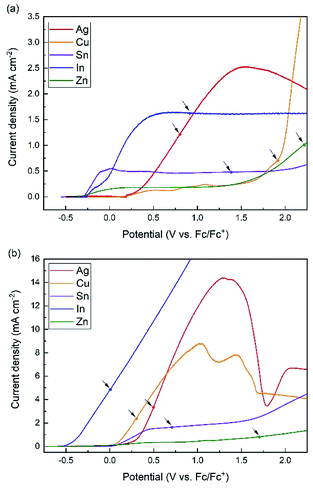
Metal ions are usually introduced to ionic liquids by dissociating metal salts such as metal chloride.3,17 Because the solubility of metal salts in ionic liquids is generally much lower than that in aqueous solutions, as high as 1 M of metal ion concentration cannot be achieved for measuring standard electrode potential. Therefore, we applied an anodic dissolution to the preparation of a designated concentration of metal ions in the ionic liquids.22,23 Once a linear sweep voltammetry is conducted for a specific couple of metal and ionic liquid, the required amount of metal ions for a designated concentration (0.1 mM) is calculated based on Faraday's law and the corresponding upper limit of dissolution potential is determined. Fig. 2 shows the linear sweep voltammetry (LSV) for five metals in [BMPyr] [NTf2] and [BMPyr] [Nf2] at room temperature. All of the metals used as working electrodes can be electrochemically oxidized in [BMPyr] [NTf2] and [BMPyr] [Nf2]. More anodic currents were observed in [BMPyr] [Nf2] than [BMPyr] [NTf2] because Nf2 anion based ionic liquids have a higher conductivity and lower viscosity than NTf2 anion based compounds.24 The amount of dissolved metals required for 0.1 mM concentration and their corresponding dissolution potential are calculated based on Faraday's law (ESI†) and Fig. 2, and tabulated in Table 1.
| Element | Amount of dissolved metals (μg) | Dissolution potential (V vs. Fc/Fc+) | |
|---|---|---|---|
| [BMPyr] [NTf2] | [BMPyr] [Nf2] | ||
| Ag | 21.6 | 0.8 | 0.5 |
| Cu | 12.7 | 1.9 | 0.3 |
| Sn | 23.7 | 1.4 | 0.7 |
| In | 23.0 | 0.9 | 0 |
| Zn | 13.1 | 2.2 | 1.7 |
To calculate the upper limit of dissolution potential, we need to assign the number of mole of transferred electrons in each redox reaction appearing in Faraday's law. Previous reports investigating the redox behaviors of Ag, Cu, Sn, In, and Zn in [BMPyr] [NTf2] or [BMPyr] [Nf2] were referred to for assigning the number of electrons, especially for metals having more than an oxidation state such as Cu and Sn.25–28 As the upper limit of dissolution potential for each metal is indicated by arrows in Fig. 2, the arrows are located on initial uphill sections or plateaus of the current density. An assumption made for the calculation in Table 1 is that all anodic currents before the upper limit of dissolution potential generate metal ions in the ionic liquids. Because ionic liquids are a non-aqueous medium with low oxygen content but extremely high concentrations of cations and anions, it is expected that unfavorable formation of oxide film that prevents dissolution of metals are prevented under the circumstance of negligible oxygen and moisture level in a glove box.29 As Abbott et al. analyzed the anodic dissolution behaviors of various metals, a sudden decrease in current following an anodic peak would be characteristic of passivation layer formation in ionic liquids.23 All of the arrows in Fig. 2 appear before reaching the downhill sections of current density, which are clearly shown for Ag in both ionic liquids and Cu in [BMPyr] [Nf2], or the onset potential of anodic decomposition of the ionic liquids (2.4 V for [BMPyr] [NTf2] and 2.8 V for [BMPyr] [Nf2]). The assumption of 0.1 mM concentration herein is based on 100% faradaic efficiency, and otherwise, the actual concentration could be monitored by a technique such as UV-vis absorption spectroscopy and adjusted to 0.1 mM by trial and error, which is set aside for future study.11,30 Incidentally, an effort was made to increase the metal ion concentration more than 0.1 mM by extending the upper limit of dissolution potential, which became unsuccessful, because most metals particularly in [BMPyr] [NTf2] soon reach the onset potential of anodic decomposition of ionic liquids. Meanwhile, an attempt to establish a more comprehensive electrochemical series was also made by dissolving other kinds of metals than the five presented metals electrochemically. Because some metals showed high open circuit potential (OCP) or otherwise large anodic overpotential, the upper limit of dissolution potential for 0.1 mM went beyond the onset potential of anodic decomposition of the ionic liquids.
Once a situation where a metal is in contact with a designated concentration of metal ions is set up, the achievement of an equilibrium state is hinted at by monitoring OCP with time.
Although OCP after the anodic dissolution process became somewhat stabilized, a lack of consistency was observed, which made us resort to a corrosion potential measurement during cathodic potentiodynamic polarization for estimating redox potential as a practical and reproducible measure. Fig. S2† shows the potentiodynamic polarization of the five metals in [BMPyr] [NTf2] and [BMPyr] [Nf2] where 0.1 mM of each metal dissolved. The thermodynamic activity of the metals in the ionic liquids is compared by measuring the corrosion potential. In [BMPyr] [NTf2], the corrosion potential is in the order of Ag (0.14 V) > In (0 V) > Cu (−0.07 V) > Sn (−0.22 V) > Zn (−0.48 V), suggesting that Ag is the most noble metal while Zn is the most reactive in this ionic liquid. The similar corrosion potential order is found in [BMPyr] [Nf2] in the order of Ag (0.49 V) > Cu (0.01 V) > In (−0.42 V) > Sn (−0.47 V) > Zn (−0.49 V) except for the reversal of order between Cu and In.
An electrochemical series in the ionic liquids can be established from formal potential, which incorporates an activity coefficient. Although the usage of formal potential is correct in this instance, the formal potential is almost equal to the standard electrode potential considering that the activity coefficient is close to unity when solution concentration is very low. The formal potential was evaluated from the corrosion potential values obtained in Fig. S2† by using Nernst equation as follows:
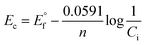 | (1) |
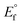 is the formal potential, n is the number of mole of transferred electrons assuming normal oxidation states (1 for Ag and Cu, 2 for Sn and Zn, and 3 for In), F is the Faraday constant (96
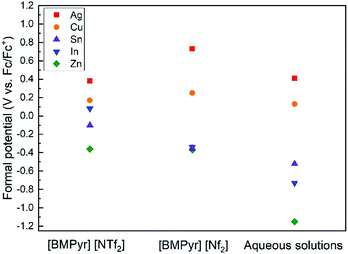
is the formal potential, n is the number of mole of transferred electrons assuming normal oxidation states (1 for Ag and Cu, 2 for Sn and Zn, and 3 for In), F is the Faraday constant (96![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 485 C mol−1), and ci is the concentration of solution (0.1 mM). Fig. 3 compares the formal potentials of Ag, Cu, Sn, In, and Zn in the ionic liquids and aqueous solutions where standard hydrogen reference electrode is converted to Fc/Fc+ reference electrode scale.31 Overall, the order of redox activity is maintained regardless of the kinds of solvents with an exception that the reversal of electrochemical series between Sn and In is observed in the ionic liquids, more notably in [BMPyr] [NTf2]. The absolute values of formal potential are all different depending on the electrolyte systems, and the formal potentials of some metals such as Ag and In are fairly different in the two pyrrolidinium-based ionic liquids of which anions have similar structures. The formal potentials of Ag, Cu, Sn, In, and Zn from Abbott et al.'s electrochemical series in ethaline are directly compared in Fig. S3† on the same Fc/Fc+ reference electrode basis. The absolute values of formal potentials in ethaline appear to shift much from those in [BMPyr] [NTf2] and [BMPyr] [Nf2], taking the relatively smaller variation of formal potentials in between similarly structured [BMPyr] [NTf2] and [BMPyr] [Nf2] into consideration. Noteworthy is the fact that the order of overall redox activity is also maintained in ethaline including the reversal of electrochemical series between Sn and In.
485 C mol−1), and ci is the concentration of solution (0.1 mM). Fig. 3 compares the formal potentials of Ag, Cu, Sn, In, and Zn in the ionic liquids and aqueous solutions where standard hydrogen reference electrode is converted to Fc/Fc+ reference electrode scale.31 Overall, the order of redox activity is maintained regardless of the kinds of solvents with an exception that the reversal of electrochemical series between Sn and In is observed in the ionic liquids, more notably in [BMPyr] [NTf2]. The absolute values of formal potential are all different depending on the electrolyte systems, and the formal potentials of some metals such as Ag and In are fairly different in the two pyrrolidinium-based ionic liquids of which anions have similar structures. The formal potentials of Ag, Cu, Sn, In, and Zn from Abbott et al.'s electrochemical series in ethaline are directly compared in Fig. S3† on the same Fc/Fc+ reference electrode basis. The absolute values of formal potentials in ethaline appear to shift much from those in [BMPyr] [NTf2] and [BMPyr] [Nf2], taking the relatively smaller variation of formal potentials in between similarly structured [BMPyr] [NTf2] and [BMPyr] [Nf2] into consideration. Noteworthy is the fact that the order of overall redox activity is also maintained in ethaline including the reversal of electrochemical series between Sn and In.
Conclusions
We first report the quantitative evaluation of electrochemical series in pure ionic liquids. The electrochemical series in pyrrolidinium-based ionic liquids is established by designing a redox system where only one kind of anion is present in the electrolyte and metal ions are supplied by anodic dissolution. There is the inversion of redox activity between a couple of metals in the electrochemical series in the ionic liquids. Therefore, the unusual galvanic displacement reaction where a less noble metal is deposited on a more noble metal in ionic liquids can be explained by the quantitative electrochemical series in ionic liquids. It is also worth mentioning that the extension of electrochemical series is limited by the amount of electrochemically dissolved metal ions and the onset potential of anodic decomposition of ionic liquids.Conflicts of interest
There are no conflicts to declare.Acknowledgements
This study was supported by the R&D Center for Valuable Recycling (Global-Top Environmental Technology Development Program) funded by the Ministry of Environment (Project No.: 2016002220004).Notes and references
- M. Armand, F. Endres, D. R. MacFarlane, H. Ohno and B. Scrosati, Nat. Mater., 2009, 8, 621 CrossRef CAS PubMed.
- F. Endres, ChemPhysChem, 2002, 3, 144 CrossRef CAS.
- A. P. Abbott and K. J. McKenzie, Phys. Chem. Chem. Phys., 2006, 8, 4265 RSC.
- W. Simka, D. Puszczyk and G. Nawrat, Electrochim. Acta, 2009, 54, 5307 CrossRef CAS.
- J. Park, C. K. Lee, K. Kwon and H. Kim, Int. J. Electrochem. Sci., 2013, 8, 4206 CAS.
- A. P. Abbott, G. Frisch, J. Hartley and K. S. Ryder, Green Chem., 2011, 13, 471 RSC.
- B. Dilasari, Y. Jung, J. Sohn, S. Kim and K. Kwon, Int. J. Electrochem. Sci., 2016, 11, 1482 CAS.
- T. Khoo, P. C. Howlett, M. Tsagouria, D. R. MacFarlane and M. Forsyth, Electrochim. Acta, 2011, 58, 583 CrossRef CAS.
- F. Mizuno, T. S. Arthur and K. Takechi, ACS Energy Lett., 2016, 1, 542 CrossRef CAS.
- H. Tian, S. Zhang, Z. Meng, W. He and W.-Q. Han, ACS Energy Lett., 2017, 2, 1170 CrossRef CAS.
- C. Yang, Q. B. Zhang and A. P. Abbott, Electrochem. Commun., 2016, 70, 60 CrossRef CAS.
- A. Lahiri, N. Borisenko, M. Olschewski, G. Pulletikurthi and F. Endres, Faraday Discuss., 2018, 206, 339 RSC.
- A. Lahiri, G. Pulletikurthi and F. Endres, Front. Chem., 2019, 7, 85 CrossRef CAS PubMed.
- en.wikipedia.org/wiki/Standard_electrode_potential_(data_page).
- en.wikipedia.org/wiki/Galvanic_series.
- J. Park, Y. Jung, P. Kusumah, J. Lee, K. Kwon and K. C. Lee, Int. J. Mol. Sci., 2014, 15, 15320 CrossRef.
- A. P. Abbott, G. Frisch, S. J. Gurman, A. R. Hillman, J. Hartley, F. Holyoak and K. S. Ryder, Chem. Commun., 2011, 47, 10031 RSC.
- S. P. Ong, O. Andreussi, Y. Wu, N. Marzari and G. Ceder, Chem. Mater., 2011, 23, 2979 CrossRef CAS.
- B. Dilasari, Y. Jung, G. Kim and K. Kwon, ACS Sustainable Chem. Eng., 2016, 4, 491 CrossRef CAS.
- M. P. S. Mousavi, B. E. Wilson, S. Kashefolgheta, E. L. Anderson, S. He, P. Buhlmann and A. Stein, ACS Appl. Mater. Interfaces, 2016, 8, 3396 CrossRef CAS PubMed.
- B. Dilasari, Y. Jung and K. Kwon, Electrochem. Commun., 2016, 73, 20 CrossRef CAS.
- A. Cox and D. J. Fray, J. Electrochem. Soc., 2003, 150, D200 CrossRef CAS.
- A. P. Abbott, G. Frisch, J. Hartley, W. O. Karim and K. S. Ryder, Prog. Nat. Sci., 2015, 25, 595 CrossRef CAS.
- Q. Zhou, W. A. Henderson, G. B. Appetecchi, M. Montanino and S. Passerini, J. Phys. Chem. B, 2008, 112, 13577 CrossRef CAS PubMed.
- N. Serizawa, Y. Katayama and T. Miura, Electrochim. Acta, 2010, 56, 346 CrossRef CAS.
- S. Z. E. Abedin, A. Y. Saad, H. K. Farag, N. Borisenko, Q. X. Liu and F. Endres, Electrochim. Acta, 2007, 52, 2746 CrossRef.
- N. Tachikawa, N. Serizawa, Y. Katayama and T. Miura, Electrochim. Acta, 2008, 53, 6530 CrossRef CAS.
- B. Dilasari, Y. Jung and K. Kwon, J. Ind. Eng. Chem., 2017, 45, 375 CrossRef CAS.
- Y.-C. Wang, T.-C. Lee, J.-Y. Lin, J.-K. Chang and C.-M. Tseng, Corros. Sci., 2014, 78, 81 CrossRef CAS.
- H. Lei, X. Li, C. Sun, J. Zeng, S. S. Siwal and Q. B. Zhang, Small, 2019, 15, 1804722 CrossRef PubMed.
- I. Noviandri, K. N. Brown, D. S. Fleming, P. T. Gulyas, P. A. Lay, A. F. Masters and L. Phillips, J. Phys. Chem. B, 1999, 103, 6713 CrossRef CAS.
Footnotes |
| † Electronic supplementary information (ESI) available: Experimental methods, calculation of dissolution potential, Fig. S1 and S2. See DOI: 10.1039/d0ra03598j |
| ‡ Present address: Department of Chemical Engineering, The City College of New York, 160 Convent Ave, New York, NY 10031, USA. |
| This journal is © The Royal Society of Chemistry 2020 |