Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
One-step synthesis of polyethyleneimine-coated magnetite nanoparticles and their structural, magnetic and power absorption study
Lizbet León Félix *a,
Marco Antonio Rodriguez Martínezb,
David Gregorio Pacheco Salazar
*a,
Marco Antonio Rodriguez Martínezb,
David Gregorio Pacheco Salazar a and
José Antonio Huamani Coaquira
a and
José Antonio Huamani Coaquira ab
ab
aLaboratorio de Películas Delgadas, Escuela Profesional de Física, Universidad Nacional de San Agustín de Arequipa, Av. Independencia s/n, Arequipa, Peru. E-mail: lleonf@unsa.edu.pe
bLaboratory of Magnetic Characterization, Instituto de Física, Universidade de Brasília, DF 70910-900, Brasília, Brazil
First published on 17th November 2020
Abstract
Magnetic nanoparticles (NPs) are especially interesting for several biomedical applications due to their chemical surface, especially for targeted cancer imaging and therapeutics. In order to realize these applications, it is important to know their magnetic properties among other complementary properties that help to improve the understanding of the synthesis process. In this work, we report the magnetic properties of polyethyleneimine-coated magnetite (PEI-Fe3O4) NPs synthesized by a one-step method via the co-precipitation method and using PEI as a stabilizer. Transmission electron microscopy (TEM) images revealed agglomerated magnetic nanoparticles with an average size of ∼10 nm; meanwhile, the X-ray diffraction (DRX) analysis confirmed a pure magnetite phase. The study of magnetic properties shows a superparamagnetic system with coexistence of non-interacting single NPs with a low blocking temperature (∼35 K) and interacting NPs in the aggregates with a higher blocking temperature (>150 K), in which the interparticle interactions of magnetic cores dominate over surface spin disorder. The interaction between the surface spin-disorder layer and NP core was found to be weak, related to a weak exchange bias effect. A maximum specific loss power (SLP) value of 70 W g−1 was obtained (f = 571 kHz and H = 23.87 kA m−1) indicating that the magnetic response plays a crucial role in determining the heating efficiency for future applications.
1. Introduction
Iron oxide magnetic NPs with an appropriate biocompatible coating to improve colloidal stability1 and a good biocompatibility or low cytotoxicity are increasingly used in many biomedical applications including protein separation,2 drug and gene delivery,3 magnetic resonance imaging (MRI)4 and magnetic hyperthermia therapy.5,6Magnetic hyperthermia is an alternative to therapeutic treatments which uses hysteresis cycle of magnetic nanoparticles (MNPs) to locally increases cancer cells temperature to values of 43–45 °C inducing their apoptosis or death.7 The heating capacity of MNPs with colloidal stability under an alternating magnetic field (AMF) is quantified by the specific loss power (SLP).8 In order to improve the heating process, it is important to know the influence of the different parameters such as size and shape of NPs, solvent, colloidal stability, NPs biocompatibility, and intrinsic magnetic properties. Those parameters can lead to a more complex behavior of the system that could affect the heating efficiency of the NPs.
Also, it is important to consider the influence of the surface, interface effects such as surface spin disorder, defects, breaking of exchange bonds, changes in the surface atom coordination number,9 and also the distance among MNPs assemblies that leads to interparticle interactions10 that can dominate over single domain nanoparticles response and affect the heating efficiency.
Some nanoparticulated features such as the surface spin disorder and magnetic frustrations, related to the presence of structurally disordered grain boundaries, can provide a dominant contribution to the effective anisotropy and lead to a surface spin-glass like state at low temperatures.11 It is known that the occurrence of a spin-glass state is related to a magnetic disorder, randomness (exchange, anisotropy, field), and frustrations,12 where the competing interactions (ferromagnetic and antiferromagnetic) show equivalent strengths.
In addition, in a system where interparticle interactions are non-negligible, the system eventually shows collective behavior, which overcomes the anisotropy properties of individual particles, and leads to the increase of the blocking temperature (TB). When the interparticle interactions are strong enough in a nanoparticle ensemble,13 also can lead to the spin-glass behavior besides the increment of the TB.14
In particle systems, collective effects related to different kinds of magnetic interactions play an important role such as the long-range dipole–dipole interactions that are the predominant mechanism; meanwhile, the short-range exchange interactions play a leading role in nanoparticle assemblies, where the electrons at the surface of the particles are in close contact12 and both mechanisms may exist simultaneously. De Toro et al. reported that despite the close contact of the maghemite particles (with nonmagnetic shell thickness < 3 nm) the superexchange interactions play a minor role in establishing the collective, and superspin-glass state of the NPs below a critical temperature in comparison to dipolar interactions.15 The origin of the superspin-glass behavior is strongly related to the interplay of intra- and interparticle interactions effects, where the role of dipolar interactions is very important for establishing the superspin-glass phase.14
Magnetic NPs systems can exhibit the so-called exchange bias (EB) effect, which is related to the exchange coupling between core and surface spins at the interface and the interparticle exchange coupling. A study of NPs with Fe/Fe oxide core (10 nm)/shell (3.5 nm) structure shows a superparamagnetic behavior and the structural disorder in particles outer shell could lead to a larger number of uncompensated spins at the interface of the core–shell structure, which, in turn, causes a high magnetocrystalline anisotropy and an enhanced EB effect (22 kA m−1 at 5 K and using a field of 1591.5 kA m−1 to cool the system).16 On the other hand, it has been reported an EB effect for a core–shell structure of Fe3O4/γ-Fe2O3 NPs of 12 nm size with an EB field of 11.14 kA m−1 at 10 K and after field cooling with 37.8 kA m−1 (ref. 17) the authors reported that there is an intrinsic single-particle property, as the large magnetic anisotropy of the γ-Fe2O3 shell with a spin glass-like behavior or a possibly disordered magnetic state no related to the interparticle interaction.18 Meanwhile, the observed EB effect in ultrasmall ∼2 nm MnFe2O4 NPs has been assigned to the exchange coupling between core and surface spins at the interface and the interparticle exchange coupling.16 In magnetite NPs of 40 nm a spin-glass like behavior with a freezing temperature of ∼35 K was determined and could be observed in both, the in-phase and the out-phase magnetic susceptibility curves.19 These characteristics can be tuned during the synthesis process.
So far, various experimental methods have been employed to produce PEI coated magnetic NPs such as solvothermal, hydrothermal, and co-precipitation methods. They have been applied for the synthesis at high temperatures,20 during long periods of synthesis6 using several reaction steps,21,22 such as first core synthesis, and after coated with PEI.23–27 PEI molecule is considered a good candidate for the functionalization of other molecules, the biocompatibility and stability of MNPs, that are important in the fields of biomedicine.28
In order to improve and simplify the synthesis for the formation of PEI-coated MNPs, we propose an effective procedure that combines co-precipitation steps in a one-step procedure in an aqueous medium. The design of PEI-MNPs has a huge importance in the heat generation via electromagnetic energy conversion.
In this work, we report a simple route for an efficient and facile one-step PEI-Fe3O4 NPs synthesis, using the co-precipitation method. We obtained NPs of ∼9.4 nm in size, and the study of their magnetic properties and the power absorption response are presented and discussed. The phase, crystal structure, and magnetic properties were characterized by X-ray diffraction, transmission electron microscopy, dc, and ac magnetic measurement and power absorption for future applications in magnetic hyperthermia.
2. Experimental section
2.1 Chemicals
Iron(III) chloride hexahydrate (FeCl3·6H2O), iron(II) chloride tetrahydrate (FeCl2·4H2O), sodium hydroxide (NaOH) and polyethylenimine branched (PEI) with a molecular weight of ∼25![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 were purchased from Sigma Aldrich.
000 were purchased from Sigma Aldrich.
2.2 Synthesis of polyethyleneimine coated magnetite nanoparticles
The synthesis protocol used for the samples was based on a co-precipitation method. At the beginning of the synthesis, 8 mol L−1 NaOH solution (water) was heated up to 90 °C by vigorous constant stirring and bubbled with N2. After 10 min, the mixture of 1 mol L−1 FeCl2 and 2 mol L−1 FeCl3 with 2.1 g PEI (25 kDa) was dissolved in distilled water and was added in a basic solution while constantly stirring. Then the system was maintained at 90 °C for 2 h under N2. When the precipitation was completed, the suspension with black precipitate was removed from the heating source and cooled at room temperature with an ice bath then washed with water several times in order to isolate the supernatants by magnetic decantation, and re-dispersed in water until getting pH 7, the particle presented a good water dispersibility and stability. The final product was dried with N2 flux.2.3 Characterization
The morphology and structure of the resulting NPs were analyzed by a high resolution transmission electron microscopy (HRTEM), using an FEI Tecnai F30 microscope operated at an acceleration voltage of 300 kV. Lattice fringes were measured from the fast-Fourier transform of HRTEM images analyses, using Gatan Digital Micrograph software. The mean particle size, and its polydispersion index, σ, were obtained by calculating the average number manually measuring the equivalent diameter of N > 500 particles from TEM micrographs. XRD patterns were obtained using a Rigaku Miniflex 600 diffractometer operating at 30 mA and 40 kV from 20 to 80° (2θ value) using Cu K-α radiation (0.15418 nm). The samples were prepared placing a concentrated NPs suspension drop on a zero-diffraction silicon wafer. The Rietveld method analysis was used to confirm the structural analysis of NPs. The lattice parameters were determined using the GSAS (General Structure Analysis System) refinement. The magnetic properties of the sample were measured by ac and dc magnetic susceptibility measurements, performed on a Superconducting Quantum Interference Device (SQUID) magnetometer, model MPMS3 (Quantum Design). The magnetization curves were recorded using a maximum applied field of 5570.4 kA m−1 at temperatures of 5 and 300 K. For zero-field-cooled (ZFC) and field-cooled (FC) curves were measured at temperatures between 2 to 300 K, with a cooling field HFC = 2.39 kA m−1 (30 Oe). For ac magnetic susceptibility measurements, the data was obtained in a temperature range of 2 to 300 K, and frequencies ranging from 0.2 to 1 kHz and under an excitation field Hac = 400 A m−1. The Specific Loss Power (SLP) of the SLP NPs was measured under AMFs as a function of the field amplitude (13.53 ≤ H0 ≤ 23.87 kA m−1) and a fixed frequency of f = 571 kHz. At 478 nm, the wavelength was used to measure the absorbance of NPs and for determining the iron concentration.3. Results and discussion
3.1 Structural and morphological characterization
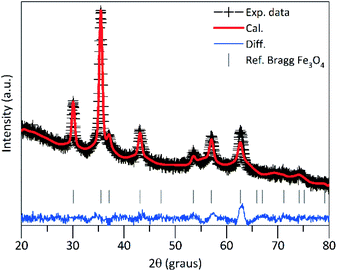
In Fig. 1 is shown the ray-X diffraction data analysis of the PEI-FE3O4 NPs. The XRD pattern was refined using the Rietveld refinement method, which indicates that all diffraction peaks correspond to the cubic spinel structure of magnetite (space group: Fd![[3 with combining macron]](https://www.rsc.org/images/entities/char_0033_0304.gif) m). The lattice parameter calculated from the XRD pattern is ≈8.37 Å, which is very close to the standard lattice parameter of bulk magnetite (8.39 Å). Additionally, the full width at half-maximum of the peaks was used to estimate the crystallite size (DXRD) from the Scherrer equation. From this analysis, the calculated value was DXRD ≈ 10.1 nm.
m). The lattice parameter calculated from the XRD pattern is ≈8.37 Å, which is very close to the standard lattice parameter of bulk magnetite (8.39 Å). Additionally, the full width at half-maximum of the peaks was used to estimate the crystallite size (DXRD) from the Scherrer equation. From this analysis, the calculated value was DXRD ≈ 10.1 nm.
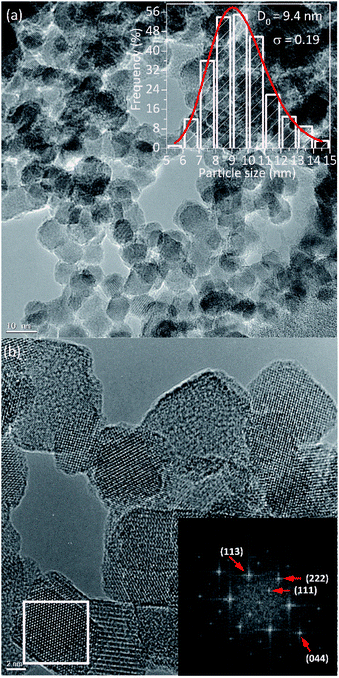
Fig. 2 shows the TEM images of PEI-coated Fe3O4 NPs, which were used to determine the structure, morphology, and size distribution. After counting N = 518 sizes, the histogram built using the Sturges criterion could be fitted with a log-normal distribution, yielding an average size of 〈d〉 = 9.6 nm and a polydispersion index σ = 0.19 nm (see Fig. 2a). As observed in the images, the NPs show mainly spherical and octahedral shapes, and also agglomeration of particles is observed that could mimics a large particle. Especially, Fig. 2b displays the high-resolution TEM images with clear lattice fringe patterns, which confirm the high crystalline quality of the sample. The Fourier transform of the HR-TEM images show spots corresponding to the spacing distances of 4.85 Å, 2.48 Å, 2.59 Å and 1.55 Å, which are consistent with the interplanar distances of (111), (222), (113) and (044) lattices planes of magnetite, respectively.
3.2 Magnetic characterization
In order to characterize the magnetic properties in detail, the static and dynamic magnetic responses were investigated. We begin the analysis with the estimation of the TB of non-interacting particles, using the average size obtained by TEM and the relation, TB = KeffV/25kB, where kB is the Boltzmann constant, considering the anisotropy constant (Keff) of magnetite bulk. A mean value of 〈TB〉 ≈ 22 K was obtained.In Fig. 3 are shown zero-field-cooled (ZFC) and field-cooled (FC) magnetization curves of PEI-Fe3O4 NPs obtained with an applied field of 2.39 kA m−1. The ZFC curve shows, a broad maximum temperature at Tmax ∼ 195 K and a shoulder in the low-temperature region at around 30 K. The high Tmax value suggests the occurrence of strong dipolar interparticle interactions29 due to the NPs being very close to each other, forming particle aggregates as observed by TEM images, which favor the interparticle interactions. Moreover, the shoulder could be related to the blocking of non-interacting single particles. At temperatures below TB, the magnetic moments of the particles must show random orientation in a zero-field cooled condition and cannot rotate freely.30 Then, the system would exhibit a transition from a high-temperature superparamagnetic state to a low temperature blocked state.
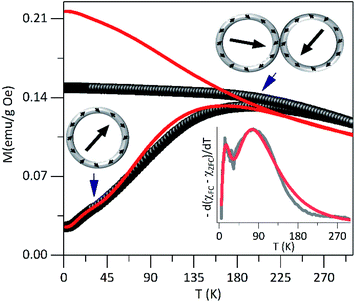 | ||
| Fig. 3 The dc magnetization curves in ZFC–FC modes for PEI-Fe3O4 NPs, carried out at 2.39 kA m−1. In the inset is shown the simulated data fit (red solid lines). | ||
The distribution of TB can be obtained from ZFC–FC curves according to f(T) ≈ d(MZFC − MFC)/dT.31,32 As it is shown in the inset of Fig. 3, the experimental data shows bimodal features that suggest the occurrence of two TB distributions, which can be modeled using the lognormal distribution. The fit provides two maxima at 〈T1B〉 ∼ 25 K and 〈T2B〉 ∼ 105 K. The 〈T1B〉 value is close to the one expected for non-interacting single particles with the determined size from TEM images, and 〈T2B〉 is not directly related to the particle size distribution but could be associated with the presence of interactions among close particles or particle agglomerations that promote the interparticle interactions. Those values obtained from the fit of the f(T) ≈ d(MZFC − MFC)/dT vs. T curve was used to simulate the ZFC and FC curves.
It is known that the effect of the particle size distribution gives rise to a distribution of TB, which can drive to the superposition of responses coming from the superparamagnetic state (first term in eqn (1) and (2)) and in the blocked state (second term in eqn (1) and (2)).33,34 Then, the ZFC (eqn (1)) and FC (eqn (2)) susceptibility can be given by:
 | (1) |
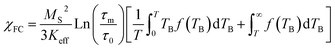 | (2) |
These results confirm the presence of magnetic interactions among particles35 that displaces TB to high temperatures. The magnetic interactions such as exchange interaction36 might be negligible due to particle separation, by the presence of PEI on the particle surface. However, the dipolar interparticle interactions,37 which remain important at larger distances, would be the ones that rule the magnetic behavior of the system. In addition, the surface effects are not negligible due to the lack of translational symmetry, the low coordination number of magnetic ions,9 and the existence of broken magnetic exchange bonds, which are responsible for the surface spin disorder.38
The irreversibility temperature onset between the experimental ZFC and FC curves is around 295 K that reflects the strong magnetic interaction. The experimental FC curve grows weakly as the temperature decreases and shows a tendency towards saturation below TB, but also it shows clear differences concerning the theoretical FC curve. This finding corroborates the presence of magnetic interactions37,38 in the system.
The hysteresis loops measured at 300 K for PEI-Fe3O4 NPs are shown in Fig. 4a. The saturation magnetization (MS) does not reach a saturation value, neither at 300 K nor 5 K, even at the highest applied field of 7 T. Suggesting that the occurrence of a strong anisotropy field and/or magnetic disorder at the surface layer,39 that makes difficult the alignment along the field direction. To estimate the MS, we use the approach to saturation model,40 providing MS values of 66.5 Am2 kg−1 and 79.4 Am2 kg−1 for 300 K and 5 K, respectively. The smaller values in comparison to the one expected for bulk magnetite (MS ≈ 92 Am2 kg−1)41 is due to the non-magnetic mass present in our samples. We also observed the absence of coercive field and remanence magnetization above 150 K, confirming the superparamagnetic regime above this temperature, where the thermal energy is predominant and the orientations of magnetic moments are random, so the M(H) curve shows reversible trend.
The M(H) curve at 300 K can be fitted to the Langevin function42 since relaxed states are expected. Accordingly, the magnetization is described by 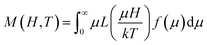 , where the log-normal distribution of magnetic moments is considered, and the mean magnetic moment (〈μ〉) of each particle is related to its volume (〈V〉) by 〈μ〉 = MS〈V〉,43–45 where MS is the saturation magnetization. The best-fitting is achieved with the parameters μ0 = 9747μB, and σ = 1.61 as shown in Fig. 4a, where μ0 is the median of the distribution related to the mean magnetic moment and σ is the polydispersion index. From these results, we can estimate the mean magnetic moment value given by 〈μ〉 = μ0
, where the log-normal distribution of magnetic moments is considered, and the mean magnetic moment (〈μ〉) of each particle is related to its volume (〈V〉) by 〈μ〉 = MS〈V〉,43–45 where MS is the saturation magnetization. The best-fitting is achieved with the parameters μ0 = 9747μB, and σ = 1.61 as shown in Fig. 4a, where μ0 is the median of the distribution related to the mean magnetic moment and σ is the polydispersion index. From these results, we can estimate the mean magnetic moment value given by 〈μ〉 = μ0![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) exp(σ2/2). Assuming spherical particles and using the equation: 〈μ〉 = MS〈V〉 = πMS〈D〉3/6, a value of 〈D〉 ≈ 10.1 nm, is estimated for the mean particle size. This value is in excellent agreement with the particle size value determined from TEM data analysis (∼9.6 nm). Besides, Fig. 4a shows a simulated M(H) curve considering parameters obtained from TEM and the Langevin function. This result suggests that the relaxed states (superparamagnetic state of non-interacting particles) is influenced by the magnetic intra- and interparticle interactions and the magnetic polydispersion index.
exp(σ2/2). Assuming spherical particles and using the equation: 〈μ〉 = MS〈V〉 = πMS〈D〉3/6, a value of 〈D〉 ≈ 10.1 nm, is estimated for the mean particle size. This value is in excellent agreement with the particle size value determined from TEM data analysis (∼9.6 nm). Besides, Fig. 4a shows a simulated M(H) curve considering parameters obtained from TEM and the Langevin function. This result suggests that the relaxed states (superparamagnetic state of non-interacting particles) is influenced by the magnetic intra- and interparticle interactions and the magnetic polydispersion index.
In Fig. 4b is showing the HC vs. T curve obtained from the M(H) curves at different temperatures for the PEI-Fe3O4 NPs. As it is observed, the HC shows an increase with temperature decrease. The inset of Fig. 4b shows the M(H) curve measured at 5 K, with an HC ∼ 311 Oe. It is known HC is very sensitive to factors such as anisotropy type,14 size and distribution of particles, morphology, surface spin disorder, and interparticle interaction.37 In our sample, we must consider the surface disorder, and the interparticle interaction which delays the thermal relaxation of the magnetic moments of NPs, are predominant factors that determine the value of HC.
However, the temperature dependence of the coercive field can be modeled taking into account the particle size distribution (distribution of TB obtained from the ZFC–FC analysis) and/or the interaction effects. The coercive field is given by 〈HC〉T = Mr(T)/(χr + Mr(T)/HCB(T)), where Mr is the remanence magnetization, χr is the susceptibility of the superparamagnetic particles, and HCB is the coercive field of blocked particles. An empirical parameter γ is included in f(γTB) which is related to the particle interactions.34,46 As shown in the main panel of Fig. 4b, the fit provides a γ = 0.8, which is close to the value expected for systems with negligible interparticle interactions (γ = 1).46
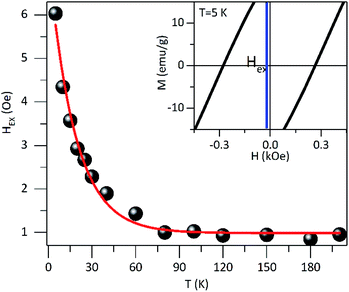
In order to explore possible exchange coupling effects between the disordered surface spins and the magnetic core, we measured the M(H) loops at low temperature after field-cooling the sample with a field HFC = 1591.5 kA m−1, to ascertain the presence of an EB anisotropy field (HEX). Fig. 5 shows the presence of an exchange field47 for temperatures below ≈60 K, with a thermal dependence of exponential type down to T = 5 K.
The HEX origin was assigned to the coupling of a layer of disordered spins at the particle surface and a well-ordered region of spin in the core region of the particle. The core spins exert torque on the surface spins that do not follow the anisotropy direction of the core due to the disorder, leading to the HEX occurrence. To evaluate the exchange anisotropy at low temperatures we use the HEX thermal dependence, given by HEX(T) = HEX(0)exp(−BT) where HEX(0) is the EB field at T = 0 K and B is a constant. The fit provides a HEX(0) = 0.5 kA m−1 and B = 5.5 10−2 K−1. The small value of HEX(0) shows the EB effect is rather weak, and it seems to be associated with the magnetic coupling between the disordered surface spins and the magnetic core. This is consistent with previous reports on core–shell Au/Fe3O4 NPs where the disordered spins are present at both the inner and outer surface of the magnetite shell in the Au/Fe3O4 NPs.34
The HEX low value indicates that the two magnetic regions are weakly coupling, where the effective anisotropy of the core is expected to be greater to achieve the reversal of the surface spins region and where the surface spins rearrange faster than core spins. It is worth mentioning that we are considering just the intrinsic origin of HEX and dipolar interactions do not affect the value of HEX, which could result in HEX reduction in large cooling fields18 and HEX increase in small particles due to the fact that shell is thicker than core.17
Fig. 6 shows the in-phase (χ′) and the out-of-phase (χ′′) susceptibility components as a function of temperature with a range from 0.2 Hz to 1 kHz and in an oscillating magnetic field of 0.08 kA m−1. As was observed, the peak position of χ' × T curve is located at ∼200 K for the lowest frequency (0.2 Hz) and this maximum was shifted to higher temperatures with the frequency increase. This high-temperature peak is expected for NPs with interparticle interactions and this behavior was also observed in χ′′ × T curve (Fig. 6b). Also, a shoulder in the low temperature that clearly shows a dependence on frequency is evidenced, as shown in the dχ′′/T × dT curve (inset on Fig. 6b). It could be attributed to non-interacting particles. Other low-temperature reports were assigned to a significant amount of surface spin disorder at 15 K for 7 nm maghemite NPs48 while spin-glass-like-transition at 35 K for 40 nm magnetite NPs.19
Considering that non-interacting particles where the barrier energy is determined by the uniaxial anisotropy, the spin relaxation is a thermally activated process, as is proposed by the Néel–Arrhenius model, τ = τ0![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) exp(Ea/kBT), where Ea/kB is the activation energy. Assuming that the temperatures in χ′′(T,f) correspond to the TB and the low-temperature peak is best observed in dχ′′/dT vs. T curve (inset on see Fig. 6b). The ln(τ) × 1/T curve fit (see Fig. 6c) provides values of τ1–0 = 1.9 10−8 s, Ea/kB = 325 K and τ2–0 = 9.8 10−14 s, Ea/kB = 4224 K for the low and high temperature peaks, respectively. The characteristic time τ1–0 is very close to the values reported for non-interacting NPs in an applied field (10−9–10−11 s) close to zero, while the τ2–0 is consistent with interacting NPs.39
exp(Ea/kBT), where Ea/kB is the activation energy. Assuming that the temperatures in χ′′(T,f) correspond to the TB and the low-temperature peak is best observed in dχ′′/dT vs. T curve (inset on see Fig. 6b). The ln(τ) × 1/T curve fit (see Fig. 6c) provides values of τ1–0 = 1.9 10−8 s, Ea/kB = 325 K and τ2–0 = 9.8 10−14 s, Ea/kB = 4224 K for the low and high temperature peaks, respectively. The characteristic time τ1–0 is very close to the values reported for non-interacting NPs in an applied field (10−9–10−11 s) close to zero, while the τ2–0 is consistent with interacting NPs.39
The high-temperature peak analysis with the Neél model suggests taking into account the presence of interparticle interactions, and that can be assessed using the Vogel–Fulcher model,49 τ = τ0![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) exp(Ea/(kB(Tmax − T0))), where T0 is a characteristic temperature which magnifies the interaction energy among the NPs and Tmax is the onset temperature of the blocked state. Considering a characteristic time of τ0 = 10−9 s, he obtained parameters from the experimental data fit are T0 ∼ 56 K and Ea/kB ∼ 1760 K. According to what was reported by Yasin et al., when T0 > 0 has a collection of interacting spins; meanwhile, when T0 < 0 indicates a spin-glass system.50
exp(Ea/(kB(Tmax − T0))), where T0 is a characteristic temperature which magnifies the interaction energy among the NPs and Tmax is the onset temperature of the blocked state. Considering a characteristic time of τ0 = 10−9 s, he obtained parameters from the experimental data fit are T0 ∼ 56 K and Ea/kB ∼ 1760 K. According to what was reported by Yasin et al., when T0 > 0 has a collection of interacting spins; meanwhile, when T0 < 0 indicates a spin-glass system.50
Besides that, in the absence of an applied low magnetic field, Ea is given by Ea = KeffV, where Keff is the effective magnetic anisotropy constant. Using the NPs size obtained from TEM data analysis, we estimated a K1-eff = 104 J m−3 for the low-temperature peak. The K1-eff value is very close to the magnetocrystalline value of bulk magnetite (1.35 104 J m−3).51 In addition, the value obtained from Ea/kB was used to estimate the NPs mean diameter (considering them as spherical). Using Keff of bulk magnetite, a diameter size of ∼8.7 nm was calculated being this very close to the size found by TEM while ∼21 nm corroborates the presence of agglomerated NPs.
For further characterization, we used the empirical parameter, ϕ, given by:49 ϕ = ΔTf/TfΔlog10(f), which is the relative shift of the peak temperature (Tf) per AC frequency decade change. The experimental data provides a value of ϕ = 0.14 for the low-temperature peak. This value is very close to the ones reported for non-interacting particles or superparamagnetic systems (0.10 < ϕ< 0.13). For the high-temperature peak, a ϕ = 0.071 was determined, which is in the range of values corresponding to interacting particles (0.03 < ϕ < 0.1).51 From these results, we can infer that the studied system contains both non-interacting NPs that present superparamagnetic behavior and interacting NPs associate with the aggregates that show collective response even to the presence of PEI.
3.3 Power absorption
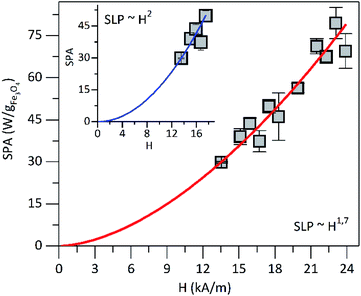
Further characterization was performed to assess the heating ability of PEI-FE3O4 NPs in the aqueous medium at pH 7. In order to determine the magnetic properties impact (as surface effects, interparticle interactions) in the heating efficiency of the superparamagnetic PEI-Fe3O4 NPs which is quantified by in the specific loss power (SLP). In Fig. 7 is shown the SLP value which increases with the increasing field in agreement with reports in the literature.52For fields below 17.51 kA m−1 and using the power law, the SLP displays a field dependence of SLP ∼ H2 (see the inset in Fig. 7), which is in agreement with the linear response theory (LRT) for superparamagnetic particles as predicted by Roseinsweig.53 However, for all measured field values, the dependence is SLP ∼ H1.7, which is not predicted by the LRT. On the other hand, the measured SLP value at 23.87 kA m−1 was of ∼70 W g−1 for the PEI-Fe3O4 NPs studied in this work. In Table 1 is summarized the SLP values reported in the literature for iron oxide NPs with different sizes, surface coatings, AMF, and frequency. The SLP value obtained in this work is comparable to the values reported in the literature as observed in Table 1. Therefore, we concluded that the parameters followed in this work were optimized in such a way that the efficiency to transform electromagnetic energy into heat was higher than those reported in the literature. Furthermore, the differences among the SLP values seems to be related to the dependence on parameters such as particle size distributions,54 coating, NPs aggregation, hydrodynamic size, presence of magnetic interactions,55 magnetic anisotropy,56 magnetic volume,57 and/or different experimental conditions, among others. All these factors can affect the LRT behavior of power loss efficiency.
| Name | Surface coating | Core size [nm] | SLP [W g−1] | Measured conditions [kA m−1]/[kHz] | Ref. |
|---|---|---|---|---|---|
| a The solvent used was hexane and water, in the others. | |||||
| Fe3O4 | PEI-poly(acrylic acid-co-maleic acid) | 10.3 | 15 | 7.28/621.7 | 58 |
| Fe3O4 | Polyethylene glycol | 11.0 | 4.5 | 3.2/600 | 59 |
| Fe3O4 | 10.0 | 16 | ∼8/524.2 | 60 | |
| Iron oxidea | Oleic acid-oleylamine-trioctylphosphine oxide | 8.8 | 80.7 | 23.87/580.5 | 61 |
| Fe3O4 | 10.2 | 63 | 9.8/276 | 62 | |
| Fe3O4 | PEI-poly(acrylic acid-co-maleic acid) | 10.0 | 12.15 | 11.94/111 | 63 |
| Fe3O4 | Oleic acid | 8.0 | 33.5 | 26.6/265 | 64 |
| Fe3O4 | PEI | 9.6 | 70 | 23.87/571 | This work |
4. Conclusions
PEI-coated Fe3O4 NPs of narrow size distribution and mean size of ∼10 nm were successfully synthesized by a one-step co-precipitation route. The systematic investigation of the magnetic properties suggests the presence of non-interacting particles showing a superparamagnetic behavior with a low blocking temperature (∼35 K) and interacting particles, likely forming agglomerates, with a higher blocking temperature (>150 K), in which the surface spin disorder is weak and dominated by interparticle interactions. A high SLP value of ∼70 W g−1 was obtained (at 571 kHz and 45 kA m−1). Therefore, the magnetic properties play a crucial role in determining the heating efficiency obtained in this work, which is attractive for future application.Conflicts of interest
The authors declare no competing financial interest.Acknowledgements
This work was financially support by CONCYTEC – FONDECYT within the framework of the call E038-01 [contract no. 07-2019-FONDECYT-BM-INC. INV]. J. A. H. C. thanks to the Brazilian agencies CNPq [grants number 301455/2017-1, 443652/2018-0] and FAPDF [grant number 00193.0000151/2019-20] for the financial support. The authors would like to thank Dr Gerardo. F. Goya, Dr R. Fernández-Pacheco and Dr A. Ibarra and the Laboratorio de Microscopias Avanzadas for their advices and technical support with the HRTEM analysis, and to the Servicios Científco Técnicos CIBA-IACS-UZ for the use of its facilities.References
- H. Cai, X. An, J. Cui, J. Li, S. Wen, K. Li, M. Shen, L. Zheng, G. Zhang and X. Shi, ACS Appl. Mater. Interfaces, 2013, 5, 1722–1731 CrossRef CAS
.
- S. Bucak, D. A. Jones, P. E. Laibinis and T. A. Hatton, Biotechnol. Prog., 2003, 19, 477–484 CrossRef CAS
.
- M. Dowaidar, H. Nasser Abdelhamid, M. Hällbrink, K. Freimann, K. Kurrikoff, X. Zou and Ü. Langel, Sci. Rep., 2017, 7, 9159 CrossRef
.
- Y. Bao, J. A. Sherwood and Z. Sun, J. Mater. Chem. C, 2018, 6, 1280–1290 RSC
.
- Z. Li, M. Kawashita, N. Araki, M. Mitsumori, M. Hiraoka and M. Doi, Mater. Sci. Eng., C, 2010, 30, 990–996 CrossRef CAS
.
- L. León Félix, B. Sanz, V. Sebastián, T. E. Torres, M. H. Sousa, J. A. H. Coaquira, M. R. Ibarra and G. F. Goya, Sci. Rep., 2019, 9, 4185 CrossRef
.
- A. Hervault and N. T. K. Thanh, Nanoscale, 2014, 6, 11553–11573 RSC
.
- Y. Hadadian, A. P. Ramos and T. Z. Pavan, Sci. Rep., 2019, 9, 18048 CrossRef CAS
.
- R. . Kodama, J. Magn. Magn. Mater., 1999, 200, 359–372 CrossRef CAS
.
- G. T. Landi, Phys. Rev. B: Condens. Matter Mater. Phys., 2014, 89, 014403 CrossRef
.
- E. Bonetti, L. Del Bianco, D. Fiorani, D. Rinaldi, R. Caciuffo and A. Hernando, Phys. Rev. Lett., 1999, 83, 2829–2832 CrossRef CAS
.
- S. Bedanta and W. Kleemann, J. Phys. D: Appl. Phys., 2009, 42, 013001 CrossRef
.
- P. Dutta, A. Manivannan, M. S. Seehra, N. Shah and G. P. Huffman, Phys. Rev. B: Condens. Matter Mater. Phys., 2004, 70, 174428 CrossRef
.
- B. Aslibeiki, M. H. Ehsani, F. Nasirzadeh and M. A. Mohammadi, Mater. Res. Express, 2017, 4, 075051 CrossRef
.
- J. A. De Toro, P. S. Normile, S. S. Lee, D. Salazar, J. L. Cheong, P. Muñiz, J. M. Riveiro, M. Hillenkamp, F. Tournus, A. Tamion and P. Nordblad, J. Phys. Chem. C, 2013, 117, 10213–10219 CrossRef CAS
.
- M. Vasilakaki, G. Margaris, D. Peddis, R. Mathieu, N. Yaacoub, D. Fiorani and K. Trohidou, Phys. Rev. B, 2018, 97, 094413 CrossRef CAS
.
- A. Ceylan, C. C. Baker, S. K. Hasanain and S. Ismat Shah, J. Appl. Phys., 2006, 100, 034301 CrossRef
.
- Y. Hwang, S. Angappane, J. Park, K. An, T. Hyeon and J.-G. Park, Curr. Appl. Phys., 2012, 12, 808–811 CrossRef
.
- K. L. López Maldonado, P. de la Presa, E. Flores Tavizón, J. R. Farias Mancilla, J. A. Matutes Aquino, A. Hernando Grande and J. T. Elizalde Galindo, J. Appl. Phys., 2013, 113, 17E132 CrossRef
.
- H. Zhao, C. Zhang, D. Qi, T. Lü and D. Zhang, J. Dispersion Sci. Technol., 2019, 40, 231–238 CrossRef CAS
.
- X. Wang, L. Zhou, Y. Ma, X. Li and H. Gu, Nano Res., 2009, 2, 365–372 CrossRef CAS
.
- A. H. Nguyen, G. N. Abdelrasoul, D. Lin, H. Maadi, J. Tong, G. Chen, R. Wang, A. Anwar, L. Shoute, Q. Fang, Z. Wang and J. Chen, Appl. Nanosci., 2018, 8, 811–821 CrossRef CAS
.
- I. Karimzadeh, M. Aghazadeh, T. Doroudi, M. Ganjali and P. Kolivand, Curr. Nanosci., 2017, 13, 167–174 CrossRef CAS
.
- W. Lu, M. Ling, M. Jia, P. Huang, C. Li and B. Yan, Mol. Med. Rep., 2014, 9, 1080–1084 CrossRef CAS
.
- L. Ma, N. Sun, J. Zhang, C. Tu, X. Cao, D. Duan, A. Diao and S. Man, Nanoscale, 2017, 9, 17699–17703 RSC
.
- J.-H. Kim, S.-M. Kim, I.-H. Yoon, S.-J. Choi and I. Kim, Sci. Total Environ., 2020, 706, 136020 CrossRef CAS
.
- S. S. Rohiwal, N. Dvorakova, J. Klima, M. Vaskovicova, F. Senigl, M. Slouf, E. Pavlova, P. Stepanek, D. Babuka, H. Benes, Z. Ellederova and K. Stieger, Sci. Rep., 2020, 10, 4619 CrossRef CAS
.
- Z. Chen, Z. Lv, Y. Sun, Z. Chi and G. Qing, J. Mater. Chem. B, 2020, 8, 2951–2973 RSC
.
- R. Prozorov and T. Prozorov, J. Magn. Magn. Mater., 2004, 281, 312–317 CrossRef CAS
.
- J. Vejpravová, V. Sechovský, J. Plocek, D. Nižňanský, A. Hutlová and J.-L. Rehspringer, J. Appl. Phys., 2005, 97, 124304 CrossRef
.
- G. Muscas, G. Concas, S. Laureti, A. M. Testa, R. Mathieu, J. A. De Toro, C. Cannas, A. Musinu, M. A. Novak, C. Sangregorio, S. S. Lee and D. Peddis, Phys. Chem. Chem. Phys., 2018, 20, 28634–28643 RSC
.
- I. J. Bruvera, P. Mendoza Zélis, M. Pilar Calatayud, G. F. Goya and F. H. Sánchez, J. Appl. Phys., 2015, 118, 184304 CrossRef
.
- J. C. Denardin, A. L. Brandl, M. Knobel, P. Panissod, A. B. Pakhomov, H. Liu and X. X. Zhang, Phys. Rev. B: Condens. Matter Mater. Phys., 2002, 65, 064422 CrossRef
.
- L. León Félix, J. A. H. Coaquira, M. A. R. Martínez, G. F. Goya, J. Mantilla, M. H. Sousa, L. de los S. Valladares, C. H. W. Barnes and P. C. Morais, Sci. Rep., 2017, 7, 41732 CrossRef
.
- B. Mehdaoui, A. Meffre, L.-M. Lacroix, J. Carrey, S. Lachaize, M. Gougeon, M. Respaud and B. Chaudret, J. Magn. Magn. Mater., 2010, 322, L49–L52 CrossRef CAS
.
- K. Nadeem, H. Krenn, T. Traussnig, R. Würschum, D. V. Szabó and I. Letofsky-Papst, J. Appl. Phys., 2012, 111, 113911 CrossRef
.
- S. Pal, S. Chandra, M.-H. Phan, P. Mukherjee and H. Srikanth, Nanotechnology, 2009, 20, 485604 CrossRef
.
- A. G. Roca, D. Niznansky, J. Poltierova-Vejpravova, B. Bittova, M. A. González-Fernández, C. J. Serna and M. P. Morales, J. Appl. Phys., 2009, 105, 114309 CrossRef
.
- L. Bordonali, Y. Furukawa, M. Kraken, F. J. Litterst, C. Sangregorio, M. F. Casula and A. Lascialfari, Phys. Rev. B: Condens. Matter Mater. Phys., 2012, 85, 174426 CrossRef
.
- Y. Melikhov, J. E. Snyder, D. C. Jiles, A. P. Ring, J. A. Paulsen, C. C. H. Lo and K. W. Dennis, J. Appl. Phys., 2006, 99, 08R102 CrossRef
.
- P. Dutta, S. Pal, M. S. Seehra, N. Shah and G. P. Huffman, J. Appl. Phys., 2009, 105, 07B501 CrossRef
.
- G. Mihajlović, K. Aledealat, P. Xiong, S. von Molnár, M. Field and G. J. Sullivan, Appl. Phys. Lett., 2007, 91, 172518 CrossRef
.
- E. F. Ferrari, F. C. S. da Silva and M. Knobel, Phys. Rev. B: Condens. Matter Mater. Phys., 1997, 56, 6086–6093 CrossRef CAS
.
- F. C. Fonseca, G. F. Goya, R. F. Jardim, R. Muccillo, N. L. V. Carreño, E. Longo and E. R. Leite, Phys. Rev. B: Condens. Matter Mater. Phys., 2002, 66, 104406 CrossRef
.
- J. S. Blázquez, V. Franco, C. F. Conde and A. Conde, J. Nanosci. Nanotechnol., 2007, 7, 1043–1051 CrossRef
.
- W. C. Nunes, W. S. D. Folly, J. P. Sinnecker and M. A. Novak, Phys. Rev. B: Condens. Matter Mater. Phys., 2004, 70, 014419 CrossRef
.
- Q.-L. Ye, H. Yoshikawa and K. Awaga, Materials, 2010, 3, 1244–1268 CrossRef CAS
.
- T. N. Shendruk, R. D. Desautels, B. W. Southern and J. van Lierop, Nanotechnology, 2007, 18, 455704 CrossRef
.
- J. L. Dormann, L. Bessais and D. Fiorani, J. Phys. C: Solid State Phys., 1988, 21, 2015–2034 CrossRef
.
- S. Mohammad Yasin, R. Saha, V. Srinivas, S. Kasiviswanathan and A. K. Nigam, J. Magn. Magn. Mater., 2016, 418, 158–162 CrossRef CAS
.
- G. F. Goya, T. S. Berquó, F. C. Fonseca and M. P. Morales, J. Appl. Phys., 2003, 94, 3520–3528 CrossRef CAS
.
- G. Goya, V. Grazu and M. Ibarra, Curr. Nanosci., 2008, 4, 1–16 CrossRef CAS
.
- R. E. Rosensweig, J. Magn. Magn. Mater., 2002, 252, 370–374 CrossRef CAS
.
- M. Palihawadana-Arachchige, H. Nemala, V. M. Naik and R. Naik, J. Appl. Phys., 2017, 121, 023901 CrossRef
.
- S. Bae, S. W. Lee and Y. Takemura, Appl. Phys. Lett., 2006, 89, 252503 CrossRef
.
- E. Lima, E. De Biasi, R. D. Zysler, M. Vasquez Mansilla, M. L. Mojica-Pisciotti, T. E. Torres, M. P. Calatayud, C. Marquina, M. Ricardo Ibarra and G. F. Goya, J. Nanopart. Res., 2014, 16, 2791 CrossRef
.
- V. M. Khot, A. B. Salunkhe, N. D. Thorat, R. S. Ningthoujam and S. H. Pawar, Dalton Trans., 2013, 42, 1249–1258 RSC
.
- I. Y. Tóth, D. Nesztor, L. Novák, E. Illés, M. Szekeres, T. Szabó and E. Tombácz, J. Magn. Magn. Mater., 2017, 427, 280–288 CrossRef
.
- T. Miyazaki, T. Tange, M. Kawashita and B. Jeyadevan, RSC Adv., 2020, 10, 26374–26380 RSC
.
- M. Avolio, A. Guerrini, F. Brero, C. Innocenti, C. Sangregorio, M. Cobianchi, M. Mariani, F. Orsini, P. Arosio and A. Lascialfari, J. Magn. Magn. Mater., 2019, 471, 504–512 CrossRef CAS
.
- N. Jović Orsini, B. Babić-Stojić, V. Spasojević, M. P. Calatayud, N. Cvjetićanin and G. F. Goya, J. Magn. Magn. Mater., 2018, 449, 286–296 CrossRef
.
- A. F. Alves, S. G. Mendo, L. P. Ferreira, M. H. Mendonça, P. Ferreira, M. Godinho, M. M. Cruz and M. D. Carvalho, J. Nanopart. Res., 2016, 18, 27 CrossRef
.
- D. Nesztor, K. Bali, I. Y. Tóth, M. Szekeres and E. Tombácz, J. Magn. Magn. Mater., 2015, 380, 144–149 CrossRef CAS
.
- R. Ghosh, L. Pradhan, Y. P. Devi, S. S. Meena, R. Tewari, A. Kumar, S. Sharma, N. S. Gajbhiye, R. K. Vatsa, B. N. Pandey and R. S. Ningthoujam, J. Mater. Chem., 2011, 21, 13388 RSC
.
| This journal is © The Royal Society of Chemistry 2020 |