Single-chip based contactless conductivity detection system for multi-channel separations
Audrius
Maruška
 *a,
Tomas
Drevinskas
*a,
Tomas
Drevinskas
 a,
Mantas
Stankevičius
a,
Kristina
Bimbiraitė-Survilienė
a,
Vilma
Kaškonienė
a,
Linas
Jonušauskas
b,
Roaldas
Gadonas
b,
Staffan
Nilsson
ac and
Olga
Kornyšova
a
a,
Mantas
Stankevičius
a,
Kristina
Bimbiraitė-Survilienė
a,
Vilma
Kaškonienė
a,
Linas
Jonušauskas
b,
Roaldas
Gadonas
b,
Staffan
Nilsson
ac and
Olga
Kornyšova
a
aInstrumental Analysis Open Access Centre, Faculty of Natural Sciences, Vytautas Magnus University, Vileikos 8, LT44404 Kaunas, Lithuania. E-mail: audrius.maruska@vdu.lt
bFemtika Ltd, Saulėtekio Ave. 15, Vilnius LT-10224, Lithuania
cPure and Applied Biochemistry, Center for Chemistry and Chemical Engineering, Lund University, 22002 Lund, Sweden
First published on 23rd November 2020
Abstract
In this work, the design and characterization of a multi-cell capacitively coupled contactless conductivity detection system are described. The operation and simultaneous acquisition from 3 detector cells are demonstrated, however, the system is capable of supplying 8 detection cells and can be easily upgraded to maintain 64 capacitively coupled contactless conductivity detection cells. On performing flow-injection analysis, the system recorded as low as 0.01 mM of acetic acid, phosphoric acid, NaH2PO4, and Na2B4O7 solutions in water. The instrument was also capable of recording and distinguishing different mixtures of organic solvents: (a) methanol–acetonitrile, (b) hexane–acetone. The designed detection system is expected to be used coupled with multi-channel separation devices for monitoring simultaneous processes.
Introduction
Following the advancement of novel, high throughput, portable, and in situ analytical systems as well as their demanding applications the need for improved detection systems is observed.1–5The capacitively coupled contactless conductivity detection (C4D) method is widely used in separation science.6,7 The method is mainly used with capillary electrophoresis but also applicable to high-performance liquid chromatography and flow injection analytical techniques.8–10 Independently published by two groups, C4D has undergone various modifications and upgrades.11,12 Regardless of circuitry, excitation frequency, and amplitude optimization as well as miniaturization, C4D has also been attempted to exploit resonant properties that can be used in obtaining optimal excitation and sensing parameters of C4D.13–16 Knowing the resonant zone of the C4D detection cell would help to identify the range of C4D operation properties. Currently, there are multiple versions of this detector including a commercial and open-source version (https://github.com/claudimir-lago/openC4D). Regarding the miniaturization of C4D, a single-chip detector utilizing the capacitance-to-digital conversion technology (AD7745, Analog Devices) has been designed.15,16 However, measurement with a 2-channel containing counterpart – AD7746 – has been applied and demonstrated for ion chromatography and related applications.8,17,18 Regarding typical C4D multiplexing, an array of detectors has been reported.19
Various applications focus on using this detector in situ for investigating the environment, planetary exploration, and life detection that involve harsh environments.20–22 C4D has also found its application in monitoring biotechnological, food, and pharmaceutical processes.23–25
Multiple analytical techniques are used with a single detector; however, multiple detectors including cases where the same detector is used multiple times in a line provide the benefit of additional analytical information. In cases where the technological process provides multiple products that have to be monitored, there is no other way except for using multiple detections.
This work aimed at designing and testing a capacitively coupled contactless conductivity detection system for simultaneous multi-channel monitoring.
Experimental
Chemicals
Acetic acid (99.8%) (Reachem, Slovakia), sodium phosphate (99.0%), sodium tetraborate (99.0%), methanol (99.9%), acetonitrile (99.9%), hexane (97.0%), and acetone (99.5%) were purchased from Sigma-Aldrich (Taufkirchen, Germany).Bidistilled water was produced in the laboratory using a Fistreem Cyclon bidistiller (UK).
Instrumentation
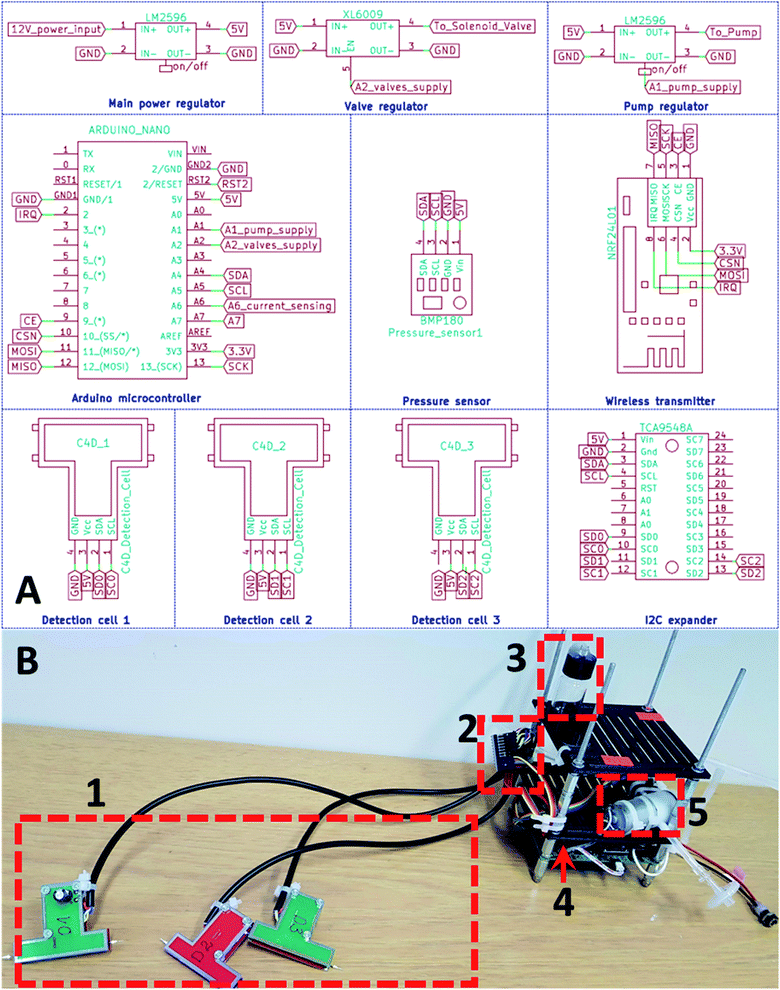
The whole system design was based on a previously developed capillary electrophoresis platform that demonstrated separations on a hovering drone.4 An Arduino Nano (Arduino, Italy) was used as a microcontroller development platform that performed the needed automation and calculations. The Arduino Nano was connected to a TCA9548A (Texas Instruments, USA) inter-integrated circuit (I2C) expansion board. Previously developed single-chip based C4D detection cells were connected to the TCA9548A. Each C4D detection cell contained an AD7745 (Analog Devices, USA) integrated circuit that was responsible for the measurement.26 The detection cell contained 2 pairs of 20 mm length stainless steel tube-electrodes that were prepared using a syringe needle. The inner diameter of the electrode was 0.4 mm and the outer diameter was 0.6 mm. The electrodes were directly soldered onto printed circuit board (PCB) pads that were separated from the ground plane by 0.5 mm. The electrodes had a gap between each other of approximately 0.1 mm. For better shielding against the environment and reduction of parasitic capacitance, a similar-shaped PCB with a ground plane only was placed on top of the C4D cell circuitry. With the factory default settings, the AD7745 integrated circuit is calibrated to measure capacitance in picofarads (pF), so the recorded detector readings were also in pF. The circuit diagram is depicted in Fig. 1A. The whole system received power from a 12 V AC wall adapter. The power input of 12 V was regulated down to 5 V using an LM2596 (Texas Instruments, USA) DC–DC step-down voltage regulator. The Arduino microcontroller received a power of 5 V. For communication between the instrument and computer, an NRF24L01+ (Nordic Semiconductor, Norway) wireless transmitter was used. To regulate the pressure a PID controller was programmed into the Arduino microcontroller: the PID controller calculated for how many milliseconds in 1 second the pump has to be kept on. The pressure was read using a BMP180 pressure sensor (Bosh, Germany). The LM2596 voltage regulator stepped down the voltage from 5 V to 4.5 and supplied power to the pump. An XL6009 stepped up the voltage from 5 V to 12 V and switched on the valve during pressure operation. The actual system is represented in the photograph in Fig. 1B.Experimental setup and measurements
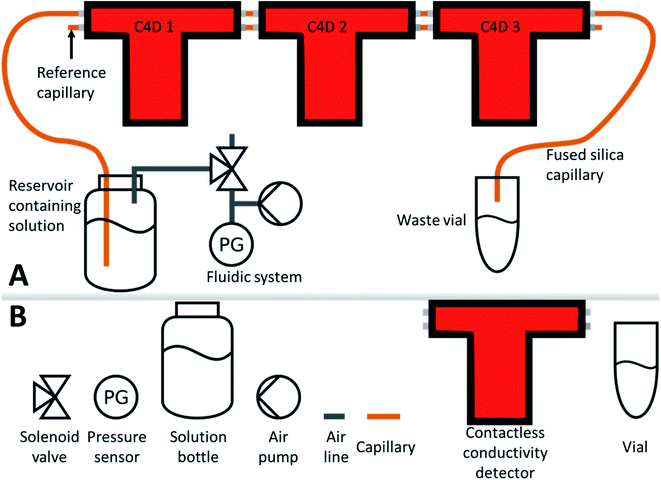
Measurements were performed with three C4D detectors connected in a row for measuring the content of a single capillary (Fig. 2). The whole experimental setup was based on a pneumatic system that was developed for a handheld portable solid-phase microextraction instrument and a capillary electrophoresis platform that was operated on a hovering drone.4,27 The system pressurized the bottle containing measured liquid forcing flow through the capillary. For convenience purposes, 1 m length capillaries were used for the setup. Pressure in the bottle forced the solution to flow through the channel of the capillary passing a detection gap of each C4D detector. Waste was collected into a waste vial. For the experiments, the outer diameter (OD) of the capillaries was 365 μm, and the inner diameter (ID) 50 μm. In the reference position of the electrodes, a similar capillary was used.Stock solutions of 1 M were prepared in bidistilled water before each experiment and kept in a freezer. Before measurement, stock solutions were defrosted and diluted with bidistilled water to obtain the required concentration levels. The flow rate has been determined gravimetrically. The bottle with the solution or solvent was pressurized at 40 kPa (+ambient pressure (∼100 kPa)) and the liquid was forced to flow into a known weight centrifuge vial. After 5 minutes of continuous flow, the vial with the liquid was weighed again, and knowing the viscosity, the volume was calculated and divided by 5 (min).
To measure the solutions of inorganic and organic salts, 10 different levels of concentration in the range of 1 μM to 100 mM were prepared and pumped. Organic solvent solutions were measured separately and in the mixtures with other organic solvents. Organic solvents and their mixtures were prepared in volumetric proportions: (a) 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3, (b) 1
3, (b) 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 2, (c) 1
2, (c) 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1, (d) 2
1, (d) 2![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1, (e) 3
1, (e) 3![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1. Relative permittivities of the mixtures were calculated following the methodology that was applied for the determination of ethanol in the mixtures.28 A slightly modified equation was used (eqn (1)):
1. Relative permittivities of the mixtures were calculated following the methodology that was applied for the determination of ethanol in the mixtures.28 A slightly modified equation was used (eqn (1)):
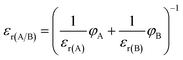 | (1) |
 | (2) |
![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 Hz) and C is the measured capacitance (F).
000 Hz) and C is the measured capacitance (F).
Results
Measurement of inorganic and organic solutions
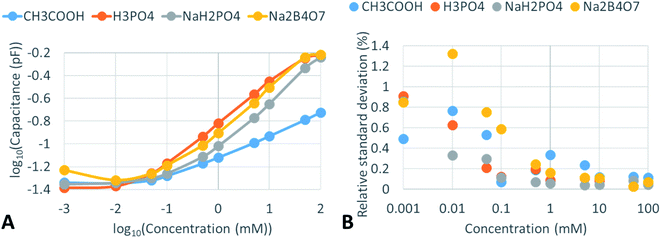
The system was assembled and prepared solutions were pumped through the fused silica capillary and the detection cells recorded the signal. Initially, aqueous solutions of acetic acid and phosphoric acid in the range of 0.001–100.0 mM were prepared and measured (Fig. 3). The flow rate for 0.001 mM acetic acid solution was 13 μL min−1. A non-linear response was recorded that did not fit linear, logarithmic, power, and polynomial fitting, therefore data are represented using a logarithmic scale. On the other hand, in typical applications, the peaks, or separated substances provide a linear calibration profile up to 4 orders of magnitude and in some cases even more.The signal indicated relatively low readings for small concentrations of the acids. For acetic acid in the range of 0.001 mM to 0.05 mM, the readings were between 0.047 and 0.049 pF (106 and 102 MΩ). On increasing the concentration further, a steep increase of the detector reading was observed – in the range of 0.1–1.0 mM the reading of the detector was between 0.054 and 0.077 pF (92 and 65 MΩ). In the range of 5 mM to 100 mM, the signal level was at 0.104–0.191 pF (48 and 26 MΩ).
For phosphoric acid at low concentrations (0.001–0.1 mM) similar observations were recorded, 0.042–0.070 pF (118–71 MΩ). However, on increasing the concentration, a steeper increase in the detector reading was observed. In the range of 0.5 to 100 mM the detector recorded a signal level between 0.118 and 0.612 pF (42 and 8 MΩ).
Inorganic salts of NaH2PO4 and Na2B4O7 showed a similar tendency. At low concentrations, 0.001–0.1 mM, the readings were between 0.051 and 0.061 pF (98 and 82 MΩ). At the highest concentration level (100.0 mM) the reading was similar to that of phosphoric acid (for NaH2PO4 it was 0.574 pF (9 MΩ) and for Na2B4O7 solution, it was 0.605 pF (8 MΩ)). However, intermediate concentrations of inorganic salts indicated higher-level readings than those observed for phosphoric acid. In the range of 0.5 to 50.0 mM the detector readings were between 0.077 and 0.576 pF (65 and 9 MΩ).
The mutual reproducibility of the signal obtained from the individual C4D cells for all investigated solutions and solvents over the range of all tested concentrations was between 5.2 and 0.03%. The large differences between the recorded signals of different C4D cells, especially for highly conductive solutions, can be explained by the fact that electrodes were soldered manually and if precision pick-and-place machinery were used for that purpose it is expected to have much higher reproducibility. On investigating detector reading errors, the relative standard deviation was calculated for 3 readings and expressed as a percentage (Fig. 3B). At low concentrations, 0.001–0.1 mM, the relative standard deviation ranges between 1.4 and 0.3%, except 2 points of acetic acid and NaH2PO4 solutions at 0.1 mM concentration, which is probably a statistical outlier. If measured concentrations are 1 mM and higher it is expected that the RSD of the reading will be up to one order of magnitude lower – 0.4–0.03%.
In typical C4D applications, the signal-to-noise ratio usually decreases at a high concentration in high conductivity solutions. For this reason, narrow-bore capillaries and high excitation frequency, up to 2 MHz are used to preserve the sensitivity of the detection. In this case, a common capillary of 50 μm ID and relatively low excitation frequency (32 kHz) was used indicating low RSD of the measurement. This is probably since the detection cell which is used as the C4D detector is originally calibrated for capacitance measurements.15
Measurement of organic mixtures
In the later attempts, organic mixtures were investigated using the designed system. A combination of 2 miscible organic solvents was selected: (a) methanol–acetonitrile and (b) hexane–acetone. Due to their donor–acceptor and polarity properties, such solvents are used in chromatographic applications as eluents or extractants in the extraction and sample preparation process. In the investigated range of mixture ratio, it was found that mathematical fitting models can be applied to the recorded data (Fig. 4). Interestingly, plotted calculated permittivities of the mixtures show highly linear correlation with the detector reading in the investigated ranges. Linear equations in Fig. 4A and B can be used transforming detector readings into a calculated permittivity, which can later be transformed into a different component ratio (eqn (1)).The mixture ratio and detector reading dependency are not linear. Methanol and acetonitrile mixture can be mathematically described by the power-fitting equation (y = 0.052x0.1201, where y is the capacitance (pF) and x is the mixture ratio). The coefficient of determination for these measurements and fitting was 0.988 suggesting that the model fits well.
Hexane and acetone mixture data can be described mathematically by a natural logarithm fit (y = −5 × 10−4![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) ln(x) + 0.0362) which perfectly fits as the coefficient of determination was 0.999. Methanol–acetonitrile mixture provided readings between 0.044 and 0.051 pF (113 and 98 MΩ) and a mixture of hexane–acetone provided readings between 0.036 and 0.037 pF (138 and 134 MΩ). Significantly lower readings for the hexane–acetone mixture can be explained by the fact that hexane is a nonpolar organic solvent which has a relative permittivity of 2.0 and is incapable of transferring electrical charge. Acetone has a relative permittivity of 20.7, whereas the relative permittivity of methanol is 32.7 and that of acetonitrile is 37.5. Both methanol and acetonitrile have higher relative permittivities than acetone and hexane.
ln(x) + 0.0362) which perfectly fits as the coefficient of determination was 0.999. Methanol–acetonitrile mixture provided readings between 0.044 and 0.051 pF (113 and 98 MΩ) and a mixture of hexane–acetone provided readings between 0.036 and 0.037 pF (138 and 134 MΩ). Significantly lower readings for the hexane–acetone mixture can be explained by the fact that hexane is a nonpolar organic solvent which has a relative permittivity of 2.0 and is incapable of transferring electrical charge. Acetone has a relative permittivity of 20.7, whereas the relative permittivity of methanol is 32.7 and that of acetonitrile is 37.5. Both methanol and acetonitrile have higher relative permittivities than acetone and hexane.
Another important feature is the relative standard deviation of the measurement which indicated low values in the whole investigated range of organic solvent mixtures: 0.15–0.03%. Interestingly, water which has a relative permittivity of 78.4 provided higher RSD of the measurements at low concentrations of dissolved acids and salts than the organic solvent mixtures with low relative permittivities. Interestingly, for the hexane–acetone mixture, the lowest relative standard deviation of the measurement was recorded at 33% (vol) hexane, which is near the hexane–acetone azeotropic point (41% (weight) hexane).
Discussion
In typical C4D measurements, mainly aqueous solutions are investigated. This is mainly due to the very low detector response of organic solvents. However, findings of this work suggest that due to the approximately an order of magnitude lower error of measurement the method has the potential to be used for investigating organic solvents and solutions. This might be relevant in a solvent distillation or purification process especially when it is aimed at obtaining different fractions of the product. In most cases where organic solvents are produced one of the major quality parameters is the presence of inorganic cations and anions. Inorganic cations and anions have relatively high conductivities and can be easily assessed using conductometric techniques. However, as discussed in previous publications, this type of detector is calibrated for the measurement of capacitance.16 Capacitance is a fundamental electrical parameter that does not only depend on the ionic mobility of the solution but also the relative permittivity of the solvent. Different mixtures of different solutions in the capillary affect the capacitance value obtained using the detector cell.Considering the experimental setup, in this work 3 cells were investigated. However, the system allows the connection of up to 8 detection cells and to perform measurements simultaneously. Additionally, it is possible to connect up to 8 TCA9548A I2C expander circuits that allow having 8 detection cells on each of the TCA9548A I2C expander board. This would allow a total number of 64 independent C4D detection cells. Another feature that should be investigated in the future is the application of different frequencies for different measurements. The AD7745 integrated circuit that is used in the design of the detection cell allows reducing the excitation frequency by a factor of 2. Interestingly, detection and determination of ionic species in aqueous solutions require high frequency. In contrast, in this work it is demonstrated that low frequency can help measure organic, non-ionic substances. A similar tendency has been observed in detecting inorganic anions with a C4D detector at <1 kHz using an ion chromatograph where the eluent was an organic solvent mixture with water.9,10
The designed detection system is planned to be used with a simultaneous multi-channel fractionation instrument that is dedicated to separate low and high molecular weight substances in mixture solutions.
Conclusions
A multi-channel single-chip based capacitively coupled contactless conductivity detection system has been developed and demonstrated for measuring different solutions and solvents. Even though 3 detection cells were investigated in this work, up to 8 detection cells can be connected to the existing system for multi-channel measurements and it is possible to expand the system up to 64 detection cells. The detector is calibrated for capacitance measurements and provides data in pF (picofarads). Mixtures of organic solvents indicated recordings between 0.038 and 0.053 pF. Solutions of organic and inorganic acids and inorganic salts in water provided readings between 0.047 and 0.622 pF.Conflicts of interest
Authors declare no conflict of interest.Acknowledgements
The Research Council of Lithuania financed this project, No. S-MIP-19-60.References
- M. F. Mora, F. Kehl, E. Tavares da Costa, N. Bramall and P. A. Willis, Anal. Chem., 2020, 92, 12959–12966 CrossRef CAS PubMed.
- F. Huo, T. wan, Y. Wang, Y. Liu, P. G. Karmaker and X. Yang, J. Chromatogr. A, 2020, 1619, 460935 CrossRef CAS PubMed.
- P. Saar-Reismaa, E. Erme, M. Vaher, M. Kulp, M. Kaljurand and J. Mazina-Šinkar, Anal. Chem., 2018, 90, 6253–6258 CrossRef CAS PubMed.
- T. Drevinskas, A. Maruška, V. Girdauskas, G. Dūda, J. Gorbatsova and M. Kaljurand, Anal. Methods, 2020, 12, 4977–4986 RSC.
- A. Scott, D. Mills, C. Birch, S. Panesar, J. Li, D. Nelson, M. Starteva, A. Khim, B. Root and J. P. Landers, Lab Chip, 2019, 19, 3834–3843 RSC.
- P. Kubáň and P. C. Hauser, Electrophoresis, 2017, 38, 95–114 CrossRef PubMed.
- M. Pumera, Talanta, 2007, 74, 358–364 CrossRef CAS PubMed.
- K. R. Elkin, J. Chromatogr. A, 2014, 1352, 38–45 CrossRef CAS PubMed.
- M. Zhang, B. N. Stamos and P. K. Dasgupta, Anal. Chem., 2014, 86, 11547–11553 CrossRef CAS PubMed.
- B. Yang, M. Zhang, T. Kanyanee, B. N. Stamos and P. K. Dasgupta, Anal. Chem., 2014, 86, 11554–11561 CrossRef CAS PubMed.
- J. A. Fracassi Da Silva and C. L. Do Lago, Anal. Chem., 1998, 70, 4339–4343 CrossRef.
- A. J. Zemann, E. Schnell, D. Volgger and G. K. Bonn, Anal. Chem., 1998, 70, 563–567 CrossRef CAS PubMed.
- J. Tanyanyiwa and P. C. Hauser, Electrophoresis, 2002, 23, 3781–3786 CrossRef CAS.
- Z. Huang, J. Long, W. Xu, H. Ji, B. Wang and H. Li, Flow Meas. Instrum., 2012, 27, 67–70 CrossRef CAS.
- T. Drevinskas, M. Kaljurand and A. Maruška, Electrophoresis, 2014, 35, 2401–2407 CrossRef CAS PubMed.
- T. Drevinskas, A. Maruška and V. Briedis, Electrophoresis, 2015, 36, 292–297 CrossRef CAS PubMed.
- M. Takeuchi, Q. Li, B. Yang, P. K. Dasgupta and V. E. Wilde, Talanta, 2008, 76, 617–620 CrossRef CAS PubMed.
- I. K. Kiplagat, P. Kubáň, P. Pelcová and V. Kubáň, J. Chromatogr. A, 2010, 1217, 5116–5123 CrossRef CAS PubMed.
- M. Stojkovic, I. J. Koenka, W. Thormann and P. C. Hauser, Electrophoresis, 2014, 35, 482–486 CrossRef CAS PubMed.
- M. Kaljurand, Trends Environ. Anal. Chem., 2014, 1, e2–e7 CrossRef CAS.
- M. S. Ferreira Santos, T. G. Cordeiro, A. C. Noell, C. D. Garcia and M. F. Mora, Electrophoresis, 2018, 39, 2890–2897 CrossRef CAS PubMed.
- M. S. Ferreira Santos, A. C. Noell and M. F. Mora, Anal. Methods, 2020, 12, 3205–3209 RSC.
- P. Tůma, K. Málková, E. Samcová and K. Štulík, Anal. Chim. Acta, 2011, 698, 1–5 CrossRef PubMed.
- P. Paul, C. Sänger-van de Griend, E. Adams and A. Van Schepdael, J. Pharm. Biomed. Anal., 2018, 158, 405–415 CrossRef CAS PubMed.
- P. Kubáň and P. C. Hauser, TrAC - Trends Anal. Chem., 2018, 102, 311–321 CrossRef.
- T. Drevinskas, L. Telksnys, A. Maruška, J. Gorbatsova and M. Kaljurand, Electrophoresis, 2018, 39, 2877–2883 CrossRef CAS PubMed.
- K. Bimbiraitė-Survilienė, T. Drevinskas, A. Maruška, O. Kornyšova, J. Gorbatsova, H. Ihara and M. Kaljurand, Microchem. J., 2020, 159, 105576 CrossRef.
- P. Tůma and F. Opekar, Electrophoresis, 2015, 36, 1976–1981 CrossRef PubMed.
| This journal is © The Royal Society of Chemistry 2021 |