Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Large optical anisotropy-oriented construction of a carbonate-nitrate chloride compound as a potential ultraviolet birefringent material†
Meng
Cheng
ab,
Wenqi
Jin
ab,
Zhihua
Yang
 ab and
Shilie
Pan
ab and
Shilie
Pan
 *ab
*ab
aResearch Center for Crystal Materials, CAS Key Laboratory of Functional Materials and Devices for Special Environments, Xinjiang Technical Institute of Physics & Chemistry, CAS, 40-1 South Beijing Road, Urumqi 830011, China. E-mail: slpan@ms.xjb.ac.cn
bCenter of Materials Science and Optoelectronics Engineering, University of Chinese Academy of Sciences, Beijing 100049, China
First published on 9th November 2022
Abstract
The design of new birefringent materials is very significant owing to their indispensable role in modulating the polarization of light and is vital in laser technology. Herein, by applying a large optical anisotropy-oriented construction induced by a synergy effect of multiple anionic groups, a promising carbonate-nitrate chloride, Na3Rb6(CO3)3(NO3)2Cl·(H2O)6, has been designed and synthesized successfully by the solvent evaporation method and single crystals of centimeter size were obtained by the recrystallization method in aqueous solution. It crystallizes in the hexagonal P63/mcm space group, the RbO9Cl polyhedra and the NaO7 polyhedra construct a three-dimensional (3D) framework by sharing O or Cl atoms and trigonal plane units (CO3 and NO3). The transmittance spectrum based on a 1 mm thick single-crystal plate shows that its short UV cut-off edge is about 231 nm. And the refractive index differences (0.14 @ 546 nm) measured by using a polarizing microscope on the (101) crystal plane, proves that Na3Rb6(CO3)3(NO3)2Cl·(H2O)6 has a large birefringence, which has potential application in the solar blind ultraviolet region. The theoretical calculations reveal that the π-conjugated CO3 and NO3 groups are the main cause of the birefringence. It demonstrates that combining π-conjugated CO3 and NO3 groups in one structure is an extremely effective strategy to explore new UV birefringent crystals.
Introduction
Exploring new birefringent materials is a current academic and technological hotspot due to their applications in polarimetry, optical communication, and scientific instrumentation. Hitherto, several birefringent crystals have been commercialized, such as MgF2,1 α-BaB2O4,2 CaCO3,3 and so on, but some drawbacks hinder their practical application in the ultraviolet (UV) including the solar blind ultraviolet region (200–280 nm) or the deep-ultraviolet (DUV, ≤200 nm) region. For example, although a natural calcite crystal (CaCO3) with large birefringence (0.17 @ 633 nm) is the most widely used birefringent material for a prism polarizer in the UV to the near–infrared spectral range, its poor optical homogeneity and cleavability limit its application. Thus, designing new UV birefringent crystals is important for scientific and technological research.Birefringence is the key parameter for birefringent crystals with non-cubic crystal structures, which is generally quantified as the maximum difference between refractive indices exhibited by the material.4 Generally, the metal cations and the types of anionic groups play an important role in the magnitude of birefringence. A metal cation centered polyhedron (Pb, Sn, Bi, etc.)5–12 with a stereochemically active lone pair is beneficial to the generation of high-polarizability anisotropy, which would enhance the optical anisotropy and birefringence of crystals, but they are often impracticable in designing new UV/DUV birefringent crystals since the cut-off edge may be redshifted with these cations. Alkali metal or alkaline-earth metal cations are usually chosen to ensure wide transparency in the UV region, but they generally contribute less to the polarizability anisotropy.13–22 Thus, the contribution of anionic groups that are active for birefringence would be crucial in exploring new UV birefringent crystals. Non-π-conjugated anionic groups, i.e., PO4 or BO4, exhibit a large bandgap, while their relatively small polarizability anisotropy imparts a small birefringence. And it is found that the introduction of fluorine in the tetrahedron can enhance the birefringence or optimize the dispersion of refractive indices, TO4−xFx (T = B, x = 1–4; T = P, x = 1–2; T = S, and x = 1).23–35 Planar π-conjugated anionic groups, such as BO2, BO3, B3O6, C3N3O3, CO3, NO3, etc.,35–44 exhibit strong polarizability anisotropy, which are regarded as the preferred functional units to induce large birefringence. Borates with relatively wide transparency and large birefringence have been studied systematically in recent decades. Among borate birefringent materials, the commercial birefringent material of α-BaB2O4 shows relatively large birefringence (Δn = 0.12 @ 546 nm)45 due to large polarizability anisotropy of B3O6 anionic groups. Simultaneously, as birefringent crystals, carbonates have emerged in recent years, but nitrates have rarely been reported because they always show water solubility and deliquescence. However, in comparison with BO3 and CO3 anionic groups, NO3 has higher microscopic polarizability anisotropy (Table S5†), and it is easier to obtain large size single crystals, which are useful to further study other properties.
Based on these aspects, our strategy is to introduce multi anionic groups such as CO3 and NO3 π-conjugated units in one structure to improve the crystal growth of carbonate while maintaining the optical performance of birefringent crystals. Based on the Inorganic Crystal Structure Database (ICSD), there are six examples where CO3 and NO3 π-conjugated anionic groups coexist in one structure. Except for the [Pb6O4](OH)(NO3)(CO3)46 compound, other compounds are transition metal (Pt or Co) amine complexes ((Pt(NH3)2)4(CO3)2)(NO3)4·(H2O)3,47 (Co(NH3)4(CO3)(NO3))2·H2O,48 (Co(CO3)(NH3)4)(NO3),49 (Co(NH3)4CO3)(NO3)·(H2O)0.5![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 50 and (Co(NH3)5(CO3))(NO3)·H2O.51 In this work, we combine two types of π-conjugated anionic groups and a halogen anion in one compound. And Na3Rb6(CO3)3(NO3)2Cl·(H2O)6, the first carbonate-nitrate chloride has been successfully synthesized by the solvent evaporation method. And the crystal growth was carried out by the recrystallization method, and the single crystals of centimeter size were obtained. Herein, the details of synthesis and crystal growth, the IR spectrum, thermal behavior, the UV-vis-NIR diffuse-reflectance spectrum, the transmittance spectrum and the refractive index difference of Na3Rb6(CO3)3(NO3)2Cl·(H2O)6 are reported. Simultaneously, we analyzed the influence mechanism of multiple anionic units by first-principles calculations. The real space atom-cutting method and electron density difference map were employed to analyze the origin of the large birefringence. The experimental and theoretical results show that Na3Rb6(CO3)3(NO3)2Cl·(H2O)6 is a potential UV birefringent crystal with a large birefringence and short UV cut-off edge.
50 and (Co(NH3)5(CO3))(NO3)·H2O.51 In this work, we combine two types of π-conjugated anionic groups and a halogen anion in one compound. And Na3Rb6(CO3)3(NO3)2Cl·(H2O)6, the first carbonate-nitrate chloride has been successfully synthesized by the solvent evaporation method. And the crystal growth was carried out by the recrystallization method, and the single crystals of centimeter size were obtained. Herein, the details of synthesis and crystal growth, the IR spectrum, thermal behavior, the UV-vis-NIR diffuse-reflectance spectrum, the transmittance spectrum and the refractive index difference of Na3Rb6(CO3)3(NO3)2Cl·(H2O)6 are reported. Simultaneously, we analyzed the influence mechanism of multiple anionic units by first-principles calculations. The real space atom-cutting method and electron density difference map were employed to analyze the origin of the large birefringence. The experimental and theoretical results show that Na3Rb6(CO3)3(NO3)2Cl·(H2O)6 is a potential UV birefringent crystal with a large birefringence and short UV cut-off edge.
Experimental
Synthesis of the single crystal
Na3Rb6(CO3)3(NO3)2Cl·(H2O)6 was synthesized by the solvent evaporation method through reacting NaHCO3 (10 mmol, 0.8401 g), RbNO3 (10 mmol, 1.4747 g), RbCl (10 mmol, 1.2092 g) and deionized water. The mixture was stirred at room temperature and the solution turned colorless and transparent, and then the solution was evaporated at room temperature. Finally, transparent and block crystals grew out in 3 days. As shown in Fig. S1,† single crystals of centimeter size were obtained by the recrystallization method in aqueous solution in 14 days.Characterization
A coreless single crystal with suitable size of the title compound was selected for single crystal X-ray diffraction. All diffraction data were collected on a Bruker D8 Venture with Mo Kα (λ = 0.71073 Å) at 298(2) K. The intensity, reduction, and cell refinement investigations were carried out on a Bruker SAINT.52All the structures were solved by a direct method and refined through the full-matrix least-squares fitting on F2 with SHELX53 and OLEX254 software. PLATON55 was used to confirm the higher symmetry. Crystallographic data and further details for structural analyses are listed in Table 1, and the selected bond distances are summarized in Table S2.† Powder X-ray diffraction (PXRD) data were collected by putting the powder sample onto flat sample holders utilizing a Bruker D2 Phaser X-ray diffractometer equipped with Cu-Kα radiation (λ = 1.54056 Å) and the diffraction patterns were taken in the range from 10 to 70° (2 theta). The powder XRD patterns of pure polycrystalline samples exhibit good consistency with the calculated XRD ones. The calculated XRD patterns were produced by the Mercury v3.8 program and their single-crystal structure data. Polycrystalline samples used for thermogravimetric (TG) analysis and differential scanning calorimetry (DSC) were ground from bulk crystals directly. Infrared spectroscopy was carried out on a Shimadzu IR Affinity-1 Fourier transform IR spectrometer with a resolution of 2 cm−1 in the 500 to 4000 cm−1 range. The polycrystalline samples were mixed thoroughly with dried KBr with a mass ratio of about 1 (polycrystalline sample)![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 100 (KBr). A Shimadzu Solid Spec-3700 DUV spectrophotometer was used to collect the UV-vis-NIR diffuse-reflectance data for the title compounds. The spectrophotometer worked ranging from 175 to 2600 nm at room temperature. The transmittance spectrum was measured using a transparent crystal plate with 1 mm thickness in the 175–1600 nm wavelength range. Absorption data (K/S) were worked out from the following Kubelka–Munk56 function:
100 (KBr). A Shimadzu Solid Spec-3700 DUV spectrophotometer was used to collect the UV-vis-NIR diffuse-reflectance data for the title compounds. The spectrophotometer worked ranging from 175 to 2600 nm at room temperature. The transmittance spectrum was measured using a transparent crystal plate with 1 mm thickness in the 175–1600 nm wavelength range. Absorption data (K/S) were worked out from the following Kubelka–Munk56 function:
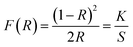 | (1) |
| R = |Ng − Np| × d = Δn × d | (2) |
| Empirical formula | Na3Rb6(CO3)3(NO3)2Cl·(H2O)6 |
|---|---|
| a R 1 = ∑‖Fo| − |Fc‖/∑|Fo| and wR2 = [∑w(Fo2 − Fc2)2/∑wFo4]1/2 for Fo2 > 2σ(Fo2). | |
| Formula weight | 1029.39 |
| Temperature | 298 (2) K |
| Wavelength | 0.71073 Å |
| Crystal system | Hexagonal |
| Space group | P63/mcm |
| Unit cell dimensions | a = 9.5732(3) Å |
| c = 15.8202(11) Å | |
| Volume | 1255.62(10) Å3 |
| Z | 2 |
| Density (calculated) | 2.723 Mg m−3 |
| Absorption coefficient | 11.849 mm−1 |
| F(000) | 968 |
| Crystal size | 0.17 × 0.16 × 0.11 mm3 |
| Theta range for data collection | 2.457 to 27.541° |
| Index ranges | −10 ≤ h ≤ 12, −12 ≤ k ≤ 12, −20 ≤ l ≤ 20 |
| Reflections collected | 10![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 235 235 |
| Independent reflections | 557 [R(int) = 0.0775] |
| Completeness to theta = 27.541° | 100.0% |
| Refinement method | Full-matrix least-squares on F2 |
| Data/restraints/parameters | 557/0/44 |
| Goodness-of-fit on F2 | 1.074 |
| Final R indices [I > 2sigma(I)]a | R 1 = 0.0238, wR2 = 0.0457 |
| R indices (all data)a | R 1 = 0.0393, wR2 = 0.0511 |
| Largest diff. peak and hole | 0.436 and −0.484 e Å−3 |
Theoretical calculations
The electronic structure was calculated using density functional theory (DFT) performed using the plane wave pseudopotential implemented in the CASTEP package.57 Using the norm-conserving pseudopotential (NCP),58 the explicitly treated valence electrons for each atom were calculated as follows: Na, 3s1; Rb, 5s1; C, 2s22p2; N, 2s22p3; H, 1s1, O, 2s22p4, and Cl, 3s23p5. The exchange–correlation functional was the Perdew–Burke–Ernzerhof (PBE) functional within the generalized gradient approximation (GGA).59,60 The TS method was used for the DFT-D correction.61 The plane-wave energy cutoff was set at 750.0 eV. The separation of the k-point was set as 0.03 Å in the Brillouin zone. The number of empty bands were set as 3 times of valence bands for the calculation of the optical properties. Because the GGA method always underestimates the bandgap, the scissors operators were utilized to shift the conduction bands so that they agree with the experimental bandgap values, and then the refractive indices were obtained by the real part of the dielectric function on the base of the Kramers–Kronig transform. The HOMO–LUMO energy gap and polarizability anisotropy (δ) of anionic groups were calculated using DFT implemented by the Gaussian09 package.62 The B3LYP (Becke, three-parameter, Lee–Yang–Parr) exchange–correlation functional with the Lee–Yang–Parr correlation functional at the 6-31G basis set in Gaussian was employed.Results and discussion
Crystal structure
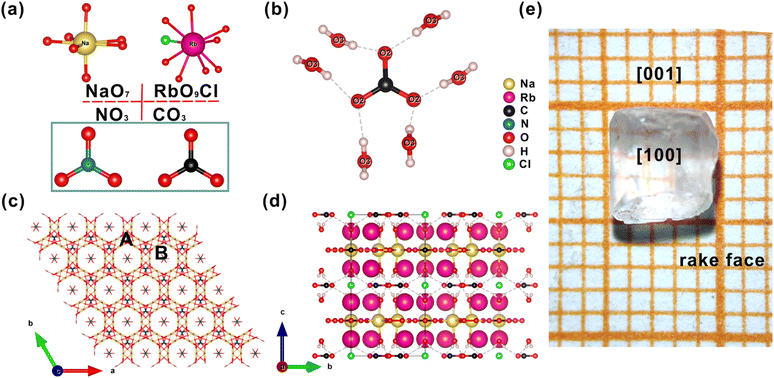
The crystallographic analysis reveals that Na3Rb6(CO3)3(NO3)2Cl·(H2O)6 (CCDC 2181425) crystallizes in the space group P63/mcm (no. 193) of the hexagonal crystal system. There is one independent Na (6g), one independent Rb (12k), two independent C (4d and 2a), one independent N (4c), four independent O (12i, 12j, 12k, and 6g), one independent Cl (2b) and one independent H (24l) wyckoff sites (Table S1†) in the asymmetric unit. In the structure of the title compound, there are two different π-conjugated anionic groups of CO3 and NO3. In the structure, the Na–O bond lengths vary from 2.268(4) to 2.715(4) Å. The two C atoms are three-coordinated in a π-conjugated planar triangle with the C–O lengths in the range of 1.277(4)–1.280(3) Å. The N atoms are three-coordinated in a π-conjugated planar triangle with the N–O bond lengths of 1.250(3) Å. The Rb–O bond lengths vary from 3.030(2) to 3.401(3) Å and the Rb–Cl bond length is 3.2282(4) Å. The O–C–O and O–N–O bond angles are of the 3-fold symmetrical 120° angle (Table S2†). The water molecule plays the role of a hydrogen bond donor, and the O(2) atom of carbonate ions plays the role of a hydrogen bond acceptor. The distance between O(2) and O(4) atoms is 2.702(5) Å and the angle of O(3)–H⋯O(2) is 176(3) °. Each carbonate ion connects with six water molecules by hydrogen bond interaction. Furthermore, bond valence sum (BVS) calculations give values of 1.22, 1.10, 4.91, 4.08, 4.04, 2.09, 1.60, 0.45, 2.08, and 1.26 for Na(1), Rb(1), N(1), C(1), C(2), O(1), O(2), O(3), O(4), and Cl(1), which are consistent with oxidation states of 1, 1, 5, 4, 2, and 1 for Na, Rb, N, C, non-hydroxyl O, and Cl, respectively. The calculated BVS for O(2) is 1.60, since the hydrogen bond interaction can be found among O(2), O(3) and H atoms, i.e., O(3)–H⋯O(2). The calculated BVS for O(3) is 0.45, suggesting that this is for H2O groups, which was also confirmed by the IR data.For the alkali metal atoms, as indicated in Fig. 1a, the Na atoms are coordinated by seven O atoms to form a NaO7 pentagonal bipyramid and the Rb atoms are coordinated by nine O atoms and one Cl atom to form an RO9Cl polyhedron. The Rb-centered polyhedron and the NaO7 polyhedron construct a three-dimensional (3D) framework by sharing O or Cl atoms with each other. As shown in Fig. 1c, there are two types (9-membered ring channel (type A) and 6-membered ring channel (type B)) of channels in the 3D framework. In the framework, C(2)O3 and N(1)O3 trigonal plane units reside in 9-membered channels and Cl atoms and C(1)O3 trigonal plane units reside in 6-membered channels. The NaO7 pentagonal bipyramid and C(1)O3 and N(1) O3 trigonal plane units form a 2D layer and stack along the [001] direction. Simultaneously, owing to the directivity and saturation of hydrogen bonds, C(2)O3 trigonal plane units and water molecules are connected by hydrogen bonding forces to further stabilize the structure. For clarity, the Rb–O/Cl bonds are deleted, Fig. 1d shows the total structure of title compounds in the bc plane. As shown in Fig. 1e, the photograph of an as-grown Na3Rb6(CO3)3(NO3)2Cl·(H2O)6 crystal is consistent with the theoretical crystal morphology (Fig. S6†), and the crystal orientation is determined by using an X-ray crystal orientation instrument.
Optical properties and birefringence
In order to further confirm the coordination environments of anionic groups in the structure, IR spectroscopic measurements were carried out (see Fig. S2b†). The characteristic vibration frequency of the O–H bond is 3500–3730 cm−1. Affected by hydrogen bonds (O–H⋯Y), H is pulled over by Y, and the characteristic vibration frequency will be reduced to 3100–3500 cm−1. Therefore, the broad IR absorption bands at ∼3160 cm−1 confirm the presence of water molecules and hydrogen bonds (O–H⋯O) in the structure. The broad and strong absorption bands in the range of 1451–1367 cm−1 and 883–660 cm−1 can be assigned to the characteristic absorption of the NO3 or CO3 groups. These results are in accordance with those of previously reported studies. The UV-vis-NIR diffuse reflectance spectrum of Na3Rb6(CO3)3(NO3)2Cl·(H2O)6 is shown in Fig. S2a.† Its UV cut-off absorption edge is at about 220 nm, which demonstrates that the title compound is a UV optical material. To further confirm the UV cut-off edge, the crystal was grown for the UV-vis-NIR transmittance spectrum measurement, and the result based on a 1 mm thick preliminary polishing single-crystal plate shows that it has a UV cut-off edge of 231 nm.It is well-known that the planar triangular groups of CO3 and NO3 have large polarizability anisotropy. Therefore, the crystal may show a large birefringence when the CO3 and NO3 groups are stacked parallelly to form a perfect layered structure. The calculated linear optical results of Na3Rb6(CO3)3(NO3)2Cl·(H2O)6 show that nx = ny > nz, that is, no > ne, indicating that the title compound is a negative uniaxial optical crystal. The interference pattern of polarized light indicates that Na3Rb6(CO3)3(NO3)2Cl·(H2O)6 is a uniaxial crystal (Fig. 2a). According to the calculated refractive index dispersion curves, its birefringence is 0.12 at 546 nm (Fig. 2d), which is comparable to that of α-BBO. The refractive index difference was measured under the polarizing microscope method, using (101) crystal planes. The crystal thickness of the title compound is 25.655 μm. And the optical path differences at 546 nm are 12.75 and 12.95 μm. According to the formula, the refractive index differences on the (101) crystal planes can be calculated to be 0.14 @ 546 nm, which indicates that Na3Rb6(CO3)3(NO3)2Cl·(H2O)6 may have a birefringence larger than 0.14 @ 546 nm.
Electronic structure and mechanism of birefringence
In order to get more insight into the interaction between the microstructure and optical properties for Na3Rb6(CO3)3(NO3)2Cl·(H2O)6, systematic theoretical calculations on the basis of density functional theory (DFT) were used. The partial densities of states (PDOS) reflect the distribution of atomic orbitals. The valence bands (VBs) are mainly composed of H-s, C-p, N-p, O-p, Cl-p and Rb-p, and the conductive bands (CBs) mainly originate from H-s, C-p, N-p, O-p, Na-sp and Rb-sp. The top of the VB, quite close to the Fermi level, is occupied by O-p and H-s; with further analysis of each crystallographically independent O atom together with a H atom, a clearer hydrogen bond interaction can be found among O(2), O(3) and H atoms, i.e., O(3)-H⋯O(2) (Fig. 3b), which is similar to the hydrogen bond in paracetamol.63 At the bottom of the CB, several separate electronic states of N-2p, O-2p (specifically, O(4)-2p, Fig. 3b) and Na-p appear, among which the interaction between N-2p and O(4)-2p means a NO3 group. These separate electronic structures at the bottom of the CB were also reported in other nitrates.64,65 Therefore, the bandgap is mainly determined by the hydrogen bond, Na-p, N-p and O-p.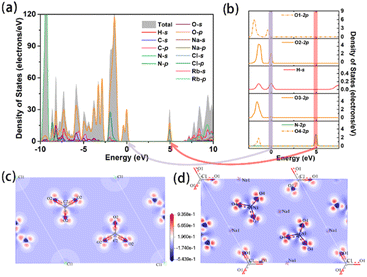 | ||
| Fig. 3 (a) Total density of states; (b) partial density of states of O and H atoms; (c and d) electron density difference of CO3 and NO3 groups. | ||
In order to identify the origin of the large birefringence, the real space atom-cutting (RSAC)66 method and electron density difference map67 are employed. From the calculated electron density difference map of CO3 and NO3 (Fig. 3c and d), we can see that there are obvious covalent characteristics between the C–O or N–O bonds. As shown in Table S6,† the main contribution comes from the CO3 and NO3 anionic units. To evaluate the contribution of hydrogen bonds to birefringence, H2O was cut off from the structure, and the calculated birefringence is also unchanged (Table S6†). In this case, the calculated birefringence is 0.12 @ 546 nm. Thus, we speculate that hydrogen bonds do not contribute significantly to the birefringence of the title compound. Based on the calculated results which are listed in Table S6,† the planar π-conjugated CO3 and NO3 anionic units play a major role in the large birefringence.
Conclusions
In conclusion, the first carbonate-nitrate chloride, Na3Rb6(CO3)3(NO3)2Cl·(H2O)6, with two types of π-conjugated anionic groups has been characterized as a new UV birefringent crystal with large birefringence and a short UV cut-off edge. For Na3Rb6(CO3)3(NO3)2Cl·(H2O)6, the RbO9Cl and NaO7 polyhedra construct a three-dimensional framework by sharing O or Cl atoms, and the planar π-conjugated groups (CO3 and NO3) reside in a 9-membered ring channel and 6-membered ring channel, respectively in the 3D framework. And the hydrogen bond interaction between CO3 and H2O will further stabilize the structure. We have obtained single crystals of centimeter size by a simple and environmentally friendly aqueous solution method. Simultaneously, we analyze the influence mechanism of multiple anionic units by first-principles calculations. The result shows that the planar π-conjugated CO3 and NO3 anionic units play a major role in the large birefringence. The experimental refractive index difference is 0.14 @ 546 nm on the (101) crystal plane, which is comparable to that of the α-BBO (0.12 @ 546 nm) crystal. The synthesis and investigation of other new alkaline-metal carbonate-nitrate compounds with excellent properties are still in progress.Data availability
All of the related experimental and computational data are provided in the ESI.†Author contributions
M. C. and S. L. P. designed the research study; M. C. synthesized the compound and performed the experiments. W. Q. J. and Z. H. Y. performed the optical theoretical calculations. All authors wrote and revised the manuscript. All the authors contributed to the final manuscript preparation.Conflicts of interest
The authors declare no competing financial interests.Acknowledgements
This work was supported by the National Natural Science Foundation of China (61835014, 51972336, and 22193044), the Xinjiang Major Science and Technology Project (2021A01001), the International Partnership Program of the CAS (1A1365KYSB20200008), the West Light Foundation of the CAS (2019-YDYLTD-002), the National Key Research and Development Program of China (2021YFB3601501 and 2021YFB3601502), the CAS Project for Young Scientists in Basic Research (YSBR-024), the Instrument Developing Project of the CAS (GJJSTD20200007) and the Science and Technology Service Network Initiative of the CAS (KFJ-STS-QYZD-130).Notes and references
- F. Sedlmeir, R. Zeltner, G. Leuchs and H. G. L. Schwefel, Opt. Express, 2014, 22, 30934–30942 CrossRef CAS PubMed.
- G. Q. Zhou, J. Xu, X. D. Chen, H. Y. Zhong, S. T. Wang, K. Xu, P. Z. Deng and F. X. Gan, J. Cryst. Growth, 1998, 191, 517–519 CrossRef CAS.
- G. Ghosh, Opt. Commun., 1999, 163, 95–102 CrossRef CAS.
- S. Niu, G. Joe, H. Zhao, Y. Zhou, T. Orvis, H. Huyan, J. Salman, K. Mahalingam, B. Urwin, J. Wu, Y. Liu, T. E. Tiwald, S. B. Cronin, B. M. Howe, M. Mecklenburg, R. Haiges, D. J. Singh, H. Wang, M. A. Kats and J. Ravichandran, Nat. Photonics, 2018, 12, 392–396 CrossRef CAS.
- R. L. Tang, C. L. Hu, F. F. Mao, J. H. Feng and J. G. Mao, Chem. Sci., 2019, 10, 837–842 RSC.
- J. Guo, A. Tudi, S. Han, Z. Yang and S. Pan, Angew. Chem., Int. Ed., 2019, 58, 17675–17678 CrossRef CAS PubMed.
- Q. Jing, Z. Yang, S. Pan and D. Xue, Phys. Chem. Chem. Phys., 2015, 17, 21968–21973 RSC.
- J. Guo, A. Tudi, S. Han, Z. Yang and S. Pan, Angew. Chem., Int. Ed., 2021, 60, 24901–24904 CrossRef CAS PubMed.
- S. Han, M. Mutailipu, A. Tudi, Z. Yang and S. Pan, Chem. Mater., 2020, 32, 2172–2179 CrossRef CAS.
- X. Dong, Q. Jing, Y. Shi, Z. Yang, S. Pan, K. R. Poeppelmeier, J. Young and J. M. Rondinelli, J. Am. Chem. Soc., 2015, 137, 9417–9422 CrossRef CAS PubMed.
- K. Kim, M. H. Lee and K. M. Ok, Chem. Mater., 2021, 33, 6564–6571 CrossRef CAS.
- G. Zou and K. M. Ok, Chem. Sci., 2020, 11, 5404–5409 RSC.
- X. L. Chen, B. B. Zhang, F. F. Zhang, Y. Wang, M. Zhang, Z. Yang, K. R. Poeppelmeier and S. L. Pan, J. Am. Chem. Soc., 2018, 140, 16311–16319 CrossRef CAS PubMed.
- Z. Bai, L. Liu, D. Wang, C. L. Hu and Z. Lin, Chem. Sci., 2021, 12, 4014–4020 RSC.
- M. Mutailipu, M. Zhang, Z. H. Yang and S. L. Pan, Acc. Chem. Res., 2019, 52, 791–801 CrossRef CAS PubMed.
- M. Mutailipu and S. Pan, Angew. Chem., Int. Ed., 2020, 59, 20302–20317 CrossRef CAS PubMed.
- W. Zhang, Z. Zhang, W. Jin, R. Zhang, M. Cheng, Z. Yang and S. Pan, Sci. China. Chem., 2021, 64, 1498–1503 CrossRef CAS.
- Z. Li, Z. Lin, Y. Wu, P. Fu, Z. Wang and C. Chen, Chem. Mater., 2004, 16, 2906–2908 CrossRef CAS.
- G. Han, B. Lei, Z. Yang, Y. Wang and S. Pan, Angew. Chem., Int. Ed., 2018, 57, 9828–9832 CrossRef CAS PubMed.
- S. Li, X. Liu, H. Wu, Z. Song, H. Yu, Z. Lin, Z. Hu, J. Wang and Y. Wu, Chem. Sci., 2021, 12, 13897–13901 RSC.
- Z. Li, W. Jin, F. Zhang, Z. Chen, Z. Yang and S. Pan, Angew. Chem., Int. Ed., 2022, 61, e202112844 CAS.
- B. Zhang, G. Shi, Z. Yang, F. Zhang and S. Pan, Angew. Chem., Int. Ed., 2017, 56, 3916–3919 CrossRef CAS PubMed.
- H. Cheng, F. Li, Z. Yang and S. Pan, Angew. Chem., Int. Ed., 2022, 61, e202115669 CAS.
- M. Mutailipu, M. Zhang, H. Wu, Z. Yang, Y. Shen, J. Sun and S. Pan, Nat. Commun., 2018, 9, 3089 CrossRef PubMed.
- B. Zhang, G. Han, Y. Wang, X. Chen, Z. Yang and S. Pan, Chem. Mater., 2018, 30, 5397–5403 CrossRef CAS.
- J. Lu, J. N. Yue, L. Xiong, W. K. Zhang, L. Chen and L. M. Wu, J. Am. Chem. Soc., 2019, 141, 8093–8097 CrossRef CAS PubMed.
- W. Jin, W. Zhang, A. Tudi, L. Wang, X. Zhou, Z. Yang and S. Pan, Adv. Sci., 2021, 8, 2003594 CrossRef CAS PubMed.
- Y. Wang, B. Zhang, Z. Yang and S. Pan, Angew. Chem., Int. Ed., 2018, 57, 2150–2154 CrossRef CAS PubMed.
- H. Wu, H. Yu, S. Pan, Z. Huang, Z. Yang, X. Su and K. R. Poeppelmeier, Angew. Chem., Int. Ed., 2013, 52, 3406–3410 CrossRef CAS PubMed.
- X. Wang, Y. Wang, B. Zhang, F. Zhang, Z. Yang and S. Pan, Angew. Chem., Int. Ed., 2017, 56, 14119–14123 CrossRef CAS PubMed.
- G. Shi, Y. Wang, F. Zhang, B. Zhang, Z. Yang, X. Hou, S. Pan and K. R. Poeppelmeier, J. Am. Chem. Soc., 2017, 139, 10645–10648 CrossRef CAS PubMed.
- M. Mutailipu, M. Zhang, B. Zhang, L. Wang, Z. Yang, X. Zhou and S. Pan, Angew. Chem., Int. Ed., 2018, 57, 6095–6099 CrossRef CAS PubMed.
- Z. Z. Zhang, Y. Wang, B. B. Zhang, Z. H. Yang and S. L. Pan, Angew. Chem., Int. Ed., 2018, 57, 6577–6581 CrossRef CAS PubMed.
- Z. Yang, B. Lei, W. Zhang and S. Pan, Chem. Mater., 2019, 31, 2807–2813 CrossRef CAS.
- J. Zhao, Y. Ma and R. Li, Appl. Opt., 2015, 54, 9949–9953 CrossRef CAS PubMed.
- L. Cao, H. Tian, D. Lin, C. Lin, F. Xu, Y. Han, T. Yan, J. Chen, B. Li, N. Ye and M. Luo, Chem. Sci., 2022, 13, 6990–6997 RSC.
- C. Huang, M. Mutailipu, F. Zhang, K. J. Griffith, C. Hu, Z. Yang, J. M. Griffin, K. R. Poeppelmeier and S. Pan, Nat. Commun., 2021, 12, 2597 CrossRef CAS PubMed.
- K. Kang, F. Liang, X. Meng, J. Tang, T. Zeng, W. Yin, M. Xia, Z. Lin and B. Kang, Inorg. Chem., 2019, 58, 9553–9556 CrossRef CAS PubMed.
- Z. Chen, H. Zeng, S. Han, Z. Yang and S. Pan, New J. Chem., 2021, 45, 17544–17550 RSC.
- X. Liu, L. Kang, P. Gong and Z. Lin, Angew. Chem., Int. Ed., 2021, 60, 13574–13578 CrossRef CAS PubMed.
- X. Zhang, L. Guo, B. Zhang, J. Yu, Y. Wang, K. Wu, H. j. Wang and M. H. Lee, Chem. Commun., 2021, 57, 639–642 RSC.
- B. Cheng, Z. Li, Y. Chu, A. Tudi, M. Mutailipu, F. Zhang, Z. Yang and S. Pan, Natl. Sci. Rev., 2022, 9, nwac110 CrossRef PubMed.
- S. Guo, X. Jiang, L. Liu, M. Xia, Z. Fang, X. Wang, Z. Lin and C. Chen, Chem. Mater., 2016, 28, 8871–8875 CrossRef CAS.
- H. Liu, Y. Wang, B. Zhang, Z. Yang and S. Pan, Chem. Sci., 2020, 11, 694–698 RSC.
- V. P. Solntsev, E. G. Tsvetkov, V. A. Gets and V. D. Antsygin, J. Cryst. Growth, 2002, 236, 290–296 CrossRef CAS.
- Y. P. Li, S. V. Krivovichev and P. C. Burns, J. Solid State Chem., 2000, 153, 365–370 CrossRef CAS.
- H. K. Mahtani, S. C. Chang, J. R. Ruble, I. N. L. Black and P. B. Stein, Inorg. Chem., 1993, 32, 4976–4978 CrossRef CAS.
- I. Bernal and J. Cetrullo, Struct. Chem., 1990, 1, 227–234 CrossRef CAS.
- A. Le Bail, Acta Crystallogr., Sect. E: Struct. Rep. Online, 2013, 69, i42–i43 CrossRef CAS PubMed.
- A. N. Christensen and R. G. Hazell, Acta Chem. Scand., 1999, 53, 399–402 CrossRef CAS.
- J. H. Zhu, H. X. Wu and A. Le Bail, Solid State Sci., 1999, 1, 55–62 CrossRef CAS.
- SAINT, version 7.60A, Bruker Analytical X-ray Instruments, Inc., Madison, WI, 2008 Search PubMed.
- G. M. Sheldrick, SHELXTL, version 6.14, Bruker Analytical X-ray Instruments, Inc.Madison, WI, 2008 Search PubMed.
- O. V. Dolomanov, L. J. Bourhis, R. J. Gildea, J. A. K. Howard and H. Puschmann, J. Appl. Crystallogr., 2009, 42, 339–341 CrossRef CAS.
- A. L. Spek, J. Appl. Crystallogr., 2003, 36, 7–13 CrossRef CAS.
- P. Kubelka and F. Munk, Zeit. Tekn. Physik., 1931, 12, 593–601 Search PubMed.
- G. M. Sheldrick, Acta Crystallogr., Sect. C: Cryst. Struct. Commun., 2015, 71, 3–8 CrossRef PubMed.
- A. M. Rappe, K. M. Rabe, E. Kaxiras and J. D. Joannopoulos, Phys. Rev. B: Condens. Matter Mater. Phys., 1990, 41, 1227–1230 CrossRef PubMed.
- J. S. Lin, A. Qteish, M. C. Payne and V. Heine, Phys. Rev. B: Condens. Matter Mater. Phys., 1993, 47, 4174–4180 CrossRef PubMed.
- J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1997, 78, 1396 CrossRef CAS.
- A. Tkatchenko and M. Scheffler, Phys. Rev. Lett., 2009, 102, 073005 CrossRef PubMed.
- M. J. Frisch, G. W. Trucks, H. B. Schlegel, G. E. Scuseria, M. A. Robb, J. R. Cheeseman, G. Scalmani, V. Barone, B. Mennucci, G. A. Petersson, H. Nakatsuji, M. Caricato, X. Li, H. P. Hratchian, A. F. Izmaylov, J. Bloino, G. Zheng, J. L. Sonnenberg, M. Hada, M. Ehara, K. Toyota, R. Fukuda, J. Hasegawa, M. Ishida, T. Nakajima, Y. Honda, O. Kitao, H. Nakai, T. Vreven, J. A. Montgomery Jr., J. E. Peralta, F. Ogliaro, M. Bearpark, J. J. Heyd, E. Brothers, K. N. Kudin, V. N. Staroverov, R. Kobayashi, J. Normand, K. Raghavachari, A. Rendell, J. C. Burant, S. S. Iyengar, J. Tomasi, M. Cossi, N. Rega, J. M. Millam, M. Klene, J. E. Knox, J. B. Cross, V. Bakken, C. Adamo, J. Jaramillo, R. Gomperts, R. E. Stratmann, O. Yazyev, A. J. Austin, R. Cammi, C. Pomelli, J. W. Ochterski, R. L. Martin, K. Morokuma, V. G. Zakrzewski, G. A. Voth, P. Salvador, J. J. Dannenberg, S. Dapprich, A. D. Daniels, O. Farkas, J. B. Foresman, J. V. Ortiz, J. Cioslowski and D. J. Fox, Gaussian 09, Revision A.02, Gaussian, Inc., Wallingford CT, 2009 Search PubMed.
- G. W. An, H. Zhang, X. L. Cheng, Q. L. Zhuo and Y. C. O. Lv, Struct. Chem., 2008, 19, 613–617 CrossRef CAS.
- K. E. Korzhneva, V. L. Bekenev, O. Y. Khyzhun, A. A. Goloshumova, A. Y. Tarasova, M. S. Molokeev, L. I. Isaenko and A. F. Kurus, J. Solid State Chem., 2021, 294, 121910 CrossRef CAS.
- D. V. Korabel’nikov and Y. N. Zhuravlev, J. Phys. Chem. Solids, 2015, 87, 38–47 CrossRef.
- J. Lin, M. H. Lee, Z. P. Liu, C. T. Chen and C. J. Pickard, Phys. Rev. B: Condens. Matter Mater. Phys., 1999, 60, 13380–13389 CrossRef CAS.
- B. Lei, S. Pan, Z. Yang, C. Cao and D. J. Singh, Phys. Rev. Lett., 2020, 125, 187402 CrossRef CAS PubMed.
Footnote |
| † Electronic supplementary information (ESI) available: Crystal pictures, calculated and experimental X-ray diffraction patterns, UV-vis-NIR diffuse reflectance spectrum, IR spectrum, TG analysis, calculated band gap and birefringence, HOMO–LUMO energy gap and polarizability anisotropy of BO3, NO3, and CO3 and real-space atom-cutting analysis. CCDC 2181425. For ESI and crystallographic data in CIF or other electronic format see DOI: https://doi.org/10.1039/d2sc03771h |
| This journal is © The Royal Society of Chemistry 2022 |