Open Access Article
Open Access ArticleSuperconductivity in single-crystalline ZrTe3−x (x ≤ 0.5) nanoplates†
Jie
Wang‡
 ab,
Min
Wu‡
*a,
Weili
Zhen
ab,
Tian
Li
a,
Yun
Li
ac,
Xiangde
Zhu
a,
Wei
Ning
*a and
Mingliang
Tian
*ad
ab,
Min
Wu‡
*a,
Weili
Zhen
ab,
Tian
Li
a,
Yun
Li
ac,
Xiangde
Zhu
a,
Wei
Ning
*a and
Mingliang
Tian
*ad
aAnhui Key Laboratory of Condensed Matter Physics at Extreme Conditions, High Magnetic Field Laboratory, HFIPS, Chinese Academy of Sciences, Hefei 230031, Anhui, P. R. China. E-mail: minwu@hmfl.ac.cn; ningwei@hmfl.ac.cn; tianml@hmfl.ac.cn
bDepartment of Physics, University of Science and Technology of China, Hefei 230026, P. R. China
cDepartment of Materials Science and Engineering, ARC Centre of Excellence in Future Low-Energy Electronics Technologies (FLEET), Monash University, Clayton, Victoria 3800, Australia
dDepartment of Physics, School of Physics and Materials Science, Anhui University, Hefei 230601, P. R. China
First published on 22nd November 2022
Abstract
Superconductivity with an unusual filamented character below 2 K has been reported in bulk ZrTe3 crystals, a well-known charge density wave (CDW) material, but still lacks in its nanostructures. Here, we systemically investigated the transport properties of controllable chemical vapor transport synthesized ZrTe3−x nanoplates. Intriguingly, superconducting behavior is found at Tc = 3.4 K and can be understood by the suppression of CDW due to the atomic disorder formed by Te vacancies. Magnetic field and angle dependent upper critical field revealed that the superconductivity in the nanoplates exhibits a large anisotropy and two-dimensional character. This two-dimensional nature of superconductivity was further satisfactorily described using the Berezinsky–Kosterlitz–Thouless transition. Our results not only demonstrate the critical role of Te vacancies for superconductivity in ZrTe3–x nanoplates, but also provide a promising platform to explore the exotic physics in the nanostructure devices.
Two-dimensional (2D) layered transition metal dichalcogenides (TMDs) have attracted extensive attention owing to their plethora of remarkable physical properties,1–3 including superconductivity,4–6 charge density wave (CDW),7–9 Mott insulator,10–12 topological phase,13–15 moiré electronics etc.16–19 Among the TMDs, zirconium tellurium compounds have attracted increasing research interest because of the existence of different compositions and properties. ZrTe was theoretically proposed to be a non-abelian topological semimetal with triply degenerate nodes,20,21 and experimentally demonstrated to possess multiple Fermi surfaces with light effective masses by the measurement of quantum oscillations.22 Massless Dirac fermions and negative magnetoresistance were observed in topological material candidate ZrTe2.23–25 Although the topological nature of ZrTe5 remains a puzzle, a number of interesting phenomena have been discovered in transport experiments, such as log-periodic oscillations,26 the quantum Hall effect,27 four-fold splitting of the non-zero Landau levels,28 gigantic magneto-chiral anisotropy,29etc. Another fascinating composition is layered ZrTe3, which was known for decades as a CDW material.30
ZrTe3 crystallizes in TaSe3-type structure (space group P21/m), which consists of a quasi-one-dimensional chain along the b-axis and quasi-two-dimensional layer along the ac plane.31 Electronically, a resistance anomaly associated with the formation of the CDW state due to Fermi surface nesting is established at 63 K in ZrTe3 crystals.30 More importantly, filamentary superconductivity below Tc = 2 K is observed at ambient pressure when the CDW is quenched.32 To increase the superconducting transition temperature Tc, metal atom intercalation,33 element substitution34 and pressure32 have been widely used. However, most studies on the superconductivity of ZrTe3 were focused on the bulk crystals, and experimental evidence for the superconductivity in low-dimensional ZrTe3 nanoplates or nanowires was rarely reported. Previous studies uncovered that the mechanically exfoliated ZrTe3 nanowires display a semiconducting behavior,35 and the ZrTe3 nanoribbons prepared by chemical vapor deposition exhibit an unexpected ferromagnetism coming from structural imperfection and edge-states.36 These observations limit the possibilities for unveiling the superconducting state in ZrTe3 nanostructures. Therefore, whether the superconductivity survives in the ZrTe3 nanostructures is still unknown.
In this work, we carried out comprehensive electrical transport measurements on chemical vapor transport (CVT) synthesized ZrTe3−x nanoplates, which were characterized by scanning electron microscopy (SEM), energy dispersive X-ray (EDX) spectroscopy and Raman spectroscopy. The ZrTe3−x nanoplates show a superconducting transition at Tc = 3.4 K, which was attributed to the existence of Te vacancies that are determined by using the EDX spectrum. Moreover, the superconducting behavior in ZrTe3−x nanoplates shows strong anisotropy with magnetic field orientation and 2D characteristics of that are consistent with the Berezinskii–Kosterlitz–Thouless (BKT) transition. Our results signify that ZrTe3−x nanoplates offer an opportunity for discovering novel phenomena in the nanostructure devices.
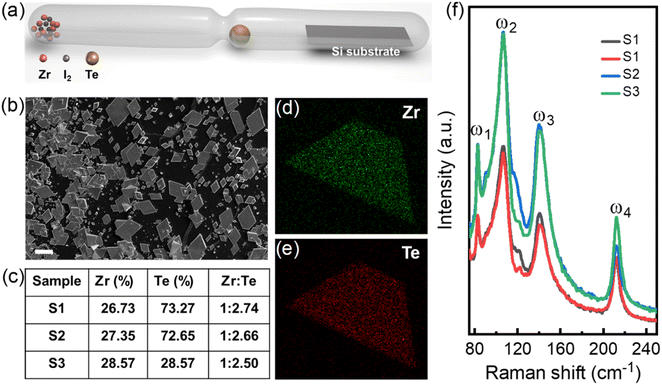
Usually, the chemical vapor deposition method was employed to grow high-quality nanoplates/nanowires of layered TMDs.37 Very recently, it had been demonstrated that the CVT method also can be used to directly prepare thin flakes of TMDs with comparable quality by slowing down the growth rate.38,39 Moreover, because of the CVT growth progress in an evacuated ampule, it is a useful way to grow ambient sensitive materials.40 Thus, in this work, we utilized the CVT approach to synthesize the ZrTe3 nanoplates in a neck ampule with iodine as a transport agent (to slow down the reaction rate, the ingredients are separated in the ampule), as shown in Fig. 1(a). 5 g Zr pieces and 100 mg I2 were placed in an evacuated quartz tube, together with 0.3 g Te located at the position of the narrow neck of a quartz tube with a diameter of less than 1 cm. The evacuated ampule was placed into a horizontal two zone furnace, where the source zone was heated to 540 °C and the growth zone was heated to 450 °C at a rate of 1 °C per minute. After 150 minutes, the furnace was cooled down to room temperature. To study the transport properties, the grown ZrTe3 nanoplates were transferred onto the conventional SiO2/Si substrates in the glovebox, and then onto patterned electrodes by standard electron-beam lithography and lift-off techniques. To prevent contamination or oxidation, the devices were covered with poly (methyl meth-acrylate) layers on the surface before the transport measurements.
Fig. 1(b) shows the SEM image of the as-grown nanoplates on a pure silicon substrate, where most are rhombus or parallelogram shaped. However, when transferred onto SiO2/Si substrates, the shapes of nanoplates changed into trapezoid (see the upper inset of Fig. 2(a)). The corresponding EDX data are shown in Fig. 1(c). Apparently, the nanoplates are composed of Zr and Te with an atomic ratio less than 1![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 3, indicating the presence of Te deficiencies in the nanoplates, and the stoichiometry should be ZrTe3−x (x ≤ 0.5). Fig. 1(d) and (e) show the elemental mappings measured by EDX, from which Zr and Te atoms are uniformly distributed across the nanoplates. More structural characterizations (HRTEM and SAED) about ZrTe3−x nanoplates can be seen in the ESI Fig. S1.† The typical Raman spectra of synthesized ZrTe3−x nanoplates are shown in Fig. 1(f). Four obvious Raman peaks are found at ω1 = 83 cm−1, ω2 = 106 cm−1, ω3 = 141 cm−1, and ω4 = 212 cm−1, which are consistent with the previous reports.31,33
3, indicating the presence of Te deficiencies in the nanoplates, and the stoichiometry should be ZrTe3−x (x ≤ 0.5). Fig. 1(d) and (e) show the elemental mappings measured by EDX, from which Zr and Te atoms are uniformly distributed across the nanoplates. More structural characterizations (HRTEM and SAED) about ZrTe3−x nanoplates can be seen in the ESI Fig. S1.† The typical Raman spectra of synthesized ZrTe3−x nanoplates are shown in Fig. 1(f). Four obvious Raman peaks are found at ω1 = 83 cm−1, ω2 = 106 cm−1, ω3 = 141 cm−1, and ω4 = 212 cm−1, which are consistent with the previous reports.31,33
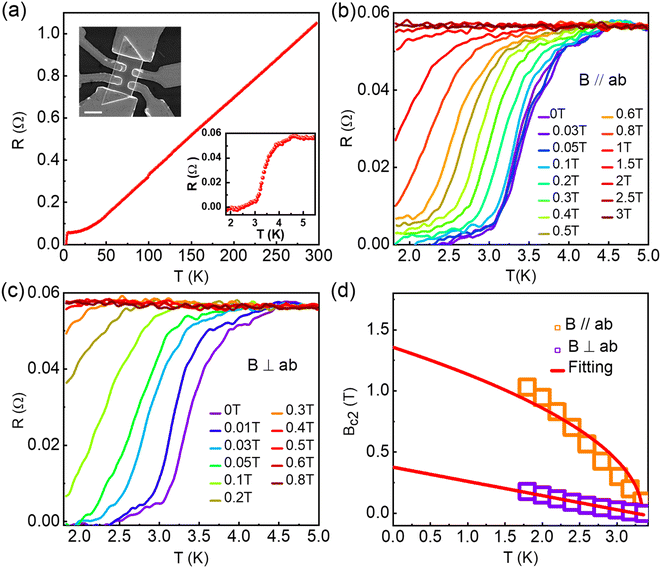
Fig. 2(a) shows the temperature dependence of nanoplate resistance under a zero magnetic field. Metallic behavior was observed above 4.5 K, below which the resistance begins to decrease and it drops to zero at 2.3 K (as indicated by the enlarged R(T) curve in the bottom inset of Fig. 2(a)), indicating the occurrence of a superconducting transition in ZrTe3−x nanoplates. To quantitatively analyze the superconductivity, the “50% criterion” was used to define Tc (upper critical field Bc) as the temperature (magnetic field) at which the resistance drops to 50% of the normal state value. In this case, Tc is about 3.4 K, which is larger than that of bulk ZrTe3 crystals.28 Superconductivity transport of different samples can be seen from ESI Fig. S2–S5.† Previous studies have demonstrated that high growth temperature and doping can enhance the superconducting transition temperature Tc of bulk ZrTe3 through inducing disorders that suppress the CDW state.34,41 Superconductivity and charge density waves are in significant competition and the existence of charge density waves is detrimental to superconductivity. In our samples, by introducing appropriate tellurium vacancies, we found that the charge density wave was significantly suppressed and the superconductivity emerged during transport measurements, which is consistent with previous results, such as element substitution, intercalation and high pressure. Therefore, we conclude that the observed superconductivity with higher Tc in ZrTe3−x nanoplates can be ascribed to the presence of Te vacancies determined from the EDX spectrometry (Fig. 1(c) and ESI Table S1†).
To study the anisotropy of superconductivity in ZrTe3−x nanoplates, we investigated the temperature dependence of resistance when different magnetic fields are parallel and perpendicular to the sample plane and the results are presented in Fig. 2(b) and (c). In both configurations, the superconducting transition temperature shifts to a lower temperature with the increase in the magnetic field. The magnetic field that completely suppresses the superconductivity is about 2.5 T for the parallel field direction (B‖ab), which is 5 times larger than that for the perpendicular scenario (B⊥ab, ∼0.5 T). The large anisotropy of the upper critical field indicates 2D characteristics of superconductivity in ZrTe3−x nanoplates. In addition, the dependence of the out-of-plane upper critical field on a temperature close to Tc displays a linear behavior, as shown in Fig. 2(d), which is in accordance with the standard Ginzburg–Landau (GL) theory:42
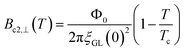 | (1) |
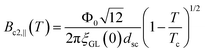 | (2) |
To further uncover the dimensionality of superconductivity in ZrTe3−x nanoplates, we performed the angle dependent superconducting transition measurements. Fig. 3(a) shows the magnetic field direction dependence of nanoplate resistance at T = 1.8 K, where θ is the tilted angle between the direction of current and magnetic field B, as schematically illustrated in the inset of Fig. 3(a). With decreasing angle θ, the superconducting transition shifts to a high magnetic field and the upper critical field reaches a maximum at θ = 0° (Fig. 3(b)). It has been verified that the upper critical field in a 2D superconductor is significantly enhanced when the magnetic field is parallel to the sample plane,44 which is consistent with our results, as shown in Fig. 3. Furthermore, the relationship between θ and upper critical field Bc2 exhibits a sharp cusp, as plotted and shown in Fig. 3(b). All these experimental pieces of evidence enable us to deduce that the observed superconductivity in ZrTe3−x nanoplates has a 2D nature. For a superconductor with 2D characteristics, the angle dependent Bc2 can be well described by using the Thinkham model:44,45.
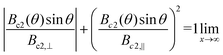 | (3) |
As indicated by the red solid curve in the inset of Fig. 3(b), the experimental data can be well captured by using the Thinkham model. It is worth pointing out that the 3D anisotropic GL model46 is also utilized to fit Bc2(θ), as indicated by the blue solid curve in the inset of Fig. 3(b). Obviously, the fitting cannot describe the Bc2(θ) curve.
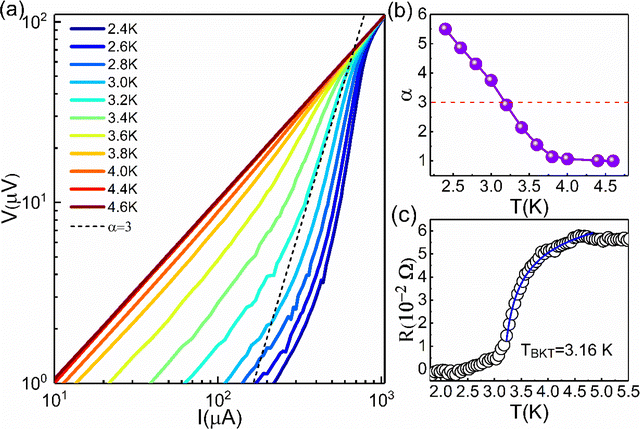
It is well known that the transport properties for 2D characteristic superconductivity feature a BKT transition that is characterized by the BKT temperature TBKT.47Fig. 4(a) shows the I–V curves on a log–log scale with temperature ranging from 2.4 K to 4.6 K. The V–I dependence is found to obey a power-law, V ∝ Iα, and α represents slopes of the curve when the current approaches the linear region. The linear line has a slope of 1 in the high temperature region (4.4–4.6 K), which means the complete disappearance of superconductivity. The dashed line corresponds to V ∝ I3 at the BKT transition. The temperature dependence of the exponent α is shown in Fig. 4(b). It can be seen that the value of α increases rapidly with T < Tc, and approaches 3 at a temperature of ∼3.17 K, which is thus identified as TBKT. In addition, according to the BKT model, the R(T) curve under a zero magnetic field at just above TBKT follows the Halperin–Nelson equation:42
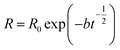 | (4) |
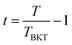 As shown in Fig. 4(c), the R(T) data can be well fitted by using eqn (3), yielding TBKT = 3.16 K, which is highly consistent with the value obtained from the power-law analysis of the I–V curves. The existence of the BKT transition provides further strong evidence for the 2D nature of superconductivity in ZrTe3−x nanoplates.
As shown in Fig. 4(c), the R(T) data can be well fitted by using eqn (3), yielding TBKT = 3.16 K, which is highly consistent with the value obtained from the power-law analysis of the I–V curves. The existence of the BKT transition provides further strong evidence for the 2D nature of superconductivity in ZrTe3−x nanoplates.
In conclusion, we have presented systematic transport properties of CVT grown ZrTe3−x nanoplates. The superconducting transition is found at T = 3.4 K due to the existence of Te vacancies in the nanoplates, as revealed by the EDX spectrum. Meanwhile, the observed superconductivity exhibits large anisotropy with the magnetic field direction and features the 2D nature of the superconducting transition that was demonstrated by the existence of the BKT transition. Our results suggest thin flakes of layered TMDs prepared by CVT provide a viable way to study the potential properties in the nanostructures.
Data availability
The data that support the findings of this study are available from the corresponding author upon reasonable request.Conflicts of interest
The authors have no conflicts of interests.Acknowledgements
This work was supported by the National Key Research and Development Program of China (Grant No. 2021YFA1600201) and the Natural Science Foundation of China (No. U19A2093, U2032214, and U2032163)References
- L. F. Gao, C. Y. Ma, S. R. Wei, A. V. Kuklin, H. Zhang and H. Agren, ACS Nano, 2021, 15, 954–965 CrossRef CAS PubMed
.
- Y. Zhang, P. Huang, J. Guo, R. C. Shi, W. C. Huang, Z. Shi, L. M. Wu, F. Zhang, L. F. Gao, C. Li, X. W. Zhang, J. L. Xu and H. Zhang, Adv. Mater., 2020, 32, 2001082 CrossRef CAS PubMed
.
- H. Qiao, Z. Y. Huang, X. H. Ren, S. H. Liu, Y. P. Zhang, X. Qi and H. Zhang, Adv. Opt. Mater., 2020, 8, 1900765 CrossRef CAS
.
- X. X. Xi, Z. F. Wang, W. W. Zhao, J. H. Park, K. T. Law, H. Berger, L. Forro, J. Shan and K. F. Mak, Nat. Phys., 2016, 12, 139–143 Search PubMed
.
- J. M. Lu, O. Zheliuk, I. Leermakers, N. F. Q. Yuan, K. T. Law and J. T. Ye, Science, 2015, 350, 1353–1357 CrossRef CAS PubMed
.
- W. Shi, J. T. Ye, Y. J. Zhang, R. Suzuki, M. Yoshida, J. Miyazaki, N. Inoue, Y. Saito and Y. Iwasa, Science, 2015, 5, 12534 CAS
.
- Y. J. Yu, F. Y. Yang, L. F. Lu, Y. J. Yan, Y. H. Cho, L. G. Ma, X. H. Niu, S. Kim, Y. W. Son, D. L. Feng, S. Y. Li, S. W. Cheong, X. H. Chen and Y. B. Zhang, Nat. Nanotechnol., 2015, 10, 270–276 CrossRef CAS PubMed
.
- X. D. Zhu, H. C. Lei and C. Petrovic, Phys. Rev. Lett., 2011, 106, 246404 CrossRef PubMed
.
- Y. K. Nakata, K. Sugawara, A. Chainani, H. Oka, C. H. Bao, S. H. Zhou, P. Y. Chuang, C. M. Cheng, T. Kawakami, Y. Saruta, T. Fukumura, S. Y. Zhou, T. Takahashi and T. Sato, Nat. Commun., 2021, 12, 5873 CrossRef CAS PubMed
.
- Y. Nakata, K. Sugawara, R. Shimizu, Y. Okada, P. Han, T. Hitosugi, K. Ueno, T. Sato and T. Takahashi, NPG Asia Mater., 2016, 8, e321 CrossRef CAS
.
- Y. Nakata, T. Yoshizawa, K. Sugawara, Y. Umemoto, T. Takahashi and T. Sato, ACS Appl. Nano Mater., 2018, 1, 1456–1460 CrossRef CAS
.
- B. H. Moon, J. J. Bae, M. K. Joo, H. Choi, G. H. Han, H. Lim and Y. H. Lee, Nat. Commun., 2018, 9, 2052 CrossRef PubMed
.
- J. Xia, D. F. Li, J. D. Zhou, P. Yu, J. H. Lin, J. L. Kuo, H. B. Li, Z. Liu, J. X. Yan and Z. X. Shen, Small, 2017, 13, 1701887 CrossRef PubMed
.
- F. Leonard, W. L. Yu, K. C. Collins, D. L. Medlin, J. D. Sugar, A. A. Talin and W. Pan, ACS Appl. Mater. Interfaces, 2017, 9, 37041–37047 CrossRef CAS PubMed
.
- Q. Q. Liu, F. C. Fei, B. Chen, X. Y. Bo, B. Y. Wei, S. Zhang, M. H. Zhang, F. J. Xie, M. Naveed, X. G. Wan, F. Q. Song and B. G. Wang, Phys. Rev. B, 2019, 99, 155119 CrossRef CAS
.
- C. H. Jin, Z. Tao, T. X. Li, Y. Xu, Y. H. Tang, J. C. Zhu, S. Liu, K. J. Watanabe, T. Taniguchi, J. C. Hone, L. Fu, J. Shan and K. F. Mak, Nat. Mater., 2022, 20, 940–944 CrossRef PubMed
.
- Y. Xu, S. Liu, D. A. Rhodes, K. J. Watanabe, T. Taniguchi, J. Hone, V. Elser, K. F. Mak and J. Shan, Nature, 2020, 587, 214–218 CrossRef CAS PubMed
.
- Y. H. Tang, L. Z. Li, T. X. Li, Y. Xu, S. Liu, K. Barmak, K. J. Watanabe, T. Taniguchi, A. H. MacDonald, J. Shan and K. F. Mak, Nature, 2020, 579, 353–358 CrossRef CAS PubMed
.
- L. Wang, E. M. Shih, A. Ghiotto, L. D. Xian, D. A. Rhodes, C. Tan, M. Claassen, D. M. Kennes, Y. S. Bai, B. Kim, K. J. Watanabe, T. Taniguchi, X. Y. Zhu, J. Hone, A. Rubio, A. N. Pasupathy and C. R. Dean, Nat. Mater., 2020, 19, 861–866 CrossRef CAS PubMed
.
- H. M. Weng, C. Fang, Z. Fang and X. Dai, Phys. Rev. B, 2016, 94, 165201 CrossRef
.
- A. Bouhon, Q. S. Wu, R. J. Slager, H. M. Weng, O. V. Yazyev and T. Bzdusek, Nat. Phys., 2020, 16, 1137–1143 Search PubMed
.
- W. L. Zhu, J. B. He, Y. J. Xu, S. Zhang, D. Chen, L. Shan, Y. F. Yang, Z. A. Ren, G. Li and G. F. Chen, Phys. Rev. B, 2020, 101, 245127 CrossRef CAS
.
- P. Tsipas, D. Tsoutsou, S. Fragkos, R. Sant, C. Alvarez, H. Okuno, G. Renaud, R. Alcotte, T. Baron and A. Dimoulas, ACS Nano, 2018, 12, 1696–1703 CrossRef CAS PubMed
.
- H. C. Wang, C. H. Chan, C. H. Suen, S. P. Lau and J. Y. Dai, ACS Nano, 2019, 13, 6008–6016 CrossRef CAS PubMed
.
- J. Wang, Y. H. Wang, M. Wu, J. B. Li, S. P. Miao, Q. Y. Hou, Y. Li, J. H. Zhou, X. D. Zhu, Y. M. Xiong, W. Ning and M. L. Tian, Appl. Phys. Lett., 2022, 120, 163103 CrossRef CAS
.
- H. H. Wang, H. W. Liu, Y. N. Li, Y. J. Liu, J. F. Wang, J. Liu, J. Y. Dai, Y. Wang, L. Li, J. Q. Yan, D. Mandrus, X. C. Xie and J. Wang, Sci. Adv., 2018, 4, eaau5096 CrossRef CAS PubMed
.
- F. D. Tang, Y. F. Ren, P. P. Wang, R. D. Zhong, J. Schneeloch, S. Y. Yang, K. Yang, P. A. Lee, G. D. Gu, Z. H. Qiao and L. Y. Zhang, Nature, 2021, 569, 537–541 CrossRef PubMed
.
- J. Y. Wang, Y. X. Jiang, T. H. Zhao, Z. L. Dun, A. L. Miettinen, X. S. Wu, M. Mourigal, H. D. Zhou, W. Pan, D. Smirnov and Z. G. Jiang, Nat. Commun., 2021, 12, 6758 CrossRef CAS PubMed
.
- Y. J. Wang, H. F. Legg, T. Bomerich, J. H. Park, S. Biesenkamp, A. A. Taskin, M. Braden, A. Rosch and Y. C. Ando, Phys. Rev. Lett., 2022, 128, 176602 CrossRef CAS PubMed
.
- M. Hoesch, X. Y. Cui, K. Y. Shimada, C. Battaglia, S. I. Fujimori and H. Berger, Phys. Rev. B, 2009, 80, 075423 CrossRef
.
- S. L. Gleason, Y. Gim, T. Byrum, A. Kogar, P. Abbamonte, E. Fradkin, G. J. MacDougall, D. J. Van Harlingen, X. D. Zhu, C. Petrovic and S. L. Cooper, Phys. Rev. B, 2015, 91, 155124 CrossRef
.
- K. M. Gu, R. A. Susilo, F. Ke, W. Deng, Y. J. Wang, L. K. Zhang, H. Xiao and B. Chen, J. Phys.: Condens. Matter, 2018, 30, 385701 CrossRef PubMed
.
- X. D. Zhu, H. C. Lei and C. Petrovic, Phys. Rev. Lett., 2011, 106, 246404 CrossRef PubMed
.
- X. D. Zhu, W. Ning, L. J. Li, L. S. Ling, R. R. Zhang, J. L. Zhang, K. F. Wang, Y. Liu, L. Pi, Y. C. Ma, H. F. Du, M. L. Min, Y. P. Sun, C. Petrovic and Y. H. Zhang, Sci. Rep., 2016, 6, 26974 CrossRef CAS PubMed
.
- A. Geremew, M. A. Bloodgood, E. Aytan, B. W. K. Woo, S. R. Corber, G. Liu, K. Bozhilov, T. T. Salguero, S. Rumyantev, M. P. Rao and A. A. Balandin, IEEE, 2018, 39, 735–738 CAS
.
- X. Yu, X. K. Wen, W. F. Zhang, L. Yang, H. Wu, X. Lou, Z. J. Xie, Y. Liu and H. X. Chang, CrystEngCom, 2019, 21, 5586–5594 RSC
.
- B. J. Tang, X. W. Wang, M. J. Han, X. D. Xu, Z. W. Zhang, C. Zhu, X. Cao, Y. M. Yang, Q. D. Fu, J. Q. Yang, X. J. Li, W. B. Gao, J. D. Zhou, J. H. Lin and Z. Liu, Nat. Electron., 2022, 5, 224–232 CrossRef CAS
.
- J. Y. Wang, H. S. Zheng, G. C. Xu, L. F. Sun, D. K. Hu, Z. X. Lu, L. Liu, J. Y. Zheng, C. G. Tao and L. Y. Jiao, J. Am. Chem. Soc., 2016, 138, 16216–16219 CrossRef CAS PubMed
.
- K. Yuan, R. Y. Yin, X. Q. Li, Y. M. Han, M. Wu, S. L. Chen, S. Liu, X. L. Xu, K. J. Watanabe, T. Taniguchi, D. A. Muller, J. J. Shi, P. Gao, X. S. Wu, Y. Ye and L. Dai, Adv. Funct. Mater., 2019, 29, 1904032 CrossRef
.
- D. K. Hu, G. C. Xu, L. Xing, X. X. Yan, J. Y. Wang, J. Y. Zheng, Z. X. Lu, P. Wang, X. Q. Pan and L. Y. Jiao, Angew. Chem., Int. Ed., 2017, 56, 3611–3615 CrossRef CAS PubMed
.
- X. Y. Zhu, B. Lv, F. Y. Wei, X. Y. Xue, B. Lorenz, L. Z. Deng, Y. Y. Sun and C. W. Chu, Phys. Rev. B, 2013, 87, 024508 CrossRef
.
- C. Xu, L. B. Wang, Z. B. Liu, L. Chen, J. K. Guo, N. Kang, X. L. Ma, H. M. Cheng and W. C. Ren, Nat. Mater., 2015, 14, 1135–1141 CrossRef CAS PubMed
.
- P. Baidya, D. Sahani, H. K. Kundu, S. Kaur, P. Tiwari, V. Bagwe, J. Jesudasan, A. Narayan, P. Raychaudhuri and A. Bid, Phys. Rev. B, 2021, 104, 174510 CrossRef CAS
.
- J. W. Zeng, E. F. Liu, Y. J. Fu, Z. Y. Chen, C. Pan, C. Y. Wang, M. Wang, Y. J. Wang, K. Xu, S. H. Cai, X. X. Yan, Y. Wang, X. W. Liu, P. Wang, S. J. Liang, Y. Cui, H. Y. Hwang, H. T. Yuan and F. Miao, Nano Lett., 2018, 18, 1410–1415 CrossRef CAS PubMed
.
- Y. C. Zou, Z. G. Chen, E. Z. Zhang, F. X. Xiu, S. Matsumura, L. Yang, M. Hong and J. Zou, Nanoscale, 2017, 9, 16591 RSC
.
- Y. T. Chan, P. L. Alireza, K. Y. Yip, Q. Niu, K. T. Lai and S. K. Goh, Phys. Rev. B, 2017, 96, 180504 CrossRef
.
- Q. L. He, H. C. Liu, M. Q. He, Y. H. Lai, H. T. He, G. Wang, K. T. Law, R. Lortz, J. N. Wang and I. K. Sou, Nat. Commun., 2014, 5, 4247 CrossRef CAS PubMed
.
Footnotes |
| † Electronic supplementary information (ESI) available. See DOI: https://doi.org/10.1039/d2na00628f |
| ‡ These authors contributed equally to this work. |
| This journal is © The Royal Society of Chemistry 2023 |