Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Gentle tension stabilizes atomically thin metallenes†
Kameyab Raza
Abidi
 and
Pekka
Koskinen
and
Pekka
Koskinen
 *
*
Nanoscience Center, Department of Physics, University of Jyväskylä, 40014 Jyväskylä, Finland. E-mail: pekka.j.koskinen@jyu.fi
First published on 1st October 2024
Abstract
Metallenes are atomically thin two-dimensional (2D) materials lacking a layered structure in the bulk form. They can be stabilized by nanoscale constrictions like pores in 2D covalent templates, but the isotropic metallic bonding makes stabilization difficult. A few metallenes have been stabilized but comparison with theory predictions has not always been clear. Here, we use density-functional theory calculations to explore the energetics and dynamic stabilities of 45 metallenes at six lattices (honeycomb, square, hexagonal, and their buckled counterparts) and varying atomic densities. We found that of the 270 different crystalline lattices, 128 were dynamically stable at sporadic densities, mostly under tensile strain. At the energy minima, lattices were often dynamically unstable against amorphization and the breaking down of metallene planarity. Consequently, the results imply that crystalline metallenes should be seen through a novel paradigm: they should be considered not as membranes with fixed structures and lattice constants but as yielding membranes that can be stabilized better under tensile strain and low atomic density. Following this paradigm, we rank the most promising metallenes for 2D stability and hope that the paradigm will help develop new strategies to synthesize larger and more stable metallene samples for plasmonic, optical, and catalytic applications.
The early days of two-dimensional (2D) materials research were greatly influenced by the easiness of graphene exfoliation.1 Since then, synthesis methods have significantly evolved, producing 2D materials beyond graphene with unique applications.2–5 This evolution has been enabled by 2D materials’ strong covalent in-plane bonding and weak van der Waals out-of-plane bonding.
In this respect, metallenes are fundamentally different. Their metallic, non-directional bonding favors 3D aggregation, makes exfoliation challenging, and renders large, free-standing membranes unstable.6,7 Small metallene patches have nevertheless been successfully stabilized by various means. One example is the stabilization in the pores of 2D covalent templates as side products from migrating metal residues on surfaces.8–10 The second example is to use electron irradiation to ionize Se atoms from MoSe2 transition metal dichalcogenides to make patches of 2D Mo11 or Ag atoms from AuAg alloy to produce a small suspended Au monolayer.12 A third example is the etching of Ti3C2 of nanolaminated Ti3AuC2 to create large-area sheets of 2D Au, goldene.13 We emphasize that, like in the experiments, our focus here is on suspended crystalline metallenes, not supported or amorphous ones. The stabilization is challenging, but metallic bonding is the very feature that makes these materials unique among other 2D materials14–16 and attractive for plasmonic, electronic, quantum dot, energy, biomedical, and catalytic applications.17–19 This attractiveness has motivated a fair amount of research.6,7,20–22
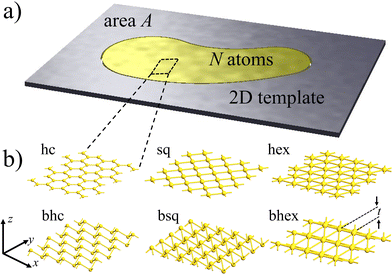
Meanwhile, density-functional theory (DFT) simulations have played a significant role in predicting diverse properties of metallenes.23–28 The dynamical stability analysis of monolayers has been a particularly valuable procedure guiding the experiments.29 So far, simulations have aimed to align with the traditional paradigm of covalent 2D materials: they have been considered periodic 2D bulk with optimized lattice constant corresponding to the energy minimum. Unfortunately, comparing simulations with a limited number of metallene samples has been ambiguous regarding both lattice symmetry and bond lengths.10,13,30–33 This ambiguity is understandable, as the number of metal atoms N in the pore may vary, while the pore area A remains fixed (Fig. 1a). Thus, given their high ductility with respect to the covalent template,15 metal patches could be strained, making stability not intrinsic to the metal but dependent on the atomic density N/A.
Therefore, in this Communication, we address the question: to what extent do the dynamical stabilities of various metallene lattices depend on atomic densities? We address this question by DFT calculations of the energetic and dynamic stabilities of six metallene lattices (honeycomb, square, hexagonal, and their buckled counterparts) for 45 elements as a function of atomic density, quantified in terms of specific area a = A/N. Unlike covalent 2D materials, we found that metallene stability is highly sensitive to atomic density. Out of the 270 monolayers, 128 were stable at sporadic densities, dominantly under tensile stress, and only occasionally at zero stress. Supported by molecular dynamics simulations, we also found that different lattices can coexist at a density where any single lattice would be unstable. Juxtaposed with metallenes’ softness and ductility,15 these results suggest a new stability paradigm that abandons fixed lattice constants and considers metallenes as yielding patches that can be stabilized better under tensile strain. In other words, the energy of the patch is not minimized separately but together with the total energy of the stabilizing interface.
To address the above question, we investigated the energetics and dynamical stabilities of the systems (Fig. 1) by density-functional simulations.34,35 We used the PBE exchange–correlation functional and an LCAO basis set,36 a level of theory that has proven robust for metallenes and that suffices well for our purposes of investigating general trends in stabilities and geometries.37 The lattices were modeled by minimal cells periodic in the xy-plane using 13 × 13 × 1 k-point sampling (Fig. 1b). The buckled lattices had initial-guess thicknesses of 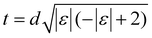 , where d is the equilibrium bond length of the corresponding flat lattice and ε is the biaxial strain. For details of the computational methods, see ESI.†
, where d is the equilibrium bond length of the corresponding flat lattice and ε is the biaxial strain. For details of the computational methods, see ESI.†
The N atoms in the periodic cell of area A were then optimized for each element and lattice type, yielding the cohesive energy per atom ELcoh(a) = EL(a)/N − Efree as a function of specific area a = A/N, where EL(a) is the optimized energy of lattice L and Efree is the energy of a single atom inside a 15 Å cube. The dynamical stabilities were analyzed by calculating phonon dispersion spectra and examining the absence of phonon modes with imaginary frequencies.38 This systematic approach resulted in a fair number of calculations: a total of 45 elements with 6 lattices were optimized for 40–70 different values of a, with complete phonon dispersion spectra calculations for most configurations, giving some ≳15![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 phonon calculations in total.
000 phonon calculations in total.
To discuss the main features of the results, let us use Au as an illustrative example. All six lattices show typical cohesion energy curves as a function of a (Fig. 2a). Buckled honeycomb and hexagonal lattices bind strongest, while honeycomb and square bind weakest. All lattices have one energy minimum, except for buckled square, which has two. The cohesive energies are large, 88% (hexagonal) and 91% (buckled honeycomb) of the 3D bulk cohesion.
However, only a fraction of the configurations are dynamically stable (solid line segments in Fig. 2a). Four of the six lattices are stable at some densities (bhc, bsq, bhex, and hex), and two are never stable (hc and sq). Overall, dynamically stable 2D Au can be found at sporadic area intervals of 3.5–4.0 Å2 (bhc), 4.1–4.4 Å2 (bsq), and 6.7–7.8 Å2 (hex). Such restricted stability starkly contrasts covalent 2D materials such as graphene, silicene, or hexagonal boron nitride (hBN) that are stable over considerably larger density ranges (Fig. S2†).
Phonon dispersion spectra provide the cohesion energy curves with a complementary viewpoint. Near the onset of buckling (a ≈ 6.0 Å2), hexagonal and buckled hexagonal lattices are near degenerate, but their phonon mode behaviors differ strongly. The buckled hexagonal lattice acquires prominent negative phonon energies, implying acute instability (Fig. 2b). The flat hexagonal lattice, in turn, becomes unstable through modestly negative energies in long-wavelength acoustic modes around the high symmetry points of the Brillouin zone. Most stable lattices become unstable through the gradual emergence of long-wavelength negative-energy phonon modes. We also investigated unstable phonon modes at or near the energy minima of different lattices. In short, it appears that following the unstable phonon modes leads to amorphous structures and the disruption of planarity, a situation not interesting for experiments.
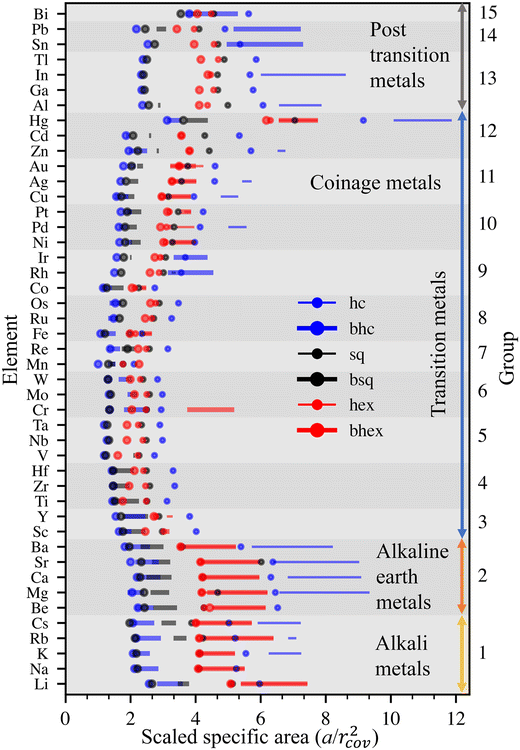
Features similar to Au can also be found in the other 44 elements. We compare the stabilities of various elements using specific areas scaled by the squares of elements’ covalent radii, a′ = a/rcov2.39 This scaling establishes visually organized trends in the positions of the cohesion energy minima of the six lattices and provides an illustrative viewpoint for inspecting the stabilities further (Fig. 3).
The best dynamical stabilities occur for alkali and earth alkali metals of periodic table groups 1 and 2, Mg showing the most extensive range of stable densities among all elements. Among transition metals (groups 3–12), the most extensive stability ranges occur for early and late transition metals, including coinage metals. In the middle of the transition series, dynamical stability is severely limited. In post-transition metals, the dynamical stabilities vary wildly. Metals are stable at very different densities, and stable intervals are separated by more significant gaps than in groups 1 and 2. Ga and Tl are stable only at singular densities, whereas Hg is stable here and there over an extensive density range.
In terms of intrinsic material properties, stability is emphasized for simple metals and metals with filled (or nearly filled) d-shells, whose properties are determined by s- and p-electrons. In other words, the directional bonding of d-orbitals decreases the overall 2D stability. Stability correlates negatively with the difference in 3D and 2D cohesion energies, small differences resulting in more stable metallenes.27 Moreover, the melting point of 3D bulk, which is caused by directional bonding of partly filled d-shell, correlates negatively with metallene stability.
The results compare well with the experiments done so far. The hexagonal lattice of Au is stable between 6.7–7.8 Å2 (2.8–3.0 Å bond lengths), which agrees with previous experiments.12,13 The bhc lattices in Sn, Pb, Bi, Cr, Mo, W, Rh, and Ir have two minima, one at higher and one at lower density. Certain experimentally observed bhc lattices appear to favor lower density.40–42 Dynamically stable Fe is found only in bhc, hex, and bhex lattices, supporting the viewpoint that the Fe monolayer lattice reported by Zhao et al.30 is in carbide and not in elemental form.31,32 Cr presents a notable exception in the stability among transition metals. It also has been observed experimentally, although the agreement with theory was ambiguous, presumably due to finite size effects.33
In particular, the results provide valuable insights to expedite future experiments. Lattices’ energy minima are dynamically stable merely occasionally, mostly for hexagonal lattices. As a rule of thumb for all lattices, metallenes are most likely dynamically stable under gentle tensile strain, like soap membranes stabilized by a hoop. They are hardly ever dynamically stable under compressive strain. For best stability, metallenes should be seen through a novel paradigm, considering them not as membranes with fixed lattice constants but as yielding membranes that can be stabilized better under gentle tension.
The results also teach a lesson about metallic bonding in 2D. Honeycomb lattices with covalent bonds can be stretched substantially. The atomic density range [specific area (max − min)/min] for dynamic stability is 43.1 % for graphene, 45.5 % for silicene, and 39.2 % for hBN (Fig. S2†). Such large stability ranges are due to bond directionality supporting lattice symmetry (Fig. S2†). However, metallic bonding is non-directional and nonlocalized in character. For many 2D metals, this character suppresses dynamic stabilities, rendering most of the studied 2D metal lattices to have stable atomic density ranges considerably smaller than covalent 2D materials. For others, lattices break down gradually upon sufficient tensile strain—the non-directionality of metallic bonding cannot preserve lattice symmetry. In other words, the mechanical ductility of metals inevitably comes along with sensitivity for tensile strain: the strain must be gentle.
So far, the focus has been on the stability of a single lattice, but certain lattices may coexist at nearly arbitrary mean density. This phenomenon is familiar from the coexistence of regular 3D phases. Let a pore of area A be filled by N = N1 + N2 atoms, N1 = xN (x∈[0,1]) atoms in lattice 1 with specific area a1 and N2 = (1 − x)N atoms in lattice 2 with specific area a2, with the mean specific area a0 = A/N. The mean energy E/N = xEcoh1(a1) + (1 − x)Ecoh2(a2) is minimized when
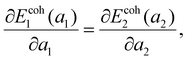 | (1) |
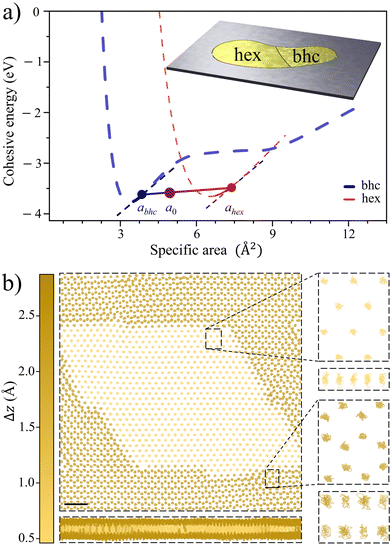 | ||
| Fig. 4 Illustrating lattice coexistence in Au. (a) Cohesion energy curves of Au for hexagonal and buckled honeycomb lattices (adopted from Fig. 2a). Buckled honeycomb lattice at abhc = 3.85 Å2 and hexagonal at ahex = 7.41 Å2 have the same stress and may exhibit coexistence with Ecohbhc/hex(a) along the line shown. Inset: schematic of a pore of area A filled by coexisting bhc and hex lattices at a0 = 5.41 Å2 mean specific area, with x = 56% of atoms in bhc lattice taking a fraction of xabhc/a0 = 40% of the total area A, corresponding to the situation in the main figure. (b) Coexisting bhc (dark) and hex (light) lattices in a 97.9 nm2 supercell with 2018 atoms. The color comes from the vertical thickness Δz of the atom's immediate neighborhood. Top and side views of 10 ps trajectories for atoms within the boxed areas, demonstrating lattice coexistence and dynamical stability, adopted from a 0.25 ns Langevin molecular dynamics simulation at 300 K, using a force field with cohesion energy curves similar to those in panel a (see ESI† for details). | ||
Let us summarize our results to address the following question: which elements show the best overall potential for experimental stability? The likelihood of experimental success increases upon increasing energetic and dynamical stability. Therefore, we rank the energetic stability of element i using the quantity 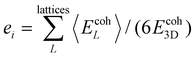 , where 〈EcohL〉 is the average cohesion energy of stable intervals of lattice L. This quantity will be close to zero with cohesion far from bulk and close to one if all lattices have stable intervals near bulk cohesion. Moreover, we rank the dynamical stability of element i using the quantity
, where 〈EcohL〉 is the average cohesion energy of stable intervals of lattice L. This quantity will be close to zero with cohesion far from bulk and close to one if all lattices have stable intervals near bulk cohesion. Moreover, we rank the dynamical stability of element i using the quantity 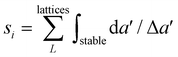 , where
, where 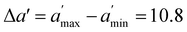 is the maximum range for stable lattices (Fig. 3). This quantity sums up all the stable intervals from all lattices and is zero for elements without any stable lattices and close to one for elements with stable lattices at all reasonable densities. Finally, we calculate the average rank for the element i as
is the maximum range for stable lattices (Fig. 3). This quantity sums up all the stable intervals from all lattices and is zero for elements without any stable lattices and close to one for elements with stable lattices at all reasonable densities. Finally, we calculate the average rank for the element i as 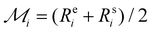 , where Rei is the rank of element i in terms of energetic stability and Rsi is the rank of element i in terms of dynamical stability. Note that our aim is to rank elements, not to create a quantitative stability measure.
, where Rei is the rank of element i in terms of energetic stability and Rsi is the rank of element i in terms of dynamical stability. Note that our aim is to rank elements, not to create a quantitative stability measure.
The heatmap of  thus emphasizes the elements’ potential for 2D stability from both energetic and dynamical viewpoints, compared to other elements (Fig. 5). The best potential is with alkali, earth alkali, and late-transition metals. Mg ranks first from both energetic and dynamic viewpoints. These trends agree with previous research.6,7,20–22,44 Groups in the middle of the transition series rank the worst, Cr being a notable exception.33 The trends also align with surface energies related to exfoliability and two-dimensional energetic stabilities.22,45 The experimentally observed Cr, Au, and Sn metallenes rank moderately among all metals,8–10,12,22,33 which implies that several more 2D metals are awaiting experimental realization.
thus emphasizes the elements’ potential for 2D stability from both energetic and dynamical viewpoints, compared to other elements (Fig. 5). The best potential is with alkali, earth alkali, and late-transition metals. Mg ranks first from both energetic and dynamic viewpoints. These trends agree with previous research.6,7,20–22,44 Groups in the middle of the transition series rank the worst, Cr being a notable exception.33 The trends also align with surface energies related to exfoliability and two-dimensional energetic stabilities.22,45 The experimentally observed Cr, Au, and Sn metallenes rank moderately among all metals,8–10,12,22,33 which implies that several more 2D metals are awaiting experimental realization.
 | ||
Fig. 5 Identifying elements with the best potential for 2D stability. Heatmap of the ranking parameter 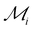 characterizing the overall 2D stability of each element; lower characterizing the overall 2D stability of each element; lower  indicates better 2D stability. indicates better 2D stability. | ||
Our results should be approached with certain limitations in mind. They have limited applicability to substrate-supported monolayers, whose dynamical stabilities are likely dominated by the substrate.46,47 A higher level of theory might influence results quantitatively, but only to a minor degree, as suggested by earlier work.37 Finally, our approach is based on investigating the lattice properties with a local viewpoint (Fig. 1a), and it does not recognize finite-size effects, effects of interfaces to the covalent template, or potential lattice stabilization by superstructures such as charge density waves;48 these effects will be investigated in forthcoming studies.
To conclude, 45 metallenes have 128 dynamically stable 2D crystalline lattices at sporadic density intervals, the extent of which can be expanded by lattice coexistence. On average, metallenes show the best stability under gentle tensile strain. Therefore, given metallenes’ ductility,15,23 experiments should approach metallenes with a paradigm that abandons fixed lattice constants and considers them as yielding membranes that can be stabilized better under gentle tension. This paradigm may also help understand certain quantitative discrepancies between theory and the experimentally observed 2D patches.8,10,33 The qualitative trends in the stability ranking of elements, which combined energetic and dynamic stability viewpoints, comply with the stability for experimentally observed metallenes (Fig. 5). At the same time, it recognizes several promising candidates, leaving plenty of room for new metallenes waiting for discovery.
Author contributions
K. Raza Abidi: investigation, validation, formal analysis, visualization, methodology (equal), funding acquisition (equal), writing – original draft; P. Koskinen: conceptualization, resources, methodology (equal), supervision, funding acquisition (equal), project administration, writing – review & editing.Data availability
The data supporting this article have been included as part of the ESI.†Conflicts of interest
There are no conflicts to declare.Acknowledgements
We acknowledge the Vilho, Yrjö, and Kalle Väisälä Foundation of the Finnish Academy of Science and Letters and the Jane and Aatos Erkko Foundation for funding (project EcoMet) and the Finnish Grid and Cloud Infrastructure (FGCI) for computational resources.References
- K. S. Novoselov, A. K. Geim, S. V. Morozov, D.-e. Jiang, Y. Zhang, S. V. Dubonos, I. V. Grigorieva and A. A. Firsov, Science, 2004, 306, 666–669 CrossRef CAS.
- A. McCreary, O. Kazakova, D. Jariwala and Z. Y. Al Balushi, 2D Mater., 2020, 8, 013001 CrossRef.
- Y. Lei, T. Zhang, Y.-C. Lin, T. Granzier-Nakajima, G. Bepete, D. A. Kowalczyk, Z. Lin, D. Zhou, T. F. Schranghamer, A. Dodda, A. Sebastian, Y. Chen, Y. Liu, G. Pourtois, T. J. Kempa, B. Schuler, M. T. Edmonds, S. Y. Quek, U. Wurstbauer, S. M. Wu, N. R. Glavin, S. Das, S. P. Dash, J. M. Redwing, J. A. Robinson and M. Terrones, ACS Nanosci. Au, 2022, 2, 450–485 CrossRef CAS.
- S. Manzeli, D. Ovchinnikov, D. Pasquier, O. V. Yazyev and A. Kis, Nat. Rev. Mater., 2017, 2, 17033 CrossRef CAS.
- V. Shanmugam, R. A. Mensah, K. Babu, S. Gawusu, A. Chanda, Y. Tu, R. E. Neisiany, M. Försth, G. Sas and O. Das, Part. Part. Syst. Charact., 2022, 39, 2200031 CrossRef.
- H. Q. Ta, R. G. Mendes, Y. Liu, X. Yang, J. Luo, A. Bachmatiuk, T. Gemming, M. Zeng, L. Fu, L. Liu and M. H. Rümmeli, Adv. Sci., 2021, 8, 2100619 CrossRef CAS.
- T. Wang, M. Park, Q. Yu, J. Zhang and Y. Yang, Mater. Today Adv., 2020, 8, 100092 CrossRef.
- J. Zhao, Q. Deng, A. Bachmatiuk, G. Sandeep, A. Popov, J. Eckert and M. H. Rümmeli, Science, 2014, 343, 1228–1232 CrossRef CAS PubMed.
- L. Zhao, H. Q. Ta, R. G. Mendes, A. Bachmatiuk and M. H. Rümmeli, Adv. Mater. Interfaces, 2020, 7, 2000436 CrossRef CAS.
- X. Yang, H. Q. Ta, W. Li, R. G. Mendes, Y. Liu, Q. Shi, S. Ullah, A. Bachmatiuk, J. Luo and L. Liu, et al. , Nano Res., 2021, 14, 747–753 CrossRef CAS.
- X. Zhao, J. Dan, J. Chen, Z. Ding, W. Zhou, K. P. Loh and S. J. Pennycook, Adv. Mater., 2018, 30, 1707281 CrossRef.
- X. Wang, C. Wang, C. Chen, H. Duan and K. Du, Nano Lett., 2019, 19, 4560–4566 CrossRef CAS PubMed.
- S. Kashiwaya, Y. Shi, J. Lu, D. G. Sangiovanni, G. Greczynski, M. Magnuson, M. Andersson, J. Rosen and L. Hultman, Nat. Synth., 2024, 3, 744–751 CrossRef.
- S. Malola, H. Häkkinen and P. Koskinen, Appl. Phys. Lett., 2009, 94, 043106 CrossRef.
- P. Koskinen and T. Korhonen, Nanoscale, 2015, 7, 10140–10145 RSC.
- P. Koskinen, H. Häkkinen, B. Huber, B. von Issendorff and M. Moseler, Phys. Rev. Lett., 2007, 98, 015701 CrossRef.
- Y. Liu, K. N. Dinh, Z. Dai and Q. Yan, ACS Mater. Lett., 2020, 2, 1148–1172 CrossRef CAS.
- C. Lu, R. Li, Z. Miao, F. Wang and Z. Zha, Chem. Soc. Rev., 2023, 52, 2833–2865 RSC.
- M. Xie, S. Tang, B. Zhang and G. Yu, Mater. Horiz., 2023, 10, 407–431 RSC.
- Y. Ma, B. Li and S. Yang, Mater. Chem. Front., 2018, 2, 456–467 RSC.
- Y. Chen, Z. Fan, Z. Zhang, W. Niu, C. Li, N. Yang, B. Chen and H. Zhang, Chem. Rev., 2018, 118, 6409–6455 CrossRef CAS PubMed.
- S. Yu, C. Zhang and H. Yang, Chem. Rev., 2023, 123, 3443–3492 CrossRef CAS.
- J. Nevalaita and P. Koskinen, Phys. Rev. B, 2018, 97, 035411 CrossRef CAS.
- Y. Ren, L. Hu, Y. Shao, Y. Hu, L. Huang and X. Shi, J. Mater. Chem. C, 2021, 9, 4554–4561 RSC.
- A. A. Sangolkar, R. Agrawal and R. Pawar, Int. J. Quantum Chem., 2022, 122, e26982 CrossRef CAS.
- J. Nevalaita and P. Koskinen, AIP Adv., 2020, 10, 065327 CrossRef CAS.
- J. Nevalaita and P. Koskinen, Nanoscale, 2019, 11, 22019–22024 RSC.
- S. Ono, Phys. Rev. Mater., 2021, 5, 104004 CrossRef CAS.
- S. Ono, Phys. Rev. B, 2020, 102, 165424 CrossRef CAS.
- J. Zhao, Q. Deng, A. Bachmatiuk, G. Sandeep, A. Popov, J. Eckert and M. H. Rümmeli, Science, 2014, 343, 1228–1232 CrossRef CAS PubMed.
- M. R. Thomsen, S. J. Brun and T. G. Pedersen, Phys. Rev. B: Condens. Matter Mater. Phys., 2015, 91, 125439 CrossRef.
- Y. Shao, R. Pang and X. Shi, J. Phys. Chem. C, 2015, 119, 22954–22960 CrossRef CAS.
- H. Q. Ta, Q. X. Yang, S. Liu, A. Bachmatiuk, R. G. Mendes, T. Gemming, Y. Liu, L. Liu, K. Tokarska and R. B. Patel, et al. , Nano Lett., 2020, 20, 4354–4361 CrossRef CAS.
- W. Kohn and L. J. Sham, Phys. Rev., 1965, 140, A1133–A1138 CrossRef.
- S. Smidstrup, T. Markussen, P. Vancraeyveld, J. Wellendorff, J. Schneider, T. Gunst, B. Verstichel, D. Stradi, P. A. Khomyakov, U. G. Vej-Hansen, M.-E. Lee, S. T. Chill, F. Rasmussen, G. Penazzi, F. Corsetti, A. Ojanperä, K. Jensen, M. L. N. Palsgaard, U. Martinez, A. Blom, M. Brandbyge and K. Stokbro, J. Phys.: Condens. Matter, 2019, 32, 015901 CrossRef.
- J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1996, 77, 3865–3868 CrossRef CAS PubMed.
- K. R. Abidi and P. Koskinen, Phys. Rev. Mater., 2022, 6, 124004 CrossRef CAS.
- I. Pallikara, P. Kayastha, J. M. Skelton and L. D. Whalley, Electron. Struct., 2022, 4, 033002 CrossRef CAS.
- B. Cordero, V. Gómez, A. E. Platero-Prats, M. Revés, J. Echeverría, E. Cremades, F. Barragán and S. Alvarez, Dalton Trans., 2008, 2832–2838 RSC.
- G. Bihlmayer, J. Sassmannshausen, A. Kubetzka, S. Blügel, K. von Bergmann and R. Wiesendanger, Phys. Rev. Lett., 2020, 124, 126401 CrossRef CAS PubMed.
- Y. Xu, B. Yan, H.-J. Zhang, J. Wang, G. Xu, P. Tang, W. Duan and S.-C. Zhang, Phys. Rev. Lett., 2013, 111, 136804 CrossRef.
- S. Sun, J.-Y. You, S. Duan, J. Gou, Y. Z. Luo, W. Lin, X. Lian, T. Jin, J. Liu, Y. Huang, Y. Wang, A. T. S. Wee, Y. P. Feng, L. Shen, J. L. Zhang, J. Chen and W. Chen, ACS Nano, 2022, 16, 1436–1443 CrossRef CAS.
- S. K. Sharma, R. Pasricha, J. Weston, T. Blanton and R. Jagannathan, ACS Appl. Mater. Interfaces, 2022, 14, 54992–55003 CrossRef CAS.
- J. Si, J. Yu, Y. Shen, M. Zeng and L. Fu, Small Struct., 2021, 2, 2000101 CrossRef CAS.
- J.-Y. Lee, M. Punkkinen, S. Schönecker, Z. Nabi, K. Kádas, V. Zólyomi, Y. Koo, Q.-M. Hu, R. Ahuja and B. Johansson, et al. , Surf. Sci., 2018, 674, 51–68 CrossRef CAS.
- F. Yin, J. Akola, P. Koskinen, M. Manninen and R. E. Palmer, Phys. Rev. Lett., 2009, 102, 106102 CrossRef CAS PubMed.
- F. Yin, P. Koskinen, S. Kulju, J. Akola and R. E. Palmer, Sci. Rep., 2015, 5, 8276 CrossRef CAS PubMed.
- S. Chowdhury, A. F. Rigosi, H. M. Hill, P. Vora, A. R. Hight Walker and F. Tavazza, Nanomaterials, 2022, 12, 504 CrossRef CAS.
Footnote |
| † Electronic supplementary information (ESI) available. See DOI: https://doi.org/10.1039/d4nr03266g |
| This journal is © The Royal Society of Chemistry 2024 |