Open Access Article
Open Access ArticleEfficient energy and memory storage capabilities in optimized BiFeO3/MnMoO4/NiFe2O4 triphasic composites for futuristic multistate devices
Izhar Sagheer *a,
Muhammad Tamoor Ansarb,
Shahid M. Ramayc,
Houbing Huangde and
Shahid Atiq
*a,
Muhammad Tamoor Ansarb,
Shahid M. Ramayc,
Houbing Huangde and
Shahid Atiq *a
*a
aCentre of Excellence in Solid State Physics, University of the Punjab, Lahore 54590, Pakistan. E-mail: izhar.phd.cssp@pu.edu.pk; satiq.cssp@pu.edu.pk
bSchool of Engineering and Built Environment, Griffith University, Parklands Drive, Gold Coast, Queensland 4222, Australia
cPhysics and Astronomy Department, King Saud University, Riyadh, Saudi Arabia
dSchool of Materials Science and Engineering, Beijing Institute of Technology, Beijing 100081, China
eAdvanced Research Institute of Multidisciplinary Science, Beijing Institute of Technology, Beijing 100081, China
First published on 16th September 2024
Abstract
The emergence of multiferroic materials particularly bismuth iron oxide (BiFeO3) with distinctive magnetoelectric, and high energy storage capabilities, present pivotal aspects for next-generation memory storage devices. However, intrinsically weak magnetoelectric coupling limits their widespread applications, that can be leap over by the integration of BiFeO3 with enriched ferroelectric, and ferro/ferrimagnetic materials. Here, a series (1 − x)[0.7BiFeO3 + 0.3MnMoO4] + xNiFe2O4 (x = 0.00, 0.03, 0.06, and 0.09) is synthesized via citrate-gel based self-ignition, and solid-state reaction routes. Phase purity and crystallinity of tri-phase composites with surfaces revealing random and arbitrarily shaped grains are assured by X-ray diffraction, and field emission scanning electron microscopy, respectively. Dielectric studies illustrated non-linear trend for broad range of frequencies as predicted by Maxwell–Wagner theory along with single semicircle arcs in Nyquist plots that exposes grain boundaries effect. An enriched 68.42% of ferroelectric efficiency is featured for x = 0.06 substitutional contents, while magnetic computations demonstrated improved saturation magnetization (Ms), remanence magnetization (Mr), and coercive applied magnetic field (Hc) values as 5.87 emu g−1, 0.96 emu g−1, and 215.19 Oe, respectively for x = 0.09 phase-fraction. The intriguing linear trends of magnetoelectric coupling for all the compositions are corroborating them propitious contenders for futuristic multistate devices.
1. Introduction
Multiferroic (MF) single phase ceramics have attracted noteworthy attention owing to their extraordinary electric properties and the coexistence of one or more distinct ferroic orders (ferroelectricity (FE), ferromagnetism (FM), ferroelasticity, and ferrotoroidicity).1 Miniaturization of MF-based low power and advanced multifunctional devices are the need of the hour as such devices can afford viable utilization in clean energy storage, water purification and medical treatment applications.2–4 Among multiple-ferroic order materials, magnetoelectric (M–E) materials have combinations of ferroelectric and ferromagnetic orders that can be switched by the applied electric as well by the magnetic field. That's why, these materials make imperative part of multifunctional devices e.g., energy storage, spintronics, transducers and sensors. In addition, coupling between electric and magnetic orders is far important to design four logic state storage devices which is the future of memory storage devices.5 However, the coupling must be strong enough to have well controlled domains by corresponding as well noncorresponding field.Amongst all MF materials, BiFeO3 (BFO) has been declared as the only known significant material to have MF property even at room temperature (RT) with 640 K and 1100 K as Néel temperature (TN) and ferroelectric Curie temperature (Tc), respectively,6 exhibiting rhombohedral distorted cubic perovskite structure with general formula ABO3.7 Its FE trend is majorly due to lone pairs or distortion in bonds that produces relatively weak coupling between both orders; thus, outcome in lower ferroelectricity, high leakage current, weak remanent polarization and magnetization drawbacks. To overcome these shortcomings, MF-based manganese molybdenum tetra oxide MnMoO4 (MMO) having monoclinic type structure is preferred that can effectively upsurge the FE order of the BFO.8 MMO is a mesoporous material as well, hence, it is very suitable to introduce well-porous ability in composites for potential storage applications.9 However, the magnetic property is optimized by the substitution of spinel-phased NiFe2O4 (NFO). Spinel ferrites have emerged as the most suitable magnet phases to be used in tri-phasic MF composites. These ferrites possess general formula AB2O4, where A is a divalent cation while B is a trivalent cation. In the present case, Fe3+ is trivalent cation (B), while Ni2+(A) is a divalent cation. Spinel ferrites are selected due to their exceptional properties like high saturation magnetization (Ms) values, low eddy current losses, large magneto-crystalline anisotropy, high Tc, excellent squareness ratio, low coercivity (Hc) values, mechanical hardness and high electric resistivity. In our case, NFO was preferred due to its high resistivity, exceptional permeability and enriched Ms values.10
In 2019, Khan et al., substituted NFO to BaTiO3 synthesized by sol–gel auto-combustion mechanism and found reduction in band gap with increase in NFO concentrations.11 Mn-doped MF-composites were developed by sol–gel self-ignition approach to have magnetically softened and very low coercive materials by Dhanalakshmi et al.12 In another work, increasing contents of spinel ferrites resulted into great impact on BFO ME-coupling to face BFO challenge of low antiferromagnetic order by preparing the samples via reliable synthesis i.e., sol–gel auto-combustion process by Umair and his co-workers.13 In another study, Ali and his group synthesized tri-phase composites by citrate-gel self-combustion approach followed by solid-state mechanism with tunable FE and magnetization for ultra-sensitive devices along with their fast switching and excellent ME-couplings.14 Augustine and co-workers also synthesized two phase composites of BFO and spinel ferrites by citrate-gel auto-ignition route and achieved significantly improved coupling of magnetic and ferroelectric orders.15 Interestingly, BFO-PVDF-CB (carbon black) composites with various weight percentages of CB sanctioned high dielectric constant with suppressed dielectric losses by synthesizing via solution casting technique by Moharana et al.16 Two phases of BFO and PZT (lead zirconate titanate) were dispersed in various weights of PVDF (poly vinylidene fluoride) to have a tri-phase composite by sol–gel auto-combustion and ball-milling methods that presented decreasing polarization while coercivity was found to increase. In addition, ME-coupling was also significantly improved by Mehak et al.17 Unique kinds of composites were synthesized by Sreekanth et al., using cost effective sol–gel self-ignition method, those were nano as well bulk composites. Compellingly, both samples showed superparamagnetic behavior at RT with coercivities close to zero.18 In March 2023, tri-phasic BFO–BaTiO3–MnFe2O4 composites were orchestrated by urea-glycine based self-ignited sol–gel mechanism for highly efficient and strong magnetoelectric coupling based ultrasensitive devices.19 In all above works, porosity and ME couplings were not targeted to enhance simultaneously.
Considering deficiencies in previous efforts, in this project; shortcomings are tried to overcome. Hence, this work emphasis on the synthesis of two phases i.e., BFO and NFO by citrate-gel followed by auto-ignition process while third MMO phase is prepared by solid-state mechanisms and then composite series (1 − x)[0.7BFO + 0.3MMO] + xNFO with x = 0.00, 0.03, 0.06, and 0.09 substitutional contents is routed through solid-state approach. In studied literature, all the researchers reported their individual efforts for electric polarization and magnetic responses while current study summarizes couplings of three phases for practical applications in energy storage and generation as well in ultra-fast switching memory devices.
2. Experimental
The targeted series (1 − x)[0.7BFO + 0.3MMO] + xNFO with x = 0.00, 0.03, 0.06, and 0.09 substitutional contents are synthesized by different mechanisms as elaborated in Fig. 1(a–c). For better crystallinity outcomes, BFO and NFO were prepared by cost-effective and bottom-up citrate-gel auto-ignition process as depicted in Fig. 1(a). For this purpose, nitrate precursors were preferred due to their good solubility in deionized (DI) water,20 hence, stoichiometric quantities of bismuth nitrate pentahydrate [Bi(NO3)3·5H2O], iron nitrate nonahydrate [Fe(NO3)3·9H2O] and nickel nitrate hexahydrate [Ni(NO3)2·6H2O] with high purity ≥99% (purchased from Sigma Aldrich) were consumed for specimen synthesis. Initially, relative proportions of [Bi(NO3)3·5H2O] and [Fe(NO3)3·9H2O] were dissolved in DI water to make homogenous solutions. Small amount of HNO3 was added to [Bi(NO3)3·5H2O] solution for better solubility.21 These two solutions were mixed in a single large beaker, afterwards, fueling agents including urea (CH4N2O) and glycine (C2H5NO2) (with 1.5![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1, respectively) were poured to metal nitrates. Then, the beaker was positioned on a hot plate with magnetic stirrer inside the beaker for smooth stirring of the solutions. Similar procedure was adopted with [Ni(NO3)2·6H2O] and [Fe(NO3)3·9H2O] without addition of HNO3. Both solutions formed gel-like stuff after being heated at 100 °C for 1 h along with stirring. After gel formation, temperature was increased gradually with time interval of 3 min and 25 °C upsurge in temperature until auto-combustion occurred. The solution with BFO contents got combustion at 275 °C solution whilst with NFO concentrations got ignition at 250 °C. Additional heat treatments transformed them to dry ashes finally. Conversely, owing to the insolubility of molybdenum oxides in water, solid-state route is preferred for the synthesis of MMO in which manganese nitrate tetrahydrate [Mn(NO3)2·4H2O] and molybdenum trioxide [MoO3] were utilized as precursors.22 Consistent with their stoichiometric ratios, the as-mentioned precursors were placed in a ball-mill machine and milled for 3 h and 30 min for each with a time span of 30 min as illustrated in Fig. 1(b).
1, respectively) were poured to metal nitrates. Then, the beaker was positioned on a hot plate with magnetic stirrer inside the beaker for smooth stirring of the solutions. Similar procedure was adopted with [Ni(NO3)2·6H2O] and [Fe(NO3)3·9H2O] without addition of HNO3. Both solutions formed gel-like stuff after being heated at 100 °C for 1 h along with stirring. After gel formation, temperature was increased gradually with time interval of 3 min and 25 °C upsurge in temperature until auto-combustion occurred. The solution with BFO contents got combustion at 275 °C solution whilst with NFO concentrations got ignition at 250 °C. Additional heat treatments transformed them to dry ashes finally. Conversely, owing to the insolubility of molybdenum oxides in water, solid-state route is preferred for the synthesis of MMO in which manganese nitrate tetrahydrate [Mn(NO3)2·4H2O] and molybdenum trioxide [MoO3] were utilized as precursors.22 Consistent with their stoichiometric ratios, the as-mentioned precursors were placed in a ball-mill machine and milled for 3 h and 30 min for each with a time span of 30 min as illustrated in Fig. 1(b).
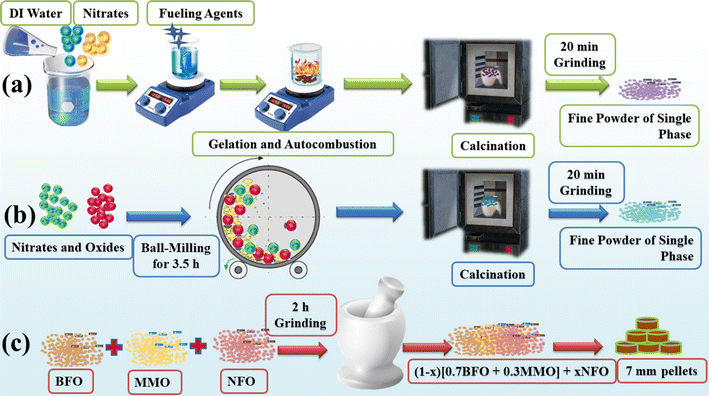 | ||
| Fig. 1 Schematics sample synthesis of (a) BFO and NFO by citrate-gel self-ignition approach, (b) MMO by solid-state route, and (c) composite preparation by grinding process. | ||
Afterwards, BFO, MMO and NFO specimen were positioned in ceramic crucibles, and then, crucibles were placed in a box furnace and calcined separately for 3 h at 550 °C, 800 °C, and 800 °C temperatures, respectively.23 Calcination process developed the desired crystal structures of the constituent phases. Subsequently, three samples were ground separately for 20 min each to get the fine powders. Then, these phases were mixed together consistent with the composition ratios as x = 0.00, 0.03, 0.06, and 0.09 in (1 − x)[0.7BFO + 0.3MMO] + xNFO series, by separately grinding the composites for 2 h of each using mortar and pestle as demonstrated in Fig. 1(c). Griding was done slowly, so that particle structure or shape would not be affected.24 Fine powders of composites were pressed by apex hydraulic press with the force of 30 kN to make the uniform pellets of 7 mm in diameter and 1.1 mm of thicknesses because most analyses are performed in pellets form. The dimensions of pellets were measured using vernier caliper and measure scale (FA/JA series) respectively, for all the as-synthesized concentrations.
Crystal structures of the as-formed composites were determined by X-ray Diffractometer (XRD) analysis, and to do so, Powder XRD, Equinox 2000, Thermo Fisher Scientific USA was employed. Surface features were probed using field emission scanning electron microscopy (FESEM) via America ixrf (model # 550i) with 200![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 00× magnification and Java scripted ImageJ software was used to perceive the particles shapes, voids and size distribution. Exact stoichiometric ratios of the elements in various composites were measured by energy dispersive X-ray spectroscopy (EDX) and in addition; elemental mapping of the separate constituent elements was also done using EDX. Dielectric, impedance spectroscopic, ac-conductivity (σac) and electric modulus analysis were conducted by 6500B Wayne Kerr impedance analyzer that examines the material's response to sinusoidally varying electric field (EF).25 Pellets were positioned between two copper electrodes and frequency dependent (20 Hz–20 MHz) series and parallel capacitance, resistance, reactance and impedance responses of the materials were recorded by applying EF.
00× magnification and Java scripted ImageJ software was used to perceive the particles shapes, voids and size distribution. Exact stoichiometric ratios of the elements in various composites were measured by energy dispersive X-ray spectroscopy (EDX) and in addition; elemental mapping of the separate constituent elements was also done using EDX. Dielectric, impedance spectroscopic, ac-conductivity (σac) and electric modulus analysis were conducted by 6500B Wayne Kerr impedance analyzer that examines the material's response to sinusoidally varying electric field (EF).25 Pellets were positioned between two copper electrodes and frequency dependent (20 Hz–20 MHz) series and parallel capacitance, resistance, reactance and impedance responses of the materials were recorded by applying EF.
Being a complex quantity, real (M′) and imaginary (M′′) parts of electric modulus were determined by following relations.26
| M′ = ε′/(ε′ + ε′′)2 | (1) |
| M′′ = ε′′/(ε′ + ε′′)2 | (2) |
Highly advanced and accurate DXV-9000 series vibrating sample magnetometer (VSM) was operated to get magnetic responses of the materials to the applied magnetic field.
Multiferroic analyzer (Radiant's Tech. USA) was employed for specific ferroelectric as well magnetoelectric characterizations like polarization–electric field (PE) loops and M–E coupling effects in tri-phase composites. Voltage was kept in between 10 to 80 V. The scrutinized results and then their precise calculations are mentioned in results and discussion.
3. Results and discussion
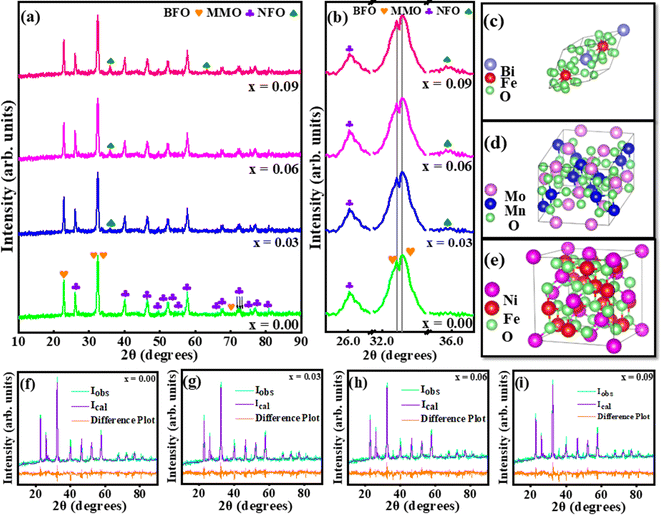
The impressions of atomic arrangements i.e., crystalline structures within the series (1 − x)[0.7BFO + 0.3MMO] + xNFO with x = 0.00, 0.03, 0.06, and 0.09 phase-fractions were determined using Cu-Kα radiations having 1.5406 Å of wavelength. The detector recorded the XRD patterns within 2θ range of 10°–90° and the relative peaks positioned at various angular positions were indexed by the analytical approach proposed by B. D. Cullity.27 The indexed XRD patterns are profiled in Fig. 2(a) in which the intense peaks attributed to BFO phase with lattice planes (100), (110), (1![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) and (2
0) and (2![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 1) traced at 22.81°, 32.33°, 32.45° and 71.81° angular positions, respectively. These indexed BFO patterns were perceived to be promising with ICSD reference # 01-072-2035 that confirmed the development of rhombohedral perovskite structure having R3c space group. Fig. 2(b) depicts the emergence of twin peaks of BFO at (110) and (1
1) traced at 22.81°, 32.33°, 32.45° and 71.81° angular positions, respectively. These indexed BFO patterns were perceived to be promising with ICSD reference # 01-072-2035 that confirmed the development of rhombohedral perovskite structure having R3c space group. Fig. 2(b) depicts the emergence of twin peaks of BFO at (110) and (1![[1 with combining macron]](https://www.rsc.org/images/entities/char_0031_0304.gif) 0) crystal planes which confirmed the existence of high crystallinity of BFO phase. Similarly, the peaks emerged at 2θ = 26.02°, 40.10°, 46.38°, 49.67°, 52.14°, 52.38°, 57.16°, 57.34°, 67.34°, 67.86°, 71.96°, 72.14°, 72.57°, 75.59°, 77.03°, and 80.82° corresponding to (002), (041), (042), (
0) crystal planes which confirmed the existence of high crystallinity of BFO phase. Similarly, the peaks emerged at 2θ = 26.02°, 40.10°, 46.38°, 49.67°, 52.14°, 52.38°, 57.16°, 57.34°, 67.34°, 67.86°, 71.96°, 72.14°, 72.57°, 75.59°, 77.03°, and 80.82° corresponding to (002), (041), (042), (![[4 with combining macron]](https://www.rsc.org/images/entities/char_0034_0304.gif) 23), (133), (
23), (133), (![[5 with combining macron]](https://www.rsc.org/images/entities/char_0035_0304.gif) 31), (024), (114), (
31), (024), (114), (![[7 with combining macron]](https://www.rsc.org/images/entities/char_0037_0304.gif) 13), (550), (
13), (550), (![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 63), (622), (063), (
63), (622), (063), (![[8 with combining macron]](https://www.rsc.org/images/entities/char_0038_0304.gif) 22), (154), and (
22), (154), and (![[2 with combining macron]](https://www.rsc.org/images/entities/char_0032_0304.gif) 06) Bragg's planes, respectively that confirmed the MMO formation. The indexed peaks were in agreement with ICSD reference # 01-072-0285 illustrated the existence of monoclinic crystal structure with C2/m space group. In addition, indexed peaks at 35.88° and 63.34° angular positions attributed to (311) and (440) lattice planes, respectively that assured the existence of NFO cubic spinel structure in the specimen for x > 0.00 substitutional contents and matched with ICSD reference # 01-074-2081 with space group Fd3m. The sample with x = 0.00 concentration is comprising of BFO and MMO phases while with x > 0.00 phase-fractions consists of tri-phases as evident by XRD patterns in which peaks of NFO became intensified by increasing NFO concentrations, demonstrating the increased crystallinity. Interestingly, peaks of BFO and MMO are not affected appreciably by the increasing concentrations and no extra peaks were appeared promising the existence of pure phases of the specimen.28
06) Bragg's planes, respectively that confirmed the MMO formation. The indexed peaks were in agreement with ICSD reference # 01-072-0285 illustrated the existence of monoclinic crystal structure with C2/m space group. In addition, indexed peaks at 35.88° and 63.34° angular positions attributed to (311) and (440) lattice planes, respectively that assured the existence of NFO cubic spinel structure in the specimen for x > 0.00 substitutional contents and matched with ICSD reference # 01-074-2081 with space group Fd3m. The sample with x = 0.00 concentration is comprising of BFO and MMO phases while with x > 0.00 phase-fractions consists of tri-phases as evident by XRD patterns in which peaks of NFO became intensified by increasing NFO concentrations, demonstrating the increased crystallinity. Interestingly, peaks of BFO and MMO are not affected appreciably by the increasing concentrations and no extra peaks were appeared promising the existence of pure phases of the specimen.28
The Scherrer's approach was used to determine the crystallite sizes (Dhkl) for each phase in all the samples. Dhkl for BFO and MMO depicts minute variations for different phase-fractions, however, NFO behaved in a unique way for its relevant substitutional contents. Herein, Dhkl values for NFO is largest for x = 0.03 substitutions and approximately same for other contents (x = 0.06, and 0.09). Scherrer's formula is given as,29
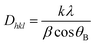 | (3) |
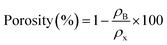 | (4) |
Table 1 enlists the values of lattice parameters (a, b and c), Dhkl, ρB, ρx and porosities (%) relative to different concentrations (x = 0.00, 0.03, 0.06, and 0.09 phase-fractions). Maximum porosity is evident for x = 0.06 concentration specifying its applications in sound absorption, heat insulation and energy storage, and mechanical-to-electrical energy conversion devices,31 as the greater active area of the material offers more place to store charges. Using determined parameters; crystal structures of BFO, MMO and NFO were designed using Vesta software and are revealed in Fig. 2(c)–(e), respectively.
| NFO contents (x) | Lattice constants (Å) | Dhkl (nm) | ρB (g cm−3) | ρx (g cm−3) | Porosity (%) ± 2% | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| BFO | MMO | NFO | BFO | MMO | NFO | ||||||
| a = b = c | a | b | c | a = b = c | |||||||
| 0.00 | 3.96 | 10.47 | 9.52 | 7.14 | Nil | 31 | 25 | — | 3.97 | 7.11 | 44 |
| 0.03 | 3.96 | 10.47 | 9.52 | 7.14 | 8.30 | 32 | 24 | 92 | 4.11 | 7.05 | 42 |
| 0.06 | 3.96 | 10.47 | 9.52 | 7.14 | 8.30 | 32 | 25 | 74 | 3.18 | 7.00 | 54 |
| 0.09 | 3.96 | 10.47 | 9.52 | 7.14 | 8.30 | 32 | 24 | 76 | 4.03 | 6.95 | 42 |
To further depict the purity of composites, Rietveld's refinement analysis was carried out using X'Pert HighScore Plus software. All the involved R-factors like R-profile (Rp), weighted R-profile (Rwp) and R-expected (Rexp) were determined using the as-mentioned software. The most important factor χ2 = Rwp/Rexp (goodness of fit) was found with its best value. The lowest values of χ2 unveiled that our experimental consequences are in accordance with calculated values i.e., successful development of composite phases and all the determined Rietveld's refined factors are computed in Table 2. This can also be observed by the graphical representation of Rietveld's refinement shown in Fig. 2(f–i) for all the compositions that clearly demonstrates excellent matching of experimental and theoretical data. Minute differences in the peaks of experimental and theoretical densities are due to existence of defects or vacancies in the specimen.
| NFO contents (x) | 0.00 | 0.03 | 0.06 | 0.09 |
| Rexp | 20.0 | 19.64 | 18.72 | 18.45 |
| Rp | 6.93 | 7.06 | 6.48 | 6.25 |
| Rwp | 8.83 | 8.94 | 8.27 | 8.14 |
| Dstatistics | 0.21 | 0.48 | 0.46 | 0.40 |
| W.Dstatistics | 0.21 | 0.53 | 0.50 | 0.41 |
| χ2 | 0.19 | 0.21 | 0.20 | 0.19 |
Morphological features of the concerning series (1 − x)[0.7BFO + 0.3MMO] + xNFO with x = 0.00, 0.03, 0.06, and 0.09 substitutional contents was achieved by FESEM and illustrated in Fig. 3(a–d). Overall, in these micrographs, surfaces were depicted to be very smooth as pellets were used for scanning and also agglomeration could be perceived due to high pressure while making pellets and magnetic nature of NFO-contents. Various shapes of the grains like rectangular, cubical and irregular are present and grain sizes were averagely ranging between 20–130 nm and are demonstrated by histograms as well. All these variations can be modified by heat treatment conditions while synthesizing the samples. Actually, changing grain and crystallite sizes affect the mean free path of electrons so resistivity is changed by this mechanism to improve our material for certain practical needs.
The existence of various elements is keenly analyzed by elemental mapping that is carried out for x = 0.03 and 0.09 substitutions as revealed in Fig. 3(e) and (f), respectively. Colored areas are indicating the presence of an element in that particular area, while dark areas characterize the absence of that particular element. The colored images are attributing various detected X-rays associated with Bi, Fe, Mn, Mo, and Ni.32 Plots in Fig. 3(g) depicts the relative intensities of X-rays emitted by the elements present in our samples, thus giving the existence of proper stoichiometric ratios of the constituent elements. There are minor changes in the peaks of Bi and Fe for all samples. Ni peaks are hardly observed due to such a small concentration substituted. To further explore the voids depicted in the specimen that directly verify the measured porosity from the pellets are sketched in Fig. 3(h).
Dielectrics are expected to have no free electrons ideally to perform polarization operation under the action of EF and dipoles switching are responsible for storing electrical energy. In fact, polarization is directly affected by temperature and frequency of varying applied EF as well. Hence, real (ε′) and imaginary (ε′′) parts of dielectrics are determined using ε' = Cpd/Aεo and ε′′ = ε′![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) tan
tan![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) δ, where tan
δ, where tan![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) δ is tangent loss determined by tan
δ is tangent loss determined by tan![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) δ = 1/(2πfCR). The variations in ε′ and ε′′ versus log of frequency (log
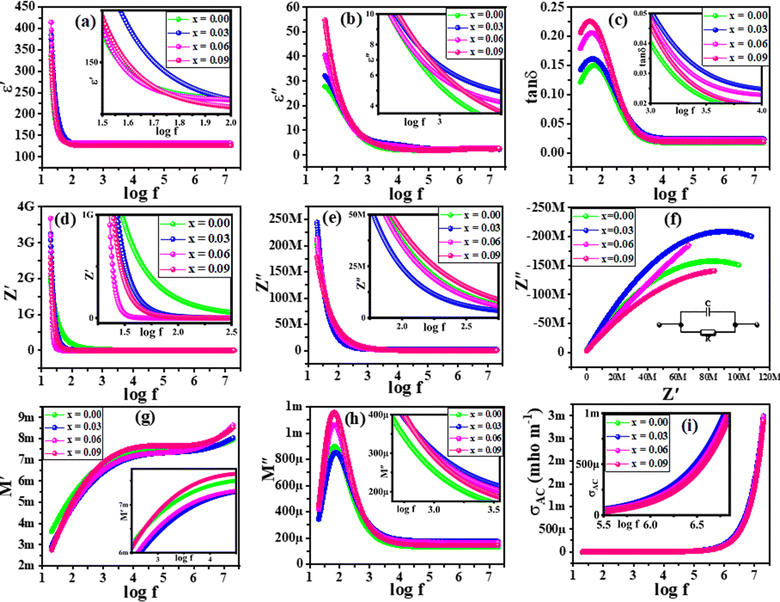
δ = 1/(2πfCR). The variations in ε′ and ε′′ versus log of frequency (log![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) f) for the series (1 − x)[0.7BFO + 0.3MMO] + xNFO (with x = 0.00, 0.03, 0.06, and 0.09 substitutional contents) are illustrated in Fig. 4(a) and (b), respectively. For low frequency region, high ε′ as well as high ε′′ trend was perceived, whilst trend changes gradually to lowest constant values in high frequency ranges. This behavior is usually associated with ferrites as they response in such a way to extremely fast fluctuating EF.33
f) for the series (1 − x)[0.7BFO + 0.3MMO] + xNFO (with x = 0.00, 0.03, 0.06, and 0.09 substitutional contents) are illustrated in Fig. 4(a) and (b), respectively. For low frequency region, high ε′ as well as high ε′′ trend was perceived, whilst trend changes gradually to lowest constant values in high frequency ranges. This behavior is usually associated with ferrites as they response in such a way to extremely fast fluctuating EF.33
In real, low frequency is very suitable for all kind of polarizations (electronic, atomic, space charge and orientational) to occur that also support each other, however; going towards high frequency domains, electronic and atomic polarizations start to takeover other two polarizations because it is not possible for space charge and orientational polarizations to response rapidly with varying EF. In all kind of polarizations, response of material is measured by relaxation time τ = 1/(2πfc) that is the time in which the dipole switches in the retort of applied EF. Interestingly, Koop's theory as well as Maxwell–Wagner model support such a fluctuating behavior. According to Maxwell–Wagner model, conduction between conducting grains is prohibited by insulating grain boundaries. Polarization occurs by self-orientation of charges along the boundaries and results into upsurge of dielectric parameters. Charges follow the fluctuating EF but as the frequency increases; it become more difficult for charges to trail the applied field; hence, dielectric parameters reduce to lower values. Essentially, electrons and holes approach the grain boundaries by hopping phenomenon and these observations are also accredited to substitution of NFO contents. As the concentration is increased, the probability of hopping through grain boundaries increases; consequently, for greater NFO concentration, enriched dielectric parameters were perceived. Energy is stored in the material due to its response to applied EF and when that energy is taken back, small part of that is lost as heat which is declared as tangent loss (tan![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) δ). Empirically, charge hopping and crystal defects are reasons for such heat losses and are plotted in Fig. 4(c). In fact, this plot is in very good agreement with previous plots of Fig. 4(a) and (b), as in low frequency region; due to very active response of grain boundaries, loss is highest according to Maxwell–Wagner model as well as to Koop's theory. However, in high frequency region; energy is lost due to delay in the orientational changes for conduction phenomenon of locally bound charges.
δ). Empirically, charge hopping and crystal defects are reasons for such heat losses and are plotted in Fig. 4(c). In fact, this plot is in very good agreement with previous plots of Fig. 4(a) and (b), as in low frequency region; due to very active response of grain boundaries, loss is highest according to Maxwell–Wagner model as well as to Koop's theory. However, in high frequency region; energy is lost due to delay in the orientational changes for conduction phenomenon of locally bound charges.
To further evaluate the grains and grain boundaries effect, complex impedance (Z*) tool is utilized (Z* = Z′ + iZ′′). This analysis makes a connection between the microstructure and impedance responses, and accordingly, electrical effects of impurities are also envisioned. It exhibits real (Z′) and imaginary (Z′′) parts.
Fig. 4(d) and (e) are depicting the variations in resistive (Z′) and reactive (Z′′) responses versus log![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) f, respectively for all the specimen. The compositions are attributing declining trend as frequency changes from low to high regime because grain boundaries are most influential; hence, high impedances at low frequency regions were affirmed. In addition, enrichment in concentrations of NFO introduce additional charge carriers in the specimen that take part in the conduction mechanism; accordingly, resistivity decreases and likewise, Z′′ follows the identical trend.
f, respectively for all the specimen. The compositions are attributing declining trend as frequency changes from low to high regime because grain boundaries are most influential; hence, high impedances at low frequency regions were affirmed. In addition, enrichment in concentrations of NFO introduce additional charge carriers in the specimen that take part in the conduction mechanism; accordingly, resistivity decreases and likewise, Z′′ follows the identical trend.
Characterization of various phases of samples is done by drawing Z′ against Z′′ i.e., Nyquist plots and are profiled in Fig. 4(f). Typically, this kind of plot consists of three semi-circular arcs, where first one being contributed by grain boundaries, second one by grains, while the third one denotes the electrode effects. Actually, such semicircles are observed if and only if, bulk material has lower resistance as compared to grain boundaries.26 Generally, this happens due to the maximum resistance at low frequency due to carrier at grain boundaries and less resistance at high frequency is credited by inner grains. In present case, only one semicircle was observed which is indicating grains boundaries effect and single relaxation phenomenon. Further, increased NFO-contents led to decrease the semicircular arc accrediting enriched conductivity due to additional charge carriers. Every semicircle in Nyquist plot can be described by a parallel RC-circuit as shown in the inset of Fig. 4(f). Resistances (R) can be estimated from the intercept of the plots on horizontal axis (Z′), while capacitances (C) can be calculated from using, 2πfmaxRC = 1. However, relaxation time (τ) can be determined from the relaxation frequencies (peaks of the arcs) using the relation as; τ = RC.
Complex dielectric modulus and ac-conductivity (σac) examinations were employed to further investigate the materials for Debye-type relaxation phenomenon. As compared to impedance spectroscopy, complex modulus spectroscopy is more fruitful to distinguish grain boundaries effect and electric polarization. M′ and M′′ are calculated using eqn (1) and (2) and corresponding plots are displayed in Fig. 4(g) and (h), respectively. M′ has a constant response of a vast range of frequencies, while M′′ has a resonance peak at 100 Hz, which is due to relaxation phenomenon, afterwards, M′′ starts to decline which is pointing to non-Debye relaxation behavior. In fact, M′ and M′′ are related to ε′ and ε′′, so it gives comparative energy stored and losses, respectively.
As the conduction is based upon the movement of charge carriers, so electron hopping, space charge distribution, grain boundaries and charge transitions are the main causes for this phenomenon. Total conductivity is summed up as; σ = σdc + σac. Where, σac is determined using relation, σac = 2πfεoε′![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) tan
tan![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) δ. Here, σdc is dc-conductivity. Changes in σac versus log
δ. Here, σdc is dc-conductivity. Changes in σac versus log![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) f for all the specimen are sketched in Fig. 4(i). Moving from low to high frequency region, σac remains almost constant; which depicts the frequency-independent behavior (concerning to σdc). Whilst, in highest frequency regime; increasing trend is perceived due to contribution of free as well as of bound charges which boosts up the conductivity.
f for all the specimen are sketched in Fig. 4(i). Moving from low to high frequency region, σac remains almost constant; which depicts the frequency-independent behavior (concerning to σdc). Whilst, in highest frequency regime; increasing trend is perceived due to contribution of free as well as of bound charges which boosts up the conductivity.
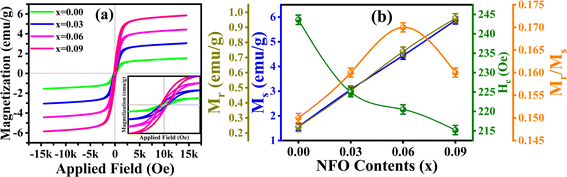
To analyze magnetic responses of all the samples, M–H (hysteresis) loops were achieved at ±15 kOe (sketched in Fig. 5(a)), and characteristics like Ms, Mr, Hc, and Mr/Ms were perceived. Deep insight of the plots reveals that all the composites have some spontaneous magnetizations i.e., ferrimagnetism. Graph analysis gives Ms, Mr and Hc, values as 1.56 emu g−1, 0.24 emu g−1 and 243.64 Oe, respectively for x = 0.00 phase-contents. However, with the introduction of NFO (x = 0.03, 0.06, and 0.09); Ms and Mr increased to 5.87 emu g−1 and 0.96 emu g−1, respectively. It may be due to asymmetrical spins present in spinel ferrites which causes enhancement in spontaneous magnetization, while Hc was observed to decrease to 215.19 Oe for highest substituted sample. Exchange coupling interaction of perovskite BFO and spinel NFO is responsible for specific magnetic domains which are in turn responsible for enhancement of ME coupling in composites. The variations in all the computed parameters are profiled in Fig. 5(b) and summarized in Table 3. Further, Mr/Ms values also specifies the ferrimagnetic behavior, however, this ratio is very low and remained approximately same for all the samples.
| NFO contents (x) | Ms (emu g−1) ± 1% | Mr (emu g−1) ± 1% | Hc (Oe) ± 0.1% | Mr/Ms |
|---|---|---|---|---|
| 0.00 | 1.56 | 0.24 | 243.64 | 0.15 |
| 0.03 | 3.06 | 0.48 | 224.97 | 0.16 |
| 0.06 | 4.44 | 0.74 | 220.52 | 0.17 |
| 0.09 | 5.87 | 0.96 | 215.19 | 0.16 |
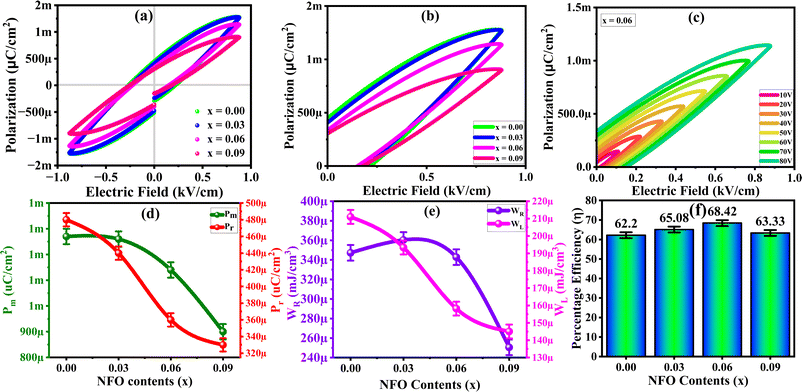
Ferroelectric materials retain their spontaneous polarization even after the removal of applied electric fields and can switch their polarization under the reversible electric field. The depicted ferroelectric trend characterizes intrinsic properties of the materials and such specimen are probable to revolutionize the energy storage world. Here, ferroelectric behavior of tri-phase composites is investigated by drawing polarization–electric field (P–E) loops under ∼900 V cm−1 of electric field strength and are presented graphically in Fig. 6(a), where all the samples responded unsaturated linear trends under the applied field. P–E loops are not closed like magnetic loops, wherein, defect dipoles, polarizability due to ions, grain size, and doping causes leakage current are the main causes for unclosed loops.34 The dipoles/polarization switching trends (charging/discharging curves) under the variation of applied fields for all the samples in 1st quadrant are displayed in Fig. 6(b) that are further used for the determination of saturation/maximum polarization (Pm), and remanence polarization (Pr). However, coercive electric field (Ec) is also determined for all the specimens. For x = 0.00 content, Pm, Pr, and Ec were recorded from the plot as 0.00127 μC cm−2, 0.00048 μC cm−2, and 0.241 kV cm−1, respectively. The inclusion of NFO contents lead to decrease the Pm, Pr and Ec values and for maximum NFO substitution these parameters were computed as 0.00090 μC cm−2, 0.00033 μC cm−2, and 0.216 kV cm−1, respectively. NFO bearing strong magnetic properties, is the cause of reducing trend for ferroelectric parameters. P–E curves for x = 0.06 substitutional contents under different applied fields are illustrated in Fig. 6(c), while, the variations in PM and PR parameters are also revealed graphically in Fig. 6(d). The application of electric field switches electric domains within the material that signifies the energy storage capability. By removing the field, this energy can be recovered and declared as recoverable energy density (WR). On the other hand, all the stored energy is not recovered completely and lost (WL) due to frictional losses and electric domain walls motion. Variation in WR and WL is shown graphically in Fig. 6(e). In addition, the sum of both energy densities (WR and WL) is depicted as total energy density (WT). In this work, Fig. 6(f) declared sample with x = 0.06 phase-fractions as the highest efficient tri-phase composition. The behavior depicted in the Fig. 6(a–f) are pointing towards suitability of the materials for energy as well as data storage devices and all the computed ferroelectric parameters are summarized in Table 4.
| NFO contents (x) | Ferroelectric parameters | ||||||
|---|---|---|---|---|---|---|---|
| Pm (μC cm−2) ± 2% | Pr (μC cm−2) ± 2% | Ec (kV cm−1) ± 2% | WT (mJ cm−3) ± 2% | WR (mJ cm−3) ± 2% | WL (mJ cm−3) ± 2% | Percentage efficiency ± 2% | |
| 0.00 | 0.00127 | 0.00048 | 0.241 | 0.000558 | 0.000347 | 0.000211 | 62.20 |
| 0.03 | 0.00126 | 0.00044 | 0.217 | 0.000553 | 0.000360 | 0.000193 | 65.08 |
| 0.06 | 0.00114 | 0.00036 | 0.189 | 0.000501 | 0.000343 | 0.000158 | 68.42 |
| 0.09 | 0.00090 | 0.00033 | 0.216 | 0.000395 | 0.000251 | 0.000145 | 63.33 |
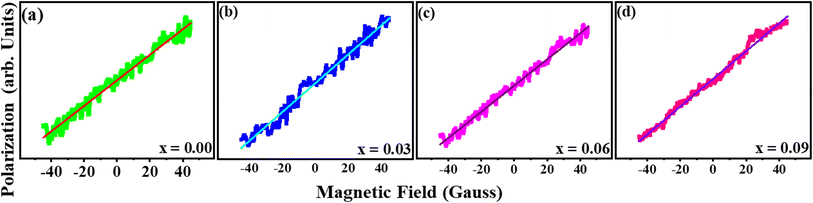
The connection between electric and magnetic orders in tri-phase composites are analyzed by M–E coupling phenomenon and are sketched in Fig. 7(a–d). The strength of coupling between the two orders can be justified by exchange interaction between them. The devices having both orders simultaneously can induce electric polarization through the application of magnetic field and the other way round. Application of magnetic field affects the magnetic domains inside the material that outcome in lattice strain, and generates polarization which fluctuates in the same fashion as the applied magnetic field that subsequently produces piezoelectric effect. Herein, the M–E coupling effects in the specimen are just reasonable signals, and are not perfect loops that are linearly fit for better understanding of the trends. In fact, this coupling is indirect as clear from the relation below,
Hence, by keeping all the results and discussion in mind, it can be concluded that NFO substituted composites are well established to have better coupling between perovskite, and spinel or between electric and magnetic orders, respectively. Hence, this coupling makes MF appreciable for energy storage, electronic, spintronics, and logic-based states [(P+, M+), (P+, M−), (P−, M+)(P−, M−)] devices to read or write the non-volatile data by any field.35
4. Conclusion
In a nutshell, this work explores the applicability of a feasible and economical citrate-gel self-ignition and solid-state ball-milling approaches for the synthesis of (1 − x)[0.7BFO + 0.3MMO] + xNFO (with x = 0.00, 0.03, 0.06, and 0.09 phase-contents) composites. The x = 0.09 substitutional contents presented the highest values of dielectric permittivity's well in agreement with Maxwell–Wagner bi-layer model while suppression of polarization phenomenon is accredited by the declining trend of the dielectric constants. The conductivity trends displayed gradually upsurged behavior towards high frequency regime specifying the inclusion of free as well as bound charges towards conduction mechanism. Further, Nyquist plots signified the existence of only one semi-circular arcs attributing the grain boundaries influence. In addition, the tuned magnetic computations validated highest values of Ms and Mr for x = 0.09 phase-fractions as 5.87 emu g−1 and 0.96 emu g−1, respectively, while Hc value as 215.19 Oe. FE testing assured 68.42% of ferroelectric efficiency with WR = 3.43 × 10−4 mJ cm−3 for x = 0.06 substitutional contents. ME coupling analysis verified MF behavior of tri-phase composites by linear trends. Looking ahead in the future, computational theoretical modelling and the computed parameters are paving a path for as-synthesized composites towards the fabrication of memory as well as modern energy storage devices.Data availability
Data will be available on request.Conflicts of interest
The authors declare that there are no financial or any other type of conflicts of interest to declare.Acknowledgements
The authors would like to acknowledge the Researchers Supporting Project number (RSP2024R71), King Saud University, Riyadh, Saudi Arabia.References
- A. Ouertani, Z. Abdelkafi, H. Khemakhem and N. Randrianantoandro, RSC Adv., 2024, 14(20), 14080–14090 RSC.
- S. Lan, C. Yu, F. Sun, Y. Chen, D. Chen, W. Mai and M. Zhu, Nano Energy, 2022, 93, 106792 CrossRef CAS.
- M. M. Arman, Appl. Phys. A-Matter, 2023, 129(6), 400 CrossRef CAS.
- N. A. Spaldin, Philos. Trans. R. Soc., A, 2020, 476(2233), 20190542 Search PubMed.
- N. D'Souza, J. Atulasimha and S. Bandyopadhyay, J. Phys. D: Appl. Phys., 2011, 44(26), 265001 CrossRef.
- D. Sando, A. Agbelele, C. Daumont, D. Rahmedov, W. Ren, I. C. Infante, S. Lisenkov, S. Prosandeev, S. Fusil, E. Jacquet, C. Carrétéro, S. Petit, M. Cazayous, J. Juraszek, J. M. Le Breton, L. Bellaiche, B. Dkhil, A. Barthélémy and M. Bibes, Philos. Trans. R. Soc., A, 2014, 372(2009), 20120438 CrossRef PubMed.
- I. Ragbaoui, S. Aydi, S. Chkoundali, M. Enneffati and A. Aydi, RSC Adv., 2024, 14(2), 1330–1340 RSC.
- J. Wang, Z. Qing, X. Zhou, H. Zou, H. Li, A. Liu, S. Duan and Y. Li, Ceram. Int., 2023, 49(14), 23627–23633 CrossRef.
- K. Yousefipour, R. Sarraf-Mamoory and S. Mollayousefi, RSC Adv., 2022, 12(43), 27868–27876 RSC.
- S. B. Narang and K. Pubby, J. Magn. Magn. Mater., 2021, 519, 167163 CrossRef.
- M. Khan, A. Mishra, J. Shukla, S. Bisen and P. Sharma, AIP Conf. Proc., 2019, 2115, 030107 CrossRef.
- B. Dhanalakshmi, B. C. Sekhar, K. V. Vivekananda, B. S. Rao, B. P. Rao and P. S. Rao, Appl. Phys. A-Matter, 2020, 126, 1–9 CrossRef.
- M. Umair, A. Quader, M. Imran, M. A. Yaqub, S. M. Ramay and S. Atiq, Ceram. Int., 2022, 48(10), 14473–14480 CrossRef.
- U. Ali, M. A. Khan, M. Mehak, M. T. Ansar, S. M. Ramay, E. A. Alghamdi, M. Shahabuddin and S. Atiq, J. Alloys Compd., 2024, 970, 172536 CrossRef.
- P. Augustine, Y. Narayana and N. Kalarikkal, Mater. Today: Proc., 2020, 25, 208–212 Search PubMed.
- S. Moharana, T. Yadav, P. A. Alvi, A. Pathak and R. N. Mahaling, J. Mater. Sci.: Mater. Electron., 2021, 32, 6038–6046 Search PubMed.
- M. Mehak, M. A. Khan, U. Ali, A. Quader, M. Saleem, G. M. Mustafa, A. S. Haidyrah and S. Atiq, Ceram. Int., 2021, 47(15), 21688–21697 CrossRef.
- K. Sreekanth, B. Dhanalakshmi and D. Madhavaprasad, J. Indian Chem. Soc., 2022, 99(7), 100565 CrossRef.
- M. A. Khan, K. Shahbaz, G. M. Mustafa, S. M. Ramay, S. Naseem and S. Atiq, J. Alloys Compd., 2023, 947, 169571 CrossRef.
- N. Patel, A. L. Srivastav, A. Patel, A. Singh, S. K. Singh, V. K. Chaudhary, P. K. Singh and B. Bhunia, Environ. Sci. Pollut. Res., 2022, 29(46), 69137–69152 CrossRef PubMed.
- P. Piza-Ruiz, D. Avila-Ramos, A. Saenz-Trevizo, O. Solís-Canto, P. Amézaga-Madrid and M. Miki-Yoshida, Ceram. Int., 2021, 47, 18969–18976 CrossRef.
- X. Hu and T. Suzuki, Materials, 2023, 16(20), 6713 CrossRef PubMed.
- E. Paradisi, M. Lassinantti Gualtieri, P. Veronesi, V. Dami, G. Lorenzi, A. Cioni, G. Baldi and C. Leonelli, ACS Appl. Nano Mater., 2023, 6(7), 5448–5459 CrossRef.
- A. K. Bhakta, Y. Snoussi, M. E. Garah, S. Ammar and M. M. Chehimi, C, 2022, 8(3), 46 Search PubMed.
- F. Deeba, A. K. Gupta, V. Kulshrestha, M. Bafna and A. Jain, J. Mater. Sci.: Mater. Electron., 2022, 33(30), 23703–23713 CrossRef.
- F. Afzal, M. T. Ansar, A. S. Haidyrah, M. A. Khan, G. M. Mustsfa, M. Saleem and S. Atiq, J. Alloys Compd., 2021, 888, 161516 CrossRef.
- B. D. Cullity, Elements of X-ray Diffraction, Addison-Wesley Publishing, 1956 Search PubMed.
- C. F. Holder and R. E. Schaak, ACS Nano, 2019, 13(7), 7359–7365 CrossRef CAS.
- S. Nasiri, M. Rabiei, A. Palevicius, G. Janusas, A. Vilkauskas, V. Nutalapati and A. Monshi, NanoTrends, 2023, 3, 100015 Search PubMed.
- H. Ayub, L. A. Khan, E. McCarthy, I. U. Ahad, K. Fleischer and D. Brabazon, Metals, 2022, 19, 208–219 Search PubMed.
- R. K. Rajaboina, U. K. Khanapuram, V. Vivekananthan, G. Khandelwal, S. Potu, A. Babu, N. Madathil, M. Velpula and P. Kodali, Small, 2023, 2306209 Search PubMed.
- S. Kavitha and M. Kurian, J. Alloys Compd., 2019, 799, 147–159 CrossRef.
- M. M. Kumar, V. R. Palkar, K. Srinivas and S. V. Suryanarayana, Appl. Phys. Lett., 2000, 76(19), 2764–2766 CrossRef.
- U. Waqas, M. U. Salman, M. A. Khan, S. M. Ramay, F. Ahmad, S. Riaz and S. Atiq, J. Mater. Sci. Technol., 2024, 29, 2971–2979 Search PubMed.
- M. Luqman, R. Shazaib, A. Raza, M. A. Khan, M. A. Shar, S. M. Ramay, S. Riaz and S. Atiq, J. Magn. Magn. Mater., 2023, 587, 171361 CrossRef.
| This journal is © The Royal Society of Chemistry 2024 |