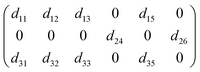Hydrogen bonding bolstered head-to-tail ligation of functional chromophores in a 0D SbF₃·glycine adduct for a short-wave ultraviolet nonlinear optical material

Abstract

The key properties of nonlinear optical (NLO) materials highly rely on the quality of functional chromophores (FCs) and their optimized interarrangement in the lattice. Despite the screening of various FCs, significant challenges persist in optimizing their arrangement within specific structures. Generally, FC alignment is achieved by designing negatively charged 2D layers or 3D frameworks, further regulated by templating cations. In this study, a novel 0D adduct NLO material, SbF₃·glycine, is reported. Neutrally charged 0D [SbF₃C₂H₅NO₂] FCs, comprising [SbF₃] pyramids and zwitterionic glycine, are well-aligned in the structure. The alignment is facilitated by the hydrogen bonding, reinforcing a ‘head-to-tail’ ligation of [SbF₃C₂H₅NO₂] FCs. Consequently, the title compound exhibits favorable NLO properties, including a large second-harmonic generation efficiency (3.6 × KDP) and suitable birefringence (cal. 0.057 @ 1064 nm). Additionally, its short absorption cut-off edge (231 nm) positions it as a promising short-wave ultraviolet NLO material. Importantly, the binary SbF₃–amino acid system is expected to serve as a new resource for exploring ultraviolet NLO crystals, owing to the abundance of the amino acid family.

---

Nonlinear optical (NLO) materials have the capability to efficiently generate coherent light with photon energies in the short-wavelength range, such as ultraviolet (UV, 200–400 nm) and deep-UV (below 200 nm), through a second-harmonic generation (SHG) process. Consequently, they find wide applications in various laser-driven technologies, including laser photolithography, quantum entanglement, medical treatment, and laser micromachining.^1–4 From a materials perspective, the ongoing challenge lies in discovering new UV NLO materials that exhibit a large SHG coefficient, possesses significant birefringence, and have a short absorption cut-off edge (a wide band gap). However, achieving these properties poses a challenge attributable to the contradictory relationship among them.⁵ Over the past few decades, the exploration of promising UV NLO crystals has primarily focused on borates. This is because planar [BO₃]³⁻ and ring-type [B₃O₆]³⁻ functional chromophores (FCs) in borates enable them to exhibit large SHG efficiency and birefringence.^6–14 Subsequently, the exploration of UV NLO materials has extended to carbonates, nitrates, and cyanurates.^15–22

In addition to π-conjugated anions, stereochemically active lone pair (SCALP) cations such as Pb²⁺, Sn²⁺, Bi³⁺, and Sb³⁺ are also considered to be good FCs for designing high-performance NLO materials.^11,23–36 This is because these SCALP ions are commonly susceptible to forming highly distorted polyhedra, thereby giving rise to large SHG efficiency and birefringence. However, Pb²⁺ is toxic, while Sn²⁺ and Bi³⁺ usually cause red-shifted cutoff edges. In recent years, Zou and coworkers discovered that SCALP Sb³⁺ could be a good UV NLO FC because it can endow materials with short cutoff edges (commonly below 266 nm, the fourth harmonic generation of a 1064 nm Nd:YAG laser) while retaining a large SHG effect and birefringence. This has been demonstrated by a few antimony sulfates and phosphates, such as CsSbF₂SO₄,³⁷ RbSbSO₄Cl₂,³⁸ and ASbP₂O₇F (A = K and Rb), among others.^39,40 To date, the development of Sb³⁺-based UV NLO materials has been mainly limited to sulfate and phosphate salts, with most of these materials exhibiting relatively lower SHG intensity attributable to the unfavorable arrangement of antimony polyhedra and the lesser contribution of SO₄²⁻ or PO₄³⁻ units to SHG.^35,41,42 Given this context, achieving a uniformly arranged lattice of Sb³⁺-based polyhedra and concurrently introducing other types of FCs has become important.⁴³ However, the desired uniform alignment of Sb³⁺-based polyhedra is only realized in a few crystals, such as 3D fresnoite-type ASbP₂O₇F (A = K and Rb). In this study, we endeavor to expand the frontiers of Sb³⁺-based UV NLO materials by combining Sb³⁺ with amino acids, a rarely explored approach. The amino acids are expected to serve not only as additional FCs but also to optimize the arrangement of Sb³⁺-based polyhedra through hydrogen bonding. Herein, we report a novel 0D adduct NLO material, SbF₃·glycine, composed of stoichiometric antimony(III) trifluoride and the amino acid glycine. The antimony polyhedra and glycine molecules interact with each other through hydrogen bonding, resulting in a uniform ‘head-to-tail’ arrangement of [SbF₃C₂H₅NO₂] clusters, which gives rise to excellent NLO properties.

SbF₃·Gly crystals were synthesized using an aqueous solution evaporation technique at room temperature (see details in the Experimental section in the ESI†). SbF₃·Gly is somewhat hygroscopic in wet air; therefore, it should be stored in a dry environment, such as a desiccator. The powder X-ray diffraction patterns (XRD) recorded on a Miniflex 600 diffractometer matched well with the simulated ones (Fig. S1†), thus confirming phase purity. Elemental analysis based on an energy-dispersive spectrometer (EDS) revealed the presence of Sb, O, F, C, and N (Fig. S2†). Thermogravimetric (TG) testing showed that SbF₃·Gly is thermally stable up to ca. 105 °C, beyond which it undergoes slow decomposition (Fig. S3†).

The single-crystal XRD analysis reveals a noncentrosymmetric polar space group, Pc (no. 7) for SbF₃·Gly, with well-refined R values (Table S1†). It belongs to an adduct compound composed of stoichiometric SbF₃ and zwitterionic glycine (Fig. 1a), which are further connected to form 0D [SbF₃C₂H₅NO₂] clusters (Fig. 1b) by sharing the O(2) atom. Antimony is four-coordinated, producing a one-sided [SbOF₃] seesaw, with an Sb–O bond length of 2.243(5) Å and Sb–F interatomic distances ranging from 1.914(4) to 2.036(5) Å (Fig. 1b). The bond valence calculations reveal a bond valence sum value of +2.906 for the cationic ion Sb³⁺, consistent with its expected oxidation state of +3 (Table S7†). In the structure, the discrete [SbF₃C₂H₅NO₂] clusters repeat with a ‘head-to-tail’ ligation model, with SbF₃ serving as the ‘head’ and glycine as the ‘tail’. It should be pointed out that the ‘head’ and ‘tail’ are connected and bolstered by N–H⋯F hydrogen bonding with H⋯F bond lengths ranging from 2.750(7) to 2.924(6) Å (Fig. 1c and Table S6†) to form the entire structure, highlighting the crucial roles of hydrogen bonding in the formation and support of ‘head-to-tail’ linkage of FCs in SbF₃·Gly. Furthermore, it is worth noting that such ‘head-to-tail’ ligation of 0D [SbF₃C₂H₅NO₂] clusters aligns them with each other, resulting in a uniform arrangement in the lattice, with the lone pair of Sb³⁺ ions oriented along the rough [111] direction (Fig. 1d). The ‘head-to-tail’ connection gives rise to constructively added net dipole moments of [SbOF₃] polyhedra along the [100] and [001] directions (Table S8†), which is favourable for stimulating large SHG for SbF₃·Gly.

Fig. 1 The crystal structure of the molecular SbF₃·Gly compound. The SbF₃ and zwitterion glycine primitives (a) combine to form 0D [SbF₃C₂H₅NO₂] clusters (b) by sharing the O(2) atom, which repeats periodically in the structure with head-to-tail ligation, reinforced by hydrogen bonding (c). The alignment of [SbF₃C₂H₅NO₂] clusters in the double unit cell (d). The purple lobe represents the lone pair of Sb³⁺.

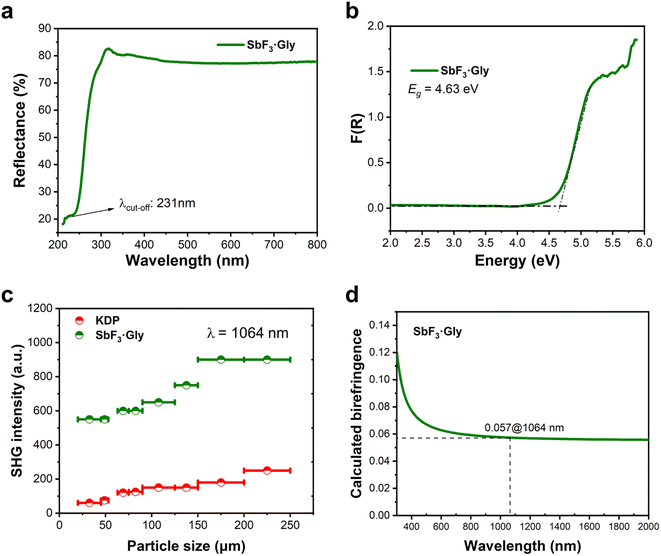
The UV-vis diffuse reflectance spectrum (Fig. 2a) suggests that SbF₃·Gly is transparent in the UV region with a short UV absorption cut-off (λ_cuf-off) edge of ca. 231 nm, falling within the solar-blind UV region (210–280 nm). This value is one of the shortest observed among Sb³⁺-based UV NLO crystals,^37,39,44 indicating that SbF₃·Gly is suitable for short-wave UV applications. Furthermore, using the Kubelka–Munk function, its optical band gap is determined to be 4.63 eV (Fig. 2b).

Fig. 2 The linear and nonlinear optical properties of SbF₃·Gly. (a) The UV-vis diffuse reflectance spectrum and (b) optical band gap (E_g) determined using the Kubelka–Munk equation. (c) Particle size-dependent powder SHG efficiency. (d) Calculated birefringence.

As the title compound crystallizes in an acentric space group, its powder SHG efficiency was measured based on the Kurtz–Perry method under 1064 nm laser irradiation.⁴⁵ The standard KH₂PO₄ (KDP) crystal was used as a reference. As shown in Fig. 2c, the SHG intensities increase with rising particle size before reaching maximum values independent of particle size, indicating that SbF₃·Gly is phase-matchable in the visible region. The phase-matching (PM) ability of SbF₃·Gly is also confirmed by its moderate birefringence, as discussed later. Furthermore, SbF₃·Gly exhibits a large powder SHG efficiency, ca. 3.6 times that of KDP for the same particle size range of 200–250 μm. It should be noted that the Flack parameter for SbF₃·Gly is 0.20(4) (Table S1†), indicating that the crystal is slightly twinned. Thus, SHG produced by one twin may be slightly weakened by the other. In other words, the real SHG efficiency is probably larger than 3.6 × KDP if the crystal is not twinned. The SHG ability of the SbF₃·Gly adduct is comparable to that of numerous potential short-wave UV NLO crystals, including Sb-based SbB₃O₆ (3.5 × KDP),⁴⁶ K₂Sb(P₂O₇)F (4.0 × KDP),³⁹ CsSbF₂SO₄ (3.0 × KDP),³⁷ ASbSO₄Cl₂ (2.7 × KDP for A = Rb and 1.7 × KDP for A = NH₄),^38,41 Rb₂SbF₃(NO₃)₂ (2.7 × KDP);⁴⁷ borates such as CaZn₂(BO₃)₂ (3.8 × KDP)⁴⁸ and Ca₂B₃O₆X (1.5 × KDP for X = Cl and 2.0 × KDP for X = Br);⁴⁹ carbonates such as AMCO₃F (A = alkali, M = alkaline earth, 1.11–4.0 × KDP),¹⁵ NaZnCO₃(OH) (5.2 × KDP),¹⁸ and LiZnCO₃(OH) (3.2 × KDP),¹⁷ sulfates including MF₂SO₄ (3.2 × KDP for M = Zr and 2.5 × KDP for M = Hf)⁵⁰ and sulfamates such as Ba(NH₂SO₃)₂ (2.7 × KDP),⁵¹ SO₂(NH₂)₂ (4.0 × KDP)⁵² and Cs₂Mg(NH₂SO₃)₄·4H₂O (2.3 × KDP);⁵³ selenites such as Sc(HSeO₃)₃ (5.0 × KDP)⁵⁴ and Y₃F(SeO₃)₄ (5.5 × KDP);⁵⁵ and a few hybrid materials such as KLi(HC₃N₃O₃)·2H₂O (5.3 × KDP),²¹ Rb[PO₂(NH₃)(CO)₂]·0.5H₂O (4.2 × KDP),⁵⁶ and 2(C₃H₇N₆)⁺·2Cl·2H₂O (4.3 × KDP),⁵⁷ among others.

To better understand the structure–property relationship of SbF₃·Gly, first principles calculations were carried out using the plane-wave pseudopotential method implemented in the CASTEP package, based on density functional theory (see details in the ESI†). The calculated band gap of SbF₃·Gly under the GGA-PBE functional is 4.23 eV (Fig. S4†), which is slightly less than the experimental value (4.63 eV). Therefore, a scissor operator of 0.40 eV was applied when assessing the optical properties. The total and partial densities of states for SbF₃·Gly are plotted in Fig. S5.† Specifically, the upper part of the valence band (−3.2 to 0.0 eV) is dominated by O 2p and Sb 5s5p electronic states, while the bands ranging from −10.0 to −3.2 eV are mainly from F 2p, O 2p, C 2p and N 2p electronic states, mixed with a small amount of H 1s and Sb 5s5p states. The conduction band primarily comprises unoccupied Sb 5p, C 2p, O 2p, and H 1s orbitals, as well as a small number of N 2p orbitals. It is worth noting that the valence band maximum is contributed by the nonbonding states of O 2p and Sb 5s5p, while the conduction band minimum originates from the π*-antibonding states of C–O bonds in glycine. Thus, the band gap of SbF₃·Gly is determined by the Sb³⁺-based polyhedra and glycine molecules.

Fig. 2d displays the calculated birefringence (Δn) curve, from which we can observe that the birefringence of SbF₃·Gly at 1064 nm is about 0.057. This value falls within a desirable range for a UV NLO material, categorized as ‘moderate’, which ensures good PM capability in the SHG process. We further examined its PM capacity based on the calculated refractive indices (Fig. S6†). The results reveal that its shortest PM wavelength reaches 366 nm, confirming its PM ability in the visible region and suggesting potential applications for UV laser output through the SHG process. It is worth noting that the shortest PM wavelength of SbF₃·Gly is 135 nm longer than its λ_cut-off edge, resulting in a 135 nm loss of PM. This discrepancy is likely owing to the severe refractive dispersion effect (the curves become suddenly steep) below the 500 nm energy region, which prevents the PM from further approaching shorter wavelengths.

Considering structural symmetry (point group: m) and Kleinman symmetry, SbF₃·Gly exhibits 10 nonvanishing SHG tensors, namely

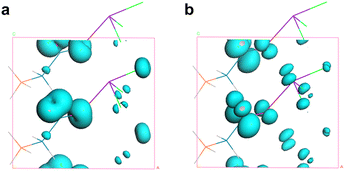
Among these, d₁₁, d₁₂ = d₂₆, d₁₃ = d₃₅, d₁₅ = d₃₁, d₂₄ = d₃₂, and d₃₃. The static values of these tensors were calculated and are listed in Table S9.† Notably, d₃₂ exhibits the largest tensor, 1.48 pm V⁻¹, which is approximately 3.8 times that of KDP (d₃₆ = 0.39 pm V⁻¹), aligning well with the powder SHG efficiency (3.6 × KDP). To further uncover the underlying origin of the strong SHG effect in SbF₃·Gly, SHG-weighted electron density analyses for d₃₂ were performed (Fig. 3). It was found that in the valence band (VB) (Fig. 3a), the O 2p nonbonding states contribute significantly to the SHG, with additional contributions from F 2p and C 2p states. In the conduction band (CB) (Fig. 3b), the SHG effect primarily originates from the π*-antibonding states of C–O bonds and Sb 5p orbitals, along with minor contributions from F 2p orbitals. Based on the SHG-density data, the group contributions can be delineated as 42.16% for [SbOF₃] and 57.84% for glycine, indicating that the collaborative effect of [SbOF₃] and glycine results in the SHG response of SbF₃·Gly.

Fig. 3 The SHG-weighted electron density in (a) the VB and (b) CB for the d₃₂ coefficient.

Conclusions

In summary, we present a new UV NLO material, SbF₃·Gly, based on Sb³⁺. This compound comprises 0D [SbF₃C₂H₅NO₂] FCs formed by [SbF₃] pyramids and zwitterionic glycine, sharing the oxygen corner. These [SbF₃C₂H₅NO₂] FCs adopt a head-to-tail arrangement sustained by hydrogen bonding, resulting in a large SHG response, 3.6 times that of KDP, and a moderate birefringence (Δn(cal.) = 0.057) at 1064 nm, which imposes a PM limit of 366 nm. In addition, the material also exhibits a short absorption edge of 231 nm, one of the smallest values among Sb³⁺-based NLO materials. These attributes position SbF₃·Gly as a potential short-wavelength UV NLO material.

Data availability

Crystallographic data for SbF₃·Gly have been deposited with the CCDC under deposition number 22049585 and can be obtained from https://www.ccdc.cam.ac.uk/. Other data are available from the authors upon request.

Author contributions

Z. Bai synthesized and characterized the compound, J. Lee carried out the SHG measurement, C.-L. Hu performed the theoretical calculations, G. Zou analyzed the data, and K. M. Ok devised and supervised the experiments. All authors contributed to the writing and editing of the manuscript.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

This work was supported by the National Research Foundation of Korea (NRF) funded by the Ministry of Science and ICT (nos. 2018R1A5A1025208 and 2019R1A2C3005530).

Notes and references

1. M. Mutailipu, M. Zhang, Z. Yang and S. Pan, Acc. Chem. Res., 2019, 52, 791–801.
2. M. Mutailipu, K. R. Poeppelmeier and S. Pan, Chem. Rev., 2021, 121, 1130–1202.
3. M. Mutailipu, J. Han, Z. Li, F. Li, J. Li, F. Zhang, X. Long, Z. Yang and S. Pan, Nat. Photonics, 2023, 17, 694–701.
4. Y. Li, J. Luo and S. Zhao, Acc. Chem. Res., 2022, 55, 3460–3469.
5. M. Mutailipu, Z. Yang and S. Pan, Acc. Mater. Res., 2021, 2, 282–291.
6. C. Chen, Y. Wu, A. Jiang, B. Wu, G. You, R. Li and S. Lin, J. Opt. Soc. Am. B, 1989, 6, 616–621.
7. C. Chen, B. Wu, A. Jiang and G. You, Sci. Sin., Ser. B, 1985, 28, 235–243.
8. S. Zhao, P. Gong, L. Bai, X. Xu, S. Zhang, Z. Sun, Z. Lin, M. Hong, C. Chen and J. Luo, Nat. Commun., 2014, 5, 4019.
9. H. Wu, S. Pan, K. R. Poeppelmeier, H. Li, D. Jia, Z. Chen, X. Fan, Y. Yang, J. M. Rondinelli and H. Luo, J. Am. Chem. Soc., 2011, 133, 7786–7790.
10. Y. Yang, S. Huang and S. Pan, J. Mater. Chem. C, 2022, 10, 11232–11238.
11. G. Zou, C. Lin, H. Jo, G. Nam, T. S. You and K. M. Ok, Angew. Chem., Int. Ed., 2016, 55, 12078–12082.
12. X. Wang, Y. Wang, B. Zhang, F. Zhang, Z. Yang and S. Pan, Angew. Chem., Int. Ed., 2017, 129, 14307–14311.
13. H. Yu, H. Wu, S. Pan, Z. Yang, X. Hou, X. Su, Q. Jing, K. R. Poeppelmeier and J. M. Rondinelli, J. Am. Chem. Soc., 2014, 136, 1264–1267.
14. M. Mutailipu, M. Zhang, H. Wu, Z. Yang, Y. Shen, J. Sun and S. Pan, Nat. Commun., 2018, 9, 3089.
15. G. Zou, N. Ye, L. Huang and X. Lin, J. Am. Chem. Soc., 2011, 133, 20001–20007.
16. G. Zou, L. Huang, N. Ye, C. Lin, W. Cheng and H. Huang, J. Am. Chem. Soc., 2013, 135, 18560–18566.
17. X. Liu, L. Kang, P. Gong and Z. Lin, Angew. Chem., Int. Ed., 2021, 60, 13574–13578.
18. G. Peng, C. Lin and N. Ye, J. Am. Chem. Soc., 2020, 142, 20542–20546.
19. J. Wang, Y. Cheng, H. Wu, Z. Hu, J. Wang, Y. Wu and H. Yu, Angew. Chem., Int. Ed., 2022, 61, e202201616.
20. X. Dong, L. Huang, Q. Liu, H. Zeng, Z. Lin, D. Xu and G. Zou, Chem. Commun., 2018, 54, 5792–5795.
21. D. Lin, M. Luo, C. Lin, F. Xu and N. Ye, J. Am. Chem. Soc., 2019, 141, 3390–3394.
22. J. Lu, Y. K. Lian, L. Xiong, Q. R. Wu, M. Zhao, K. X. Shi and L. M. Wu, J. Am. Chem. Soc., 2019, 141, 16151–16159.
23. L. Qi, Z. Chen, X. Shi, X. Zhang, Q. Jing, N. Li and M. H. Lee, Chem. Mater., 2020, 32, 8713–8723.
24. M. Abudoureheman, S. Han, B. H. Lei, Z. Yang, X. Long and S. Pan, J. Mater. Chem. C, 2016, 4, 10630–10637.
25. S.-H. Kim, J. Yeon and P. S. Halasyamani, Chem. Mater., 2009, 21, 5335–5342.
26. Y. Z. Huang, L. M. Wu, X. T. Wu, L. H. Li, L. Chen and Y. F. Zhang, J. Am. Chem. Soc., 2010, 132, 12788–12789.
27. M. Luo, Y. Song, F. Liang, N. Ye and Z. Lin, Inorg. Chem. Front., 2018, 5, 916–921.
28. F. You, F. Liang, Q. Huang, Z. Hu, Y. Wu and Z. Lin, J. Am. Chem. Soc., 2019, 141, 748–752.
29. H. Zhang, M. Zhang, S. Pan, X. Dong, Z. Yang, X. Hou, Z. Wang, K. B. Chang and K. R. Poeppelmeier, J. Am. Chem. Soc., 2015, 137, 8360–8363.
30. X. Chen, Q. Jing and K. M. Ok, Angew. Chem., Int. Ed., 2020, 59, 20323–20327.
31. H. Yu, N. Z. Koocher, J. M. Rondinelli and P. S. Halasyamani, Angew. Chem., Int. Ed., 2018, 57, 6100–6103.
32. Y. J. Jia, X. Zhang, Y. G. Chen, X. Jiang, J. N. Song, Z. Lin and X. M. Zhang, Inorg. Chem., 2022, 61, 15368–15376.
33. J. Guo, S. Cheng, S. Han, Z. Yang and S. Pan, Adv. Opt. Mater., 2020, 9, 2001734.
34. K. Chen, Y. Yang, G. Peng, S. Yang, T. Yan, H. Fan, Z. Lin and N. Ye, J. Mater. Chem. C, 2019, 7, 9900–9907.
35. Q. Wei, C. He, K. Wang, X. F. Duan, X. T. An, J. H. Li and G. M. Wang, Chem.–Eur. J., 2021, 27, 5880–5884.
36. Q. Wei, K. Wang, C. He, L. Wei, X. F. Li, S. Zhang, X. T. An, J. H. Li and G. M. Wang, Inorg. Chem., 2021, 60, 11648–11654.
37. X. Dong, L. Huang, C. Hu, H. Zeng, Z. Lin, X. Wang, K. M. Ok and G. Zou, Angew. Chem., Int. Ed., 2019, 58, 6528–6534.
38. F. He, Y. Deng, X. Zhao, L. Huang, D. Gao, J. Bi, X. Wang and G. Zou, J. Mater. Chem. C, 2019, 7, 5748–5754.
39. Y. Deng, L. Huang, X. Dong, L. Wang, K. M. Ok, H. Zeng, Z. Lin and G. Zou, Angew. Chem., Int. Ed., 2020, 59, 21151–21156.
40. X. Dong, H. Huang, L. Huang, Y. Zhou, B. Zhang, H. Zeng, Z. Lin and G. Zou, Angew. Chem., Int. Ed., 2024, e202318976,  DOI:10.1002/anie.202318976.
41. F. He, Q. Wang, C. Hu, W. He, X. Luo, L. Huang, D. Gao, J. Bi, X. Wang and G. Zou, Cryst. Growth Des., 2018, 18, 6239–6247.
42. F. Yang, L. Wang, Y. Ge, L. Huang, D. Gao, J. Bi and G. Zou, J. Alloys Compd., 2020, 834.
43. K. M. Ok, Acc. Chem. Res., 2016, 49, 2774–2785.
44. S. Han, A. Tudi, W. Zhang, X. Hou, Z. Yang and S. Pan, Angew. Chem., Int. Ed., 2023, 62, e202302025.
45. S. K. Kurtz and T. T. Perry, J. Appl. Phys., 1968, 39, 3798–3813.
46. Y. Liu, X. Liu, S. Liu, Q. Ding, Y. Li, L. Li, S. Zhao, Z. Lin, J. Luo and M. Hong, Angew. Chem., Int. Ed., 2020, 59, 7793–7796.
47. L. Wang, H. Wang, D. Zhang, D. Gao, J. Bi, L. Huang and G. Zou, Inorg. Chem. Front., 2021, 8, 3317–3324.
48. M. Mutailipu, F. Li, C. Jin, Z. Yang, K. R. Poeppelmeier and S. Pan, Angew. Chem., Int. Ed., 2022, 61, e202202096.
49. H. Qiu, F. Li, Z. Li, Z. Yang, S. Pan and M. Mutailipu, J. Am. Chem. Soc., 2023, 145, 24401–24407.
50. C. Wu, C. Jiang, G. Wei, X. Jiang, Z. Wang, Z. Lin, Z. Huang, M. G. Humphrey and C. Zhang, J. Am. Chem. Soc., 2023, 145, 3040–3046.
51. X. Hao, M. Luo, C. Lin, G. Peng, F. Xu and N. Ye, Angew. Chem., Int. Ed., 2021, 60, 7621–7625.
52. H. Tian, N. Ye and M. Luo, Angew. Chem., Int. Ed., 2022, 61, e202200395.
53. X. Wang, X. Leng, Y. Kuk, J. Lee, Q. Jing and K. M. Ok, Angew. Chem., Int. Ed., 2024, 63, e202315434.
54. Z. Bai, J. Lee, H. Kim, Y. Kuk, M. H. Choi, C. L. Hu and K. M. Ok, Small, 2023, 2207709.
55. P. F. Li, C. L. Hu, F. Kong and J. G. Mao, Angew. Chem., Int. Ed., 2023, 62, e202301420.
56. X. Song, Z. Du, B. Ahmed, Y. Li, Y. Zhou, Y. Song, W. Huang, J. Zheng, J. Luo and S. Zhao, Inorg. Chem. Front., 2023, 10, 5462–5467.
57. L. Liu, C. L. Hu, Z. Bai, F. Yuan, Y. Huang, L. Zhang and Z. Lin, Chem. Commun., 2020, 56, 14657–14660.

---

Footnote

† Electronic supplementary information (ESI) available: Tables of crystal data and structure refinement, fractional atomic coordinates, anisotropic displacement parameters, selected bond lengths and bond angles, hydrogen bonds, dipole moments, and second-order nonlinear optical coefficients, figures of PXRD, EDS, TG, band structures, PDOS, and simulated phase-matching wavelength. CCDC 2049585. For ESI and crystallographic data in CIF or other electronic format see DOI: https://doi.org/10.1039/d4sc01353k

---