Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Understanding and removing surface states limiting charge transport in TiO2 nanowire arrays for enhanced optoelectronic device performance†
Xia
Sheng
a,
Liping
Chen
a,
Tao
Xu
c,
Kai
Zhu
b and
Xinjian
Feng
*a
aCollege of Chemistry, Chemical Engineering and Materials Science, Soochow University, Suzhou 215123, P. R. China. E-mail: xjfeng@suda.edu.cn
bNational Renewable Energy Laboratory, 1617 Cole Boulevard, Golden, Colorado 80401, USA
cDepartment of Chemistry and Biochemistry, Northern Illinois University, DeKalb, Illinois 60115, USA
First published on 8th December 2015
Abstract
Charge transport within electrode materials plays a key role in determining the optoelectronic device performance. Aligned single-crystal TiO2 nanowire arrays offer an ideal electron transport path and are expected to have higher electron mobility. Unfortunately, their transport is found not to be superior to that in nanoparticle films. Here we show that the low electron transport in rutile TiO2 nanowires is mainly caused by surface traps in relatively deep energy levels, which cannot be removed by conventional approaches, such as oxygen annealing treatment. Moreover, we demonstrate an effective wet-chemistry approach to minimize these trap states, leading to over 20-fold enhancement in electron diffusion coefficient and 62% improvement in solar cell performance. On the basis of our results, the potential of TiO2 NWs can be developed and well-utilized, which is significantly important for their practical applications.
Introduction
Nanoscale semiconducting metal oxides have become promising low-cost electrode materials for solar cells, solar fuels and electric energy storage applications.1–5 Charge transport within electrode materials is a major determinant of device performance. It has been generally accepted that electrons undergo a random walk through electrode networks and are impeded mainly by surface trap states, grain boundaries and structural disorder.6–9 Compared to randomly packed rutile NP films, ordered single-crystal (grain boundaries free) TiO2 nanowire (NW) arrays are generally expected to have higher electron mobility, and have been the subject of extensive research.10–14 Unfortunately, measurements have shown that their electron mobility is not superior to that in NP films with the same phase (Fig. S1 ESI†).15 This implies that the influence of material architectures on electron transport is less evident in the presence of a large density of surface trap states.8,9 Thus, it is critical to understand the nature of the surface trap states and minimize them in order to exert the expected high electron mobility and device performance of NW arrays. In this study we reveal and demonstrate an effective way to remove the trap states that limit the electron transport in single-crystal rutile TiO2 NW arrays and their device performance.Results and discussion
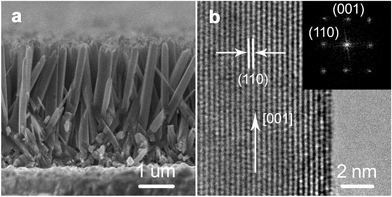
Aligned singe-crystal rutile TiO2 NW arrays were prepared via a conventional hydrothermal method.10–14 As shown in Fig. 1a, the as-prepared TiO2 NWs grow almost vertically from the substrate with an average diameter and length of about 100 nm and 3 μm, respectively. According to the high-resolution transmission electron microscopy (HR-TEM) image (Fig. 1b) and the selected area electron diffraction (SAED) pattern (the inset of Fig. 1b) analysis, the NW is highly crystallized and grows along the [001] direction with side surfaces of {110} crystal plane. Surface treatment is commonly used to boost the performance of semiconductors.16–18 In this report, the as-prepared TiO2 NW arrays were then processed with a wet-chemistry treatment by immersing in a H2O2–NH3 (aq) (10![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) :
:![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 1 v/v) solution at room temperature for different times, then rinsing with a copious amount of distilled water. Subsequently, both the treated and untreated NWs were annealed at 723 K for 30 min in an oxygen-rich environment.
1 v/v) solution at room temperature for different times, then rinsing with a copious amount of distilled water. Subsequently, both the treated and untreated NWs were annealed at 723 K for 30 min in an oxygen-rich environment.
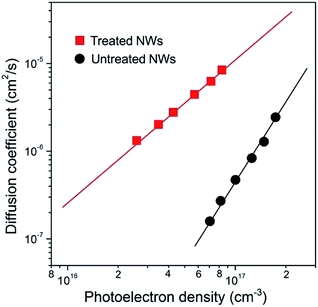
Electron transport in these NWs was probed by using intensity modulated photocurrent spectroscopy (IMPS) and the results are shown in Fig. 2. The values of the electron diffusion coefficient (D) and photoelectron densities (n) are determined from the transport time constants (τc) and film thickness (d) using procedures described elsewhere.19 Compared to the NWs without treatment, the D value in treated NWs was enhanced by over 20 times, for example, at a given n of 1 × 1017 cm−3. According to the previous study, the transient photocurrent response revealed from IMPS measurement is dominated by electron transport within electrode materials and can be explained by a trap-assisted diffusion model. The relation between D and the total trap density (NT) can be described using the following equation:19
| D = C1(NT)−(1/α)+(1/3)n(1/α)−1 |
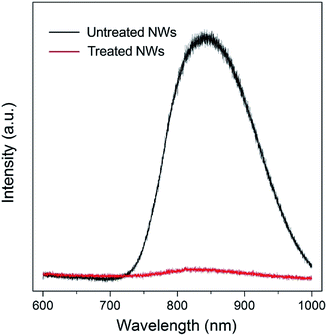
Surface trap states are commonly associated with surface defects. N-type rutile TiO2 is usually in a nonstoichiometric reduced form that has intrinsic defects including oxygen vacancy and titanium interstitial (Tiint3+).22,23 Oxygen vacancies are thermodynamically unstable and can be readily eliminated via simple oxygen annealing at elevated temperature.24,25 Regarding the Tiint3+, it prefers to have a high coordination and is mainly located in the bulk. However, recent studies on rutile TiO2 (110) surface using atomic-resolution scanning tunneling microscopy have shown that Tiint3+ defects can diffuse from the bulk to the surface at temperature higher than 400 K and result in surface reconstruction.25–28 Photoluminescence (PL) is a highly sensitive technique for investigating surface characteristics of semiconductors. As shown in Fig. 3 (black line), a strong near-infrared (NIR) PL peak centered at around 835 nm was observed from untreated NWs. Such a PL peak (835 nm) of rutile TiO2 has been indexed to surface Ti interstitials, specifically to the Tiint4+.29–31 Since the rutile TiO2 NWs were prepared at a temperature of 453 K, and that their side surfaces are {110} crystal plane, Tiint3+ can diffuse to the {110} crystal plane. When the NWs were further annealed in an oxygen-rich environment, these outward-diffused Ti interstitials will be oxidized and form added islands that occupy preferentially at the surface interstitial sites, which results in the strong NIR PL spectrum. Such interstitial defects will likely lead to the formation of trap states in relatively deeper energy levels that extend virtually all the way to the conduction band edge. It is worth noting that, unlike oxygen vacancies, surface Ti interstitial defects cannot be easily removed via conventional oxygen annealing treatment; in contrast, oxygen annealing will promote their outward-diffusion and surface reconstruction process.
Fig. 3 (red line) shows the PL spectrum of TiO2 NWs after 10 min wet-chemistry treatment in H2O2–NH3 (aq) solution followed by 30 min oxygen annealing at 725 K. The peak intensity is significantly reduced, implying that the vast majority of surface Ti interstitial defects were removed after the treatment. Based on the differences of PL intensity and electron diffusion coefficient of NW arrays with and without treatment, it can be concluded that the surface Ti interstitial defects related trap states limit the electron transport of ordered single-crystal TiO2 NW arrays.
During the wet-chemistry treatment, H2O2 can react with surface Tiint4+ and form the soluble complex (TiO2·H2O2) via a possible chemical reaction:32 Tiint4+ + H2O2 + 2H2O → TiO2·H2O2 + 4H+. In the meanwhile, NH3 (aq) will neutralize the H+ and promote the forward reaction. The process of removing surface interstitials is rather fast as confirmed via PL studies. Fig. S3† shows the PL spectra of TiO2 NWs as a function of treatment time. The PL peak intensity was significantly lowered with only 30 s treatment. Upon the treatment, a Ti interstitial depletion layer near the surface will be formed, and consequently, their related trap states can be minimized. Crystal structures analysis including X-ray photoemission spectroscopy (XPS) (Fig. S4†), X-ray diffraction patterns (XRD) (Fig. S5†) and Raman spectra (Fig. S6†) of NW arrays with and without treatment remain unchanged, which confirms that no new phase or impurity was introduced during the treatment. Thus, the wet-chemistry treatment that is presented here is an effective approach to remove surface trap states in deeper energy levels, as exemplified by the observed over 20-fold enhancement in electron transport.
Fast electron transport is expected to lead to high electron collection efficiency and enhanced optoelectronic device performance. Fig. 4a compares the recombination times (τr) as a function of photoelectron density n of solar cells based on the two samples. Base on the data in Fig. 2 and 4a, we determined that the electron diffusion length (Ln) for treated NW is approximately 20 μm, 4 times longer than that for NWs without treatment (∼5 μm) using the relation of Ln = (Dτr)1/2. The charge collection efficiency (ηcc) of treated NW-based cells, as described by the relation: ηcc = 1/[1+(d/Ln)2], where d is the electrode thickness, can be calculated to be 98%, which is 23% larger than that of untreated NW counterpart.
Fig. 4b compares the photocurrent density–voltage (J–V) characteristics of NW array-based solar cells under simulated AM 1.5 light. The cell based on NWs with faster electron transport rate exhibits a short-circuit photocurrent density (Jsc) of 6.01 mA cm−2, 25% higher than that obtained on untreated NW-based cell (4.81 mA cm−2). In general, Jsc is determined by light-harvesting efficiency (ηlh), charge-injection efficiency (ηinj), and ηcc. It worth noting that the surface areas of NW arrays with and without treatment are almost identical according to dye desorption results (Fig. S7†), suggesting that both ηlh and ηinj values of these devices are similar. Thus, the 25% improvement in Jsc of the treated NW-based cells should be mainly ascribed to the enhancement in ηcc of the electrode materials as described above. Besides, the treated NW-based cell exhibits a higher fill factor (FF), which can also be ascribed to the much enhanced electron transport property. Under similar surface areas, the faster electron transport leads to higher values of Jsc and FF, and 62% enhancement in solar to electricity conversion efficiency. In the future, if the growth technique could be extended to very long 1D ordered nanostructures then one would have a superior electrode material for various kinds of sensitized and heterojunction solar cells.
Conclusions
In conclusion, we have revealed that the surface trap states of rutile TiO2 NWs in relatively deeper energy levels result in slower-than-expected electron transport. Moreover, we have demonstrated an effective wet-chemistry approach to remove these trap states, leading to over 20-fold enhancement in electron transport and 62% improvement in solar conversion efficiency. Considering the wide range of studies and applications of 1D single crystal TiO2 nanowire arrays, the significant charge transport enhancement achieved in this work will make it a real enabling technology for future solar cells, water splitting and electric energy storage device applications.Acknowledgements
X. F. acknowledges financial support from the National Natural Science Foundation of China (21371178), the Jiangsu Province Science Foundation for Distinguished Young Scholars (BK20150032), and the Chinese Thousand Youth Talents Program (YZBQF11001). X. S. acknowledges financial support from the National Natural Science Foundation of China (21501193). K. Z. acknowledges the support by the Division of Chemical Sciences, Geosciences, and Biosciences, Office of Basic Energy Sciences, U.S. Department of Energy, under contract No. DE-AC36-08GO28308 with the National Renewable Energy Laboratory. The authors acknowledge D. L. of State Key Laboratory of Silicon Materials, Zhejiang University for PL measurements.References
- E. J. W. Crossland, N. Noel, V. Sivaram, T. Leijtens, J. A. A. Webber and H. J. Snaith, Nature, 2013, 495, 215–219 CrossRef CAS PubMed
.
- J. Burschka, N. Pellet, S.-J. Moon, R. Humphry-Baker, P. Gao, M. K. Nazeeruddin and M. Grätzel, Nature, 2013, 499, 316–320 CrossRef CAS PubMed
.
- D. Q. He, X. Sheng, J. Yang, L. P. Chen, K. Zhu and X. J. Feng, J. Am. Chem. Soc., 2014, 136, 16772–16775 CrossRef CAS PubMed
.
- M. G. Walter, E. L. Warren, J. R. Mckone, S. W. Boettcher, Q. X. Mi, E. A. Santori and N. S. Lewis, Chem. Rev., 2010, 110, 6446–6473 CrossRef CAS PubMed
.
- S. N. Habisreutinger, L. Schmidt-Mende and J. K. Stolarczyk, Angew. Chem., 2013, 125, 7516–7557 (
Angew. Chem., Int. Ed.
, 2013
, 52
, 7372–7408
) CrossRef
.
- J. Nelson, Phys. Rev. B: Condens. Matter, 1999, 59, 15374–15380 CrossRef CAS
.
- J. van de Lagemaat and A. J. Frank, J. Phys. Chem. B, 2001, 105, 11194–11205 CrossRef CAS
.
- C. Richter and C. A. Schmuttenmaer, Nat. Nanotechnol., 2010, 5, 769–772 CrossRef CAS PubMed
.
- J. Villanueva-Cab, S. R. Jang, A. F. Halverson, K. Zhu and A. J. Frank, Nano Lett., 2014, 14, 2305–2309 CrossRef CAS PubMed
.
- B. Liu and E. S. Aydil, J. Am. Chem. Soc., 2009, 131, 3985–3990 CrossRef CAS PubMed
.
- H. S. Kim, J. W. Lee, N. Yantara, P. P. Boix, S. A. Kulkarni, S. Mhaisalkar, M. Grätzel and N. G. Park, Nano Lett., 2013, 13, 2412–2417 CrossRef CAS PubMed
.
- Y. J. Hwang, C. Hahn, B. Liu and P. D. Yang, ACS Nano, 2012, 6, 5060–5069 CrossRef CAS PubMed
.
- J. Li, S. K. Cushing, P. Zheng, T. Senty, F. Meng, A. D. Bristow, A. Manivannan and N. Wu, J. Am. Chem. Soc., 2014, 136, 8438–8449 CrossRef CAS PubMed
.
- G. Wang, H. Wang, Y. Ling, Y. Tang, X. Yang, R. C. Fitzmorris, C. Wang, J. Z. Zhang and Y. Li, Nano Lett., 2011, 11, 3026–3033 CrossRef CAS PubMed
.
- E. Enache-Pommer, B. Liu and E. S. Aydil, Phys. Chem. Chem. Phys., 2009, 11, 9648–9652 RSC
.
- W. Zhou, F. Sun, K. Pan, G. Tian, B. Jiang, Z. Ren, C. Tian and H. Fu, Adv. Funct. Mater., 2011, 21, 1922–1930 CrossRef CAS
.
- W. Zhou, W. Li, J. Wang, Y. Qu, Y. Yang, Y. Xie, K. Zhang, L. Wang, H. Fu and D. Zhao, J. Am. Chem. Soc., 2014, 136, 9280–9283 CrossRef CAS PubMed
.
- M. Matsukawa, R. Ishikawa, T. Hisatomi, Y. Moriya, N. Shibata, J. Kubota, Y. Ikuhara and K. Domen, Nano Lett., 2014, 14, 1038–1041 CrossRef CAS PubMed
.
- K. Zhu, N. Kopidakis, N. R. Neale, J. van de Lagemaat and A. J. Frank, J. Phys. Chem. B, 2006, 110, 25174–25180 CrossRef CAS PubMed
.
- A. J. Frank, N. Kopidakis and J. van de Lagemaat, Coord. Chem. Rev., 2004, 248, 1165–1179 CrossRef CAS
.
- J. A. Anta, J. Nelson and N. Quirke, Phys. Rev. B: Condens. Matter, 2002, 65, 125324 CrossRef
.
- U. Diebold, Surf. Sci. Rep., 2003, 48, 53–229 CrossRef CAS
.
- S. Wendt, P. T. Sprunger, E. Lira, G. K. H. Madsen, Z. Li, J. Ø. Hansen, J. Matthiesen, A. Blekinge-Rasmussen, E. Lægsgaard, B. Hammer and F. Besenbache, Science, 2008, 320, 1755–1759 CrossRef CAS PubMed
.
- S. Krischok, J. Günster, D. W. Goodman, O. Höfft and V. Kempter, Surf. Interface Anal., 2004, 36, 77–82 Search PubMed
.
- H. Onishi and Y. Iwasawa, Phys. Rev. Lett., 1996, 76, 791–794 CrossRef CAS PubMed
.
- N. Shibata, A. Goto, S.-Y. Choi, T. Mizoguchi, S. D. Findlay, T. Yamamoto and Y. Ikuhara, Science, 2008, 322, 570–573 CrossRef CAS PubMed
.
- Z. Zhang, J. Lee, J. T. Yates Jr, R. Bechstein, E. Lira, J. Ø. Hansen, S. Wendt and F. Besenbacher, J. Phys. Chem. C, 2010, 114, 3059–3062 CAS
.
- K. T. Park, M. H. Pan, V. Meunier and E. W. Plummer, Phys. Rev. Lett., 2006, 96, 226105 CrossRef CAS PubMed
.
- A. K. Ghosh, F. G. Wakim and R. R. Addiss Jr, Phys. Rev., 1969, 184, 979–980 CrossRef CAS
.
- B. Santara, P. K. Giri, K. Imakita and M. Fujii, J. Phys. Chem. C, 2013, 117, 23402–23411 CAS
.
- B. Santara, P. K. Giri, K. Imakita and M. Fujii, J. Phys. D: Appl. Phys., 2014, 47, 215302 CrossRef
.
- G. M. Eisenberg, Ind. Eng. Chem., Anal. Ed., 1943, 15, 327–328 CrossRef CAS
.
Footnote |
| † Electronic supplementary information (ESI) available. See DOI: 10.1039/c5sc04076k |
| This journal is © The Royal Society of Chemistry 2016 |