Enhancement of CO₂ binding and mechanical properties upon diamine functionalization of M₂(dobpdc) metal–organic frameworks

Abstract

The family of diamine-appended metal–organic frameworks exemplified by compounds of the type mmen–M₂(dobpdc) (mmen = N,N′-dimethylethylenediamine; M = Mg, Mn, Fe, Co, Zn; dobpdc⁴⁻ = 4,4′-dioxidobiphenyl-3,3′-dicarboxylate) are adsorbents with significant potential for carbon capture, due to their high working capacities and strong selectivity for CO₂ that stem from a cooperative adsorption mechanism. Herein, we use first-principles density functional theory (DFT) calculations to quantitatively investigate the role of mmen ligands in dictating the framework properties. Our van der Waals-corrected DFT calculations indicate that electrostatic interactions between ammonium carbamate units significantly enhance the CO₂ binding strength relative to the unfunctionalized frameworks. Additionally, our computed energetics show that mmen–M₂(dobpdc) materials can selectively adsorb CO₂ under humid conditions, in agreement with experimental observations. The calculations further predict an increase of 112% and 124% in the orientationally-averaged Young's modulus E and shear modulus G, respectively, for mmen–Zn₂(dobpdc) compared to Zn₂(dobpdc), revealing a dramatic enhancement of mechanical properties associated with diamine functionalization. Taken together, our calculations demonstrate how functionalization with mmen ligands can enhance framework gas adsorption and mechanical properties.

---

1 Introduction

Metal–organic frameworks (MOFs) consist of metal clusters or ions that are joined by organic linkers to form porous network solids with large surface areas, high crystallinity, and, in some cases, redox-active open metal sites.^1–10 MOFs are promising for gas storage and separation applications, particularly in the area of carbon capture,^11–16 and accordingly have received significant recent attention in the literature. Carbon dioxide is mainly produced from the combustion of fossil fuels, and in 2011 alone such CO₂ emissions exceeded 32 Gt.¹⁶ It is well-known that CO₂ produced by combustion is a major driver of global warming,^17,18 contributing to rising sea levels and ocean climate change. Therefore, reducing CO₂ emissions is among the most urgent problems facing humanity today.

Among numerous MOFs currently under investigation for CO₂ capture, frameworks of the type M₂(dobdc) (M = Mg, Mn, Fe, Co, Ni, Cu, Zn; dobdc⁴⁻ = 2,5-dioxidobenzene-1,4-dicarboxylate) have been extensively studied due to their high density of open metal sites, which have been shown to engender high selectivity in the separation of various gas molecules.^11,19–28 Previous studies have reported impressive uptake capacities, together with heats of CO₂ adsorption at the open metal sites as high as 43.5 kJ mol⁻¹ in Mg₂(dobdc).²⁹ Notably, this value can be tuned by metal substitution,²⁸ although the M₂(dobdc) family exhibits poor CO₂ selectivity in the presence of H₂O.^30–34 Recently, Mason et al.³² reported equilibrium adsorption isotherms for mixtures of CO₂, N₂, and H₂O, the three most prevalent components of flue gas,³⁵ and showed that the CO₂ capture performance of M₂(dobdc) (M = Mg, Ni) is significantly diminished under humid conditions, due to preferential binding of H₂O over CO₂ at the open metal sites. For Ni₂(dobdc), CO₂ uptake is almost zero in the presence of water.

Although the M₂(dobdc) materials do not selectively adsorb CO₂ under humid conditions, functionalization of open metal sites in the expanded framework M₂(dobpdc) (dobpdc⁴⁻ = 4,4′-dioxidobiphenyl-3,3′-dicarboxylate) with N,N′-dimethylethylenediamine (mmen) has been shown to enhance both the CO₂ affinity and selectivity under humid conditions.^36–38 In mmen–M₂(dobpdc), diamine molecules are grafted onto the open metal sites and dangle into the pore interiors. Notably, the measured heat of CO₂ adsorption in mmen–Mg₂(dobpdc) is as high as 71 kJ mol⁻¹, almost 30 kJ mol⁻¹ greater than in Mg(dobdc), and mmen–Mg₂(dobpdc) is also stable under humid conditions.³² The impressive CO₂ capture performance of mmen–Mg₂(dobpdc) and other mmen–M₂(dobpdc) frameworks stems from a unique cooperative CO₂ capture mechanism, which has been shown to persist even in the presence of H₂O, based on infrared spectroscopy measurements.³² Interestingly, multicomponent adsorption measurements additionally show that the amount of adsorbed CO₂ from a mixture of CO₂, N₂, and H₂O in mmen–Mg₂(dobpdc) is slightly higher than that from pure CO₂ in mmen–Mg₂(dobpdc).

In view of its exceptional CO₂ capture performance, we seek to understand quantitatively the properties of mmen–M₂(dobpdc) using accurate first-principle density function theory (DFT) calculations. DFT is the most promising method for studying the adsorption (and related) properties of MOFs at the molecular level, owing to its efficiency and accuracy relative to other quantum mechanical methods. In previous theoretical studies, van der Waals (vdW)-corrected DFT in particular has been shown to accurately predict the binding energies and mechanisms of small gas molecules in MOFs,^{28,29,31,39–42} including MOFs having localized metal 3d electrons and non-zero spin moments.^43,44 Other properties, such as mechanical strength, can be predicted with similar accuracy.⁴⁵ For mmen–M₂(dobpdc), the CO₂ adsorption mechanism and CO₂ interactions with open metal sites in the form of carbamate have also been successfully studied with DFT methods.^37,38,46,47

Prior DFT-based studies notwithstanding,^{37,38,41,46–51} a complete and detailed understanding of the CO₂ adsorption energetics in mmen–M₂(dobpdc) is still lacking. In particular, the contributions of vdW dispersion interactions to adsorption enthalpies and related properties of mmen–M₂(dobpdc) have yet to quantified, and thus a predictive approach for adsorption energies in these complex systems does not yet exist. Moreover, the strength of electrostatic interactions between the ammonium carbamate units formed upon CO₂ adsorption and the effect of such interactions on key macroscopic observables, such as mechanical properties, have yet to be quantified and understood. Here, we use vdW-corrected DFT calculations to demonstrate a quantitative approach to predict binding and formation energies in this important class of MOFs. We further quantify the significant electrostatic interactions between ammonium carbamate units; compute and understand the effect of adsorption on mechanical properties; and determine the binding site of H₂O on the ammonium carbamate chains and evaluate the influence of humidity on the overall CO₂ performance.

2 Methodology

2.1 Computational details

In order to elucidate the role of mmen ligands in the CO₂ capture properties of mmen–M₂(dobpdc), we perform first-principles DFT calculations within the generalized gradient approximation (GGA) of Perdew, Burke, and Ernzerhof (PBE).⁵² We use a plane-wave basis and projector augmented-wave (PAW)^53,54 pseudopotentials with the Vienna ab initio Simulation Package (VASP) code.^55–58 To include the effect of the vdW dispersive interactions on binding energies and mechanical properties, we perform structural relaxations with vdW dispersion-corrected functionals (vdW-DF2)⁵⁹ as implemented in VASP. The initial structures for the MOFs we consider here are obtained from previous studies.^37,38,51,60 For all calculations, we use (i) a Γ-point sampling of the Brillouin zone and (ii) a 600 eV plane-wave cutoff energy. We explicitly treat two valence electrons for Mg (3s²), seven for Mn (3d⁵4s²), eight for Fe (3d⁶4s²), nine for Co (3d⁷4s²), twelve for Zn (3d¹⁰4s²), six for O (2s²2p⁴), five for N (2s²2p³), four for C (2s²2p²), and one for H (1s¹). All structural relaxations are performed with a Gaussian smearing of 0.05 eV ⁶¹ and with the structure constrained to the space group P3₂21. The computed CO₂ binding energies are within 0.4 kJ mol⁻¹ when we relax these symmetry constraints. The ions are relaxed until the Hellmann–Feynman forces are less than 0.02 eV Å⁻¹. The convergence threshold for self-consistency is 10⁻⁵ eV. For phonon frequency calculations, we use a more rigorous criterion (10⁻⁸ eV) (see Table S4†). Hubbard U values of 5.5 eV, 6.5 eV, and 5.3 eV for Mn, Fe, and Co 3d states are chosen following previous studies for M₂(dobdc) (M = Ti, V, Cr, Mn, Fe, Co, Ni, Cu).⁴⁴ Based on the ground state magnetic structure of Fe₂(dobdc),^21,62 we use ferromagnetic ordering along the metal oxide chain direction and antiferromagnetic ordering between the chains for mmen–M₂(dobpdc) (M = Mn, Fe, Co). Our computed electronic structure and measured adsorption spectra are given in the ESI.†

To compute CO₂ binding energies, we optimize mmen–M₂(dobpdc) prior to CO₂ adsorption (E_mmen–MOF), interacting with CO₂ in the gas phase (E_CO2) within a 15 Å × 15 Å × 15 Å cubic supercell, and mmen–M₂(dobpdc) with adsorbed CO₂ molecules (E_{CO2–mmen–MOF}) using vdW-corrected DFT. The binding energies (E_B) are obtained via the difference

−E_B = E_{CO2–mmen–MOF} − (E_mmen–MOF + E_CO2). (1)

We also consider zero-point energy (ZPE) and thermal energy (TE) corrections to compare computed binding energies with experimentally determined CO₂ heats of adsorption, following a previous DFT study.²⁸ We calculate vibrational frequencies of bound CO₂, H₂O, N₂, mmen, and CO₂–mmen in the framework; we also compute vibrational frequencies of free CO₂, H₂O, N₂, mmen, and CO₂–mmen molecules within a 15 Å × 15 Å × 15 Å cubic supercell. In the former case, we assume that changes in the frequency of framework phonon modes are small relative to those of molecular modes. All ZPE and TE corrections are computed at 298 K. All computed Kohn–Sham energies, vibrational frequencies, ZPE, and TE corrections are given in Tables S2, S3, S5, and S6 in the ESI.†

To calculate mechanical properties, we generate the stress tensor with (i) the Γ-point, (ii) a 1000 eV plane-wave cutoff energy, and (iii) a 0.01 eV Å⁻¹ force criterion. The linear elastic properties are then obtained using Hooke's law, which describes the relationship between stress, σ, and strain, ε:

σ_i = C_ijε_j (2)

where the C_ij are components of the single crystal elastic stiffness tensor. Here, we adopt the standard Voigt notation, with C_ij calculated as follows.⁶³ First, we fully relax the unit cell, optimizing all internal coordinates; we then apply a series of strains to this optimized hexagonal unit cell. Two different strain types are applied: ε₁ and ε₃₊₄ (see ESI†). For each strain, five different amplitudes of deformation are used: 0%, ±0.5%, and ±1%. Second, we relax atoms while fixing the deformed lattice parameters. By doing so, we obtain stress tensors σ_ij for all applied strains ε_ij. Third, C_ij are obtained from linear least squares fitting using the stress–strain relationship (the computed C_ij are given in the ESI†). Then, we calculate the single crystal elastic compliant constant S_ij as:

S_ij = C_ij⁻¹. (3)

After obtaining C_ij and S_ij, the orientationally-averaged elastic moduli including Young's modulus E, bulk modulus B, shear modulus G, and Poissons ratio v can be simply estimated using the Voigt–Reuss–Hill (VRH) average.⁶⁴ The detailed formulae used for all reported quantities in this work are given in the ESI.†

3 Results and discussion

3.1 CO₂ binding energies

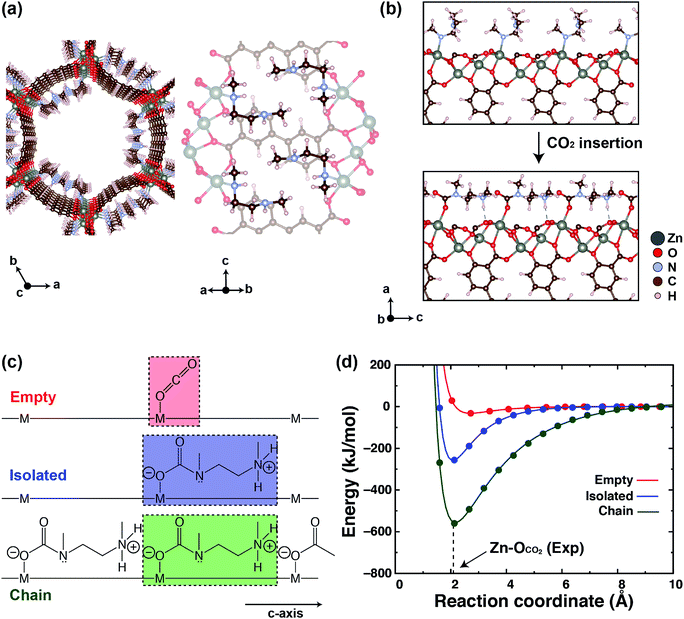
Fig. 1a depicts the optimized crystal structure of mmen–Zn₂(dobpdc), which consists of a periodic arrangement of ZnO₅ square pyramidal units, dobpdc⁴⁻ linkers, and mmen ligands crystallizing in the P3₂21 space group. A right panel of Fig. 1a shows that dangling amines interact with neighbors in the ab-plane via dispersive interactions between methyl groups. These interactions are reflected in the experimentally-determined crystal structure of mmen–Zn₂(dobpdc).⁶⁰ Our calculated lattice parameters for mmen–M₂(dobpdc) (M = Mg, Mn, Fe, Co, Zn) are given in Table 1. We do not include results for mmen–Ni₂(dobpdc) because the CO₂ capture mechanism of this framework is still under investigation. We note that our computed lattice parameters are not in perfect agreement with the experimental values, as expected. As the reparametrized PW86 exchange functional in the vdW-DF2 exchange–correlation functional overestimates repulsive interactions, equilibrium unit cell volumes, and bond distances,^59,65,66 the computed lattice parameters and unit cell volumes of M₂(dobpdc), CO₂–mmen–Mn₂(dobpdc), and CO₂–mmen–Zn₂(dobpdc) are generally larger than the experimental values as shown in Table 1. In addition, our calculations with periodic boundary conditions do not capture any disorder associated with the mmen and CO₂–mmen units shown in Fig. S3.† Any degree of disorder in these mmen and CO₂–mmen units can alter the average structural properties including the lattice parameters, unit cell volumes, and bond lengths. As example of this, our DFT calculations show that a different mmen ordering of mmen–Fe₂(dobpdc) significantly changes the a- and c-axes – by about 0.7 Å and 0.4 Å, respectively – and the unit cell volume by 12.5% (see Fig. S4†). Moreover, according to the previous experimental study by some of us,⁶⁰ disordered solvent (toluene) is likely present in the pores of the single-crystal structure of mmen–Zn₂(dobpdc), for which structural data are collected using a solvated crystal; furthermore, the CO₂-inserted single crystal structure of mmen–Zn₂(dobpdc) was refined to have 75% occupancy of ammonium carbamate chains, indicating the presence of solvent, CO₂, or water at the remaining Zn(II) sites. Hence, these features are mainly responsible for the discrepancies. Nevertheless, despite the presence of some disorder, our vdW-corrected DFT calculations accurately predict the CO₂ binding energies (see Table 2) and give the powder diffraction patterns in good overall agreement with the experiments as shown in Fig. S5 and S6.†

Fig. 1 (a) The optimized crystal structure of mmen–Zn₂(dobpdc). (b) Well-ordered ammonium carbamate chain formed upon CO₂ insertion. (c) Depictions of empty, isolated, and chain geometries along the channel direction (c-axis in the P3₂21 setting). (d) Potential curves of empty, isolated, and chain geometries compared to the experimental Zn–O_CO2 distance indicated by the dotted line.

Table 1 Computed lattice parameters, M–N, and M–O distances for M₂(dobpdc), mmen–M₂(dobpdc), and CO₂–mmen–M₂(dobpdc) (M = Mg, Mn, Fe, Co, Zn) compared to experimental values (unit: Å).^37,51,60 All structures are characterized in the space group P3₂21

M		This work			Experiment
		Empty	mmen–M	CO2–mmen–M	Empty	mmen–M	CO2–mmen–M
Mg	a	22.041	21.074	21.498	21.446	—	—
	c	6.939	6.672	7.005	6.824	—	—
	Mg–N	—	2.421	—	—	—	—
	Mg–O	—	—	2.072	—	—	—
Mn	a	22.253	22.650	21.788	21.629	21.729	21.682
	c	7.162	6.536	7.072	6.958	7.128	7.079
	Mn–N	—	2.432	—	—	2.289	—
	Mn–O	—	—	2.194	—	—	2.097
Fe	a	22.230	21.387	21.890	21.848	—	—
	c	6.963	6.622	7.013	6.814	—	—
	Fe–N	—	2.408	—	—	—	—
	Fe–O	—	—	2.195	—	—	—
Co	a	22.086	21.263	21.639	21.537	—	—
	c	6.920	6.540	7.005	6.798	—	—
	Co–N	—	2.324	—	—	—	—
	Co–O	—	—	2.147	—	—	—
Zn	a	22.087	21.683	21.881	21.547	21.391	21.546
	c	6.973	7.251	6.833	6.775	6.896	6.928
	Zn–N	—	2.212	—	—	2.155	—
	Zn–O	—	—	2.127	—	—	2.087

---

Table 2 A comparison of computed CO₂ binding energies (E_B) and enthalpies (H_B) (in kJ mol⁻¹) in mmen–M₂(dobpdc) (M = Mg, Mn, Fe, Co, Zn) with the experimental values at a CO₂ loading of 2 mmol g⁻¹.³⁷ Zero-point energy (ZPE) and thermal energy (TE) corrections of ammonium carbamate and mmen are considered. All ZPE and TE values are computed at 298 K

	This work				Exp
	E B	ZPE	TE	H B	H B
Mg	74.7	−2.8	1.1	73.0	71
Mn	68.9	−2.2	0.6	67.3	67
Fe	56.2	−1.9	0.7	55.1	58
Co	52.4	−1.3	0.4	51.6	52
Zn	62.4	−1.5	1.3	62.1	57

---

Previous experimental^32,37 and computational studies³⁸ demonstrated that mmen–M₂(dobpdc) (M = Mg, Mn, Fe, Co, Zn) exhibit step-like isotherms above a threshold CO₂ partial pressure, and that these steps originate from a mechanism wherein CO₂ gas molecules cooperatively and reversibly insert into metal–mmen bonds followed by formation of a well-ordered ammonium carbamate chain structure, as shown in Fig. 1b. Table 2 compares the computed CO₂ binding enthalpies (H_B) from this study with those obtained from experiment,³⁷ revealing that our vdW-DF2 calculations can accurately predict CO₂ binding enthalpies within ∼5 kJ mol⁻¹. According to our calculations, in all cases MOFs with mmen exhibit an ∼30 kJ mol⁻¹ enhancement in H_B compare to the unfunctionalized frameworks (see Tables 2 and 4).

To understand this increase, we performed calculations using three geometries as presented in Fig. 1c. We use the term “Empty” to indicate unappended M₂(dobpdc) and “Chain” to indicate the structure with well-ordered ammonium carbamate chains along the channel direction (c-axis in the space group P3₂21). The term “Isolated” refers to an alternative scenario in which there is no chain formation, and the calculations consider an ammonium carbamate unit bound at just one of the metal sites in the unit cell, with the other metal sites empty.

We then compute the potential interatomic distance curves for the three geometries, varying the separation between CO₂ and its binding site, by adopting a a × b × 2c supercell for the isolated and chain geometries. Fig. 1d shows the calculated energy as a function of Zn–O_CO2 distance, relative to the experimental Zn–O_CO2 distance of 2.087(4) Å for the chain geometry. Similar curves are given for M = Mg, Mn, Fe, Co in Fig. S7 in the ESI.† To calculate these curves, we systematically displace a CO₂ molecule and ammonium carbamate along the direction of the ground state Zn–O bond to CO₂.

In our DFT calculations, the vdW-DF2 functional slightly overestimates (by ∼2%) the Zn–O distance (2.127 Å) compared with experiment (2.087(4) Å), as reported in previous DFT studies.^39,66 In Fig. 1d, the isolated and chain geometries have much deeper binding curves with higher curvature when compared to the empty geometry, reflecting the fact that the binding strength of ammonium carbamate to the MOF interior is much stronger than that of CO₂ alone. The binding enthalpy of CO₂ in the empty geometry is 29.3 kJ mol⁻¹, which is comparable to the values computed and experimentally measured for Zn₂(dobdc) (30.2 kJ mol⁻¹ and 26.8 ± 0.1 kJ mol⁻¹, respectively).^28,29 On the other hand, the binding enthalpy of an ammonium carbamate (H_iso) in the isolated geometry is 259.0 kJ mol⁻¹, approximately nine times that of the CO₂ binding enthalpy. More interestingly, the ammonium carbamate binding enthalpy (H_chain) in the chain geometry is 562.1 kJ mol⁻¹, which is two times larger than that of the isolated geometry. The other MOFs also show similar behavior (see Fig. S7, ESI†). Thus the magnitude of the electrostatic interaction between two ammonium carbamate units in the chain geometry is equal to 151.5 kJ mol⁻¹ (=(H_chain − H_iso)/2) (because ammonium carbamate unit interacts with two neighboring CO₂–mmen sites along the pore axis, we divide by two). This large interaction energy is dominated by the ion pairing and hydrogen bonding interactions of the ammonium group to the unbound oxygen atom of the neighboring carbamate. In magnitude, these interactions are comparable to the heat of formation for ammonium carbamate (∼152 kJ mol⁻¹).⁶⁷ The results of these calculations show that cooperative CO₂ insertion is a very favorable spontaneous process, that the carbamate chain geometry is quite stable, and that electrostatic interactions play a key role in the stability of CO₂–mmen–M₂(dobpdc) (chain geometry).

The apparent enhancement of CO₂ binding strength by the presence of mmen can be qualitatively understood by considering how charges rearrange at the metal sites upon CO₂ binding. Fig. 2 shows the charge density difference (Δρ = ρ_MOF+molecule − (ρ_MOF + ρ_molecule)) isosurface plots for CO₂–mmen binding sites in the isolated and chain geometries. The Δρ value calculated for the empty geometry is negligible compared to the values of the isolated and chain geometries at the same isosurface level (see Fig. S8, ESI†). In the isolated geometry, two sites are visible with prominent charge redistribution. One is the Zn–O (carbamate) bond formed upon CO₂ insertion into the Zn–mmen bond, and the other is N–H⋯O, which corresponds to hydrogen bonding between the ammonium group of ammonium carbamate and the non-bridging carboxylate oxygen atom on the dobpdc⁴⁻ linker (Fig. 2a). More interestingly, in the case of the chain geometry there are two additional sites in which ammonium carbamate units interact with their neighbors along the channel direction, as shown in the inset of Fig. 2b. These additional attractive interactions deepen the potential curve compared to the isolated structure (Fig. 1d). Our calculations therefore suggest that strong electrostatic interactions between ammonium carbamate units primarily drive the cooperative CO₂ insertion in mmen–M₂(dobpdc) and significantly enhance the CO₂ binding strength over non-amine-functionalized MOFs.

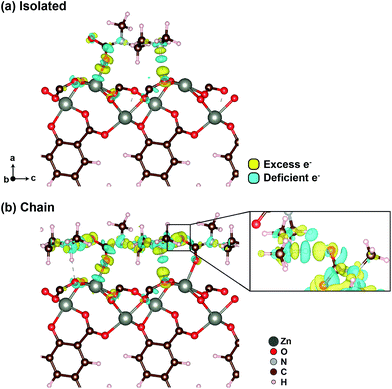Fig. 2 Isosurface plots of the charge density difference (Δρ) for the CO₂–mmen binding site in (a) isolated and (b) chain structures. The isosurface level is equal to 0.02 e Å⁻³.

3.2 Mechanical properties

Having quantified the CO₂ binding enhancement afforded by mmen, we now address the mechanical properties of empty and CO₂-loaded MOFs, which can be calculated via the elastic modulus C and the curvature of the binding curves in the linear elastic regime,^68,69 where E_tot is the total energy and r is reaction coordinate. From the binding energy curves shown in Fig. 1d and S7,† we expect that elastic moduli of mmen–M₂(dobpdc) and CO₂–mmen–M₂(dobpdc) will be much larger than that of M₂(dobpdc), and that CO₂ adsorption and functionalization can strongly and reversibly alter these quantities. This is because the elastic modulus C is directly proportional to the curvature of the binding curves . Our calculations of the Young's modulus E, bulk modulus B, shear modulus G, and Poisson's ratio v for M₂(dobpdc), mmen–M₂(dobpdc), and CO₂–mmen–M₂(dobpdc) are summarized in ESI Section 5.† In Fig. 3, we illustrate three-dimensional contours of directionally-dependent values of E for all the frameworks considered. In general, mmen and ammonium carbamate units greatly enhance E along all directions. The most prominent enhancement occurs along the channel direction, along which the ammonium carbamate chains run.

Fig. 3 Three-dimensional contour of directionally-dependent Young's moduli E for (a) Mg–MOFs, (b) Mn–MOFs, (c) Fe–MOFs, (d) Co–MOFs, and (e) Zn–MOFs. Left, center, and right panels correspond to M₂(dobpdc), mmen–M₂(dobpdc), and CO₂–mmen–M₂(dobpdc), respectively. The z-axis or [001] direction is the channel direction.

We now examine more quantitatively the effect of mmen and ammonium carbamate units on E, B, G, and v. The orientationally-averaged E, B, G, and v values obtained from eqn (1)–(8) in the ESI† are summarized in Table 3. Averaged over all directions, these values can be considered as elastic moduli of polycrystalline samples with randomly-oriented grains of equal volume fraction. As shown in Fig. 4, E, B, and G are generally larger for mmen–M₂(dobpdc) and CO₂–mmen–M₂(dobpdc) than for M₂(dobpdc), an enhancement that can be attributed to mmen and ammonium carbamate units, and this is most pronounced for E and G. For example, the magnitude of E for mmen–Zn₂(dobpdc) increases by 112% compared to that of Zn₂(dobpdc). More remarkably, the magnitude of E for CO₂–mmen–Mn₂(dobdpc) increases by 141% compared to that of Mn₂(dobpdc) (see Table 3). All E values for mmen–M₂(dobdpc) and CO₂–mmen–M₂(dobpdc) (10.7–16.7 GPa) are higher than the experimental values of conventional frameworks such as MOF-5 (2–8 GPa), HKUST-1 (6 GPa), and ZIFs (2–9 GPa), and lower than that of the hybrid MOF MOFP-1 (23–27 GPa).^70–72 For G, the enhancement is even larger than that of E, for instance the G values of mmen–Zn₂(dobpdc) and CO₂–mmen–Mn₂(dobpdc) increase by 124% and 159% compared to Zn₂(dobpdc) and Mn₂(dobpdc). All G values (4.0–6.4 GPa) are comparable to those of Zr-UiO-67 (5.69 GPa) and Zr-UiO-68 (4.18 GPa).⁷³

Table 3 Computed orientationally-averaged Young's modulus E (in GPa), bulk modulus B (in GPa), shear modulus G (in GPa), Poisson's ratio v, and enhancement of E and G for M₂(dobpdc), mmen–M₂(dobpdc), and CO₂–mmen–M₂(dobpdc)

	E	B	G	v	Enhancement
					E	G
Mg	8.97	10.05	3.32	0.35
mmen–Mg	14.10	10.49	5.53	0.28	57%	67%
CO2–mmen–Mg	16.66	14.52	6.37	0.31	86%	92%
Mn	6.42	11.98	2.28	0.41
mmen–Mn	10.72	10.36	4.04	0.33	67%	77%
CO2–mmen–Mn	15.48	13.66	5.90	0.31	141%	159%
Fe	8.63	9.69	3.19	0.35
mmen–Fe	13.56	9.55	5.37	0.26	57%	68%
CO2–mmen–Fe	13.64	14.22	5.09	0.34	58%	59%
Co	6.95	8.51	2.55	0.36
mmen–Co	10.66	8.24	4.15	0.28	53%	63%
CO2–mmen–Co	15.05	13.51	5.73	0.31	117%	125%
Zn	6.89	10.28	2.48	0.39
mmen–Zn	14.58	13.03	5.55	0.31	112%	124%
CO2–mmen–Zn	14.02	15.28	5.21	0.35	103%	110%

---

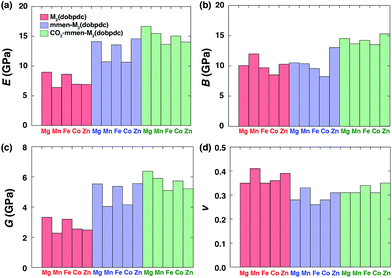Fig. 4 The computed orientationally-averaged (a) Young's modulus E, (b) bulk modulus B, (c) shear modulus G, and (d) Poisson's ratio v values for all frameworks under consideration.

Our calculations thus show that mmen and CO₂ binding play a key role in the enhancement of the mechanical properties of the M₂(dobpdc) framework materials. Moreover, these results demonstrate that the mechanical properties of MOFs can be tuned by functionalization with ligands such as mmen. Importantly, this bears direct relevance to CO₂ capture applications, since carbon capture materials must be mechanically robust and should not exhibit mechanical fatigue during CO₂ uptake and release cycling. Relatedly, it has been shown that functionalization with ligands such as mmen can significantly reduce plasticization and enhance selectivity in membrane-type devices.^74–76

3.3 CO₂ selectivity

In previous multicomponent adsorption measurements incorporating humidity,³² mmen–Mg₂(dobpdc) and mmen–Ni₂(dobpdc) maintained high CO₂ selectivity, while Mg₂(dobdc) and Ni₂(dobdc) exhibited poor CO₂ capture performance under humid conditions. Mason et al. demonstrated that H₂O does not alter the cooperative CO₂ capture mechanism in mmen–Mg₂(dobpdc),³² showing that instead mmen–Mg₂(dobpdc) maintains a significant CO₂ capacity under multicomponent equilibrium conditions. However, the structural and energetic influence of H₂O on CO₂ adsorption in mmen–M₂(dobpdc) remains unknown.

To address this question, we computed H_B for CO₂, H₂O, and N₂ in the M₂(dobpdc) and mmen–M₂(dobpdc) frameworks. Table 4 shows the computed enthalpies of first and second guest molecules (CO₂, H₂O, and N₂) in the frameworks with and without mmen functionalization. In the bare frameworks, water has the highest H_B among all three guest molecules at open metal sites. Furthermore, H₂O also has the highest H_B at secondary binding sites when it has occupied the open metal sites.

Table 4 Computed binding enthalpies H_B (kJ mol⁻¹) of first (CO₂, H₂O, N₂, and CO₂–mmen) and second guest molecules (CO₂, H₂O, and N₂) in M₂(dobpdc) and mmen–M₂(dobpdc) (M = Mg, Mn, Fe, Co, Zn)

	First guest	H B	Second guest	H B
Mg	H2O	62.6	H2O	39.4
			CO2	25.3
			N2	16.0
	CO2	38.5
	N2	27.3
			H2O	40.9
	CO2–mmen–Mg	597.0	CO2	26.3
			N2	11.2
Mn	H2O	54.6	H2O	42.9
			CO2	26.6
			N2	18.0
	CO2	34.7
	N2	23.6
			H2O	43.3
	CO2–mmen–Mn	582.2	CO2	29.4
			N2	14.6
Fe	H2O	53.9	H2O	42.8
			CO2	20.3
			N2	11.5
	CO2	35.6
	N2	24.6
			H2O	40.1
	CO2–mmen–Fe	588.4	CO2	25.3
			N2	9.5
Co	H2O	53.0	H2O	38.7
			CO2	23.8
			N2	10.9
	CO2	34.9
	N2	22.4
			H2O	39.5
	CO2–mmen–Co	570.3	CO2	25.7
			N2	10.6
Zn	H2O	44.2	H2O	38.6
			CO2	23.2
			N2	16.7
	CO2	29.3
	N2	18.6
			H2O	40.5
	CO2–mmen–Zn	562.1	CO2	23.7
			N2	8.2

---

Fig. 5a shows the most stable computed configuration of Zn₂(dobpdc) in the presence of H₂O without mmen. Here, the secondary H₂O interacts with the first Zn-bound H₂O via hydrogen bonding. Previous multicomponent measurements^32,77 on the smaller pore MOFs Mg₂(dobdc), Co₂(dobdc), and Ni₂(dobdc) are consistent with this configuration. In ref. 32, in the case of Mg₂(dobdc), the amount of CO₂ adsorbed was 0.5 mmol g⁻¹, while adsorbed H₂O reached over 15 mmol g⁻¹ (1.8 mmol H₂O per mmol Mg). Moreover, the amount of CO₂ adsorbed in Ni₂(dobdc) is almost zero while that of H₂O is over 20 mmol g⁻¹ (3.1 mmol H₂O per mmol Ni). Therefore, water molecules significantly degrade CO₂ selectivity in M₂(dobdc) frameworks, and our calculations show that the larger-pore M₂(dobpdc) frameworks will exhibit the same behavior (see Table 4).

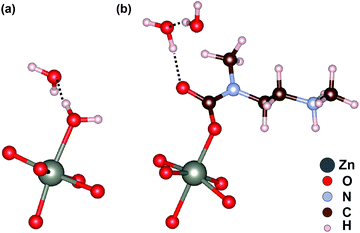Fig. 5 The most stable configurations of (a) Zn₂(dobpdc) and (b) mmen–Zn₂(dobpdc) in the presence of a mixture of CO₂, H₂O, and N₂.

In contrast, our calculations indicate that CO₂ insertion to form an O-bound carbamate for mmen–M₂(dobpdc) frameworks is much more favorable than binding H₂O at the open metal site of the corresponding bare M₂(dobpdc) frameworks. As shown in Table 4, the ammonium carbamate binding enthalpies, H_chain including ZPE and TE corrections, are about ten times higher than those for H₂O for all M₂(dobpdc) frameworks. Thus, the mmen–M₂(dobpdc) frameworks are predicted to maintain their unique CO₂ capture mechanism even under humid conditions. Interestingly, our energetics suggest that H₂O molecules bind near the negatively charged end of the carbamate via hydrogen bonding interactions (Fig. 5b). This configuration also explains the large amount of H₂O adsorbed from the mixture of CO₂, H₂O, and N₂ in CO₂–mmen–Mg₂(dobpdc).³² In previous multicomponent measurements with mmen–Mg₂(dobpdc), the amount of H₂O adsorbed from the mixture was about 7 mmol g⁻¹ (1.7 H₂O per mmen–Mg), while CO₂ adsorbed from the mixture was about 4.2 mmol g⁻¹ (1.0 CO₂ per mmen–Mg). In fact, we find that accumulated H₂O increases the CO₂H_B by an amount equal to the H₂O binding strength. For example for mmen–Mn₂(dobpdc), the binding energy of the second adsorbed H₂O molecule hydrogen-bonded to non-bonded O ion on the carbamate via hydrogen bonding (Fig. 5b) is as high as 43.3 kJ mol⁻¹. Thus, the additional hydrogen bonding in the presence of H₂O can further stabilize the CO₂H_B by the same amount (=43.3 kJ mol⁻¹). We speculate that this may be related to an increase of the amount of CO₂ adsorbed in the presence of H₂O.³² As a result, our computed energetics demonstrate that instead of hampering the CO₂ capture process, H₂O plays a crucial role in affording stability to CO₂–mmen–M₂(dobpdc).

4 Conclusions

We have examined the effect of mmen on the binding enthalpies and mechanical properties of mmen–M₂(dobpdc) (M = Mg, Mn, Fe, Co, Zn), finding that mmen ligands enhance the CO₂H_B and selectivity under humid conditions, and that mmen and CO₂–mmen ligands significantly enhance framework mechanical properties. These results elucidate the energetics of individual interactions underlying the cooperative CO₂ insertion mechanism in mmen–M₂(dobpdc) under dry and humid conditions. Furthermore, our results demonstrate the remarkable increase in mechanical properties afforded by functionalization of M₂(dobpdc) with diamines. Overall, our work highlights the unique advantages in CO₂ capture performance and physical properties accessible with diamine-appended frameworks.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

This work was supported by the Center for Gas Separations Relevant to Clean Energy Technologies, an Energy Frontier Research Center, funded by the U.S. Department of Energy, Office of Science, Office of Basic Energy Sciences, under Award DE-SC0001015. Work at the Molecular Foundry was supported by the Office of Science, Office of Basic Energy Sciences, U.S. Department of Energy, under Contract DE-AC02-05CH11231, and computational resources were provided by DOE (LBNL Lawrencium and NERSC).

Notes and references

1. A. K. Cheetham, G. Férey and L. Thierry, Angew. Chem., Int. Ed., 1999, 38, 3268–3292.
2. S. L. James, Chem. Soc. Rev., 2003, 32, 276–288.
3. H. C. Zhou, J. R. Long and O. M. Yaghi, Chem. Rev., 2012, 112, 673–674.
4. J. L. C. Rowsell and O. M. Yaghi, Microporous Mesoporous Mater., 2004, 73, 3–14.
5. H. Furukawa, K. Cordova, M. O'Keeffe and O. M. Yaghi, Science, 2013, 341, 1230444.
6. M. L. Foo, R. Matsuda and S. Kitagawa, Chem. Mater., 2014, 26, 310–322.
7. G. Férey, Dalton Trans., 2009, 4400–4415.
8. O. K. Farha and J. T. Hupp, Acc. Chem. Res., 2010, 43, 1166–1175.
9. K. K. Tanabe and S. M. Cohen, Chem. Soc. Rev., 2011, 40, 498–519.
10. J. R. Long and O. M. Yaghi, Chem. Soc. Rev., 2009, 38, 1213–1214.
11. N. L. Rosi, J. Eckert, M. Eddaoudi, D. T. Vodak, J. Kim, M. O'Keeffe and O. M. Yaghi, Science, 2003, 73, 12–15.
12. A. R. Millward and O. M. Yaghi, J. Am. Chem. Soc., 2005, 127, 17998–17999.
13. K. Sumida, D. L. Rogow, J. A. Mason, T. M. McDonald, E. D. Bloch, Z. R. Herm, T.-H. Bae and J. R. Long, Chem. Rev., 2012, 112, 724–781.
14. J. Liu, P. K. Thallapally, B. P. McGrail, D. R. Brown and J. Liu, Chem. Soc. Rev., 2012, 41, 2308–2322.
15. T. A. Makal, J.-R. Li, W. Lu and H.-C. Zhou, Chem. Soc. Rev., 2012, 41, 7761–7779.
16. International Energy Agency, CO₂ Emissions From Fuel Combustion Highlights, 2015.
17. F. Joos, G.-K. Plattner, T. F. Stocker, O. Marchal and A. Schmittner, Science, 1999, 284, 464–467.
18. P. M. Cox, R. A. Betts, C. D. Jones, S. A. Spall and J. Totterdell, Nature, 2000, 408, 184–187.
19. W. Zhou, H. Wu and T. Yildirim, J. Am. Chem. Soc., 2008, 2, 15268–15269.
20. S. R. Caskey, A. G. Wong-Foy and A. J. Matzger, J. Am. Chem. Soc., 2008, 130, 10870–10871.
21. E. D. Bloch, L. J. Murray, W. L. Queen, S. Chavan, S. N. Maximoff, J. P. Bigi, R. Krishna, V. K. Peterson, F. Grandjean, G. J. Long, B. Smit, S. Bordiga, C. M. Brown and J. R. Long, J. Am. Chem. Soc., 2011, 133, 14814–14822.
22. R. Sanz, F. Martínez, G. Orcajo, L. Wojtas and D. Briones, Dalton Trans., 2013, 42, 2392–2398.
23. W. L. Queen, C. M. Brown, D. K. Britt, P. Zajdel, M. R. Hudson and O. M. Yaghi, J. Phys. Chem. C, 2011, 115, 24915–24919.
24. D. J. Xiao, E. D. Bloch, J. A. Mason, W. L. Queen, M. R. Hudson, N. Planas, J. Borycz, A. L. Dzubak, P. Verma, K. Lee, F. Bonino, V. Crocella, J. Yano, S. Bordiga, D. G. Truhlar, L. Gagliardi, C. M. Brown and J. R. Long, Nat. Chem., 2014, 6, 590–595.
25. P. D. C. Dietzel, Y. Morita, R. Blom and H. Fjellvåg, Angew. Chem., Int. Ed., 2005, 44, 6354–6358.
26. P. D. C. Dietzel, B. Panella, M. Hirscher, R. Blom and H. Fjellvåg, Chem. Commun., 2006, 1, 959–961.
27. Y. Liu, H. Kabbour, C. M. Brown, D. A. Neumann and C. C. Ahn, Langmuir, 2008, 24, 4772–4777.
28. K. Lee, J. D. Howe, L.-C. Lin, B. Smit and J. B. Neaton, Chem. Mater., 2015, 27, 668–678.
29. W. L. Queen, M. R. Hudson, E. D. Bloch, J. A. Mason, M. I. Gonzalez, J. S. Lee, D. Gygi, J. D. Howe, K. Lee, T. A. Darwish, M. James, V. K. Peterson, S. J. Teat, B. Smit, J. B. Neaton, J. R. Long and C. M. Brown, Chem. Sci., 2014, 5, 4569–4581.
30. J. Yu and P. B. Balbuena, J. Phys. Chem. C, 2013, 117, 3383–3388.
31. K. Tan, S. Zuluaga, Q. Gong, Y. Gao, N. Nijem, J. Li, T. Thonhauser and Y. J. Chabal, Chem. Mater., 2015, 27, 2203–2217.
32. J. A. Mason, T. M. McDonald, T.-H. Bae, J. E. Bachman, K. Sumida, J. J. Dutton, S. S. Kaye and J. R. Long, J. Am. Chem. Soc., 2015, 137, 4787–4803.
33. S. Zuluaga, E. M. A. Fuentes-Fernandez, K. Tan, F. Xu, J. Li, Y. J. Chabal and T. Thonhauser, J. Mater. Chem. A, 2016, 4, 5176–5183.
34. J. Kundu, T. Pascal, D. Prendergast and S. Whitelam, Phys. Chem. Chem. Phys., 2016, 18, 21760–21766.
35. T. C. Drage, C. E. Snape, L. A. Stevens, J. Wood, J. Wang, A. I. Cooper, R. Dawson, X. Guo, C. Satterley and R. Irons, J. Mater. Chem., 2012, 22, 2815–2823.
36. T. M. McDonald, W. R. Lee, J. a. Mason, B. M. Wiers, C. S. Hong and J. R. Long, J. Am. Chem. Soc., 2012, 134, 7056–7065.
37. T. M. McDonald, J. A. Mason, X. Kong, E. D. Bloch, D. Gygi, A. Dani, V. Crocellà, F. Giordanino, S. O. Odoh, W. S. Drisdell, B. Vlaisavljevich, A. L. Dzubak, R. Poloni, S. K. Schnell, N. Planas, K. Lee, T. Pascal, L. F. Wan, D. Prendergast, J. B. Neaton, B. Smit, J. B. Kortright, L. Gagliardi, S. Bordiga, J. A. Reimer and J. R. Long, Nature, 2015, 519, 303–308.
38. B. Vlaisavljevich, S. O. Odoh, S. K. Schnell, A. L. Dzubak, K. Lee, N. Planas, J. B. Neaton, L. Gagliardi and B. Smit, Chem. Sci., 2015, 6, 5177–5185.
39. R. Poloni, B. Smit and J. B. Neaton, J. Phys. Chem. A, 2012, 116, 4957–4964.
40. P. Canepa, N. Nijem, Y. J. Chabal and T. Thonhauser, Phys. Rev. Lett., 2013, 110, 026102.
41. W. S. Drisdell, R. Poloni, T. M. McDonald, J. R. Long, B. Smit, J. B. Neaton, D. Prendergast and J. B. Kortright, J. Am. Chem. Soc., 2013, 135, 18183–18190.
42. B. Vlaisavljevich, J. Huck, Z. Hulvey, K. Lee, J. A. Mason, J. B. Neaton, J. R. Long, C. M. Brown, D. Alfé, A. Michaelides and B. Smit, J. Phys. Chem. A, 2017, 121, 4139–4151.
43. T. Thonhauser, S. Zuluaga, C. A. Arter, K. Berland, E. Schröder and P. Hyldgaard, Phys. Rev. Lett., 2015, 115, 136402.
44. G. W. Mann, K. Lee, M. Cococcioni, B. Smit and J. B. Neaton, J. Chem. Phys., 2016, 144, 174104.
45. J.-C. Tan, B. Civalleri, C.-C. Lin, L. Valenzano, R. Galvelis, P.-F. Chen, T. D. Bennett, C. Mellot-Draznieks, C. M. Zicovich-Wilson and A. K. Cheetham, Phys. Rev. Lett., 2012, 108, 095502.
46. N. Planas, A. L. Dzubak, R. Poloni, L. C. Lin, A. McManus, T. M. McDonald, J. B. Neaton, J. R. Long, B. Smit and L. Gagliardi, J. Am. Chem. Soc., 2013, 135, 7402–7405.
47. W. S. Drisdell, R. Poloni, T. M. McDonald, T. A. Pascal, L. F. Wan, C. D. Pemmaraju, B. Vlaisavljevich, S. O. Odoh, J. B. Neaton, J. R. Long, D. Prendergast and J. B. Kortright, Phys. Chem. Chem. Phys., 2015, 17, 21448–21457.
48. S. A. Didas, M. A. Sakwa-novak, G. S. Foo, C. Sievers and C. W. Jones, J. Phys. Chem. Lett., 2014, 5, 4194–4200.
49. W. R. Lee, H. Jo, L.-M. Yang, H. Lee, D. W. Ryu, K. S. Lim, J. H. Song, D. Y. Min, S. S. Han, J. G. Seo, Y. K. Park, D. Moon and C. S. Hong, Chem. Sci., 2015, 6, 3697–3705.
50. L. A. Darunte, K. S. Walton, D. S. Sholl and C. W. Jones, Curr. Opin. Chem. Eng., 2016, 12, 82–90.
51. D. Gygi, E. D. Bloch, J. A. Mason, M. R. Hudson, M. I. Gonzalez, R. L. Siegelman, T. A. Darwish, W. L. Queen, C. M. Brown and J. R. Long, Chem. Mater., 2016, 28, 1128–1138.
52. J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1996, 77, 3865–3868.
53. P. E. Blöchl, Phys. Rev. B: Condens. Matter Mater. Phys., 1994, 50, 17953–17979.
54. G. Kresse and D. Joubert, Phys. Rev. B: Condens. Matter Mater. Phys., 1999, 59, 1758–1775.
55. G. Kresse and J. Hafner, Phys. Rev. B: Condens. Matter Mater. Phys., 1993, 47, 558–561.
56. G. Kresse and J. Furthmüller, Phys. Rev. B: Condens. Matter Mater. Phys., 1996, 54, 11169–11186.
57. G. Kresse and J. Furthmüller, Comput. Mater. Sci., 1996, 6, 15–50.
58. G. Kresse and J. Hafner, Phys. Rev. B: Condens. Matter Mater. Phys., 1994, 49, 14251–14269.
59. K. Lee, É. D. Murray, L. Kong, B. I. Lundqvist and D. C. Langreth, Phys. Rev. B: Condens. Matter Mater. Phys., 2010, 82, 081101.
60. R. L. Siegelman, T. M. McDonald, M. I. Gonzalez, J. D. Martell, P. J. Milner, J. A. Mason, A. H. Berger, A. S. Bhown and J. R. Long, J. Am. Chem. Soc., 2017, 139, 10526–10538.
61. C. Elsässer, M. Fähnle, C. Chan and K. Ho, Phys. Rev. B: Condens. Matter Mater. Phys., 1994, 49, 13975–13978.
62. E. D. Bloch, W. L. Queen, R. Krishna, J. M. Zubairy, C. M. Brown and J. R. Long, Science, 2012, 335, 1606–1611.
63. Y. Le Page and P. Saxe, Phys. Rev. B: Condens. Matter Mater. Phys., 2002, 65, 104104.
64. R. Hill, Proc. Phys. Soc., London, Sect. A, 1952, 65, 349–354.
65. É. D. Murray, K. Lee and D. C. Langreth, J. Chem. Theory Comput., 2009, 5, 2754–2762.
66. I. Hamada, Phys. Rev. B: Condens. Matter Mater. Phys., 2014, 89, 121103.
67. K. G. Clark and H. C. Hetherington, J. Am. Chem. Soc., 1927, 49, 1909–1915.
68. D. A. Padmavathi, Mater. Sci. Appl., 2011, 22, 97–104.
69. Handbook of Advanced Ceramics, ed. S. Somiya, Academic Press, 2013.
70. J. C. Tan, T. D. Bennett and A. K. Cheetham, Proc. Natl. Acad. Sci. U. S. A., 2010, 107, 9938–9943.
71. J. C. Tan and A. K. Cheetham, Chem. Soc. Rev., 2011, 40, 1059–1080.
72. W. Li, A. Thirumurugan, P. T. Barton, Z. Lin, S. Henke, H. H. M. Yeung, M. T. Wharmby, E. G. Bithell, C. J. Howard and A. K. Cheetham, Phys. Rev. B: Condens. Matter Mater. Phys., 2014, 136, 7801–7804.
73. H. Wu, T. Yildirim and W. Zhou, J. Phys. Chem. Lett., 2013, 4, 925–930.
74. J. E. Bachman, Z. P. Smith, T. Li, T. Xu and J. R. Long, Nat. Mater., 2016, 15, 845–851.
75. J. E. Bachman and J. R. Long, Energy Environ. Sci., 2016, 9, 2031–2036.
76. L. Maserati, S. M. Meckler, J. E. Bachman, J. R. Long and B. A. Helms, Nano Lett., 2017, 17, 6828–6832.
77. R. Mercado, B. Vlaisavljevich, L.-C. Lin, K. Lee, Y. Lee, J. A. Mason, D. J. Xiao, M. I. Gonzalez, M. T. Kapelewski, J. B. Neaton and B. Smit, J. Phys. Chem. C, 2016, 120, 12590–12604.

---

Footnote

† Electronic supplementary information (ESI) available. See DOI: 10.1039/c7sc05217k

---