Towards a universal model for the foaming behavior of surfactants: a case study on per- and polyfluoroalkyl substances (PFAS)†
Muchu
Zhou
 and
Reza
Foudazi
and
Reza
Foudazi
 *
*
School of Sustainable Chemical, Biological and Materials Engineering, The University of Oklahoma, Norman, OK 73019, USA. E-mail: rfoudazi@ou.edu
First published on 23rd October 2024
Abstract
Foam fractionation offers a promising solution for the separation of surface-active contaminants from water. Therefore, this work aims to comprehensively investigate foaming behavior and its correlations with the interfacial properties. As a case study, we evaluate foaming of per- and polyfluoroalkyl substances (PFAS), which are one of significant environmental issues worldwide due their pervasive presence in the environment. Since there is no universal model to describe the foaming behavior of surfactants that can be applied to PFAS, this research utilizes dimensional analysis to establish a correlation between the foaming behavior of PFAS solutions—characterized by expansion rate of foaming—and dimensionless numbers that represent both processing and interfacial characteristics. Foaming parameters, such as gas flow rate and aeration time, are varied to study their effect on PFAS foamability. In addition, we study PFAS with different headgroups and with different chain lengths in the presence of electrolytes with different concentrations. Our study elucidates distinct, condition-specific equations for individual PFAS, revealing that long-chain PFAS foaming is significantly influenced by interfacial property-related dimensionless numbers, such as the Boussinesq number. Additionally, the Froude number and Weber number affect the foamability of both long- and short-chain PFAS. Moreover, our study identifies specific trends, including a maximum foaming capacity at a certain Capillary number, aligning with the maximum in dilatational interfacial modulus. The results suggest more studies are needed on bubble interaction and foam film behavior.
1. Introduction
Partially or fully fluorinated synthetic compounds, known as per- and polyfluoroalkyl substances (PFAS), have gained broad usage in numerous industries, such as non-stick coatings, aqueous film forming foams (AFFFs), cleaners, cosmetics, and semiconductors.1 This widespread adoption is attributed to their remarkable chemical and thermal stability, as well as their ability to repel both water and oil.2–6 However, the extensive utilization of PFAS, coupled with their notable stability attributed to the C–F bond, has resulted in their ubiquitous presence, emerging as a global environmental concern. In addition, there are significant concerns about the toxicity and bioaccumulation of PFAS.5,7–9 Exposure to PFAS is linked to an increased risk of obesity, reproductive dysfunction, elevated cholesterol, liver and kidney diseases, lipid and insulin dysregulation, and cancer.10–13One of the pathways for human exposure to PFAS is through PFAS-contaminated water sources, with a particular emphasis on drinking water.14–16 For example, the concentration ranges of perfluorooctanesulfonic acid (PFOS) and perfluorooctanoic acid (PFOA) in the United States (U.S.) drinking water are 0.04–1.80 ppt and 0.02–0.35 ppt, respectively.17 Perfluorobutanesulfonic acid (PFBS) has been found with a lower detection frequency of 0.05%, but with the mean concentrations of 212 and 136 ppt in surface water and ground water, respectively.16 In ground water, the concentration of perfluorohexanesulfonic acid (PFHxS) has been reported in 409 ppt to 1600 ppt range.16 The U.S. Environmental Protection Agency (EPA) has issued a Maximum Contaminant Levels (MCLs) and a Maximum Contaminant Level Goals (MCLGs) for PFOS and PFOA in drinking water at 4 and 0 ng L−1, respectively.18 Moreover, MCLs have been defined for other PFAS, e.g., 10 ng L−1 for PFHxS, perfluorononanoic acid (PFNA), and hexafluoropropylene oxide (HFPO) dimer acid and its ammonium salt (GenX).18 These substances have been employed as alternatives to long-chain PFAS.
In addition to water sources, discharge from industrial facilities, such as semiconductor manufacturing, represents a significant contributor to PFAS emissions.6 The concentration of PFAS in industrial wastewaters is typically several orders of magnitude higher than that in domestic waters, for instance, reaching a total PFAS concentration of 40![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) 000 ng L−1 in industrial wastewater.19–21 Despite this, there is a scarcity of studies investigating the discharge of PFAS from industrial wastewater treatment plants (WWTPs) to assess their presence and overall discharge loads.20 Moreover, industrial WWTPs often exhibit limited efficacy in PFAS removal, with the transformation of PFAS precursors into perfluoroalkyl acids (PFAA) occurring during biological treatment processes.20 It has been suggested to implement a pretreatment on industrial wastewaters to eliminate PFAS before their release into sewer systems or the environment.6 The common PFAS treatment technologies involve adsorption using activated carbon, high pressure membrane filtration, in situ degradation (oxidation), anion exchange resins, and foam fractionation.16,22,23 Nevertheless, these PFAS remediation methods have some challenges. For instance, the membrane filtration methods, such as reverse osmosis (RO), are associated with high costs and membrane fouling, the anion exchange resins are hard to reuse, the oxidation has a low PFAS degradation efficiency, and secondary PFAS waste is generated when using adsorbents, such as activated carbon.16,24,25
000 ng L−1 in industrial wastewater.19–21 Despite this, there is a scarcity of studies investigating the discharge of PFAS from industrial wastewater treatment plants (WWTPs) to assess their presence and overall discharge loads.20 Moreover, industrial WWTPs often exhibit limited efficacy in PFAS removal, with the transformation of PFAS precursors into perfluoroalkyl acids (PFAA) occurring during biological treatment processes.20 It has been suggested to implement a pretreatment on industrial wastewaters to eliminate PFAS before their release into sewer systems or the environment.6 The common PFAS treatment technologies involve adsorption using activated carbon, high pressure membrane filtration, in situ degradation (oxidation), anion exchange resins, and foam fractionation.16,22,23 Nevertheless, these PFAS remediation methods have some challenges. For instance, the membrane filtration methods, such as reverse osmosis (RO), are associated with high costs and membrane fouling, the anion exchange resins are hard to reuse, the oxidation has a low PFAS degradation efficiency, and secondary PFAS waste is generated when using adsorbents, such as activated carbon.16,24,25
Foam fractionation, also known as aeration or foam flotation, has been determined as a promising remediation method to remove PFAS from contaminated water due to their surfactancy nature, enabling them to adsorb at the air–water interface during foaming.22,23,25–28 Prior to utilization for PFAS removal, foam flotation has been used in other fields, such as enhanced oil recovery (EOR),29,30 and critical materials separation.31–33 Several groups have used custom-made aeration collection devices to achieve PFAS removal.34–36 Burns et al.37,38 successfully integrated a surface-active foam fractionation system into WWTPs for treating 90–110 m3 day−1 of groundwater, aiming to remove PFAS. The removal efficiency was reported to decrease with reducing air flow rates (Qair) and foaming times (tf). According to Meng et al.,34 the lower initial PFAS concentration (0.093 mM vs. 0.382 mM) results in greater fractional PFAS recovery. In addition, PFAS molecules with sulfonic acid headgroups (perfluorosulfonic acid), called PFSA, are easier to be removed compared to the ones with carboxylic acid headgroups (perfluorocarboxylic acid), known as PFCA.35,36 It is worth noting that aeration mostly faces challenges in efficiently removing short-chain PFAS, exemplified by a removal efficiency of around 10–30% for PFBS, and no removal for perfluorobutanoic acid (PFBA).34,35,37 Lee et al.39 explored the impact of metallic activators, such as Ca2+ and Fe3+, on the PFOS and PFOA removal efficiency by using a foam flotation reactor. The introduction of Fe3+ resulted in the highest PFOS removal efficiency, reaching 99.5%. Wang et al.36 also demonstrated that the valence of ions plays an important role on PFAS removal efficiency.
Another emerging challenge in PFAS cleanup is the lack of fast and low-cost detection methods. Liquid chromatography-mass spectrometry, known as LC-MS, is commonly used for PFAS detection.23 However, LC-MS is expansive and specialized. Except for LC-MS, electroanalytical approaches, such as interfacial electrochemical process and porous polymer sensors with a molecularly imprinted polymers electrode, were reviewed by Lamichhane et al.40 with a possibility to detect PFAS. In addition, Mahpishanian et al.41 developed a liquid phase extraction method, where a colored methylene blue-PFAS complex is formed, to detect PFAS via spectrophotometric analysis.
Dimensional analysis is a powerful tool in various fields of science and engineering, including the study of foaming process. Beyond the studies focused directly on foaming,34–39,42–51 dimensional analysis can be applied to investigate and understand foaming process more comprehensively. For instance, optimization of foaming process, scale-up and scale-down studies, comparative studies, model validation, and parameter sensitivity analysis. Nevertheless, only a few studies have focused on the dimensional analysis of foaming process based on interfacial and colloidal parameters. Bois et al.52 applied dimensional analysis to surfactant solutions (sodium laureth sulfate, Tween 20, and Brij L23) to study the influence of processing parameters on their foamability. Expansion rate of foaming, 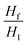 (where Hf is the foam height and Hl is the liquid height) was used to characterize the foaming behavior of surfactant solutions, and it was found that the dimensionless numbers of Reynolds number (Re) and mixing time number control the foaming process occurring in a mechanically agitated vessel. However, the effect of interfacial properties was not taken into account. Noting that the key variables that influence the foaming behavior are the interfacial properties, such as surface tension (γ) and dilatational interfacial modulus (E).24,42–44,48–51 For instance, Gibbs stability
(where Hf is the foam height and Hl is the liquid height) was used to characterize the foaming behavior of surfactant solutions, and it was found that the dimensionless numbers of Reynolds number (Re) and mixing time number control the foaming process occurring in a mechanically agitated vessel. However, the effect of interfacial properties was not taken into account. Noting that the key variables that influence the foaming behavior are the interfacial properties, such as surface tension (γ) and dilatational interfacial modulus (E).24,42–44,48–51 For instance, Gibbs stability 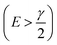 was introduced as the criteria for determining the stability of interface stabilized by particles, and thus, the foam stability.44–47 Mary et al.53 also used dimensional analysis to predict the bubble size of the surfactant solutions, and they demonstrated that the bubble size of foams is mainly controlled but not limited by Capillary number (Ca). They also suggested that the impact of more parameters, such as interfacial rheology and kinetic diffusion of surfactants to the interfaces, needs to be investigated.
was introduced as the criteria for determining the stability of interface stabilized by particles, and thus, the foam stability.44–47 Mary et al.53 also used dimensional analysis to predict the bubble size of the surfactant solutions, and they demonstrated that the bubble size of foams is mainly controlled but not limited by Capillary number (Ca). They also suggested that the impact of more parameters, such as interfacial rheology and kinetic diffusion of surfactants to the interfaces, needs to be investigated.
This work aims to comprehensively investigate the foaming behavior (foamability) and its correlations with the interfacial properties. Therefore, we study PFAS with different headgroups and with different chain lengths in the presence of electrolytes with different concentrations as a case study. However, the study is not limited to PFAS and can be applied to other surfactants. The foaming behavior characterized by 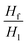 is systematically analyzed by carrying out the dimensionless analysis of a lab-scale foaming column. This theoretical methodology is reinforced by conducting experiments, where processing parameters are altered, and the progression of Hf with tf is observed. Considering that interfacial parameters of PFAS are measured and reported in our recent work,24 the objective of this study is to enhance our comprehension of the primary physical phenomena that predominantly influence foam formation in the aeration process.
is systematically analyzed by carrying out the dimensionless analysis of a lab-scale foaming column. This theoretical methodology is reinforced by conducting experiments, where processing parameters are altered, and the progression of Hf with tf is observed. Considering that interfacial parameters of PFAS are measured and reported in our recent work,24 the objective of this study is to enhance our comprehension of the primary physical phenomena that predominantly influence foam formation in the aeration process.
2. Experimental and methods
2.1. Materials
KPFOS (heptadecafluorooctanesulfonic acid potassium salt, ≥98.0%, CAS: 2795-39-3), PFOA (perfluorooctanoic acid, 95%, CAS: 335-67-1), and KPFBS (potassium nonafluoro-1-butanesulfonate, 98%, CAS: 29420-49-3) were purchased from Sigma-Aldrich. The chemical structure of PFAS compounds used in this work is shown in Fig. 1. Sodium chloride (NaCl) and calcium chloride (CaCl2) were purchased from Fisher BioReagents (≥99.0%, CAS: 7647-14-5) and Thermo Scientific (97%, CAS: 10043-52-4), respectively. All samples were prepared using deionized water produced through Millipore Synergy® Water Purification System and no additional purification of the chemicals was conducted. Lower-concentration PFAS aqueous solutions were prepared through a dilution of higher-concentration PFAS stock solutions. Table 1 presents the composition of the PFAS aqueous solutions analyzed in this study.| PFAS | Concentration (mM) | Salt | Concentration (mM) |
|---|---|---|---|
| KPFOS | 0.01–1.11 | NaCl | 0.5, 1, 5, 10, 50, 100, and 200 |
| CaCl2 | 0.1, 0.5, 1, 5, 10, 50, and 100 | ||
| PFOA | 0.40 | NaCl | 0.5, 1, 5, 10, 50, 100, and 200 |
| KPFBS | 0.40 | CaCl2 | 0.1 and 100 |
2.2. Foaming behavior analysis
The dynamic foaming behavior of PFAS aqueous solutions was recorded and analyzed by using the foam analyzer (Krüss Scientific, DFA 100) with ADVANCE software. The setup is shown in Fig. S1A (ESI†). About 30 mL of PFAS aqueous solution was added into a prism column (CY4572) of 40 mm inner diameter with a max volume of 230 mL, which was fixed onto the sample holder. An O-ring was placed onto the sample holder, followed by a glass filter and another O-ring for sealing as seen in Fig. S1B (ESI†). Two glass filters with a pore size ranging of 16–40 μm (FL4503, ∅ 50 mm) and 40–100 μm (FL4502, ∅ 50 mm), respectively, were used to aerate the PFAS aqueous solution (Table 2).| Glass filter pore size range (Df, μm) | 16–40 and 40–100 |
| Sample volume (mL) | 30 |
| Q air (L min−1) | 0.2–1.0 |
| t f (s) | 30–300 |
| Camera position | 60 mm from Hl0 (initial liquid level) |
The PFAS aqueous solution in the prism column was sparged with air at various Qair ranging from 0.2 to 1.0 L min−1 for different tf of 30, 60, 120, and 300 s to create the PFAS aqueous foams. The video showing the foaming process is available as ESI.† The Qair and tf play important roles in determining the properties of created foams. Given the Qair at 0.2 L min−1, 30 s is enough for KPFBS and PFOA foams to reach the steady state, while it takes about 100 s for KPFOS foams to reach the dynamic equilibrium. Two liquid content sensors were placed inside the prism column, and each of the sensors has seven chips to measure the liquid content (ε) and resistance (the inverse of the electrical conductivity) across the foam at different heights, and one chip on the bottom as the reference (Fig. S1B, ESI†). A camera (2 fps at 1280 × 1024 px), of which the vertical position can be changed, was used to record the foam structure during the foaming process through the prism attachment of the prism column as seen in Fig. S1C (ESI†). Trials for this experiment were conducted at room temperature and the pH was not adjusted. For each formulation, at least two replicates were done.
2.3. Dynamic surface tension
To determine the γ of PFAS aqueous solutions at 25 ± 0.5 °C, the Wilhelmy plate tensiometer (Dataphysics, DCAT 25) was utilized. The γ data points were recorded over a 1 h period, with a minimum of two replicates conducted for each composition. The instrument was calibrated prior to use. The platinum plate was meticulously cleaned by using a Bunsen burner before each measurement to ensure its dryness and cleanliness. The equilibrium surface tension was determined from the intercept of dynamic surface tension against t−0.5.242.4. Dilatational interfacial rheology
The pendent drop tensiometer (Attension KSV Instruments, Biolin Scientific, Finland) was used to characterize the dilatational interfacial rheology of the PFAS-adsorbed air–water interface at room temperature. The pendent drop volume periodically oscillated in the linear viscoelastic range at 0.5 Hz, and the oscillation of γ and surface area (A) were recorded accordingly. The γ was determined by the software according to the Young–Laplace equation.54 The definition of E is as follows:24,55–57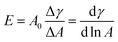 | (1) |
E′ = |E|![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) cos(ϕ) cos(ϕ) | (2a) |
E′′ = |E|![[thin space (1/6-em)]](https://www.rsc.org/images/entities/char_2009.gif) sin(ϕ) = 2πfηs sin(ϕ) = 2πfηs | (2b) |
3. Results and discussion
3.1. Foaming capacity of PFAS aqueous solutions
Two important foaming characteristics are capacity (foamability) and stability, both of which are affected by the foam preparation parameters, tf and Qair, and cross-section area of foam column. For example, Pal et al. prepared foams stabilized by gemini surfactants and reported that 300 s of agitation resulted in the foams with largest volume, while a smaller volume was obtained by stirring the system for 400 s due to the increased bubble rupture tendency.30,34 In this study, we only discuss foamability and the foam stability will be presented in future works. Fig. 2A shows the Hf of 0.4 mM KPFOS aqueous foams during the 30 s of aeration by using Qair of 0.2, 0.4, and 0.6 L min−1, and two glass filters with Df of 40–100 and 16–40 μm, respectively. The Hf increases with an increase in Qair. It should be mentioned that the use of the filter with a smaller Df has no significant impact on the Hf, while the kinetics of foaming,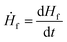 , is slightly affected. For example, lower Ḣf is obtained at the beginning of foaming by using the Qair of 0.2 L min−1 and smaller Df. Furthermore, the Hf does not approach the equilibrium status at 30 s for KPFOS. The system can be considered to reach the equilibrium once the Hf measurements show that the changes fall below a predetermined threshold, and the corresponding time is noted as the equilibrium time (te). The equation below is used to define the Hf change:
, is slightly affected. For example, lower Ḣf is obtained at the beginning of foaming by using the Qair of 0.2 L min−1 and smaller Df. Furthermore, the Hf does not approach the equilibrium status at 30 s for KPFOS. The system can be considered to reach the equilibrium once the Hf measurements show that the changes fall below a predetermined threshold, and the corresponding time is noted as the equilibrium time (te). The equation below is used to define the Hf change: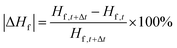 | (3) |
If the |ΔHf| is smaller than 1.5% within 10 s, then the beginning of this 10 s period is considered as the te. Examples of Hf equilibrium status are shown in Fig. 2B. After the equilibrium, Hf drops a little bit and undergoes a small oscillation because the foam structure is separated by some large air bubbles. According to Amani and Firouzi,58 the flow regime in a vertical tube/pipe during the aeration includes (1) bubble, (2) foamy bubble, (3) slug flow, (4) churn flow, and (5) annular flow, depending on the Qair. Our result suggests that when slowly sparging the solutions, the KPFOS foaming flow regime transits from foamy bubble to slug flow/churn flow once the Hf reaches the steady state. When the Qair > 0.2 L min−1, the equilibrium status for KPFOS foams cannot be reached by using DFA 100 due to the overflow alarm, for instance, the Hf reached the overflow alarm height at 36 s when the Qair was 0.4 L min−1, yet the equilibrium status was not even approaching.
Compared to KPFOS, PFOA and KPFBS reach equilibrium status in shorter durations when the Qair is 0.2 L min−1 (Fig. 2B). However, at 0.2 L min−1, PFOA and KPFBS only have a thin layer of bubbles on the top of solutions. Fig. 2C shows the Hf of 0.4 mM KPFOS, PFOA, and KPFBS foams within the 30 s of aeration for Qair of 0.4 L min−1, implying that the KPFOS have the best foamability. When increasing the Qair to 0.6 L min−1, the KPFOS still have the best foaming capacity as seen in Fig. S2 (ESI†). Furthermore, the ε of foams is another crucial concept determining the structure of foams,49,59 which is defined as follows:
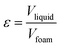 | (4) |
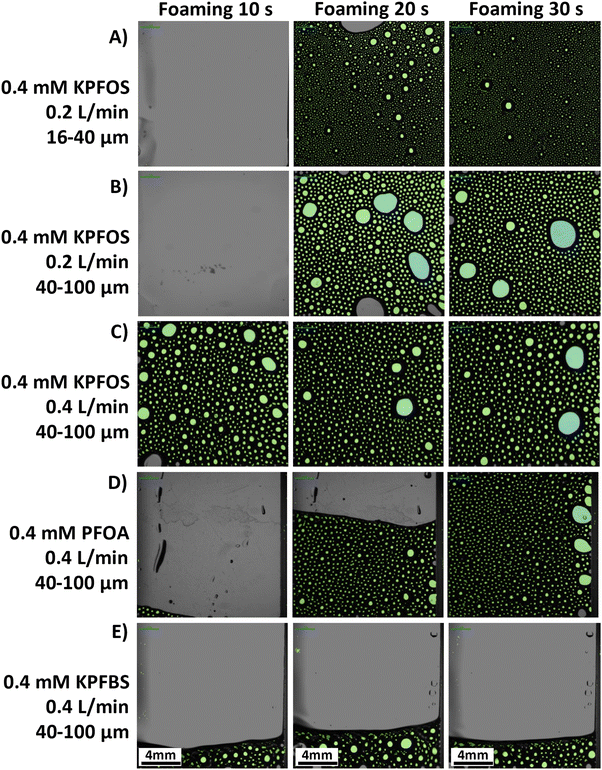
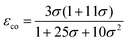 | (5) |
Fig. 3 shows the foam structure at tf of 10, 20, and 30 s for 0.4 mM PFAS foams created by employing different conditions. During foaming, the camera captures the bubbles at 13–15 s for KPFOS foams when the Qair is 0.2 L min−1. Different types of bubble radius are obtained from DFA 100. Fig. S3A (ESI†) shows the average bubble radius (Rave) and Sauter mean bubble radius (R32) of KPFOS foams. The bubble size becomes smaller with aerating. The R32 keeps decreasing until reaching the steady state approximately close to equilibrium Hf. Fig. S3B (ESI†) depicts the bubble count per mm2. Expectedly, the Df has an impact on the bubble size of PFAS foams. Smaller bubble size is obtained by using the filters with a smaller Df as shown in Fig. 3A and B. However, when the steady state is reached, the foams sparged by a smaller Df have a greater R32 as seen in Table S1 (ESI†). The greater difference between Rave and R32 indicates the presence of very large bubbles when using smaller Df, leading to increased drainage and reduced foam stability. In this study, the R32 is used for discussion, and the last 10 data points before stopping aeration are used to obtain the final bubble radius as R. Fig. S4 (ESI†) presents another foam trial for 0.4 mM KPFOS but extending the tf to 120 s. It is clear that the bubble size distribution becomes narrower as the KPFOS foam reaches the steady state.
For 0.4 mM PFOA and KPFBS aqueous solutions, the bubbles were not captured by the camera due to the insufficient Hf for camera field of view at 0.2 L min−1 flow rate. When Qair is 0.4 L min−1 and Df is 40–100 μm, as seen in Fig. 3C and D, PFOA have the smaller bubble size, while KPFOS have larger bubble size. For PFOA, the foam approaches equilibrium status at around 15–18 s, whereas the te is around 7–10 s for KPFBS. The te is increased to around 30 s for PFOA foams with increasing the Qair to 0.6 L min−1, whilst it takes around 12–18 s to reach the equilibrium status for KPFBS foams. In other words, the te becomes greater with increasing the Qair. Furthermore, Qair in the studied range has no significant effect on the PFAS foam bubble size. Comparing PFOA and KPFOS results in Fig. 2 and 3, it indicates that smaller bubble size does not necessarily lead to higher foam capacity.
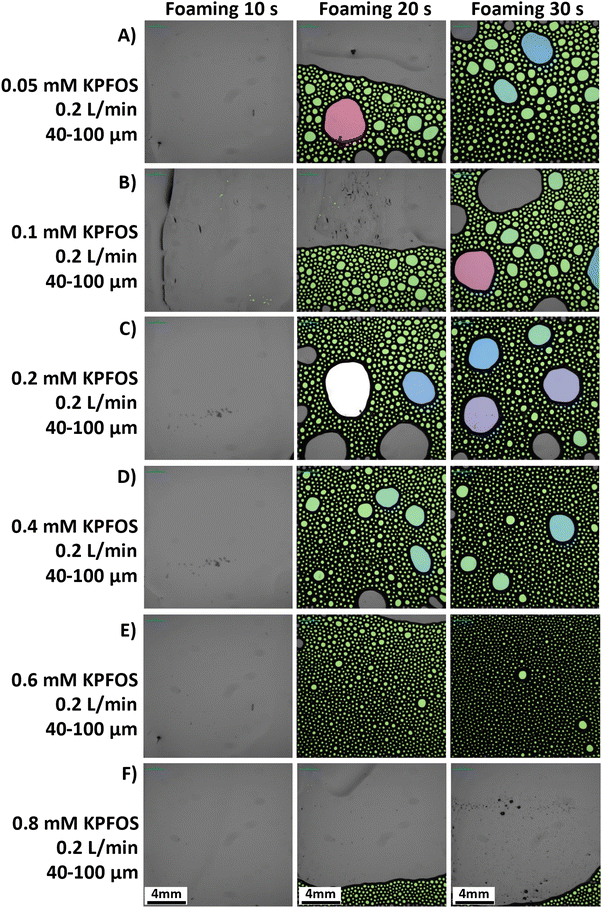
The concentration of PFAS also influences foam structure. Taking the example of KPFOS, the foamability is not linearly correlated with the bulk concentrations. Fig. 4 depicts the foam structure of KPFOS foams with various bulk concentrations, ranging from 0.05 mM to 0.8 mM. A layer of bubbles is obtained when the KPFOS bulk concentrations are 0.01 and 0.02 mM. Nevertheless, the Hf is too small to be recorded. Below 0.01 mM, the KPFOS solutions cannot be foamed. Ranging from 0.01 to 0.4 mM, the foamability is enhanced (i.e., Hf is increased) with increasing the concentration due to the increased KPFOS concentration at the air–water interface.61,62 Additionally, the bubble size of KPFOS foams becomes more uniform and smaller with an increase in concentration. Increasing the KPFOS concentration beyond 0.4 mM, the bubble size further becomes smaller and more monodispersed. Nonetheless, the Hf is reduced as seen in Fig. 4F. Above 0.8 mM, the foam bubbles cannot be recorded due to the small Hf. Only a thin layer of bubbles is obtained when the concentration is near the KPFOS solubility limit (1.1–1.2 mM) in water. It should be noted that the KPFOS does not reach the critical micelle concentration (CMC) before solubility limit. A similar reduction in foam height by concentration was reported by Behara et al.62 for a mixture of sodium dodecyl benzenesulfonate (DOBS) and sodium lauryl ether sulfate (SLES), which was attributed to the reduction in Gibbs elasticity of the plateau borders.
3.2. Dimensional analysis of foaming behavior: expansion rate of foaming
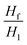 is related to several dimensionless numbers, such as Re and Ca.52,53,63,64 We consider the equilibrium status of foams for studying
is related to several dimensionless numbers, such as Re and Ca.52,53,63,64 We consider the equilibrium status of foams for studying 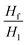 and use the Buckingham π theorem by listing all independent variables as follows:
and use the Buckingham π theorem by listing all independent variables as follows:| Hf = f1(ρ,γ,η,ηs,E,te,Qair,R,Dc,Df,Hl,g) | (6) |
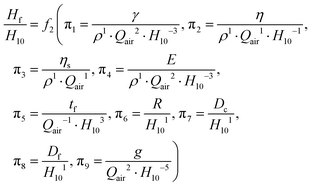 | (7) |
Eqn (7) can be rearranged by combining variables to form the known dimensionless groups as follows:
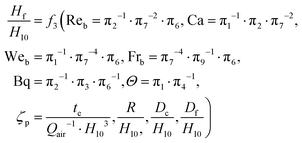 | (8) |
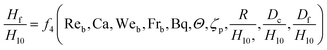 | (9) |
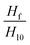 function have no salts added because the steady state cannot be achieved due to the foam overflow. Fig. 5A–G show the effect of various dimensionless numbers on the
function have no salts added because the steady state cannot be achieved due to the foam overflow. Fig. 5A–G show the effect of various dimensionless numbers on the 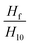 of KPFOS foams.
of KPFOS foams.
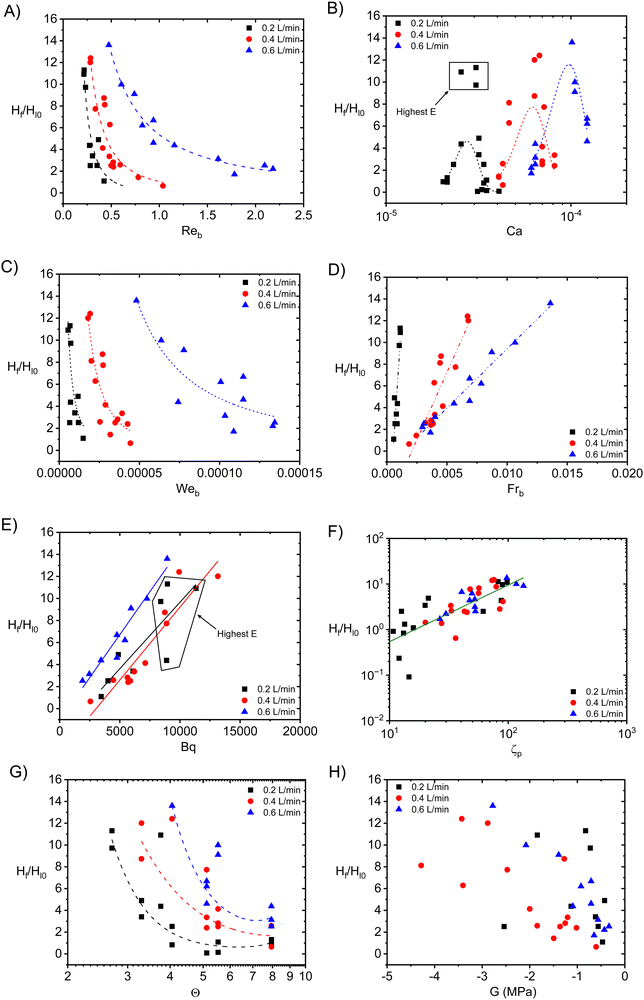
One can see from Fig. 5A, the 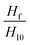 is clearly correlated with Reb. The correlation of
is clearly correlated with Reb. The correlation of  with Reb follows Freundlich model (lines in Fig. 5A), and the fitted equations are shown in Table S2 (ESI†). The Reb represents the ratio of inertial force and viscous force during bubble rise. The low Reb indicates that the flows are laminar, where the viscous force is dominant. This result explains the spherical bubble shape seen in Fig. 3 and 4 as the bubbles will become non-spherical at high Reb (>500).63 A significant increase in
with Reb follows Freundlich model (lines in Fig. 5A), and the fitted equations are shown in Table S2 (ESI†). The Reb represents the ratio of inertial force and viscous force during bubble rise. The low Reb indicates that the flows are laminar, where the viscous force is dominant. This result explains the spherical bubble shape seen in Fig. 3 and 4 as the bubbles will become non-spherical at high Reb (>500).63 A significant increase in 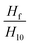 with a decrease in Reb is observed for different Qair. In Bois et al.'s work, it was claimed that the lower foaming expansion rate in the high Reb regime is due to the transition from intermediate flow to turbulent, where the bubble breakup is easier to take place.52 However, this is not the case for our system since all PFAS foaming experiments remain in laminar flow. In addition, at the same Reb, higher Qair gives rise to higher foamability. Therefore, the observed trend in Fig. 5A is dominated by the bubble size.
with a decrease in Reb is observed for different Qair. In Bois et al.'s work, it was claimed that the lower foaming expansion rate in the high Reb regime is due to the transition from intermediate flow to turbulent, where the bubble breakup is easier to take place.52 However, this is not the case for our system since all PFAS foaming experiments remain in laminar flow. In addition, at the same Reb, higher Qair gives rise to higher foamability. Therefore, the observed trend in Fig. 5A is dominated by the bubble size.
The Ca measures the effect of viscous force versus interfacial force across the gas–liquid interface. It was reported that the increase of Ca is associated with the change of bubble size and shape. Mary et al.53 used the Ca to predict the R and found the inverse relationship between the bubble size and Ca. Drenckhan et al.64 pointed out that the elongation of bubbles is associated with the greater Ca. Fig. S5 (ESI†) shows the R as a function of Ca. A minimum is observed in R against Ca variation for all three Qairs. Additionally, a peak is also observed for the relationship between the 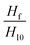 and Ca. From our previous work,24 the highlighted areas in Fig. 5B and Fig. S5 (ESI†) have the highest E, which occurs in the concentration range of 0.2–0.4 mM. Hence, the appearance of
and Ca. From our previous work,24 the highlighted areas in Fig. 5B and Fig. S5 (ESI†) have the highest E, which occurs in the concentration range of 0.2–0.4 mM. Hence, the appearance of 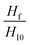 peak and R minimum versus Ca coincides with the maximum E.24 Considering very small Ca in all experiments, the interfacial force dominates the viscous force. According to our previous work, Gibbs adsorption is the dominant mechanism before the maximum, whereas the molecular exchange of PFAS between the interface and bulk prevails on the right side of E maximum.65 Thus, the correlation between the
peak and R minimum versus Ca coincides with the maximum E.24 Considering very small Ca in all experiments, the interfacial force dominates the viscous force. According to our previous work, Gibbs adsorption is the dominant mechanism before the maximum, whereas the molecular exchange of PFAS between the interface and bulk prevails on the right side of E maximum.65 Thus, the correlation between the 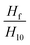 of KPFOS and Ca has regime I and II before and after the peak, respectively. In adsorption-dominated regime I, the foamability increases and the bubble size decreases with increasing the Ca, whereas in the molecular exchange-dominated regime II, the foaming behavior becomes worse with increasing the Ca. The equation y = e(ax2+bx+c) is used to fit the
of KPFOS and Ca has regime I and II before and after the peak, respectively. In adsorption-dominated regime I, the foamability increases and the bubble size decreases with increasing the Ca, whereas in the molecular exchange-dominated regime II, the foaming behavior becomes worse with increasing the Ca. The equation y = e(ax2+bx+c) is used to fit the 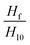 as a function of Ca, with the fitted equations showing in Table S2 (ESI†). It should be noted that this equation form was used for easing the linearization of final equation. Since
as a function of Ca, with the fitted equations showing in Table S2 (ESI†). It should be noted that this equation form was used for easing the linearization of final equation. Since 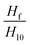 increases with increasing the air injection rates, some data points are not considered for fitting to correctly show the trend. For example, the highlighted points for 0.2 L min−1 are excluded (Fig. 5B) since the same concentrations show overflow before reaching the steady state for 0.4 and 0.6 L min−1.
increases with increasing the air injection rates, some data points are not considered for fitting to correctly show the trend. For example, the highlighted points for 0.2 L min−1 are excluded (Fig. 5B) since the same concentrations show overflow before reaching the steady state for 0.4 and 0.6 L min−1.
The variation of 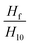 against Web also follows the Freundlich model as seen in Fig. 5C, and the fitted equations are shown in Table S2 (ESI†). The Web is used to determine the significance of foam's inertia in comparison to interfacial forces. As seen, the foamability increases with a decrease in the Web, thus, the foamability is improved when the effect of interfacial forces becomes more significant than inertia. The
against Web also follows the Freundlich model as seen in Fig. 5C, and the fitted equations are shown in Table S2 (ESI†). The Web is used to determine the significance of foam's inertia in comparison to interfacial forces. As seen, the foamability increases with a decrease in the Web, thus, the foamability is improved when the effect of interfacial forces becomes more significant than inertia. The  is linearly related to Frb as seen in Fig. 5D. The linear relationships of
is linearly related to Frb as seen in Fig. 5D. The linear relationships of 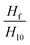 as a function of Frb for various Qairs are shown in Table S2 (ESI†). The Frb refers to the ratio of inertial force and gravitational force. In our case, the foaming capacity of KPFOS increases with increasing the effect of inertial force compared to the gravitational force, with this trend being less sharp for higher Qair.
as a function of Frb for various Qairs are shown in Table S2 (ESI†). The Frb refers to the ratio of inertial force and gravitational force. In our case, the foaming capacity of KPFOS increases with increasing the effect of inertial force compared to the gravitational force, with this trend being less sharp for higher Qair.
As seen in Fig. 5E and Table S2 (ESI†), 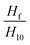 is linearly correlated with Bq. The importance of the surface-to-bulk viscous forces is given by the Bq, when the Reynolds number is small.66 It was reported that the liquid drainage velocity is correlated with the interfacial shear viscosity represented by Bq.67,68 In this work, since PFAS are small molecules (i.e., soluble surfactants), measuring interfacial shear viscosity is challenging. Thus, we calculated the Bq from the measured interfacial dilatational viscosity. The Bq can also represent the surface mobility since the ηs is the effective parameter to characterize the surface mobility.69 Since E′ and ηs are related to the surface mobility, the surfactant flow in the plateau borders is regulated by the adsorption behavior occurring at the surface of node.69 One can see from Fig. 5E that
is linearly correlated with Bq. The importance of the surface-to-bulk viscous forces is given by the Bq, when the Reynolds number is small.66 It was reported that the liquid drainage velocity is correlated with the interfacial shear viscosity represented by Bq.67,68 In this work, since PFAS are small molecules (i.e., soluble surfactants), measuring interfacial shear viscosity is challenging. Thus, we calculated the Bq from the measured interfacial dilatational viscosity. The Bq can also represent the surface mobility since the ηs is the effective parameter to characterize the surface mobility.69 Since E′ and ηs are related to the surface mobility, the surfactant flow in the plateau borders is regulated by the adsorption behavior occurring at the surface of node.69 One can see from Fig. 5E that  increases with increasing the Bq. For 0.2 L min−1, as pointed in Fig. 5E, the samples having greater values of Bq also have higher E. It can be concluded that the effect of ηs on the foaming behavior of KPFOS is significant. Lower
increases with increasing the Bq. For 0.2 L min−1, as pointed in Fig. 5E, the samples having greater values of Bq also have higher E. It can be concluded that the effect of ηs on the foaming behavior of KPFOS is significant. Lower 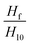 obtained for lower ηs can be attributed to the increased mobility of molecules at the air–water interface.
obtained for lower ηs can be attributed to the increased mobility of molecules at the air–water interface.
As for ζp, the 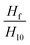 increases with ζp due to the increased te (see Fig. 5F and Table S2, ESI†). The correlation between
increases with ζp due to the increased te (see Fig. 5F and Table S2, ESI†). The correlation between 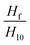 and Θ was fitted with equation y = e(ax2+bx+c), shown as the dashed lines in Fig. 5G (fitted parameters are shown in Table S2, ESI†). Lower Θ, corresponding to better foamability, indicates a lower surface tension and/or higher E influence. The dimensionless numbers that can be analyzed are not limited to the ones mentioned above, but more details will not be discussed in this study.
and Θ was fitted with equation y = e(ax2+bx+c), shown as the dashed lines in Fig. 5G (fitted parameters are shown in Table S2, ESI†). Lower Θ, corresponding to better foamability, indicates a lower surface tension and/or higher E influence. The dimensionless numbers that can be analyzed are not limited to the ones mentioned above, but more details will not be discussed in this study.
Gibbs stability can be used as the criterion to determine the stability of air–water interfaces, as suggested for Pickering foams.44–47 The eqn (10) is originally used to predict bubble coarsening:70
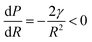 | (10) |
 | (11) |
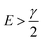 is known as the Gibbs stability criterion.70,71Fig. 5H shows the
is known as the Gibbs stability criterion.70,71Fig. 5H shows the 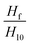 versus G for KPFOS foams. Negative G implies that the Gibbs stability criterion is not fulfilled for KPFOS foams. Unexpectedly, the foamability decreases as the system is approaching the Gibbs stability criterion, at least for KPFOS aqueous foams without any additives. In other words, since in our system
versus G for KPFOS foams. Negative G implies that the Gibbs stability criterion is not fulfilled for KPFOS foams. Unexpectedly, the foamability decreases as the system is approaching the Gibbs stability criterion, at least for KPFOS aqueous foams without any additives. In other words, since in our system 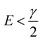 , when E approaches
, when E approaches 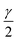 , the interface is more likely to resist against surface area reduction and Ostwald ripening, thus, it is expected that foamability is enhanced. However, this is not the case and as Maestro et al.70 also pointed out, the Gibbs stability criterion is essential to prevent the bubble coarsening, but it is not sufficient to determine the bubble stability.
, the interface is more likely to resist against surface area reduction and Ostwald ripening, thus, it is expected that foamability is enhanced. However, this is not the case and as Maestro et al.70 also pointed out, the Gibbs stability criterion is essential to prevent the bubble coarsening, but it is not sufficient to determine the bubble stability.
 on the dimensionless numbers for PFOA. The
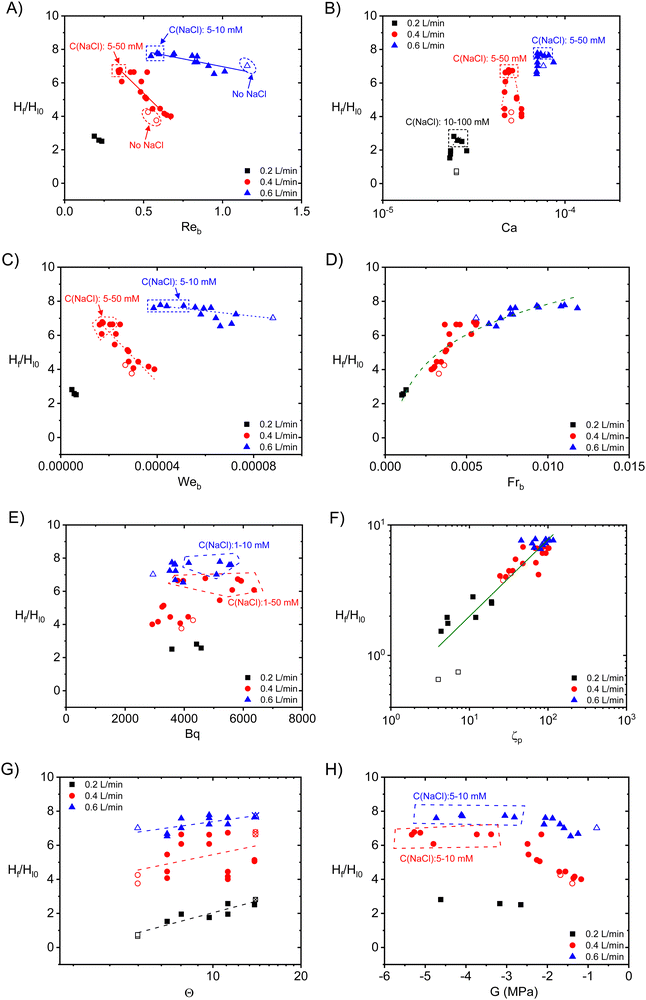
on the dimensionless numbers for PFOA. The 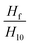 is negatively correlated with the Reb as shown in Fig. 6A. Linear relationships can be used to describe their correlation, and the fitted parameters are shown in Table S3 (ESI†). The Reb is smaller than 1.5, confirming the laminar flow. The open points in Fig. 6A are the PFOA foams without adding NaCl, corresponding to low foamability. Nonetheless, the foamability is not linearly increasing with increasing the NaCl concentration. For example, the highest foamability of PFOA is obtained when the NaCl concentration range is 5–50 mM as pointed out in Fig. 6A. Ndiritu et al.72 studied the effect of NaCl on the foamability of cricket proteins, and they found that there is a certain NaCl concentration at which the highest foamability is obtained. They discussed that the increased foamability is due to the enhanced protein solubility, and the further decrease in the foaming capacity is due to the charge-screening and enhanced hydrophobic interaction and intermolecular cohesion between protein molecules.72,73 Similar explanation may be considered for the foaming of PFOA aqueous solutions.
is negatively correlated with the Reb as shown in Fig. 6A. Linear relationships can be used to describe their correlation, and the fitted parameters are shown in Table S3 (ESI†). The Reb is smaller than 1.5, confirming the laminar flow. The open points in Fig. 6A are the PFOA foams without adding NaCl, corresponding to low foamability. Nonetheless, the foamability is not linearly increasing with increasing the NaCl concentration. For example, the highest foamability of PFOA is obtained when the NaCl concentration range is 5–50 mM as pointed out in Fig. 6A. Ndiritu et al.72 studied the effect of NaCl on the foamability of cricket proteins, and they found that there is a certain NaCl concentration at which the highest foamability is obtained. They discussed that the increased foamability is due to the enhanced protein solubility, and the further decrease in the foaming capacity is due to the charge-screening and enhanced hydrophobic interaction and intermolecular cohesion between protein molecules.72,73 Similar explanation may be considered for the foaming of PFOA aqueous solutions.
The peak of  as a function of Ca is also observed for PFOA foams containing various concentrations of NaCl (Fig. 6B). The fitting equations for different Qairs are shown in Table S3 (ESI†). We call the NaCl concentration range where the highest foamability over the Reb is obtained (as indicated in this figure) as the optimum range. The linear relationship is used to fit with the
as a function of Ca is also observed for PFOA foams containing various concentrations of NaCl (Fig. 6B). The fitting equations for different Qairs are shown in Table S3 (ESI†). We call the NaCl concentration range where the highest foamability over the Reb is obtained (as indicated in this figure) as the optimum range. The linear relationship is used to fit with the 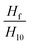 as a function of Web, and the fitted equations are shown in Table S3 (ESI†). When the NaCl concentration is in between 5–50 mM, smaller Webs and higher
as a function of Web, and the fitted equations are shown in Table S3 (ESI†). When the NaCl concentration is in between 5–50 mM, smaller Webs and higher  ratios are obtained.
ratios are obtained.
A single equation can be used to describe the relationship between the  and Frb for all PFOA samples as seen in Fig. 6D (see Table S3, ESI†). At a certain point, the effect of inertial force with respect of gravitational force on PFOA foamability is no longer significant as
and Frb for all PFOA samples as seen in Fig. 6D (see Table S3, ESI†). At a certain point, the effect of inertial force with respect of gravitational force on PFOA foamability is no longer significant as  is reaching a plateau. While the highest Bq (largest ηs) corresponds to the optimized NaCl concentration range, the
is reaching a plateau. While the highest Bq (largest ηs) corresponds to the optimized NaCl concentration range, the 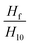 does not show a considerable dependence on the Bq (Fig. 6E). Addition of NaCl increases the ζp (Fig. 6F and Table S3, ESI†) due to the enhanced te. However, the dependency of
does not show a considerable dependence on the Bq (Fig. 6E). Addition of NaCl increases the ζp (Fig. 6F and Table S3, ESI†) due to the enhanced te. However, the dependency of 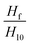 on the ζp becomes less significant for higher Qair. In the case of Θ, the
on the ζp becomes less significant for higher Qair. In the case of Θ, the 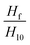 increases with Θ in the 6–14 range, which is unexpected since higher Θ means higher surface tension or lower interfacial modulus. The crossed points in Fig. 6G have the NaCl concentration of 10 mM, above which the foamability decreases (solid points refer to the other NaCl concentrations, both lower and higher than 10 mM).
increases with Θ in the 6–14 range, which is unexpected since higher Θ means higher surface tension or lower interfacial modulus. The crossed points in Fig. 6G have the NaCl concentration of 10 mM, above which the foamability decreases (solid points refer to the other NaCl concentrations, both lower and higher than 10 mM).
Fig. 6H depicts the 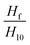 against G. The G is still negative for PFOA foams with and without NaCl. Therefore, the Gibbs criterion is not fulfilled within the studied regime for PFOA. Additionally, adding NaCl reduces the G, but the foamability increases. Also, the foamability is independent of the G within the optimum NaCl concentrations as highlighted in Fig. 6H. In the case of non-optimum NaCl concentrations, as the E is getting closer to
against G. The G is still negative for PFOA foams with and without NaCl. Therefore, the Gibbs criterion is not fulfilled within the studied regime for PFOA. Additionally, adding NaCl reduces the G, but the foamability increases. Also, the foamability is independent of the G within the optimum NaCl concentrations as highlighted in Fig. 6H. In the case of non-optimum NaCl concentrations, as the E is getting closer to 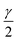 , the foaming capacity is somewhat hindered. The results suggest the significance of colloidal forces between bubbles, which is not considered in Gibbs criterion.
, the foaming capacity is somewhat hindered. The results suggest the significance of colloidal forces between bubbles, which is not considered in Gibbs criterion.
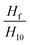 on various dimensionless numbers for KPFBS. We observe similar correlations between
on various dimensionless numbers for KPFBS. We observe similar correlations between 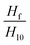 and Reb, Ca, Web, Frb, and ζp for KPFBS similar to that of KPFOS and PFOA. As an example in Fig. 7A, the
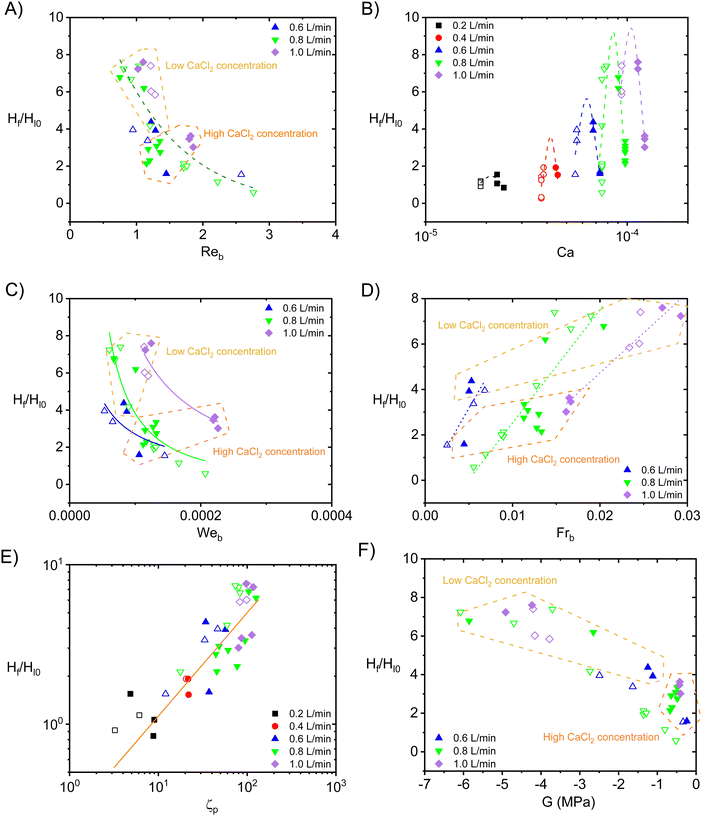
and Reb, Ca, Web, Frb, and ζp for KPFBS similar to that of KPFOS and PFOA. As an example in Fig. 7A, the 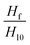 decreases when the effect of inertial force becomes more significant. Since the data points are to some extent superimposed, one equation can be used to describe the relationship as seen in Table S4 (ESI†). Additionally, the introduction of CaCl2 does not remarkably enhance the foamability of KPFBS. However, higher concentrations of CaCl2, such as 100 mM, tend to weaken the foaming capacity of KPFBS and increase the Reb as highlighted in Fig. 7A.
decreases when the effect of inertial force becomes more significant. Since the data points are to some extent superimposed, one equation can be used to describe the relationship as seen in Table S4 (ESI†). Additionally, the introduction of CaCl2 does not remarkably enhance the foamability of KPFBS. However, higher concentrations of CaCl2, such as 100 mM, tend to weaken the foaming capacity of KPFBS and increase the Reb as highlighted in Fig. 7A.
In Fig. 7B, 0.2 and 0.4 L min−1 were also included to determine the relationship between the 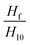 and Ca since bubble size is not required (it should be noted that if the foam height does not reach the camera's field of view, the bubble size data is not available). The fitted equations are shown in Table S4 (ESI†). Due to the limited concentrations of either KPFBS or CaCl2, the R2 is reduced. However, the peaks of
and Ca since bubble size is not required (it should be noted that if the foam height does not reach the camera's field of view, the bubble size data is not available). The fitted equations are shown in Table S4 (ESI†). Due to the limited concentrations of either KPFBS or CaCl2, the R2 is reduced. However, the peaks of  are still appearing. Moreover, the addition of CaCl2 increases the Ca for KPFBS foams, revealing that the viscous force effect becomes more important. In Fig. 7C, the Freundlich model was used to describe the correlation between the
are still appearing. Moreover, the addition of CaCl2 increases the Ca for KPFBS foams, revealing that the viscous force effect becomes more important. In Fig. 7C, the Freundlich model was used to describe the correlation between the 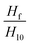 and Web, for which the equations are shown in Table S4 (ESI†). The linear relationships between the
and Web, for which the equations are shown in Table S4 (ESI†). The linear relationships between the 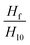 and Frb for 0.6, 0.8, and 1.0 L min−1 are shown in Table S4 (ESI†) and Fig. 7D. The foaming capacity decreases with increasing the Web and Frb. Furthermore, higher concentration of CaCl2 tend to enhance the Web and reduce the Frb, and at the same time, the foamability is hindered.
and Frb for 0.6, 0.8, and 1.0 L min−1 are shown in Table S4 (ESI†) and Fig. 7D. The foaming capacity decreases with increasing the Web and Frb. Furthermore, higher concentration of CaCl2 tend to enhance the Web and reduce the Frb, and at the same time, the foamability is hindered.
From our previous work,24 dilatational interfacial rheology of 0.4 mM of KPFBS could not be measured due to its high solubility and weaker amphiphilicity, thus, the E is not considered. Hence, the Bq and Θ are not discussed for KPFBS foams. No significant influence of adding CaCl2 on ζp is observed according to Fig. 7E, although the 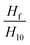 increases with increasing the ζp (Table S4, ESI†). The more scattered points in Fig. 7E compared to KPFOS results can be due to the worst foamability of short-chain KPFBS. In other words, the KPFBS foams always have a relatively poorer quality in terms of bubble imaging due to the fastest bubble film rupture. However, the effect of processing on KPFBS foams is still significant. In addition, similar to KPFOS and PFOA systems, the
increases with increasing the ζp (Table S4, ESI†). The more scattered points in Fig. 7E compared to KPFOS results can be due to the worst foamability of short-chain KPFBS. In other words, the KPFBS foams always have a relatively poorer quality in terms of bubble imaging due to the fastest bubble film rupture. However, the effect of processing on KPFBS foams is still significant. In addition, similar to KPFOS and PFOA systems, the 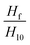 is reduced as KPFBS samples reach the Gibbs stability criterion. Also, adding CaCl2 does not dramatically enhance or decrease the G as shown in Fig. 7E. Nonetheless, if only considering the KPFBS foams with CaCl2, higher CaCl2 concentrations (100 mM) qualitatively make the systems approach the Gibbs stability criterion.
is reduced as KPFBS samples reach the Gibbs stability criterion. Also, adding CaCl2 does not dramatically enhance or decrease the G as shown in Fig. 7E. Nonetheless, if only considering the KPFBS foams with CaCl2, higher CaCl2 concentrations (100 mM) qualitatively make the systems approach the Gibbs stability criterion.
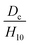 and
and 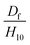 remain constant. Generally, the dimensionless numbers of Reb, Frb, ζp, and
remain constant. Generally, the dimensionless numbers of Reb, Frb, ζp, and  represent the processing parameters, while Ca, Web, Bq, and Θ indicate the effect of interfacial properties. Consequently, the eqn (9) can be simplified as follow:
represent the processing parameters, while Ca, Web, Bq, and Θ indicate the effect of interfacial properties. Consequently, the eqn (9) can be simplified as follow: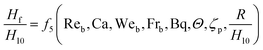 | (12) |
The eqn (12) can be written, for example, as the monomial form shown below:
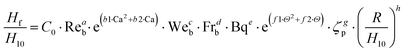 | (13) |
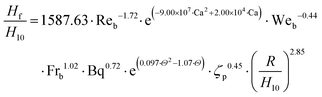 | (14) |
The foamability of KPFOS is affected by all dimensionless numbers, but one should notice that the relatively larger power coefficients of Reb and 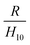 in eqn (14) suggest the relatively more significant influence of Reb and R on the foamability of KPFOS in the studied concentration range. The Qair and R have the major effects on the expansion rate of KPFOS foams, and both Qair and R influence the Reb. Additionally, the Web and Frb also contain Qair and R, and higher coefficients for Reb and Frb suggest that the inertial force effect is significant for KPFOS foamability. Moreover, comparing Web to Bq, higher coefficient for Bq suggests that the interfacial viscosity affects the KPFOS foamability much more than expected. The tick marks in Table 3 indicate whether a dimensionless number significantly contributes to the foaming behavior. Given the range of dimensionless numbers according to Fig. 5, we can further estimate the influence of each number on the KPFOS foamability. For example, the Reb ranges from 0.2 to 2.2, giving the Reb−1.72 range between 0.26 and 15.93. If the range is close to 1, the effect will be negligible. Also, the numbers in Table 3 rank the effect of each dimensionless number.
in eqn (14) suggest the relatively more significant influence of Reb and R on the foamability of KPFOS in the studied concentration range. The Qair and R have the major effects on the expansion rate of KPFOS foams, and both Qair and R influence the Reb. Additionally, the Web and Frb also contain Qair and R, and higher coefficients for Reb and Frb suggest that the inertial force effect is significant for KPFOS foamability. Moreover, comparing Web to Bq, higher coefficient for Bq suggests that the interfacial viscosity affects the KPFOS foamability much more than expected. The tick marks in Table 3 indicate whether a dimensionless number significantly contributes to the foaming behavior. Given the range of dimensionless numbers according to Fig. 5, we can further estimate the influence of each number on the KPFOS foamability. For example, the Reb ranges from 0.2 to 2.2, giving the Reb−1.72 range between 0.26 and 15.93. If the range is close to 1, the effect will be negligible. Also, the numbers in Table 3 rank the effect of each dimensionless number.
For PFOA, as shown in Fig. S7 (ESI†), the R2 of the fit (R2 = 0.96797) to eqn (13) based on Table S6 (ESI†) implies good agreement between the model values and experimental values of 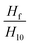 with following equation:
with following equation:
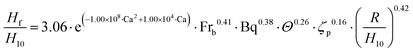 | (15) |
 in eqn (15) reveal that the foamability of PFOA in the presence of NaCl highly depends on the Qair and R. Table 3 shows that the addition of NaCl improves the foamability of PFOA through affecting the interfacial properties, represented by Bq. Fig. S8 (ESI†) shows the prediction of foaming capacity for KPFBS solutions with a R2 of 0.73937. One of the possible reasons for the lower R2 of fitting for KPFBS foaming behavior could be the absence of Θ and Bq experimental values for dimensional analysis. It might also be due to the weak adsorption activity of KPFBS.24 The monomial equation is shown below:
in eqn (15) reveal that the foamability of PFOA in the presence of NaCl highly depends on the Qair and R. Table 3 shows that the addition of NaCl improves the foamability of PFOA through affecting the interfacial properties, represented by Bq. Fig. S8 (ESI†) shows the prediction of foaming capacity for KPFBS solutions with a R2 of 0.73937. One of the possible reasons for the lower R2 of fitting for KPFBS foaming behavior could be the absence of Θ and Bq experimental values for dimensional analysis. It might also be due to the weak adsorption activity of KPFBS.24 The monomial equation is shown below: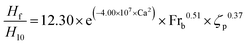 | (16) |
 ) in this study is not significant. Table S7 (ESI†) summarizes the universal equations for the studied PFAS foams.
) in this study is not significant. Table S7 (ESI†) summarizes the universal equations for the studied PFAS foams.
4. Conclusions
In this present study, the foaming behavior of three PFAS, which are KPFOS, PFOA and KPFBS, is studied by aerating their aqueous solutions with varied processing parameters of air flow rate and foaming time. Generally, the foaming capacity follows the order of KPFOS > PFOA > KPFBS. The foaming capacity of KPFOS, PFOA and KPFBS is further investigated by using dimensional analysis approach to correlate the expansion rate of foaming with several different dimensionless numbers.PFAS bulk concentration affects their foaming behavior. Taking the example of KPFOS, there is a concentration (around 0.4 mM) at which the highest foam height is obtained. However, the bubble size continuously decreases with increasing the bulk concentration. Both processing and interfacial parameters influence KPFOS foamability with the Boussinesq number affecting the expansion rate of foaming the most in the studied regime. The addition of salt, NaCl, and its concentration affects PFOA foaming behavior. The NaCl concentration range of 5–50 mM improves PFOA foaming capacity by influencing the Boussinesq number. Interestingly, the maximum value of foam height with changing the Capillary number and interface number appear around this NaCl concentration range. The situation of KPFBS is much simpler. According to our previous study, 0.4 mM KPFBS has negligible dilatational interfacial modulus. Therefore, Boussinesq and interface numbers could not be considered for KPFBS. The foaming expansion rate for KPFBS is governed by the Froude number. Our study suggests that lower CaCl2 concentration is helpful to obtain the relatively higher foaming expansion rate for short-chain PFAS, coinciding with lower Reynolds and Weber numbers, as well as Gibbs stability. Nevertheless, the lower accuracy of the fit for KPFBS foaming expansion rate suggests the significant role of PFAS interfacial properties when predicting their foaming properties.
Compared to the processing parameters, our study demonstrates the significant impact of interfacial properties, representing by Capillary, Weber, Boussinesq and interface numbers on foaming behavior, which could be also the case of other surfactants. A maximum PFAS foaming capacity with changing the Capillary number is observed, coinciding with the appearance of highest dilatational interfacial modulus. In this work, the interfacial rheology parameters are for the first time considered for performing the dimensional analysis on foaming of surfactant solutions, providing an insight into the scale-up of foam fractionation for removal of emerging contaminants. Moreover, the Gibbs criterion is not fulfilled, implying the PFAS foams are relatively unstable, which may be beneficial in real application since the collected foam after fractionation should be destabilized for chemical degradation of PFAS.
Finally, the models for different PFAS do not superimpose, likely due to the lack of data accounting for bubble interactions and foam thin film behavior. Therefore, further studies are needed to incorporate these effects and move towards a universal model.
Data availability
The data supporting this article have been included as part of the ESI.†Conflicts of interest
There are no conflicts to declare.Acknowledgements
The Bureau of Reclamation (BoR) provided financial support for this work under the Grant of R21AC10418-00. The authors also thank the Institute for Applied Surfactant Research (IASR) for providing instruments used in this work.References
- J. Glüge, M. Scheringer and I. T. Cousins,
et al., An overview of the uses of per- And polyfluoroalkyl substances (PFAS), Environ. Sci.: Processes Impacts, 2020, 22(12), 2345–2373, 10.1039/d0em00291g
.
- S. Kurwadkar, J. Dane and S. R. Kanel,
et al., Per- and polyfluoroalkyl substances in water and wastewater: A critical review of their global occurrence and distribution, Sci. Total Environ, 2022, 809, 151003, DOI:10.1016/j.scitotenv.2021.151003
.
- Z. Zhang, D. Sarkar, J. K. Biswas and R. Datta, Biodegradation of per- and polyfluoroalkyl substances (PFAS): A review, Bioresour. Technol., 2022, 344, 126223, DOI:10.1016/j.biortech.2021.126223
.
- J. Li, J. Sun and P. Li, Exposure routes, bioaccumulation and toxic effects of per- and polyfluoroalkyl substances (PFASs) on plants: A critical review, Environ. Int., 2022, 158, 106891, DOI:10.1016/j.envint.2021.106891
.
- B. Trang, Y. Li, X.-S. Xue, M. Ateia, K. N. Houk and W. R. Dichtel, Low-temperature mineralization of perfluorocarboxylic acids, Science, 2022, 377(6608), 839–845, DOI:10.1126/science.abm8868
.
- A. B. Nienhauser, M. S. Ersan, Z. Lin, F. Perreault, P. Westerhoff and S. Garcia-Segura, Boron-doped diamond electrodes degrade short- and long-chain per- and polyfluorinated alkyl substances in real industrial wastewaters, J. Environ. Chem. Eng., 2022, 10(2), 107192, DOI:10.1016/j.jece.2022.107192
.
- X. Liu, X. Zheng and L. Zhang,
et al., Joint Toxicity Mechanisms of Binary Emerging Pfas Mixture on Algae (Chlorella Pyrenoidosa) at Environmental Concentration, J. Hazard. Mater., 2022, 437, 129355, DOI:10.2139/ssrn.4063771
.
- P. Pierozan, M. Kosnik and O. Karlsson, High-content analysis shows synergistic effects of low perfluorooctanoic acid (PFOS) and perfluorooctane sulfonic acid (PFOA) mixture concentrations on human breast epithelial cell carcinogenesis, Environ. Int., 2023, 172, 107746, DOI:10.1016/j.envint.2023.107746
.
- B. P. Rickard, I. Rizvi and S. E. Fenton, Per- and poly-fluoroalkyl substances (PFAS) and female reproductive outcomes: PFAS elimination, endocrine-mediated effects, and disease, Toxicology, 2022, 465, 153031, DOI:10.1016/j.tox.2021.153031
.
- T. G. Ambaye, M. Vaccari, S. Prasad and S. Rtimi, Recent progress and challenges on the removal of per- and poly-fluoroalkyl substances (PFAS) from contaminated soil and water, Environ. Sci. Pollut. Res., 2022, 29(39), 58405–58428, DOI:10.1007/s11356-022-21513-2
.
- S. E. Fenton, A. Ducatman and A. Boobis,
et al., Per- and Polyfluoroalkyl Substance Toxicity and Human Health Review: Current State of Knowledge and Strategies for Informing Future Research, Environ. Toxicol. Chem., 2021, 40(3), 606–630, DOI:10.1002/etc.4890
.
- E. M. Sunderland, X. C. Hu, C. Dassuncao, A. K. Tokranov, C. C. Wagner and J. G. Allen, A review of the pathways of human exposure to poly- and perfluoroalkyl substances (PFASs) and present understanding of health effects, J. Exposure Sci. Environ. Epidemiol., 2019, 29(2), 131–147, DOI:10.1038/s41370-018-0094-1
.
- M. N. Ehsan, M. Riza, M. N. Pervez, M. M. O. Khyum, Y. Liang and V. Naddeo, Environmental and health impacts of PFAS: Sources, distribution and sustainable management in North Carolina (USA), Sci. Total Environ., 2023, 878, 163123, DOI:10.1016/j.scitotenv.2023.163123
.
- S. Y. Wee and A. Z. Aris, Revisiting the “forever chemicals”, PFOA and PFOS exposure in drinking water, npj Clean Water, 2023, 6(1), 57, DOI:10.1038/s41545-023-00274-6
.
- B. E. Blake and S. E. Fenton, Early life exposure to per- and polyfluoroalkyl substances (PFAS) and latent health outcomes: A review including the placenta as a target tissue and possible driver of peri- and postnatal effects, Toxicology, 2020, 443, 152565, DOI:10.1016/j.tox.2020.152565
.
- B. C. Crone, T. F. Speth and D. G. Wahman,
et al., Occurrence of per- and polyfluoroalkyl substances (PFAS) in source water and their treatment in drinking water, Crit. Rev. Environ. Sci. Technol., 2019, 49(24), 2359–2396, DOI:10.1080/10643389.2019.1614848
.
- X. C. Hu, D. Q. Andrews and A. B. Lindstrom,
et al., Detection of Poly- and Per fl uoroalkyl Substances (PFASs) in U.S. Drinking Water Linked to Industrial Sites, Military Fire Training Areas, and Wastewater Treatment Plants, Environ. Sci. Technol. Lett., 2016, 3(10), 344–350, DOI:10.1021/acs.estlett.6b00260
.
- PFAS National Primary Drinking Water Regulation. US EPA.:40 CFR Parts 141 and 142, https://www.federalregister.gov/d/2024-07773.
- E. Tavasoli, J. L. Luek, J. P. Malley and P. J. Mouser, Distribution and fate of per- And polyfluoroalkyl substances (PFAS) in wastewater treatment facilities, Environ. Sci.: Processes Impacts, 2021, 23(6), 903–913, 10.1039/d1em00032b
.
- S. Barisci and R. Suri, Occurrence and removal of poly/perfluoroalkyl substances (PFAS) in municipal and industrial wastewater treatment plants, Water Sci. Technol., 2021, 84(12), 3442–3468, DOI:10.2166/wst.2021.484
.
- S. Liu, B. Jin, H. P. H. Arp, W. Chen, Y. Liu and G. Zhang, The Fate and Transport of Chlorinated Polyfluorinated Ether Sulfonates and Other PFAS through Industrial Wastewater Treatment Facilities in China, Environ. Sci. Technol., 2022, 56(5), 3002–3010, DOI:10.1021/acs.est.1c04276
.
- P. H. N. Vo, T. Buckley, X. Xu, T. M. H. Nguyen, V. Rudolph and P. Shukla, Foam fractionation of per- and polyfluoroalkyl substances (PFASs) in landfill leachate using different cosurfactants, Chemosphere, 2023, 310, 136869, DOI:10.1016/j.chemosphere.2022.136869
.
- S. J. Smith, C. Keane, L. Ahrens and K. Wiberg, Integrated Treatment of Per- and Polyfluoroalkyl Substances in Existing Wastewater Treatment Plants—Scoping the Potential of Foam Partitioning, ACS ES&T Eng., 2023, 3(9), 1276–1285, DOI:10.1021/acsestengg.3c00091
.
- M. Zhou, Z. Abbasian Chaleshtari, B.-J. Shiau, B. P. Grady and R. Foudazi, Air-water interfacial properties of perfluorosulfonic acid salts with different chain lengths, Colloids Surf., A, 2024, 694, 134129, DOI:10.1016/j.colsurfa.2024.134129
.
- Z. Abbasian Chaleshtari and R. Foudazi, A Review on Per-and Polyfluoroalkyl Substances (PFAS) Remediation: Separation Mechanisms and Molecular Interactions, ACS ES&T Water, 2022, 2(12), 2258–2272, DOI:10.1021/acsestwater.2c00271
.
- Pennsylvania DEP. PFAS Action Team; Pennsylvania's Department of Environmental Protection, 2019, https://files.dep.state.pa.us/Water/DrinkingWater/PerfluorinatedChemicals/Reports/20191205-PFAS-Action-Team-Initial-Report-Pennsylvania.pdf.
- Y. Lyu, M. L. Brusseau, W. Chen, N. Yan, X. Fu and X. Lin, Adsorption of PFOA at the Air-Water Interface during Transport in Unsaturated Porous Media, Environ. Sci. Technol., 2018, 52(14), 7745–7753, DOI:10.1021/acs.est.8b02348
.
- T. Buckley, X. Xu, V. Rudolph, M. Firouzi and P. Shukla, Review of foam fractionation as a water treatment technology, Sep. Sci. Technol., 2022, 57(6), 929–958, DOI:10.1080/01496395.2021.1946698
.
- N. Pal, N. Kumar, A. Verma, K. Ojha and A. Mandal, Performance Evaluation of Novel Sunflower Oil-Based Gemini Surfactant(s) with Different Spacer Lengths: Application in Enhanced Oil Recovery, Energy Fuels, 2018, 32(11), 11344–11361, DOI:10.1021/acs.energyfuels.8b02744
.
- N. Pal, A. Verma, K. Ojha and A. Mandal, Nanoparticle-modified gemini surfactant foams as efficient displacing fluids for enhanced oil recovery, J. Mol. Liq., 2020, 310, 113193, DOI:10.1016/j.molliq.2020.113193
.
- S. Shetty, I. V. Chernyshova and S. Ponnurangam, Foam flotation of rare earth elements by conventional and green surfactants, Miner. Eng., 2020, 158, 106585, DOI:10.1016/j.mineng.2020.106585
.
- W. Zhang and R. Honaker, A fundamental study of octanohydroxamic acid adsorption on monazite surfaces, Int. J. Miner. Process., 2017, 164, 26–36, DOI:10.1016/j.minpro.2017.05.006
.
- C. Marion, R. Li and K. E. Waters, A review of reagents applied to rare-earth mineral flotation, Adv. Colloid Interface Sci., 2020, 279, 102142, DOI:10.1016/j.cis.2020.102142
.
- P. Meng, S. Deng and A. Maimaiti,
et al., Efficient removal of perfluorooctane sulfonate from aqueous film-forming foam solution by aeration-foam collection, Chemosphere, 2018, 203, 263–270, DOI:10.1016/j.chemosphere.2018.03.183
.
- S. J. Smith, K. Wiberg, P. McCleaf and L. Ahrens, Pilot-Scale Continuous Foam Fractionation for the Removal of Per- and Polyfluoroalkyl Substances (PFAS) from Landfill Leachate, ACS Environ. Sci. Technol. Water, 2022, 2(5), 841–851, DOI:10.1021/acsestwater.2c00032
.
- Y. Wang, Y. Ji, V. Tishchenko and Q. Huang, Removing per- and polyfluoroalkyl substances (PFAS) in water by foam fractionation, Chemosphere, 2023, 311, 137004, DOI:10.1016/j.chemosphere.2022.137004
.
- D. J. Burns, P. Stevenson and P. J. C. Murphy, PFAS removal from groundwaters using Surface-Active Foam Fractionation, Remediation, 2021, 31(4), 19–33, DOI:10.1002/rem.21694
.
- D. J. Burns, H. M. Hinrichsen, P. Stevenson and P. J. C. Murphy, Commercial-scale remediation of per- and polyfluoroalkyl substances from a landfill leachate catchment using Surface-Active Foam Fractionation (SAFF®), Remediation, 2022, 32(3), 139–150, DOI:10.1002/rem.21720
.
- Y. C. Lee, P. Y. Wang, S. L. Lo and C. P. Huang, Recovery of perfluorooctane sulfonate (PFOS) and perfluorooctanoate (PFOA) from dilute water solution by foam flotation, Sep. Purif. Technol., 2017, 173, 280–285, DOI:10.1016/j.seppur.2016.09.012
.
- H. B. Lamichhane and D. W. M. Arrigan, Electroanalytical chemistry of per- and polyfluoroalkyl substances, Curr. Opin. Electrochem., 2023, 40, 101309, DOI:10.1016/j.coelec.2023.101309
.
- S. Mahpishanian, M. Zhou and R. Foudazi, Magnetic amino-functionalized graphene oxide nanocomposite for PFAS removal from water, Environ. Sci. Adv., 2024 10.1039/d4va00171k
.
- D. Beneventi, B. Carre and A. Gandini, Role of surfactant structure on surface and foaming properties, Colloids Surf., A, 2001, 189(1–3), 65–73, DOI:10.1016/S0927-7757(01)00602-1
.
- H. Wang, X. Wei, Y. Du and D. Wang, Experimental investigation on the dilatational interfacial rheology of dust-suppressing foam and its effect on foam performance, Process Saf. Environ. Prot., 2019, 123, 351–357, DOI:10.1016/j.psep.2019.01.027
.
- N. Brown, A. de la Pena and S. Razavi, Interfacial rheology insights: particle texture and Pickering foam stability, J. Phys.: Condens. Matter, 2023, 35(38), 4002–4016, DOI:10.1088/1361-648x/acde2c
.
- A. C. Martinez, E. Rio, G. Delon, A. Saint-Jalmes, D. Langevin and B. P. Binks, On the origin of the remarkable stability of aqueous foams stabilised by nanoparticles: Link with microscopic surface properties, Soft Matter, 2008, 4(7), 1531–1535, 10.1039/b804177f
.
- A. Stocco, W. Drenckhan, E. Rio, D. Langevin and B. P. Binks, Particle-stabilised foams: an interfacial study, Soft Matter, 2009, 5(11), 2215–2222, 10.1039/b901180c
.
- D. Georgieva, A. Cagna and D. Langevin, Link between surface elasticity and foam stability, Soft Matter, 2009, 5(10), 2063–2071, 10.1039/b822568k
.
- S. Hilgenfeldt, S. A. Koehler and H. A. Stone, Dynamics of coarsening foams: Accelerated and self-limiting drainage, Phys. Rev. Lett., 2001, 86(20), 4704, DOI:10.1103/PhysRevLett.86.4704
.
- A. Saint-Jalmes, Physical chemistry in foam drainage and coarsening, Soft Matter, 2006, 2(10), 836–849, 10.1039/b606780h
.
- T. Tamura, Y. Kaneko and M. Ohyama, Dynamic Surface Tension and Foaming Properties of Aqueous Polyoxyethylene n-Dodecyl Ether Solutions, J. Colloid Interface Sci., 1995, 173(2), 493–499, DOI:10.1006/jcis.1995.1351
.
- P. Kanokkarn, T. Shiina, M. Santikunaporn and S. Chavadej, Equilibrium and dynamic surface tension in relation to diffusivity and foaming properties: Effects of surfactant type and structure, Colloids Surf., A, 2017, 524, 135–142, DOI:10.1016/j.colsurfa.2017.04.043
.
- R. Bois, O. Adriao, G. Delaplace, I. Pezron, A. Nesterenko and E. Van-Hecke, Influence of process variables on foaming ability of surfactants: Experimental study and dimensional analysis, Chem. Eng. Res. Des., 2021, 165, 40–50, DOI:10.1016/j.cherd.2020.10.021
.
- G. Mary, S. Mezdour, G. Delaplace, R. Lauhon, G. Cuvelier and F. Ducept, Modelling of the continuous foaming operation by dimensional analysis, Chem. Eng. Res. Des., 2013, 91(12), 2579–2586, DOI:10.1016/j.cherd.2013.05.020
.
- M. Zhou and R. Foudazi, Effect of Cosurfactant on Structure and Properties of Polymerized High Internal Phase Emulsions (PolyHIPEs), Langmuir, 2021, 37(26), 7907–7918, DOI:10.1021/acs.langmuir.1c00419
.
- M. Zhou, A. Bandegi and R. Foudazi, Control of pore interconnectivity in emulsion-templated porous polymers, Polymer, 2023, 281, 126085, DOI:10.1016/j.polymer.2023.126085
.
- C. Kotsmar, J. Krägel, V. I. Kovalchuk, E. V. Aksenenko, V. B. Fainerman and R. Miller, Dilation and shear rheology of mixed β-casein/surfactant adsorption layers, J. Phys. Chem. B, 2009, 113(1), 103–113, DOI:10.1021/jp807197s
.
- F. Ravera, G. Loglio and V. I. Kovalchuk, Interfacial dilational rheology by oscillating bubble/drop methods, Curr. Opin. Colloid Interface Sci., 2010, 15(4), 217–228, DOI:10.1016/j.cocis.2010.04.001
.
- P. Amani and M. Firouzi, Effect of salt and particles on the hydrodynamics of foam flows in relation to foam static characteristics, Chem. Eng. Sci., 2022, 254, 117611, DOI:10.1016/j.ces.2022.117611
.
- P. Amani, S. I. Karakashev and N. A. Grozev,
et al., Effect of selected monovalent salts on surfactant stabilized foams, Adv. Colloid Interface Sci., 2021, 295, 102490, DOI:10.1016/j.cis.2021.102490
.
- K. Feitosa, S. Marze, A. Saint-Jalmes and D. J. Durian, Electrical conductivity of dispersions: From dry foams to dilute suspensions, J. Phys.: Condens. Matter, 2005, 17(41), 6301–6305, DOI:10.1088/0953-8984/17/41/001
.
- N. Yekeen, M. A. Manan, A. K. Idris and A. M. Samin, Influence of surfactant and electrolyte concentrations on surfactant Adsorption and foaming characteristics, J. Pet. Sci. Eng., 2017, 149, 612–622, DOI:10.1016/j.petrol.2016.11.018
.
- M. R. Behera, S. R. Varade, P. Ghosh, P. Paul and A. S. Negi, Foaming in micellar solutions: Effects of surfactant, salt, and oil concentrations, Ind. Eng. Chem. Res., 2014, 53(48), 18497–18507, DOI:10.1021/ie503591v
.
-
A. Nguyen and H. J. Schulze, Colloidal Science of Flotation, CRC Press, 2003 DOI:10.1201/9781482276411
.
- W. Drenckhan and A. Saint-Jalmes, The science of foaming, Adv. Colloid Interface Sci., 2015, 222, 228–259, DOI:10.1016/j.cis.2015.04.001
.
- J. Yang, K. Yu, T. Tsuji, R. Jha and Y. Y. Zuo, Determining the surface dilational rheology of surfactant and protein films with a droplet waveform generator, J. Colloid Interface Sci., 2019, 537, 547–553, DOI:10.1016/j.jcis.2018.11.054
.
- A. Martínez-Calvo and A. Sevilla, Universal Thinning of Liquid Filaments under Dominant Surface Forces, Phys. Rev. Lett., 2020, 125(11), 114502, DOI:10.1103/PHYSREVLETT.125.114502
.
- A. V. Nguyen, Liquid drainage in single Plateau borders of foam, J. Colloid Interface Sci., 2002, 249(1), 194–199, DOI:10.1006/jcis.2001.8176
.
- P. Stevenson, Dimensional analysis of foam drainage, Chem. Eng. Sci., 2006, 61(14), 4503–4510, DOI:10.1016/j.ces.2006.02.026
.
- F. Rouyer, B. Haffner, N. Louvet, Y. Khidas and O. Pitois, Foam clogging, Soft Matter, 2014, 10(36), 6990–6998, 10.1039/c4sm00496e
.
- A. Maestro, E. Rio, W. Drenckhan, D. Langevin and A. Salonen, Foams stabilised by mixtures of nanoparticles and oppositely charged surfactants: Relationship between bubble shrinkage and foam coarsening, Soft Matter, 2014, 10(36), 6975–6983, 10.1039/c4sm00047a
.
- S. Barman, M. L. Davidson, L. M. Walker, S. L. Anna and J. A. Zasadzinski, Inflammation product effects on dilatational mechanics can trigger the Laplace instability and acute respiratory distress syndrome, Soft Matter, 2020, 16(29), 6890–6901, 10.1039/d0sm00415d
.
- A. K. Ndiritu, J. N. Kinyuru, P. N. Gichuhi and G. M. Kenji, Effects of NaCl and pH on the functional properties of edible crickets (Acheta domesticus) protein concentrate, J. Food Meas. Charact., 2019, 13, 1788–1796, DOI:10.1007/s11694-019-00097-5
.
- O. S. Lawal, Functionality of African locust bean (Parkia biglobossa) protein isolate: Effects of pH, ionic strength and various protein concentrations, Food Chem., 2004, 86(3), 345–355, DOI:10.1016/j.foodchem.2003.09.036
.
Footnote |
| † Electronic supplementary information (ESI) available. See DOI: https://doi.org/10.1039/d4sm00931b |
| This journal is © The Royal Society of Chemistry 2024 |