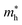Open Access Article
Open Access ArticleCreative Commons Attribution 3.0 Unported Licence
Tunable electronic structures, Rashba splitting, and optical and photocatalytic responses of MSSe-PtO2 (M = Mo, W) van der Waals heterostructures
Sadia H.
Gul
a,
Tahani A.
Alrebdi
 b,
M.
Idrees
b,
M.
Idrees
 c and
B.
Amin
c and
B.
Amin
 *a
*a
aDepartment of Physics, Abbottabad University of Science & Technology, Abbottabad 22010, Pakistan. E-mail: binukhn@gmail.com; Fax: +92-333-943-665; Tel: +92-333-943-665
bDepartment of Physics, College of Science, Princess Nourah Bint Abdulrahman University, PO Box 84428, Riyadh 11671, Saudi Arabia
cSchool of Physics and Electronic Engineering, Jiangsu University, Zhenjiang 212013, Jiangsu, China
First published on 24th August 2023
Abstract
Binding energies, AIMD simulation and phonon spectra confirm both the thermal and dynamical stabilities of model-I and model-II of MSSe-PtO2 (M = Mo, W) vdWHs. An indirect type-II band alignment in both the models of MSSe-PtO2 vdWHs and a larger Rashba spin splitting in model-II than in model-I provide a platform for experimental design of MSSe-PtO2 vdWHs for optoelectronics and spintronic device applications. Transfer of electrons from the MSSe layer to the PtO2 layer at the interface of MSSe-PtO2 vdWHs makes MSSe (PtO2) p(n)-type. Large absorption in the visible region of MoSSe-PtO2 vdWHs, while blue shifts in WSSe-PtO2 vdWHs are observed. In the case of model-II of MSSe-PtO2 vdWHs, a further blue shift is observed. Furthermore, the photocatalytic response shows that MSSe-PtO2 vdWHs cross the standard water redox potentials confirming their capability to split water into H+/H2 and O2/H2O.
1. Introduction
Although the first 2D Janus material is graphone prepared using graphene through hydrogenation via DFT,1,2 Lu (Zhang) et al.3,4 have selenized (sulfurized) MoS2 (MoSe2) through the chemical vapor deposition (CVD) technique and named the resultant product MoSSe with MXY (M = Mo, W; (X ≠ Y) = S, Se) as the general formula. The space group of MoS2 is D3h which also changed to C3v for MXY monolayers with broken symmetry.5,6 Structural stability for MXY (M = Mo, W; (X ≠ Y) = S, Se, Te) via molecular dynamics and phonon dispersion spectra has been reported in ref. 7. SOC-induced Rashba spin splitting in the electronic band dispersion in specific monolayers of the MXY family shows potential for piezoelectricity and future spintronic device applications.8–10 Employing many-body Green's function perturbation theory, substantial excitonic effects are exhibited in the MoSSe monolayer used in optoelectronic devices.11 Recently, Paul et al.12 studied Janus PtXY (X, Y = S, Se, or Te) and observed that Rashba splitting is around the M point. They also investigated whether the band gaps and the strength of the Rashba effect could be further tuned through biaxial strain.Heterostructures, fabricated by combining different 2D materials, are recognized as a helpful technique for tuning the electronic behavior of corresponding materials, for photovoltaic and nanoscale electronic devices.13–16 Vertically stacked layers are referred to as vdWHs as a result of the weak van der Waals (vdW) interactions with type-II band configuration,17,18 crucial for energy harvesting and photodetection.19 In type-II heterostructures, valence and conduction bands of one layer (layer A) are higher (in energy) than those of the other layer (layer B). The photo-generated electrons (holes) from the conduction (valence) band of layer A (B) migrate to layer B (A) which has low reduction (oxidation) potential, which results in spatial separation of electron–hole pairs, enhancing the performance of semiconductors in photocatalysis.20–22 VdWHs of TMDCs,23 MoS2–graphene,24 SiC-TMDCs,25 GeC-TMDCs,26 MoXY-WXY ((X ≠ Y) S, Se, Te),27 g-GaN-MSSe,28 BY-MX2 (Y = As, P),29 Janus Ga2SeTe/In2SSe,30 Janus-type platinum dichalcogenide heterostructures,31 PtS2/SnS2 (ref. 32), PtS2/arsenene33 and Janus-In2STe/InSe heterostructures34 have shown type-II band alignment with improved photocatalytic performance for water splitting. Li (Haleem) et al.35,36 presented a substantial Rashba spin splitting with a type-II band structure in MoSSe-WSSe (GeC-MSSe (M = Mo, W)) vdWHs, crucial for spintronic applications.
Another 2D material, PtO2, produced by exfoliation from bulk α-PtO2, survives at high temperature with better thermo-mechanical stability and superior optical absorption and carrier mobility.37,38 The PtO2 monolayer has been shown to be very closely matched with TMDCs to form vdWHs.38 It has been shown that PtO2–MoS2 (ZnO–PtO2) vdWHs with indirect (direct type-II) band alignment can be used in photocatalysis (photodetector) applications.38,39 Experimentally synthesized Ni(OH)2–PtO2 nanostructured arrays indicate enhanced hydrogen evolution reaction.40
Indeed, the small lattice mismatch, identical symmetry and high energetic feasibility of MSSe (M = Mo, W) and PtO2 led to the design of MSSe-PtO2 vdWHs. Two models of MSSe-PtO2 (M = Mo, W) vdWHs with four possible stacking configurations based on two different chalcogen atoms are fabricated. Both models of MSSe-PtO2 vdWHs with specific configuration are energetically, dynamically and thermally stable at 300 K. Furthermore, a detailed study is conducted to explore the electronic structure, Rashba spin splitting, work function, and optical and photocatalytic properties of the most stable configurations. Interestingly, we observed type-II band alignment in both models with considerable Rashba spin parameters and good photocatalytic response. These findings raised potential applications of MSSe-PtO2 vdWHs in nanoscale electronics, photovoltaics and photocatalysis.
2. Computational details
DFT with Grimme41 correction, a cut-off energy of 500 eV, a convergence criteria of 10−3 eV Å−1 (10−4 eV) for forces (energy), a k-mesh of 6 × 6 × 1 (12 × 12 × 1) and the PBE42 functional in the VASP43,44 are used for geometric relaxation (electronic structure calculations). Starting with converged PBE wave functions, the HSE06 functional45 without refining the k-mesh is also used for electronic band structure calculations. A 20 Å vacuum layer (to prevent the artifacts of the periodic boundaries along the z-axis) and the effect of SOC are also considered.46 Furthermore, using HSE06 wave functions, the Bethe–Salpeter equation was solved in the GW0 approach to study the imaginary part of the dielectric function.47AIMD48 were performed using the Nose thermostat algorithm (with 300 K and a 1 fs time interval) to investigate the thermal stabilities of the above mentioned vdWHs. Dynamical stability of these systems was investigated by using density functional perturbation theory in the phonopy code, in which the harmonic interatomic force constant is used as the input49,50
3. Results and discussion
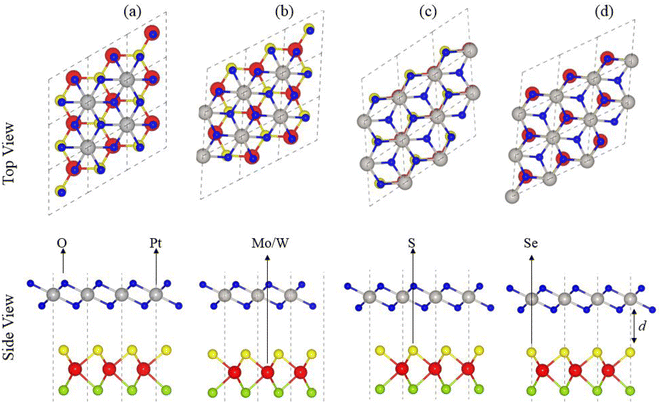
In agreement with ref. 51 and 52, optimized lattice constants (MoSSe ∼ 3.25 Å, WSSe ∼ 3.26 Å and PtO2 ∼ 3.17 Å) and bond lengths (M–S ∼ 2.411 Å, M–Se ∼ 2.39 Å and Pt–O ∼ 2.31 Å) show the reliability of our computational approach. MSSe and PtO2 have the same hexagonal lattice symmetry with a small and experimentally achievable lattice mismatch (MoSSe-PtO2 ∼ 2.46% and WSSe-PtO2 ∼ 2.27%), showing the possibility for experimental fabrication of vdWHs based on MSSe and PtO2 monolayers, i.e., MSSe-PtO2 (M = Mo, W).53As the interfacial properties are very sensitive to the layer configurations and connected atoms at the interface of vdWHs, in the case of the MSSe monolayer, two chalcogen atoms (S and Se) that terminate the surface are available for making vdWHs with the PtO2 monolayer. Therefore, we fabricated two different models of MSSe-PtO2 (M = Mo, W) vdWHs based on alternative chalcogen atoms. Each model has four possible stacking configurations, see model-I in Fig. 1. In model-I (a) stacking, the O atom of PtO2 is placed on top of the M atom of MSSe, while the S (Se) atom of MSSe and Pt atom of PtO2 are on the hexagonal site. In (b) stacking, the O atom of PtO2 is placed on top of the S (Se) atom of MSSe, while the M atom of MSSe and Pt atom of PtO2 are on the hexagonal site (just opposite of (a) stacking). In (c) stacking, the Pt atom of PtO2 is placed on top of the M atom of MSSe and one O atom of PtO2 is placed on top of the S (Se) atom of MSSe, while the other O is on the hexagonal site. In (d) stacking, the Pt atom of PtO2 is placed on top of the S (Se) atom of MSSe and one O atom of PtO2 is placed on top of the M atom of MSSe, while the other O atom is on the hexagonal site (just opposite of stacking (c)), see Fig. 1. Similar stacking configurations are also made for model-II with the alternative positions of S and Se atoms of MSSe layers.
Interlayer distance (d), binding energies (Eb), optimized lattice constant (a) and bond length for each stacking configuration in both model-I and model-II of MSSe-PtO2 vdWHs in Table 1 are in the range of those of other vdWHs in ref. 27 and 28. Favorable stacking configuration ((a) stacking) in both models of MSSe-PtO2 vdWHs has the most negative binding energy and small interlayer distance, which shows higher energetic stability and strong physical and vdW interaction between MSSe and PtO2 layers. Slightly small vertical distances and high energetic feasibilities in model-II are due to the larger covalent radius of Se than the S atom at the interface which results in more attractive energy by making these stacking configurations, see Table 1. A small lattice mismatch induces minor strain in the corresponding monolayers (compressed Pt–O, while stretched M–S and M–Se bond lengths) of MSSe-PtO2 vdWHs, in agreement with ref. 26.
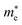 and
and 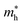 ) for MSSe-PtO2 vdWHs
) for MSSe-PtO2 vdWHs
AIMD simulations for the most stable stacking configuration ((a) stacking) of MoSSe-PtO2 vdWHs in model-I, in Fig. 2(a), show a small variation in the total energy, while no distortion was found in the structure after 6 ps, hence confirming the thermal stability at 300 K. The phonon band structure of the same vdWH for model-I, in Fig. 2(b), is free from imaginary frequency, hence confirming the dynamic stability of MSSe-PtO2 (M = Mo, W) vdWHs. Similar trends were also found for other vdWHs in both model-I and model-II.
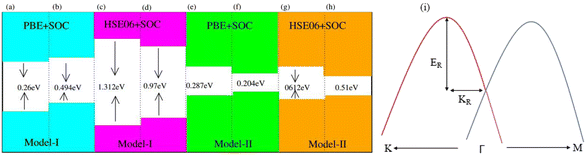
Generally, in DFT, the choice of the functional (conventional or hybrid) affects the band structure and bandgap values. Therefore, we have used both PBE and HSE06 functionals to calculate the electronic band structure of MSSe-PtO2 vdWHs, see Fig. 3. In both models, the VBM (CBM) is pinned at the K (M-Γ)-point of the first BZ and hence reveals the indirect bandgap nature of MSSe-PtO2 vdWHs. The bandgap values calculated by the HSE06 method are larger than those obtained by the calculations at the PBE level, which are further decreased by including the SOC effect due to the VBM/CBM spin splitting and mirror symmetry breaking in MSSe monolayers, see Table 1 and Fig. 4(a)–(h). The bandgap values also decrease from Mo to W and also from model-I to model-II which may be due to the different chalcogen atoms attached to PtO2 layers in MSSe-PtO2 vdWHs. Replacing one of the similar chalcogen atoms in the MX2 monolayer by a different one, with vertical stacking in vdWHs, breaks the inversion symmetry, due to which an electric field is produced and generates Rashba spin splitting at the Γ-point near the Fermi level (see Fig. 3 and schematic representation in Fig. 4(i));54 calculated using αR = 2ER/KR. ER (KR) is the Rashba energy (moment offset at the Γ-point, known as the Rashba wave vector). αR values calculated for MSSe-PtO2 vdWHs in model-I (II) are presented in Table 1. It is important to address that αR for MSSe-PtO2 vdWHs is smaller than that of the corresponding isolated monolayer,26 which may be due to the intrinsic electric field.55 Furthermore, the αR values increase from model-I to -II and also from MoSSe-PtO2 to WSSe-PtO2 vdWHs which is due to the selective atom of chalcogen atom and heavier W atom than the Mo atom. Hence, MSSe-PtO2 vdWHs with considerable αR values set a platform for practical application in spintronic devices.
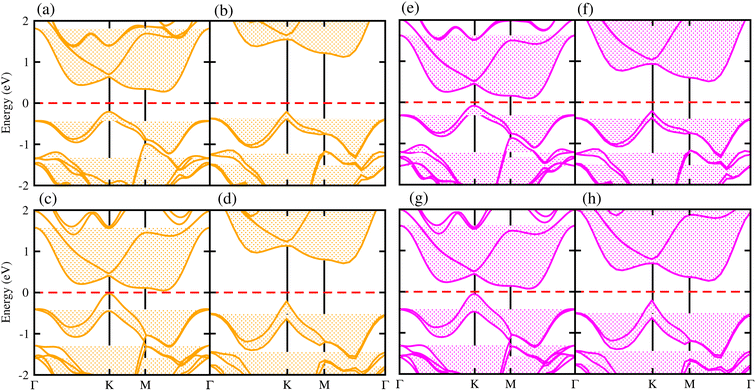 | ||
| Fig. 3 Electronic band structures of MoSSe-PtO2 (a(b) and e(f)) and WSSe-PtO2 vdWHs (c(d) and g(h)), using the PBE (HSE06) method. The left(right) column is for model-I (-II). | ||
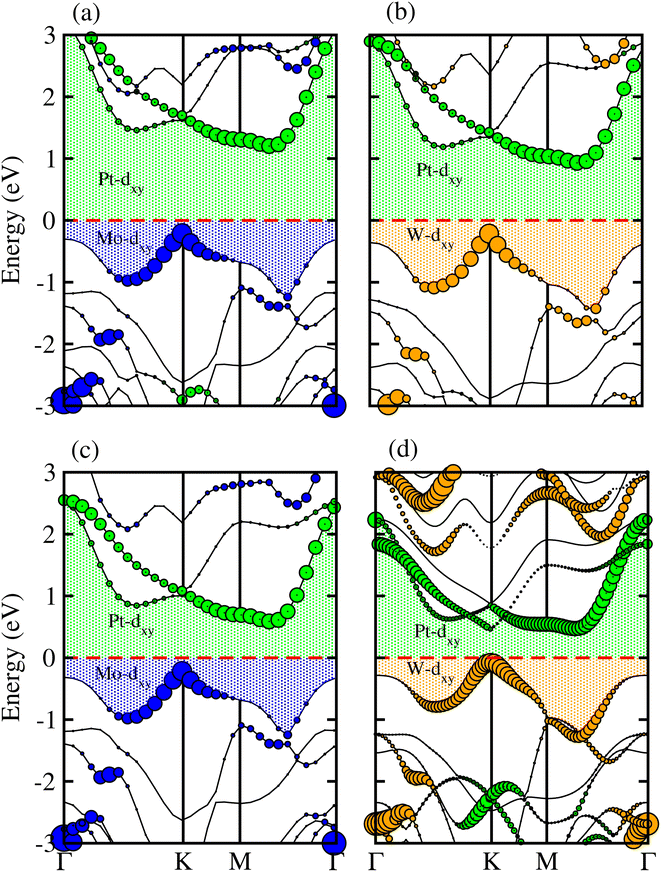
Partial band structures of MSSe-PtO2 (M = Mo, W) vdWHs, depicted in Fig. 5, show that the M-dxy (Pt-dxy) state of the MSSe (PtO2) layer mainly contributes to the VBM (CBM) of MSSe-PtO2 vdWHs. Localization of the VBM and CBM from the isolated MSSe and PtO2 layers confirms type-II (staggered) band alignment in MSSe-PtO2 vdWHs, responsible for charge carrier separation.35,36 Hence in the case of MSSe-PtO2 vdWHs, photogenerated carriers move from different layers (electrons (holes) move from the MSSe (PtO2) layer to the PtO2 (MSSe) layer), which may decrease their recombination rate and hence play an important role in photocatalysis and solar cell application.
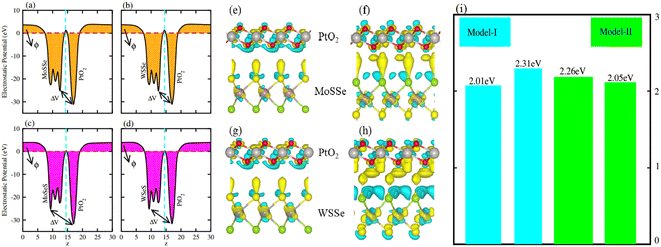
Average electrostatic potential (Fig. 6(a)–(d)) and the charge density difference (CDD) (Fig. 6(e)–(h)) are also calculated to understand both the qualitative and quantitative behaviour of the transfer of charge among the layers of MSSe-PtO2 (M = Mo, W) vdWHs. One can see that the PtO2 layer has deeper potential than the MSSe layer, indicating that electrons are moving from the MSSe layer to the PtO2 layer at the interface of MSSe-PtO2 vdWHs. The CDD in Fig. 6(e)–(h) confirms the charge transfer from the MSSe layer to PtO2; hence the MSSe (PtO2) monolayer becomes p(n)-doped after stacking. The deeper potential of S than Se is due to the electronegativity difference. Potential drops between MSSe and PtO2 layers, presented in Table 1 for model-I and model-II, differentiate the excitonic behavior of free-standing monolayers from the corresponding vdWHs, which helps promote photogenerated carrier (electrons and holes) separation. Quantitative charge transfer is also investigated by Bader charge analysis, which shows that about 0.086e (0.097e) and 0.075e (0.91e) are transferred from the MoSSe and WSSe layer to the PtO2 layer in model-I (-II) of MSSe-PtO2 vdWHs. This small transfer of charges from the MSSe layer to the PtO2 layer confirms weak vdW interactions between the layers of heterostructures. The work function (ϕ) is also calculated by using ϕ = Evac − EF, where Evac is the energy difference of the vacuum potential (derived from the electrostatic potential (see Fig. 6(i)) in the direction normal to the surface with large vacuum separation) to Fermi energy EF (given from the ground state DFT calculations). The calculated values for ϕ in Table 1 are efficient for formation of the interface and charge transfer. One can observe that the values of ϕ increase from model-I to model-II and also from MoSSe-PtO2 to WSSe-PtO2 vdWHs, which may be due to the selective atom of chalcogen atom and heavier W atom than the Mo atom. Fabrication of MSSe-PtO2 (M = Mo, W) vdWHs modulates the band structure of the corresponding monolayers, hence the effective masses. Therefore, we have calculated the effective mass of MSSe-PtO2 (M = Mo, W) vdWHs by using parabolic fitting to the band edge, given in ref. 56; 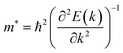 . The calculated effective mass of electrons (holes) is
. The calculated effective mass of electrons (holes) is 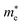 (
(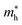 ), given in Table 1. One can see that the
), given in Table 1. One can see that the 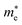 values are smaller than the corresponding
values are smaller than the corresponding 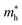 values, while smaller values for
values, while smaller values for 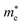 are further observed in WSSe-PtO2 (M-II) and for
are further observed in WSSe-PtO2 (M-II) and for 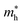 in MoSSe-PtO2 (M-I). High (smaller) carrier mobility (effective mass) is preferable for electronic and optoelectronic devices.57 From Table 1 it’s clear that WSSe-PtO2 (M-I) has miniaturized effective masses, hence sponsors high charge carrier mobility, and therefore, shows good response towards high performance device applications.57
in MoSSe-PtO2 (M-I). High (smaller) carrier mobility (effective mass) is preferable for electronic and optoelectronic devices.57 From Table 1 it’s clear that WSSe-PtO2 (M-I) has miniaturized effective masses, hence sponsors high charge carrier mobility, and therefore, shows good response towards high performance device applications.57
We have further explored the optical response of MSSe-PtO2 vdWHs in terms of ε2 (ω), directly associated with the band structure and the bandgap values,58,59 shown in Fig. 7. The optical absorption range for MoSSe and WSSe monolayers is about 2 to 5.0 eV,27 while for Mo(W)SSe-PtO2 vdWHs in model-I and model-II it is from 2.5 (3.0) to 7.0 (8.0) eV. Broader absorption in the visible region of MoSSe-PtO2 than WSSe-PtO2 vdWHs is due to the smaller bandgap of the former, while a blue shift is observed in the latter. A further blue shift in model-II than in model-I of MSSe-PtO2 vdWHs may be due to the selective atom of chalcogen atom at the interface. Although there is a blue shift in the absorption spectrum of MSSe-PtO2 vdWHs from model-I to -II, the qualitative behaviour of the peak is similar for both models. High optical absorption requires a wide range peak, which may occupy a large number of states near the Fermi level. Broadened optical absorption of vdWHs is due to high carrier density (compared to parent monolayers).60 Obviously, the fabrication of vdWHs is an effective way to understand the modulation of absorption performance of 2D layered materials. We predict that MoSSe-PtO2 vdWHs for model-I (-II) have good optical absorption in visible regions, which makes them suitable for practical applications in nanoelectronics and optoelectronic devices.61
In photocatalytic activity, illumination of solar light on semiconductors separates charge carriers in conduction and valence bands. Using Mulliken electronegativity (χ)62 and standard electrode potential on the hydrogen scale (Eelec = 4.5 eV), energies of valence (EVBM) and conduction (EVBM) band edge potentials at pH = 0 are EVBM = χ − Eelec − 0.5 Eg and ECBM = EVBM − Eg. Standard water redox potentials are −4.50 (−5.73) eV for reduction (oxidation) i.e., H+/H2 (H2O/O2),23,63 which show that the size of the bandgap and energy of the band edge potentials are the basic criteria to facilitate water splitting reactions. Both EVBM and ECBM of MSSe-PtO2 (M = Mo, W) vdWHs in model-I and model-II are presented in Fig. 8. In the case of model-I of MSSe-PtO2 vdWHs, both EVBM and ECBM cross the standard redox and oxidation potential at pH = 0, and hence show the capacity for full water splitting. In model-II of MSSe-PtO2 vdWHs, EVBM crosses standard redox potential, hence having the ability to reduce water at H+/H2 but failing for oxidation (O2/H2O). It is clear from the above discussion and Fig. 8 that photocatalytic water splitting activity is very sensitive to the order of chalcogen atoms attached at the interface of vdWHs. Similar photocatalytic water splitting is also demonstrated in ref. 29.
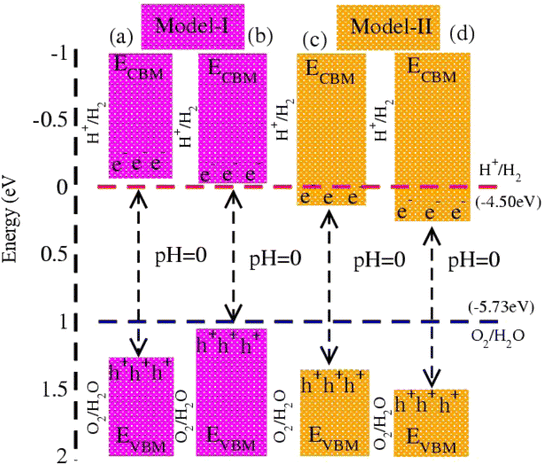 | ||
| Fig. 8 Photocatalytic water splitting of MSSe-PtO2 vdWHs, where blue and red dotted lines represent reduction and oxidation potentials at pH = 0. | ||
4. Conclusion
In summary, using first principles calculations, electronic structures, Rashba splitting, and optical and photocatalytic properties of MSSe-PtO2 (M = Mo, W) vdWHs are investigated. Binding energies, AIMD simulation and phonon spectra confirm the thermal and dynamical stabilities of MSSe-PtO2 vdWHs in model-I and model-II. Electronic band structures show that MSSe-PtO2 vdWHs are indirect type-II semiconductors. Interestingly Rashba spin splitting observed in both model-I and model-II of MSSe-PtO2 vdWHs, with larger Rashba parameters in model-I than in model-II, provides a platform for experimental design of MSSe-PtO2 vdWHs for optoelectronics and spintronic device applications. Transfer of electrons from the MSSe layer to the PtO2 layer at the interface of MSSe-PtO2 vdWHs makes the MSSe (PtO2) monolayer p(n)-doped after stacking. Broad absorption occurs in the visible region of MoSSe-PtO2 vdWHs and a blue shift is observed in WSSe-PtO2 vdWHs. A further blue shift is also observed in model-II than in model-I of MSSe-PtO2 vdWHs. MSSe-PtO2 vdWHs in model-I are also found to be exciting materials for water splitting and suggested for low cost hydrogen production.Conflicts of interest
There are no conflicts to declare.Acknowledgements
The research was funded by Princess Nourah Bint Abdulrahman University Supporting Project Number PNURSP2023R71. The authors extend their sincere appreciation to Princess Nourah Bint Abdulrahman University Researchers Supporting Project number (PNURSP2023R71), Princess Nourah Bint Abdulrahman University, Riyadh, Saudi Arabia.References
- S.-W. Ng, N. Noor and Z. Zheng, NPG Asia Mater., 2018, 10, 217 CrossRef CAS.
- J. Zhou, Q. Wang, Q. Sun and X. S. Chen, Nano Lett., 2009, 9, 3867 CrossRef CAS PubMed.
- A.-Y. Lu, H. Zhu, J. Xiao, C.-P. Chuu, Y. Han, M.-H. Chiu, C.-C. Cheng, C.-W. Yang, K.-H. Wei, Y. Yang, Y. Wang, D. Sokaras, D. Nordlund, P. Yang, D. A. Muller, M.-Y. Chou, X. Zhang and L.-J. Li, Nat. Nanotechnol., 2017, 12, 744 CrossRef CAS PubMed.
- J. Zhang, S. Jia, I. Kholmanov, L. Dong, D. Er, W. Chen, H. Guo, Z. Jin, V. B. Shenoy, L. Shi and J. Lou, ACS Nano, 2017, 11, 8192 CrossRef CAS PubMed.
- L. Dong, J. Lou and V. B. Shenoy, ACS Nano, 2017, 11, 8242 CrossRef CAS.
- R. Li, Y. Cheng and W. Huang, Small, 2018, 14, 1802091 CrossRef PubMed.
- A. B. Maghirang, Z. Q. Huang, R. A. B. Villaos, C. H. Hsu, L. Y. Feng, E. Florido, H. Lin and A. Bansil, npj 2D Mater., 2019, 3, 35 CrossRef.
- A. Kaur, S. Sharma, P. Nandi and A. D. Sarkar, Phys. E, 2023, 154, 115791 CrossRef CAS.
- J. Chen, K. Wu, W. Hu and J. Yang, J. Phys. Chem. Lett., 2021, 12(51), 12256 CrossRef CAS PubMed.
- M.-Y. Liu, L. Gong, Y. He and C. Cao, Phys. Rev. B, 2021, 103, 075421 CrossRef CAS.
- F. Li, W. Wei, B. Huang and Y. Dai, J. Phys. Chem. C, 2019, 124, 1667 CrossRef.
- P. A. L. Sino, L. Y. Feng, R. A. B. Villaos, H. N. Cruzado, Z. Q. Huang, C. H. Hsu and F. C. Chuang, Nanoscale Adv., 2021, 3, 6608 RSC.
- A. K. Geim and I. V. Grigorieva, Nature, 2013, 499, 419 CrossRef CAS PubMed.
- Q. Sun, Y. Dai, Y. Ma, W. Wei and B. Huang, J. Phys. Chem. Lett., 2015, 6, 2694 CrossRef CAS PubMed.
- W. Xia, L. Dai, P. Yu, X. Tong, W. Song, G. Zhang and Z. Wang, Nanoscale, 2017, 9, 4324 RSC.
- K. D. Pham, N. N. Hieu, H. V. Phuc, I. Fedorov, C. Duque, B. Amin and C. V. Nguyen, Appl. Phys. Lett., 2018, 113, 171605 CrossRef.
- M.-H. Chiu, C. Zhang, H.-W. Shiu, C.-P. Chuu, C.-H. Chen, C.-Y. S. Chang, C.-H. Chen, M.-Y. Chou, C.-K. Shih and L.-J. Li, Nat. Commun., 2015, 6, 7666 CrossRef CAS.
- L. Kou, T. Frauenheim and C. Chen, J. Phys. Chem. Lett., 2013, 4, 1730 CrossRef CAS PubMed.
- R. Bose, G. Manna, S. Jana and N. Pradhan, Chem. Commun., 2014, 50, 3074 RSC.
- J. Ge, Y. Zhang, Y.-J. Heo and S.-J. Park, Catalysts, 2019, 9, 122 CrossRef.
- A. Shirmardi, M. A. M. Teridi, H. R. Azimi, W. J. Basirun, F. Jamali-Sheini and R. Yousefi, Appl. Surf. Sci., 2018, 462, 730 CrossRef CAS.
- M. T. Uddin, Y. Nicolas, C. Olivier, T. Toupance, L. Servant, M. M. Müller, H.-J. Kleebe, J. Ziegler and W. Jaegermann, Inorg. Chem., 2012, 51, 7764 CrossRef CAS PubMed.
- B. Amin, N. Singh and U. Schwingenschlögl, Phys. Rev. B: Condens. Matter Mater. Phys., 2015, 92, 075439 CrossRef.
- F. Ye, J. Lee and P. X.-L. Feng, Nanoscale, 2017, 9, 18208 RSC.
- H. U. Din, M. Idrees, G. Rehman, C. V. Nguyen, L.-Y. Gan, I. Ahmad, M. Maqbool and B. Amin, Phys. Chem. Chem. Phys., 2018, 20, 24168 RSC.
- K. Ren, C. Ren, Y. Luo, Y. Xu, J. Yu, W. Tang and M. Sun, Phys. Chem. Chem. Phys., 2019, 21, 9949 RSC.
- M. Idrees, H. U. Din, R. Ali, G. Rehman, T. Hussain, C. V. Nguyen, I. Ahmad and B. Amin, Phys. Chem. Chem. Phys., 2019, 21, 18612 RSC.
- M. Idrees, C. V. Nguyen, H. D. Bui, I. Ahmad and B. Amin, Phys. Chem. Chem. Phys., 2020, 22, 20704 RSC.
- H. U. Din, M. Idrees, Q. Alam and B. Amin, Appl. Surf. Sci., 2021, 568, 150846 CrossRef CAS.
- H.-F. Lin, H.-Y. Liu, M. Wang, S.-S. Wang, T.-P. Hou and K.-M. Wu, Phys. Chem. Chem. Phys., 2022, 24, 4425–4436 RSC.
- Z. Kahraman, A. Kandemir, M. Yagmurcukardes and H. Sahin, J. Phys. Chem. C, 2019, 123(7), 4549–4557 CrossRef CAS.
- L. Zhu, Y.-F. Ding, W.-J. Yang, S.-F. Yin and M.-Q. Cai, Phys. Chem. Chem. Phys., 2021, 23, 18125–18136 RSC.
- K. Ren, W. Tang, M. Sun, Y. Cai, Y. Cheng and G. Zhang, Nanoscale, 2020, 12, 17281–17289 RSC.
- X. Li, B. Zhai, X. Song, Y. Yan, J. Li and C. Xia, Appl. Surf. Sci., 2020, 509, 145317 CrossRef CAS.
- F. Li, W. Wei, P. Zhao, B. Huang and Y. Dai, J. Phys. Chem. Lett., 2017, 8, 5959 CrossRef CAS PubMed.
- H. U. Din, M. Idrees, A. Albar, M. Shafiq, I. Ahmad, C. V. Nguyen and B. Amin, Phys. Rev. B, 2019, 100, 165425 CrossRef CAS.
- M. S. H. Khan, M. S. Islam, M. R. Islam, A. Iskanderani, I. M. Mehedi and M. T. Hasan, IEEE Access, 2021, 19, 109510 Search PubMed.
- J. Li and S.-Y. Xie, Phys. Lett. A, 2020, 384, 126286 CrossRef CAS.
- A. Shokri, A. Yazdani and K. Rahimi, Mater. Chem. Phys., 2020, 255, 123617 CrossRef CAS.
- L. Xie, X. Ren, Q. Liu, G. Cui, R. Ge, A. M. Asiri, X. Sun, Q. Zhang and L. Chen, J. Mater. Chem. A, 2018, 6, 1967 RSC.
- S. Grimme, J. Comput. Chem., 2006, 27, 1787 CrossRef CAS.
- J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1996, 77, 3865 CrossRef CAS PubMed.
- W. Kohn and L. J. Sham, Phys. Rev., 1965, 140, 1133 CrossRef.
- J. Hafner, J. Comput. Chem., 2008, 29, 2044–2078 CrossRef CAS.
- J. Heyd, G. E. Scuseria and M. Ernzerhof, J. Chem. Phys., 2003, 118, 8207–8215 CrossRef CAS.
- D. Kochan, S. Irmer and J. Fabian, Phys. Rev. B, 2017, 95, 165415 CrossRef.
- L. Chiodo, J. M. G. Lastra, A. Iacomino, S. Ossicini, J. Zhao, H. Petek and A. Rubio, Phys. Rev. B: Condens. Matter Mater. Phys., 2010, 82, 045207 CrossRef.
- R. Yuan, J. A. Napoli, C. Yan, O. Marsalek, T. E. Markland and M. D. Fayer, ACS Cent. Sci., 2019, 5, 1269 CrossRef CAS PubMed.
- S. Baroni, S. De Gironcoli and A. Dal, Rev. Mod. Phys., 2001, 73, 515 CrossRef CAS.
- A. Togo, F. Oba and I. Tanaka, Phys. Rev. B: Condens. Matter Mater. Phys., 2008, 78, 134106 CrossRef.
- C. Xia, W. Xiong, J. Du, T. Wang, Y. Peng and J. Li, Phys. Rev. B, 2018, 98, 165424 CrossRef CAS.
- A. Shokri, A. Yazdani and K. Rahimi, Mater. Chem. Phys., 2020, 255, 123617 CrossRef CAS.
- M. Liao, P. Nicolini, L. Du, J. Yuan, S. Wang, H. Yu and G. Zhang, Nat. Mater., 2022, 21, 47 CrossRef CAS PubMed.
- P. S. Eldridge, W. J. H. Leyland, P. G. Lagoudakis, R. T. Harley, R. T. Phillips, R. Winkler, M. Henini and D. Taylor, Phys. Rev. B: Condens. Matter Mater. Phys., 2010, 82, 045317 CrossRef.
- S. Patel, U. Dey, N. P. Adhikari and A. Taraphder, arXiv, 2021, preprint, arXiv:2112.15457, DOI:10.1103/PhysRevB.106.035125.
- A. Patel, D. Singh, Y. Sonvane, P. B. Thakor and R. Ahuja, ACS Appl. Mater. Interfaces, 2020, 12, 46212 CrossRef CAS PubMed.
- X. D. Liu, Y. Huang and X. Duan, Chem. Soc. Rev., 2018, 47, 6388 RSC.
- Y. G. Zhang, G. B. Zhang and Y. Xu Wang, J. Appl. Phys., 2011, 109, 063510 CrossRef.
- Q. Zhang, X. Chen, W. C. Liu and Y. Wang, Comput. Mater. Sci., 2019, 158, 272–281 CrossRef CAS.
- M. Fox, Optical Properties of Solids, Mark Fox Oxford University Press, 2010 Search PubMed.
- A. Moliton and R. C. Hiorns, Polym. Int., 2004, 53, 1397 CrossRef CAS.
- X. Ma, X. Wu, H. Wang and Y. Wang, J. Mater. Chem., 2018, 6, 2295 RSC.
- V. Chakrapani, J. C. Angus, A. B. Anderson, S. D. Wolter, B. R. Stoner and G. Sumanasekera, Science, 2007, 318, 1424 CrossRef CAS PubMed.
| This journal is © The Royal Society of Chemistry 2023 |