The cubic structure of Li₃As stabilized by pressure or configurational entropy via the solid solution Li₃As–Li₂Se

Abstract

The hexagonal to cubic phase transition of Li₃As was investigated at high pressure and temperature, revealing a cubic high-pressure polymorph in the Li₃Bi structure type. This cubic structure type is preserved in the solid solution of Li₃As–Li₂Se synthesized via mechanochemical ball milling. The solid solutions were investigated via X-ray powder diffraction, showing a linear dependency of the lattice parameter a on the mole fraction of the boundary phases Li₃As and Li₂Se, according to Vegard's law. Configurational entropy is generated by mixed anion lattice occupation between arsenide and selenide and therefore stabilizes the cubic structure of the solid solution. At elevated temperatures, the solid solution of Li₃As–Li₂Se reveals an exsolution process by forming the boundary phases Li₃As and Li₂Se, proving the metastable character of the system. Impedance spectroscopy was used to determine the lithium-ion conductivities in the Li₃As–Li₂Se system, showing significantly higher conductivity values (∼10⁻⁴ to 10⁻⁶ S cm⁻¹ at 50 °C) compared to the pure end members Li₃As (∼10⁻⁷ S cm⁻¹ at 50 °C) and Li₂Se (∼10⁻⁷ S cm⁻¹ at 175 °C).

---

Introduction

For all binary lithium pnictogenides Li₃Pn (Pn = N, P, As, Sb, Bi), a cubic modification in the Li₃Bi structure type is mentioned in the literature; however, to the best of our knowledge, for the cubic modification of Li₃As, no experimental data are available in scientific publications.^1,2 In 2022, we reported lithium-ion mobility in Li₃As and calculated the phase stability of the cubic high-pressure modification via DFT (density functional theory).³ Herein, we report experimental results on the cubic polymorph of Li₃As, i.e., X-ray powder diffraction (XRPD) of a quenched sample obtained from high-pressure and high temperature conditions in a belt press. Thus, this missing part in the puzzle for Li₃Pn (Pn = N, P, As, Sb, Bi) is available now.

We further show that the pressure induced cubic structure of Li₃As can also be stabilized by the formation of a solid solution in the Li₃As–Li₂Se system. Two examples for solid solutions between binary lithium chalcogenides and lithium pnictogenides are known in the literature. Restle et al. reported a solid solution of Li₃N and Li₂O in a rigid open framework boron structure (Li₆B₁₈(Li₃N)_1−x(Li₂O)_x, 0 ≤ x ≤ 1), since the authors claim that pristine Li₃N and Li₂O are not miscible in the solid state.^4,5 The second example for solid solutions of binary lithium chalcogenides and lithium pnictogenides was reported by Szczuka et al. in the Li₃P–Li₂S system, showing miscibility between the two phases in the range of 0.39 ≤ x ≤ 0.75 (xLi₃P–(1 − x)Li₂S).⁶ The two boundary phases Li₂S and Li₃P crystallize in the cubic antifluorite structure type and the hexagonal Na₃As structure type, respectively. Despite the significant structural difference between Li₂S and Li₃P under ambient conditions, a solid solution in the antifluorite structure type is formed. The authors explain the miscibility by the fact that under pressure (4 GPa) Li₃P undergoes a hexagonal to cubic phase transition. Since the synthesis of the solid solution is carried out by high-energy ball milling of mixtures of Li₃P and Li₂S, the phase transition of Li₃P could be induced mechanochemically to form a cubic structure which is compatible with the cubic structure of Li₂S.⁶ High temperature experiments reveal the decomposition of the solid solution into Li₃P and Li₂S at elevated temperatures (400–500 °C) indicating thermal stability of the solid solution only at lower temperatures.⁶ Investigations on lithium-ion mobility show that the samples of the mixtures exhibit significantly higher lithium-ion mobility (∼10⁻⁴ to 10⁻⁵ S cm⁻¹) than the boundary phases (Li₂S: 10⁻¹⁴ to 10⁻¹⁰ S cm⁻¹, Li₃P: 10⁻⁷ S cm⁻¹).^6–8

Li₃Pn (Pn = N, P, As, Sb, Bi) compounds that adopt the cubic structure type under less extreme conditions are Li₃Sb and Li₃Bi. Li₃Bi indeed crystallizes in the cubic structure type under ambient conditions. In a detailed computational study, Hautier et al. discussed the potential application of Li₃Sb as a high-mobility p-type transparent conductor.⁹ Furthermore, Li₃Sb and Li₃Bi have been theoretically predicted to be above-average thermoelectric materials.^10,11 The improved thermoelectric performance of Li₃Sb samples that transform to the cubic polymorph via ball milling was demonstrated by Snyder and Aldemir et al.¹²

In this work, we show that the system with the higher homologues (Li₃As–Li₂Se) has similar properties to the solid solution of Li₃P–Li₂S. X-ray powder diffraction data of ball milled samples with the stoichiometry of Li_3−xAs_1−xSe_x (0.2 ≤ x ≤ 1) confirm the formation of a solid solution in a cubic structure. Analogous to the Li₃P–Li₂S system, the formation of the solid solution in the Li₃As–Li₂Se system can be rationalized by the accessibility of the high-pressure modification of Li₃As via mechanochemical synthesis since high local temperature and pressure are involved during ball milling. Configurational entropy introduced by the cubic mixed anion lattice (As³⁻ and Se²⁻) in the solid solution could stabilize its cubic structure. Enhancing phase stability by introducing high configurational entropy to a structure is well known for cations in high entropy oxides which have gained significant interest over the recent decade.^13–15 However, stabilizing entropic contributions from anions are rarely mentioned in the literature. With the multi-anionic and -cationic oxofluoride Li_x(Co_0.2Cu_0.2Mg_0.2Ni_0.2Zn_0.2)OF_x, Wang et al. showed the stabilizing entropic effect originating from the mixed anion lattice (O²⁻/F⁻) revealing promising properties for use as a cathode material in lithium-ion batteries.¹⁶

Results and discussion

The cubic high-pressure modification of Li₃As

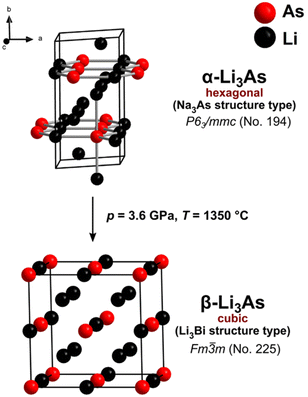
For the cubic to hexagonal phase transition of Li₃As, Beister et al. reported a pressure of 4.5 GPa, but apart from the conversion pressure, no further information or experimental data was published.²

In this work we present the synthesis of the cubic structure of Li₃As by applying high-pressure (3.6 GPa) and high temperature (1350 °C) on a phase pure sample of hexagonal Li₃As (Na₃As structure type) using a belt press. XRPD was performed on a quenched sample. The powder diffraction pattern in Fig. 1 reveals high intensities of the cubic phase of Li₃As, which was indexed and refined (see the ESI‡) with a profile function, leading to the lattice parameter a = 6.214(1) Å. Indexing and refinement of the cubic phase was performed based on the Li₃Bi structure type with the space group Fm3̄m (no. 225). The corresponding calculated diffraction profile of the cubic structure model shows perfect agreement with the measured diffraction pattern (Fig. 1). Previous DFT modelling by our group on the phase stability of Li₃As under high-pressure conditions predicted the cubic phase of Li₃As in the Li₃Bi structure type with the lattice parameter a = 6.1909 Å which differs only by 0.4% from the experimental data.³ These findings confirm a hexagonal to cubic phase transition (α-Li₃As → β-Li₃As) at high pressure and high temperature for the compound under study (Fig. 2). The still pronounced reflections of hexagonal α-Li₃As (36%) prove that the hexagonal to cubic phase transition is not completed under the applied conditions. The cubic modification of Li₃As could be stabilized after quenching the sample from high pressure and high temperature to ambient conditions. Quenching is crucial to prevent the reverse cubic to hexagonal phase transition, which is observed during slow pressure and temperature reduction to ambient conditions. A similar behavior was found for the related compound Li₃P by Leonova et al.¹⁷ In situ XRPD measurements under decreasing pressure from high to ambient pressure reveal the reverse cubic to hexagonal phase transition for Li₃P.¹⁷ The heavier homologue Li₃Sb also behaves similarly, although the cubic structure is adopted after ball-milling, independent of the starting material (from the elements or transformation from hexagonal Li₃Sb). Thus, the application of high pressure forces the phase transformation of Li₃Sb at ambient temperature,¹² while additional high temperature is necessary for Li₃As. A minimal amount of the cubic polymorph already forms at 700 °C (Fig. S4‡). Furthermore, the metastable nature of the cubic polymorph of Li₃Sb was proven by annealing the cubic samples, which then transformed to the hexagonal phase.¹²

Fig. 1 Powder diffractogram of Li₃As after quenching from high-pressure and temperature to ambient conditions (black). Intensities marked with red stars can be assigned to hexagonal Li₃As (∼36%). The intensity marked in blue cannot be assigned to any phase. Negative intensities show the calculated cubic Li₃As modification in the space group Fm3̄m (no. 225) with lattice parameters determined from the measured diffractogram.

Fig. 2 Scheme of the hexagonal to cubic phase transition of Li₃As at 3.6 GPa and 1350 °C.

The solid solution in the Li₃As–Li₂Se system

In the ternary Li–As–Se system, the phases Li₂Se,¹⁸ LiAs,¹⁹ Li₃As,²⁰ Li₃As₇,²¹ As₄Se₃,²² As₄Se₄,²³ As₂Se₃,²⁴ AsSe₂,²⁵ and LiAsSe₂ ²⁶ are known (Fig. 3). The solid solution Li_3−xAs_1−xSe_x (0.2 ≤ x ≤ 1) is highlighted in red in Fig. 3 between the two binary phases Li₃As and Li₂Se.

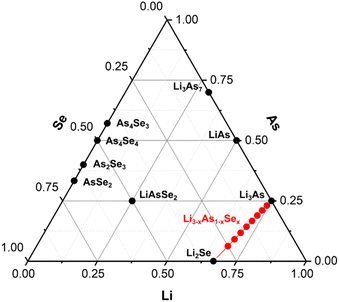Fig. 3 Ternary phase diagram of the Li–As–Se system. The solid solution Li_3−xAs_1−xSe_x (0.2 ≤ x ≤ 1) is displayed in red.

Synthesis and structure

Since the solid solution in the Li₃As–Li₂Se system was not accessible via classical solid-state reaction, it was realized by mechanochemical synthesis of the corresponding elements via ball milling. Ten samples in steps of x = 0.1 between Li₃As and Li₂Se (Li_3−xAs_1−xSe_x) were synthesized. To obtain phase pure samples, the number of ball milling cycles (5 minutes of milling followed by 4 minutes of sample equilibration) was adjusted in the range of 12 to 36, since higher proportions of Li₃As need more mechanochemical treatment (higher energy input) to form a solid solution with Li₂Se. In Fig. 4 all powder diffractograms of the prepared samples are shown depending on their composition. Since samples with a stoichiometric ratio of 1 : 9 for Li₃As : Li₂Se (equivalent to x = 0.1) show no significant formation of the cubic phase after several ball milling cycles, it is concluded that a miscibility gap is present near Li₃As in the solid solution with Li₂Se. Nevertheless, in the range of 0.2 ≤ x ≤ 1 (Li_3−xAs_1−xSe_x), all samples show XRPD pattern profiles originating from Li₂Se, which shift to lower 2θ values with increasing mole fractions of Li₃As. The cubic lattice parameter a of all samples including pure Li₂Se and the cubic modification of Li₃As was obtained from the corresponding diffraction patterns (Table S1‡) and plotted against the mole fraction x, as shown in Fig. 5. The lattice parameters of the solid solution show a Vegard-like trend, since the experimentally determined values exhibit deviations of less than 0.5% from the arithmetic mean of the lattice parameters from β-Li₃As and Li₂Se.²⁷ The small deviations, however, can be attributed to minimal amounts of oxygen in the samples, incomplete reactions or reactions with the container material, although these cannot be quantified.

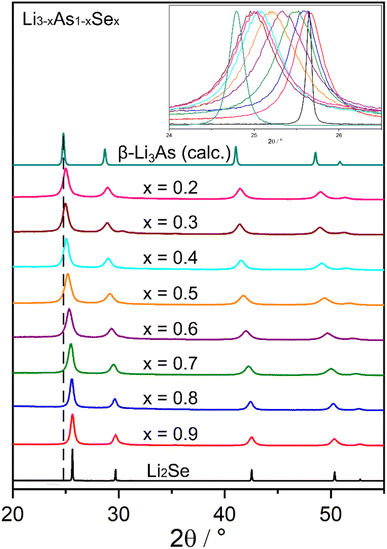Fig. 4 Powder diffractograms of the solid solution Li_3−xAs_1−xSe_x (0.2 ≤ x ≤ 1). The powder diffractogram of β-Li₃As was calculated.

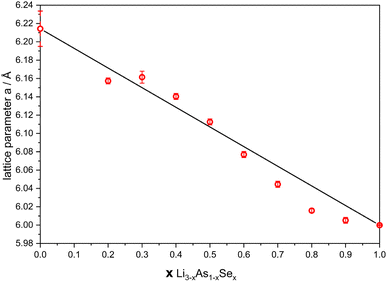Fig. 5 Lattice parameter a plotted against the mole fraction x of the solid solution Li_3−xAs_1−xSe_x (0.2 ≤ x ≤ 1).

Therefore, the stoichiometric compositions of the samples correspond with the lattice parameter a and the structure of the solid solution can be described as a cubic closed packing of arsenide and selenide ions forming a mixed occupancy site (Fig. 6). The tetrahedral voids are fully occupied with lithium cations and the octahedral voids are partly filled with lithium cations depending on the arsenide : selenide ratio of the anions, since the arsenic anions carry a higher negative charge.

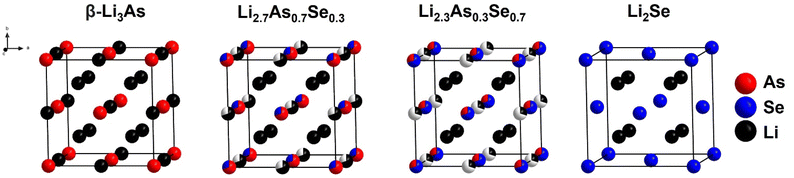Fig. 6 Structural progression of the solid solution in the system Li_3−xAs_1−xSe_x (0.2 ≤ x ≤ 1) with the parent compounds β-Li₃As and Li₂Se.

Since Li₃As (hexagonal) and Li₂Se (cubic) differ in their crystal systems under ambient conditions, complete miscibility of both phases should not be possible in the solid state. Nevertheless, a solid solution is formed and a similar behavior has been reported for the lighter homologues in the Li₃P (hexagonal) and Li₂S (cubic) systems.⁶ This can be rationalized by the fact that Li₃As and Li₃P possess a cubic high-pressure modification, which may become accessible during the ball milling process. In the presence of Li₂Se a metastable solid solution with the cubic high-pressure modification of Li₃As is formed. The formation of a solid solution series under the applied conditions (mechanochemical synthesis) can be rationalized by the smaller unit cell volume of the solid solution compared to the smaller combined unit cell volumes of the boundary phases Li₂Se and α-Li₃As as well as the gain in configurational entropy, which is generated by introducing selenide to the anion lattice of arsenide (Fig. 6). The free mixing energy (ΔG_mix) for a solid solution is defined as the sum of the mixing enthalpy (ΔH_mix) and the mixing configurational entropy (ΔS_mix).²⁸ The mixing enthalpy (ΔH_mix) can be further described as the sum of the inner energy (ΔU_mix) and the product of the applied pressure (p) and the change in the volume (ΔV_mix):

ΔG_mix = ΔU_mix + pΔV_mix − TΔS_mix (1)

ΔV_mix is negative because the unit cell volume of the solid solution is smaller than the combined unit cell volumes of the boundary phases Li₂Se and Li₃As. This is because the cubic modification of Li₃As in the solid solution has a smaller unit cell volume than its hexagonal polymorph. Additional local high-pressure applied via mechanochemical synthesis results in an increased negative contribution to ΔG_mix and therefore favors the formation of the cubic solid solution. Secondly, elevated temperatures in the mechanochemical synthesis favor the presence of configurational entropy (ΔS_mix), which can be calculated by taking the mole fractions of arsenide (x_As) and selenide (x_Se) into account:²⁸

ΔS_mix = −R(x_As ln x_As + x_Se ln x_Se), (2)

where R (J K⁻¹ mol⁻¹) is the gas constant.

This gain in entropy leads to a higher free energy (negative ΔG_mix) for the solid solution of Li₃As–Li₂Se compared to the pristine compounds Li₃As and Li₂Se and stabilizes the cubic phase. Hence the cubic structure of Li₃As can be stabilized by a sufficient degree of heterovalent substitution in the anion lattice.

Thermodynamic stability of the solid solution

Thermal properties of the Li₃As–Li₂Se system were investigated by annealing Li_2.6As_0.6Se_0.4 samples to temperatures ranging from 200 °C to 700 °C for one week. Fig. 7 shows no change in the reflection profile up to a temperature of 300 °C, indicating phase stability. At 400 °C, the powder diffractogram shows complete decomposition (exsolution) of the solid solution into hexagonal Li₃As and Li₂Se revealing the metastable character of the solid solution. Similar findings have been reported for the Li₃P–Li₂S system, where powder diffraction data display the exsolution of the solid solution into Li₃P and Li₂S in the temperature range of 400 °C to 500 °C.⁶ The thermodynamic instability of the solid solution at elevated temperatures indicates that the phases synthesized through mechanochemical methods are only kinetically stable under ambient conditions and decompose when energy (heat) is introduced to the system. This confirms the assumption that the synthesis conditions during the ball milling process must exceed ambient pressure and temperature since the solid solution is not accessible via classical solid state synthesis at elevated temperatures. At 700 °C, the two most intense reflections of Li₂Se broaden and shift to lower 2θ values accompanied by an intensity decrease of Li₃As compared to the XRPD data at 600 °C. These two observations suggest that after annealing the sample to 700 °C, some quantities of Li₃As remain incorporated in a solid solution with Li₂Se, indicating an increase in the phase stability of the solid solution. This finding may be thermodynamically explained since the free mixing energy of solid solutions at high temperatures and constant pressure is predominantly determined by the additional configurational entropy (ΔG_mix = ΔU_mix + pΔV_mix – TΔS_mix) of the arsenide/selenide lattice and therefore phase stability of the solid solution at temperatures above 700 °C might be possible. However, further investigations at higher temperatures were not performed, because the quartz tubes were already severely damaged after heat treatment at 700 °C. DTA of Li_2.6As_0.6Se_0.4 (Fig. S2‡) shows one endothermic peak in the two heating cycles (478 °C and 486 °C) and one exothermal peak in the two cooling cycles (469 °C and 470 °C), which can be assigned to melting and recrystallisation processes, since the formation of a regulus was observed. Additionally, XRPD of the analyzed sample reveals the presence of Li₃As and LiAs after DTA and the XRPD pattern of the cubic solid solution is shifted to higher 2θ values, indicating a lower mole fraction of Li₃As in the solid solution after DTA (Fig. S3‡). Both findings combined indicate a partial decomposition process of the solid solution during the DTA into hexagonal Li₃As and LiAs, forming a Li₂Se-richer solid solution. The annealed samples, as shown in Fig. 7, confirm the thermal instability of the solid solution above 400 °C. Additionally, since the cubic structure of Li₃As is not stable under these conditions, the exsolution of arsenide anions from the solid solution is plausible.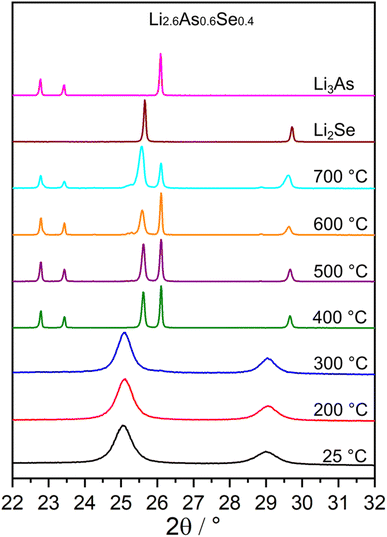

Fig. 7 Powder diffractograms of the Li_2.6As_0.6Se_0.4 compound after annealing to the displayed temperatures for one week and powder diffractograms of the end members Li₃As (hexagonal) and Li₂Se.

Impedance spectroscopy

AC impedance spectroscopy with ion blocking electrodes (indium) was performed to investigate the lithium-ion mobility in the solid solution in the Li₃As–Li₂Se system. For the phases Li_2.2As_0.2Se_0.8, Li_2.4As_0.4Se_0.6, Li_2.6As_0.6Se_0.4 and Li_2.8As_0.8Se_0.2 Nyquist plots (Fig. S5‡) were recorded in the temperature range from 50 to 100 °C in steps of 10 °C. Impedance spectra were fitted with an equivalent circuit (Table S4‡) and the resulting resistance values were used to calculate the specific ion conductivities of the samples (Table S2‡). In Fig. 8 the specific ion conductivities of the homogeneous solid solutions in the Li₃As–Li₂Se system are displayed in an Arrhenius plot. Activation energies (E_a) are given in Table S5.‡ Higher lithium-ion conductivities are observed with increasing content of As in the samples. Conductivity values at 50 °C of the solid solution range from 10⁻⁶ S cm⁻¹ for Li_2.2As_0.2Se_0.8 to 10⁻⁴ S cm⁻¹ for Li_2.8As_0.8Se_0.2 with an activation energy of approximately 0.45 eV (Table S2‡). This two-order-of-magnitude difference in lithium-ion conductivities can be explained by the increasing lithium-ion content in the octahedral voids along with the increasing As content. The higher charge carrier concentration in the octahedral voids therefore correlates with a higher overall lithium-ion conductivity. All measured ion conductivities in the Li₃As–Li₂Se system show significantly better lithium-ion mobility than the parent compounds Li₃As and Li₂Se. Our group previously reported a lithium-ion conductivity of about 10⁻⁷ S cm⁻¹ for Li₃As (gold blocking electrodes) at 50 °C and herein we observed lithium-ion conductivities of about 10⁻⁷ to 10⁻⁵ S cm⁻¹ (gold blocking electrodes) for Li₂Se in the temperature range of 175 to 300 °C (Fig. S5 and Table S3‡).³ At lower temperatures, the lithium-ion conductivities of Li₂Se were not measured because the impedance exceeded the range of our setup. Nevertheless, Li₂Se exhibits significantly lower lithium-ion conductivity values compared to the corresponding solid solution. Similar findings have been reported for the closely related solid solution in the Li₃P–Li₂S system. For this system, impedance spectroscopy (lithium blocking electrodes) shows lithium-ion conductivities of 10⁻⁵ to 10⁻⁴ S cm⁻¹ at room temperature, increasing with the P content, and these values are significantly higher compared to the binary phases Li₂S (10⁻¹⁴ to 10⁻¹⁰ S cm⁻¹)^7,8 and Li₃P (10⁻⁷ S cm⁻¹).⁶ However, the best Li ion conductors to date like Li₁₀GeP₂S₁₂ achieve conductivities up to 10⁻² S cm⁻¹,²⁹ which is not reached within the Li₃As–Li₂Se system.

Fig. 8 Arrhenius plot of the solid solution Li_3−xAs_1−xSe_x (0.2 ≤ x ≤ 1).

Experimental

Because of the high air- and moisture-sensitivity of the compounds under discussion all manipulations were carried out in an argon-filled glovebox (M Braun) with oxygen and moisture levels less than 0.5 ppm. The materials must be handled with extreme caution, since the investigated compounds are highly toxic and reach high vapor pressures upon heating.

High-pressure synthesis

High-pressure experiments were performed in a belt apparatus according to the method described by Range and Leeb by using BN crucibles.³⁰ Samples of hexagonal Li₃As were pressed for 3 days at a temperature of 1350 °C and 3.6 GPa and were subsequently quenched to ambient conditions.

Synthesis

The samples in the Li₃As–Li₂Se system were prepared via ball milling (FRITSCH Pulverisette 7 premium line, 10 zirconia grinding balls (Ø = 10 mm), and 25 mL zirconia grinding bowls). Stoichiometric amounts (batch size: 2 g) of the elements Li (Merck, 99%), As (Chempur, sublimed) and Se (99.99%). 12 to 36 milling cycles (5 minutes of milling followed by 4 minutes of sample equilibration) at a rotation speed of 600 rotations per minute were performed to synthesize the compounds.

Powder diffraction

The powders of the samples were finely ground in an agate mortar, filled in quartz capillaries (Ø = 0.3 mm) and were subsequently flame sealed. The capillaries were placed on a STOE STADI P diffractometer (Stoe & Cie) equipped with a spinner, and a Mythen 1 K detector, using CuKα₁-radiation (λ = 1.5406 Å). Raw data were handled with the WinXPOW software package (Stoe & Cie).³¹

Differential thermal analysis (DTA)

Flame-sealed quartz tubes (Ø = 2 mm) filled with the reacted samples were measured using a SETARAM TG-DTA 92.16.18. Thermal analysis was carried out in the temperature range of 25 to 800 °C at a heating/cooling rate of 10 °C min⁻¹.

Impedance spectroscopy

AC impedance conductivity measurements were performed using a Zahner Zennium impedance analyzer. Temperature was controlled using an Eurotherm heating device. The whole setup was placed in a glovebox (M Braun) under an argon atmosphere. Impedance data were recorded in steps of 10 °C from 50–100 °C for frequencies of 1 MHz to 100 mHz. Active cooling is not possible with the setup in the argon-filled glovebox. Therefore, the temperature dependent series were started at 50 °C. The samples were cold pressed to pellets (Ø = 8 mm) and placed between pieces of indium foil which were in contact with platinum electrodes. The processing and fitting of the data were performed using the Zahner Analysis software package.³²

Conclusions

We report the synthesis and characterization of the hexagonal (Na₃As structure type) to cubic (Li₃Bi structure type) phase transition of Li₃As at high temperature and high pressure. The cubic polymorph of Li₃As was obtained through heat and pressure treatment in a belt press followed by quenching to ambient conditions and characterization via XRPD.

Furthermore, we investigated the solid solution in the Li₃As–Li₂Se system stabilizing the cubic modification of Li₃As via configurational entropy by heterovalent substitution of the arsenide ions with selenide ions. The samples in the Li₃As–Li₂Se system were synthesized via ball milling of stoichiometric amounts of the corresponding elements. Powder diffraction data of the ball milled samples show miscibility of Li₃As and Li₂Se in the range of 0.2 ≤ x < 1 (Li_3−xAs_1−xSe_x). The cubic lattice parameter of these samples follow Vegard's law.²⁷ The structure of the solid solution series can be described as a filled antifluorite structure type or alternatively as a deficient Li₃Bi structure type, i.e. arsenide and selenide ions occupy the anion positions of a fcc lattice. The tetrahedral voids therein are fully occupied by lithium cations. The octahedral voids are partly filled with the remaining lithium cations, as determined from the arsenide : selenide ratio. Despite the different crystal systems of Li₃As (hexagonal) and Li₂Se (cubic) under ambient conditions a solid solution is formed after mechanochemical synthesis. Since we have evidence for the cubic high-pressure modification of Li₃As, it is plausible to claim that cubic Li₃As is accessible via mechanochemical synthesis. We propose the formation of the solid solution during the ball milling process. The gain in configurational entropy (ΔS_mix) from mixing arsenide and selenide in the anion lattice along with the smaller volume (ΔV_mix) of the solid solution compared to the boundary phases can be regarded as the driving force for the formation of the cubic solid solution. Elevated local pressure and temperature in the mechanochemical synthesis amplify both influences on the free mixing energy (ΔG_mix = ΔU_mix + pΔV_mix – TΔS_mix), thus leading to the stabilization of the solid solution. The exsolution process of the solid solution at 400 °C into the boundary phases Li₃As and Li₂Se reveals the metastable character of the solid solution at elevated temperatures. Impedance spectroscopy shows high lithium-ion conductivity for Li₃As–Li₂Se, ranging from about 10⁻⁴ to 10⁻⁶ S cm⁻¹ at 50 °C, which is significantly higher than the conductivities of the parent compounds Li₃As and Li₂Se.³

Data availability

All data, i.e., X-ray powder data, thermal analyses and impedance measurements are available from the authors on request.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

The authors express their gratitude to the Free State of Bavaria and the University of Regensburg for providing excellent working conditions and thank Ulrike Schieβl for DTA measurements.

References

1. H. J. Beister, K. Syassen and J. Klein, Phase Transition of Na₃As under Pressure, Z. Naturforsch. B, 1990, 45, 1388–1392.
2. H. J. Beister, J. Klein, I. Schewe and K. Syassen, Structural phase transitions of alkali metal pnictides and chalkogenides, High Press. Res., 1991, 7, 91–95.
3. F. Wegner, F. Kamm, F. Pielnhofer and A. Pfitzner, Li₃As and Li₃P revisited: DFT modelling on phase stability and ion conductivity, Z. Anorg. Allg. Chem., 2022, 648, me202100358.
4. T. M. F. Restle, L. Scherf, J. V. Dums, A. G. Mutschke, R. J. Spranger, H. Kirchhain, A. J. Karttunen, L. van Wüllen and T. F. Fässler, Lithium-ion Mobility in Li₆B₁₈(Li₃N) and Li Vacancy Tuning in the Solid Solution Li₆B₁₈(Li₃N)_1-x(Li₂O)_x, Angew. Chem., Int. Ed., 2023, 62, e202213962.
5. R. J. Spranger, H. Kirchhain, T. M. F. Restle, J. V. Dums, A. J. Karttunen, L. van Wüllen and T. F. Fässler, Mechanism of Li-Ion Migration in the Superionic Conducting Open-Framework Structure Li₆B₁₈(Li₃N)_1–x(Li₂O)_x (0 ≤ x ≤ 1), J. Phys. Chem. C, 2023, 127, 1622–1632.
6. C. Szczuka, B. Karasulu, M. F. Groh, F. N. Sayed, T. J. Sherman, J. D. Bocarsly, S. Vema, S. Menkin, S. P. Emge, A. J. Morris and C. P. Grey, Forced Disorder in the Solid Solution Li₃P-Li₂S: A New Class of Fully Reduced Solid Electrolytes for Lithium Metal Anodes, J. Am. Chem. Soc., 2022, 144, 16350–16365.
7. Z. Lin, Z. Liu, N. J. Dudney and C. Liang, Lithium superionic sulfide cathode for all-solid lithium-sulfur batteries, ACS Nano, 2013, 7, 2829–2833.
8. Y. Seino, T. Ota, K. Takada, A. Hayashi and M. Tatsumisago, A sulphide lithium super ion conductor is superior to liquid ion conductors for use in rechargeable batteries, Energy Environ. Sci., 2014, 7, 627–631.
9. V.-A. Ha, G. Yu, F. Ricci, D. Dahliah, M. J. van Setten, M. Giantomassi, G.-M. Rignanese and G. Hautier, Computationally driven high-throughput identification of CaTe and Li₃Sb as promising candidates for high-mobility p-type transparent conducting materials, Phys. Rev. Mater., 2019, 3, 034601.
10. K. Peng, Z. Zhou, H. Wang, H. Wu, J. Ying, G. Han, X. Lu, G. Wang, X. Zhou and X. Chen, Thermoelectric performance of binary lithium-based compounds: Li₃Sb and Li₃Bi, Appl. Phys. Lett., 2021, 119, 033901.
11. X. Yang, Z. Dai, Y. Zhao, J. Liu and S. Meng, Low lattice thermal conductivity and excellent thermoelectric behavior in Li₃Sb and Li₃Bi, J. Phys.: Condens. Matter, 2018, 30, 425401.
12. M. Yahyaoglu, T. Soldi, M. Ozen, C. Candolfi, G. J. Snyder and U. Aydemir, Stress/pressure-stabilized cubic polymorph of Li₃Sb with improved thermoelectric performance, J. Mater. Chem. A, 2021, 9, 25024–25031.
13. C. M. Rost, E. Sachet, T. Borman, A. Moballegh, E. C. Dickey, D. Hou, J. L. Jones, S. Curtarolo and J.-P. Maria, Entropy-stabilized oxides, Nat. Commun., 2015, 6, 8485.
14. A. Sarkar, Q. Wang, A. Schiele, M. R. Chellali, S. S. Bhattacharya, D. Wang, T. Brezesinski, H. Hahn, L. Velasco and B. Breitung, High-Entropy Oxides: Fundamental Aspects and Electrochemical Properties, Adv. Mater., 2019, 31, e1806236.
15. S. Senkale, M. Kamp, S. Mangold, S. Indris, L. Kienle, R. K. Kremer and W. Bensch, Multi-Method Characterization of the High-Entropy Spinel Oxide Mn_0.2Co_0.2Ni_0.2Cu_0.2Zn_0.2Fe₂O₄: Entropy Evidence, Microstructure, and Magnetic Properties, Chem. Methods, 2023, 3, e202200043.
16. Q. Wang, A. Sarkar, D. Wang, L. Velasco, R. Azmi, S. S. Bhattacharya, T. Bergfeldt, A. Düvel, P. Heitjans, T. Brezesinski, H. Hahn and B. Breitung, Multi-anionic and -cationic compounds: new high entropy materials for advanced Li-ion batteries, Energy Environ. Sci., 2019, 12, 2433–2442.
17. M. E. Leonova, I. K. Bdikin, S. A. Kulinich, O. K. Gulish, L. G. Sevast’yanova and K. P. Burdina, High-Pressure Phase Transition of Hexagonal Alkali Pnictides, Inorg. Mater., 2003, 39, 266–270.
18. E. Zintl, A. Harder and B. Dauth, Gitterstruktur der Oxyde, Sulfide, Selenide und Telluride des Lithiums, Natriums und Kaliums, Z. Elektrochem. Angew. Phys. Chem., 1934, 40, 588–593.
19. D. T. Cromer, The crystal structure of LiAs, Acta Cryst., 1959, 12, 36–41.
20. G. Brauer and E. Zintl, Konstitution von Phosphiden, Arseniden, Antimoniden und Wismutiden des Lithiums, Natriums und Kaliums, Z. Phys. Chem. B, 1937, 37, 323–352.
21. W. Hönle, J. Buresch, K. Peters, J. H. Chang and H. G. von Schnering, Crystal structure of the low-temperature modification of trilithium heptaarsenide, LT-Li₃As₇, Z. Kristallogr. N. Cryst. Struct., 2002, 217, 485–486.
22. T. J. Bastow and H. J. Whitfield, Crystal data and nuclear quadrupole resonance spectra of tetra-arsenic triselenide, J. Chem. Soc., Dalton Trans., 1977, 959.
23. T. J. Bastow and H. J. Whitfield, Crystal structure of tetra-arsenic tetraselenide, J. Chem. Soc., Dalton Trans., 1973, 1739.
24. A. C. Stergiou and P. J. Rentzeperis, The crystal structure of arsenic selenide, As₂Se₃, Z. für Kristallogr. - Cryst. Mater., 1985, 173, 185–191.
25. L. M. Lityagina, L. F. Kulikova, I. P. Zibrov, T. I. Dyuzheva, N. A. Nikolaev and V. V. Brazhkin, Structural transformations in the As–Se system under high pressures and temperatures, J. Alloys Compd., 2015, 644, 799–803.
26. T. K. Bera, J. I. Jang, J.-H. Song, C. D. Malliakas, A. J. Freeman, J. B. Ketterson and M. G. Kanatzidis, Soluble semiconductors AAsSe₂ (A = Li, Na) with a direct-band-gap and strong second harmonic generation: a combined experimental and theoretical study, J. Am. Chem. Soc., 2010, 132, 3484–3495.
27. L. Vegard, Die Konstitution der Mischkristalle und die Raumfllung der Atome, Z. Phys., 1921, 5, 17–26.
28. A. Sarkar, B. Breitung and H. Hahn, High entropy oxides: the role of entropy, enthalpy and synergy, Scr. Mater., 2020, 187, 43–48.
29. N. Kamaya, K. Homma, Y. Yamakawa, M. Hirayama, R. Kanno, M. Yonemura, T. Kamiyama, Y. Kato, S. Hama, K. Kawamoto and A. Mitsui, A lithium superionic conductor, Nat. Mater., 2011, 10, 682–686.
30. K.-J. Range and R. Leeb, Hochdruckmodifikationen der Lanthaniden(III)sulfide Ln₂S₃ (Ln = Lu-Ho, Y) mit U₂S₃-Struktur/High Pressure Modifications of the Rare Earth Sulfides Ln₂S₃ (Ln = Lu-Ho, Y) with U₂S₃-Structure, Z. Naturforsch. B, 1975, 30, 889–895.
31. STOE-WinXPOW, Vol. Version 3.0.2.5, STOE & Cie GmbH, Darmstadt, 2011.
32. Zahner-Meßtechnik GmbH& Co. KG, Vol. Version Z.3.03, Thales-Flink, Kronach.

---

Footnotes

† Dedicated to Prof. Hans Jörg Deiseroth on the occasion of his 80th birthday.

‡ Electronic supplementary information (ESI) available. See DOI: https://doi.org/10.1039/d4mr00127c

---