Structural rule of heteroatom-modified single-atom catalysts for the CO₂ electroreduction reaction

Abstract

The carbon dioxide electroreduction reaction (CO₂RR) has emerged as a viable strategy to address pressing energy and environmental challenges. Single-atom catalysts (SACs) are of particular interest for the CO₂RR due to their maximized atom utilization. The incorporation of heteroatoms as ligands is a common strategy to modify the geometric and electronic structures of metal centers to enhance performance. Here, we employed density functional theory study to investigate nitrogen-coordinated SACs with various heteroatom ligands and elucidated the structural rule of SACs on the CO₂RR. The results show that the stability of SACs exhibits a volcano-shaped trend as a function of the ligand radius, with both excessively large and small radius compromising stability, and the planar structural SACs exhibit relatively better stabilities than the raised ones. Although the raised structural SACs have better ability to activate CO₂ for the tip effect, they also hinder CO desorption and facilitate H⁺ adsorption, leading to relatively poor CO₂RR activity and selectivity (vs. the HER). In contrast, planar-structured SACs generally show better activity and CO₂RR selectivity, where promoting the CO₂ activation step is necessary. This work provides fundamental insights into the structure-dependence of SACs and offers guidance for designing SACs for the CO₂RR or other reactions.

---

1. Introduction

The electrochemical CO₂ reduction reaction (CO₂RR) presents a promising pathway for converting CO₂ into valuable fuels and chemical feedstocks under ambient conditions.^1–5 The CO₂RR is a multiple electron-transfer process, which can generate a range of products, such as carbon monoxide (CO),^6,7 formic acid,⁸ ethylene,⁹ ethanol,¹⁰ and others. Among them, CO, which has high energy efficiency and selectivity in the CO₂RR, serves as one of the most promising products in the CO₂RR.¹¹ Numerous catalysts have been developed for CO₂RR to CO, such as Ag (or Au, Cu, and Ni)-based catalysts.^12–16 Over the last decade, single-atom catalysts (SACs) have garnered significant attention due to their maximum atom-utilization efficiency by downsizing active sites to the atomic level, which can exhibit impressive efficiency for CO₂RR to CO.¹⁷ Nevertheless, SACs still face great challenges in terms of stability and activity during the CO₂RR. How to design effective SACs for the CO₂RR has become a prominent topic in the field of electrocatalysis.

The coordination environment of metal center of SACs is generally considered to play a key role in determining their catalytic performance.^18–22 In SACs, nitrogen (N) atoms are commonly employed as ligands to anchor the metal center, due to their ability to hybridize the 2p orbital with the d orbital of the metal.^23,24 The unique configuration of SACs, where a metal center is coordinated by four N atoms within the carbon matrix (denoted as M-N₄), has been demonstrated to be effective for the CO₂RR.²⁵ Recently, numerous studies have focused on modifying the coordination environment of SACs by incorporating heteroatoms [e.g., boron (B), oxygen (O), and phosphorus (P)] to manipulate the electronic structure of the active metal center, with the aim of enhancing the stability and catalytic activity of SACs for CO₂RR to CO.^26–29 The substitution of the N-ligands of M-N₄ SACs with other heteroatoms can also change the geometric structure due to the space effect; especially when the ligand atoms have a large radius, this can elevate the metal center, facilitating the adsorption of small molecules or key intermediates and thus modulating CO₂RR performance.³⁰ For instance, the larger size of the S atom protrudes the Fe center from the first shell coordination plane Fe-S₁N₃, optimizing the charge transfer between the Fe center and intermediates independently and thereby lowering the energy barrier of the CO₂RR.³¹ Although some evidence has suggested that the introduction of other heteroatom ligands into N-coordinate SACs can effectively regulate the electronic or geometric structure of the metal center, helping to improve CO₂RR performance, there is no general consensus on the structure–performance relation of the metal center of these SACs for the CO₂RR at the atomic level,³² which is important for the design of superior SACs. It remains a significant challenge to clearly identify the atomic level structures of various SACs with different metal centers and heteroatom ligands, using advanced experimental characterization technologies.

In this study, we explored the CO₂RR to CO on SACs by virtue of the density functional theory (DFT) calculations, where the metal center is anchored on the XN₃-coordinated graphene (denoted as M-XN₃, where X represents the heteroatom ligand, X = B, C, N, O and P). The results show that the planar and raised metal centers are typical geometric structures for SACs, which can result in the extremely different chemical properties of metal centers. Based on these M-XN₃ SACs, we explored the structure dependence on their stability, CO₂RR activity and selectivity and provided some fundamental understanding of the regularity of M-XN₃ SACs for the CO₂RR.

2. Computational method

All spin-polarized density functional theory (DFT) calculations were executed utilizing the Vienna ab initio simulation package (VASP),^33,34 with the project-augmented wave (PAW) method. The exchange-correlation functional was described by the Perdew–Burke–Ernzerhof (PBE) functional³⁵ within the generalized gradient approximation (GGA).³⁶ The cutoff energy for the plane wave basis of 450 eV was set. The graphene model size is 9.84 Å × 9.84 Å, in which a vacuum layer of 15 Å was applied to separate each periodic unit cell. During the structural optimization, all atoms in the model were relaxed and the Brillouin zone was sampled using 3 × 3 × 2 Monkhorst–Pack mesh k-points. As the force of each atom in the model is less than 0.05 eV Å⁻¹, the optimization process is stopped. To more accurately describe the interactions between d orbital electrons, the DFT + U method was used.³⁷ The specific U values for different metal elements are shown in Table S1.† To describe the van der Waals interactions, the empirical correction in Grimme's scheme was used. The adsorption energy of the adsorbate was calculated with the equations: E_ads[X] = E[X/sur] − E[sur] − E[X], where E[X/sur], E[sur] and E[X] are the energies of the optimized surface with the adsorbed X molecule, the clean surface, and the gaseous X molecule, respectively. The more negative E_ads[X] indicates the stronger adsorption. The Gibbs free energy change (ΔG) is calculated as: ΔG = ΔH + ΔE_ZPE − TΔS. ΔH is the enthalpy change of the reaction, resulting from DFT calculations; ΔE_ZPE and TΔS are the energy differences of the zero point energy and entropic contributions from the vibrational frequencies; for the gaseous molecules (CO₂, CO and H₂), the entropy contributions of gaseous molecules (TΔS) are obtained with the experimental values.

3. Results and discussion

3.1 Model and stability of SACs

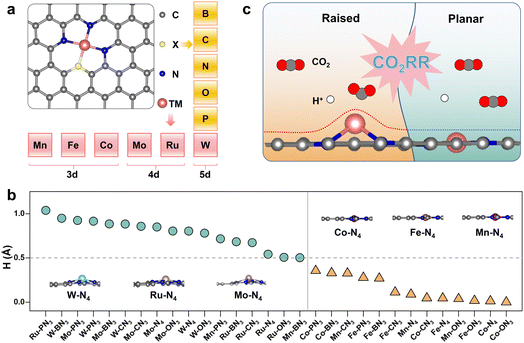
We focused on the representative N₄-coordinated metal single-atom catalysts (M-N₄) anchored on nitrogen-doped graphene as the basic model, which are popularly utilized in both experimental and theoretical studies. Several transition metal elements from different periods of the periodic table of elements experimentally used in the CO₂RR, including Mn,³⁸ Fe,³⁹ and Co⁴⁰ for 3d metals, Mo⁴¹ and Ru⁴² for 4d metals, and W^43–45 for 5d metals, were selected as metal centers (M). Here, a heteroatom (X) was introduced into M-N₄ to construct M-XN₃ (X = B, C, N, O and P), as shown in Fig. 1a. The detailed radii of the corresponding metal atom and heteroatom are shown in Fig. 2a. Accordingly, we can obtain a series of M-XN₃ catalyst models. After optimizing the structures of these M-XN₃ catalyst models, we counted the height (denoted as H, see the calculation method in Fig. S1†) of the metal center above the graphene surface, as shown in Fig. 1b. The optimized structure of each M-XN₃ is shown in Fig. S2.† It can be found that these M-XN₃ catalysts can be structurally classified into two types (Fig. 1c): (i) the raised-structured M-XN₃, where the metal center M has been pushed out of the graphene layer (H > ∼0.5 Å); (ii) the planar-structured M-XN₃, where the metal center basically stays at the graphene surface with a small H (<∼0.5 Å). A metal center with a large atomic radius generally shows raised M-XN₃ structures (Fig. 1b), such as M = W, Ru and Mo. To further illustrate the relationship between the protrusion of the metal center and the radius of the ligand X atom, we scaled the H value of different SACs with the radius of the corresponding ligand X atom; there are relatively good linear relationships between them, as shown in Fig. S3.† Their positive slopes illustrate that for a given metal center, with the increase of the radius of the ligand X atom, the protrusion of the metal center basically becomes more pronounced (i.e., larger H). In particular, in M-PN₃, where the P atom has the largest radius, the protrusion of the metal center is most prominent and reaches its maximum height.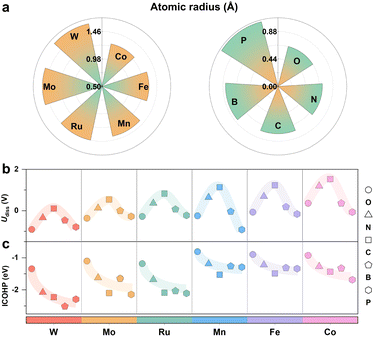

Fig. 1 (a) Structural model of an M-XN₃ catalyst, in which a heteroatom (X) is introduced into N₄-coordinated SACs anchored on nitrogen-doped graphene. The blue, gray and pink balls represent nitrogen, carbon and metal atoms, respectively, and the yellow ball represents the heteroatom. Several metal centers (M = Mn, Fe, Co, Mo, Ru and W) and heteroatoms (X = B, C, N, O and P) were chosen to construct M-XN₃ models. (b) Height (i.e., H) of the metal center above the graphene surface. (c) Schematic models of the raised and planar M-XN₃.

Fig. 2 (a) Distribution diagram of the atomic radius (Å) of the metal center and the ligand heteroatom.⁴⁶ (b) Dissolution potentials (U_diss) of each M-XN₃ as a function of the radius of the ligand heteroatom. (c) Integrated crystal orbital Hamilton population (ICOHP) of the M–X bond in M-XN₃ SACs.

The stability of SACs remains a primary challenge for their practical application in catalytic processes, as it directly determines the service life of SACs.⁴⁷ In this position, the dissolution potentials (U_diss) of M-XN₃ catalysts as an indicator of stability in the electrochemical environment were explored first (see the detailed calculation method in Note S1†). For each metal center, there is generally a volcano-shaped trend for U_diss as a function of the radius of the heteroatom (Fig. 2b). A higher U_diss means better stability of M-XN₃ in the CO₂RR. Specifically, as the radius of the heteroatom increases (from 0.66 Å for the O atom to 0.76 Å for the C atom), U_diss of each M-XN₃ generally increases and reaches the highest value at the position of the C incorporation. U_diss decreases as the radius of the heteroatom further increases (from 0.76 Å for the C atom to 1.07 Å for the P atom). It is evident that the ligand heteroatom with either too small or too large a radius negatively affects the stability of SACs, and selecting the ligand heteroatom in SACs with an atomic radius close to that of the C atom may contribute to the stability of SACs.

Structural analysis indicates that this difference in stability for a given M-XN₃ incorporated by heteroatoms with different atomic radii may be related to the compatibility between the ligand atom and the carbon graphene substrate. The radius of the P atom is 1.07 Å, which is much larger than that of the C atom in the graphene substrate of r = 0.76 Å, making P incompatible with the lattice of the graphene substrate. The incorporation of the ligand atom with a large radius (e.g., P atom) can strengthen the M–X bonds significantly, as demonstrated by the integrated crystal orbital Hamilton population (ICOHP) in Fig. 2c. A more negative ICOHP means stronger bond strength.⁴⁸ Interestingly, although M-PN₃ generally has a stronger M–P bond than other M–X bonds, the M-PN₃ structures have a decreased U_diss, implying poor stability. This instability arises because the heteroatom with a large radius could push the metal center out of the graphene plane, causing the metal center to detach from the anchoring site and potentially form clusters.⁴⁹ Therefore, the geometric structure of the metal center (i.e., raised or planar) on SACs could be an important factor in determining their stability.

In contrast, the incorporation of a heteroatom with a smaller radius (e.g., the O atom) weakens the M–X bond, as shown by the relatively more positive ICOHP values in Fig. 2c, and thus decreases the stability of M-XN₃. For example, for the O atom (r = 0.66 Å), which is smaller than the C atom in the graphene substrate, its incorporation results in the weakest M–X bond in all M-ON₃ SACs. In general, a ligand heteroatom with too large or too small a radius can lead to the large structural change of the metal center (i.e., raised one) or weaken the M–X bond, thereby affecting the stability of SACs. The N atom and C atoms with a similar atomic radius could be the most effective ligands for stabilizing SACs at the carbon graphene substrate, which have been widely used in experiments.⁵⁰

3.2 Activity and selectivity of SACs for the CO₂RR

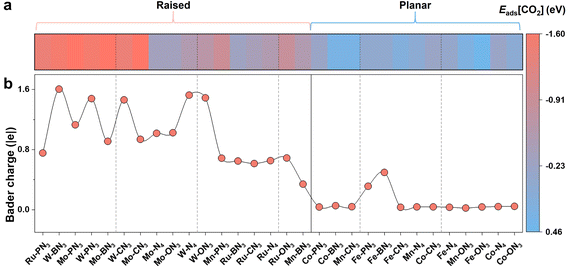
In addition to the stability, the catalytic activity of SACs is another measure of their effectiveness in the CO₂RR. The adsorption and activation of CO₂ is a key elementary process in the CO₂RR, because the CO₂ molecule is chemically inert with a linear structure and a strong C=O bond.⁵¹ Here, we first examined the adsorption energy of CO₂ (denoted as E_ads[CO₂]) on different M-XN₃ SACs and presented them in Fig. 3a. The more negative E_ads[CO₂] means the better activation of CO₂. The bending of the O–C–O bond angle and the stretching of the C=O bond serve as important indicators for CO₂ activation. From Fig. 3a, we can find that E_ads[CO₂] on M-XN₃ with a raised metal center is basically more negative than that on the planar M-XN₃, implying the relatively good adsorption and activation capacities of the raised SACs for CO₂. From the adsorption structures of CO₂ on different M-XN₃SACs, the linear CO₂ molecule becomes more bent and the C=O bonds are elongated more obviously on the raised metal center of SACs (Fig. S5†). On some M-XN₃ SACs with a planar metal center, the CO₂ molecule even remains linear, indicating their very poor ability to activate CO₂. Therefore, it can be expected that the CO₂ adsorption and activation can be better facilitated on the raised SACs compared with the planar ones.

Fig. 3 (a) Adsorption energy of the CO₂ molecule (E_ads[CO₂]) on different M-XN₃ SACs. (b) Electrons transferred from the metal center to the CO₂ molecule upon CO₂ adsorbing on different M-XN₃ SACs.

To gain a deeper insight into the different activation capacities of the raised and planar M-XN₃ SACs for CO₂, we calculated the charge density difference (CDD) upon CO₂ adsorption on different M-XN₃ structures. As shown in Fig. 3b, one can see that the raised metal center of M-XN₃ can generally donate more electrons to the CO₂ molecule via the C atom and activate the C–O bonds, leading to the remarkable bending of the O–C–O bond angle and stretching of the C–O bonds, while, on the planar M-XN₃ catalysts, the metal center usually contributes few electrons to CO₂, implying a limited ability to activate CO₂. Furthermore, the projected crystal orbital Hamilton population (pCOHP) analysis was performed to describe the interaction between the CO₂ molecule and the commonly used Fe center on different Fe-XN₃ SACs as examples, aiming to further understand the underlying mechanism of CO₂ activation on the raised or planar M-XN₃. From Fig. 4, it can be seen that the greater electron-donating capacity of the raised metal center can contribute more electrons to the filling of the bonding state of the M–CO₂ bond, strengthening the M–CO₂ bond and thereby promoting the CO₂ activation. This phenomenon can be attributed to the tip effect,⁵² where the directional aggregation of electrons in the raised metal center is promoted, thus facilitating more effective CO₂ activation.

Fig. 4 Charge density difference (CDD) for the CO₂ adsorption on the commonly used Fe center of different Fe-XN₃ SACs, and the corresponding projected crystal orbital Hamilton population (pCOHP) between the CO₂ molecule and the Fe center. The yellow and blue regions in the CDD denote the charge accumulation and depletion with an isosurface level of 0.003 e bohr⁻³.

Therefore, the raised M-XN₃ SACs are anticipated to be more effective substrates for CO₂ adsorption and activation compared to the planar one, on which the metal center exhibits the tip effect, accumulating more electrons and thereby facilitating the adsorption and activation of the CO₂ molecule. Of course, this tip effect can also promote the adsorption of other key intermediates (e.g., *COOH and *CO) in CO₂RR to CO; as shown in Fig. S6,† both *COOH and *CO on raised M-XN₃ generally exhibit a stronger adsorption energy than that on planar ones, which also have important effects on the CO₂RR (see the detailed discussion in the latter).

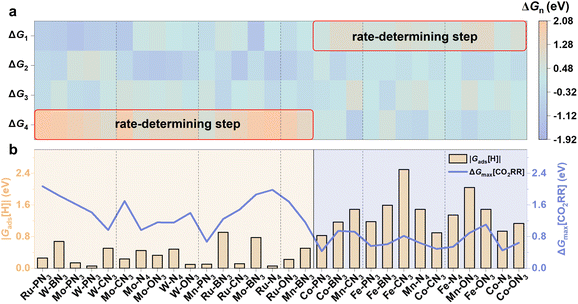
We further explored the Gibbs free energy change of the following CO₂ protonation and CO desorption steps to assess the CO₂RR activity on different M-XN₃ SACs using the computational hydrogen electrode (CHE) model⁵³ (see the calculation details in Note S2†). The maximum Gibbs free energy (ΔG_max[CO₂RR]) of the reaction steps was used to evaluate the intrinsic activities of catalysts for the CO₂RR, which is an important indicator to identify the rate-determining step. By comparing the Gibbs free energy change of each reaction step in the CO₂RR to obtain ΔG_max[CO₂RR], as shown in Fig. 5a, it is obvious that the primary rate-determining step of the CO₂RR on the raised M-XN₃ is basically located at the CO desorption step (i.e., *CO → * + CO). This mainly results from the stronger binding strength of the raised metal center compared to the planar one, which significantly hinders the CO desorption. Conversely, for planar M-XN₃, the rate-determining step basically changes to the *CO₂ adsorption and activation (i.e., * + CO₂ → *CO₂). The planar metal center provides weaker binding ability, thus limiting the CO₂ activation and protonation process. Comparing ΔG_max[CO₂RR] on different M-XN₃SACs, we can find that the planar M-XN₃ SACs basically exhibit a lower ΔG_max[CO₂RR] than the raised ones (see the blue line in Fig. 5b), implying the relatively better catalytic activity of the planar M-XN₃ SACs for CO₂RR to CO.

Fig. 5 (a) Gibbs free energies (ΔG_n) of the elementary steps in the CO₂RR. ΔG₁: CO₂ + * → *CO₂, ΔG₂: *CO₂ + H⁺/e⁻ → *COOH, ΔG₃: *COOH + H⁺/e⁻ → *CO + H₂O, and ΔG₄: *CO → * + CO. (b) Distribution of ΔG_max[CO₂RR] and |G_ads[H]| on different M-XN₃ SACs.

During the CO₂RR, the hydrogen evolution reaction (HER) serves as a common competitive side reaction, which significantly influences the efficiency of the CO₂RR.⁵⁴ In SACs, which have only one active site, once the metal center is covered by the H species to proceed with the HER, it is poisoned, thus hindering the CO₂RR. G_ads[H] is a good indicator to evaluate the activity of catalysts for the HER. As |G_ads[H]| is closer to 0 eV, catalysts could have good activity for the HER to generate H₂, which is undesirable for the CO₂RR.^55,56 To further illustrate the catalytic selectivity of the CO₂RR (vs. the HER) on different M-XN₃ SACs, we compared the absolute value of G_ads[H] (i.e., |G_ads[H]|) and ΔG_max[CO₂RR] on different M-XN₃ SACs (Fig. 5b). When the |G_ads[H]| value is larger than ΔG_max[CO₂RR], the catalyst is more likely to favor the CO₂RR over the HER. From Fig. 5b, it can be found that |G_ads[H]| on the raised M-XN₃ is generally lower than ΔG_max[CO₂RR], indicating the easier HER process compared to the CO₂RR, i.e., the poor CO₂RR selectivity of the raised M-XN₃. A similar result was also reported in previous study.²⁷ Hence, on most of the raised M-XN₃ SACs, the chance of the HER or hydrogen poisoning is more. In contrast, on the relatively planar M-XN₃ SACs, ΔG_max[CO₂RR] is generally lower than |G_ads[H]|, meaning their better adjustability for CO₂RR selectivity, which can be realized by modifying the ligand.⁵⁷ Therefore, from this perspective of the CO₂RR selectivity, planar structures are preferable for designing SACs.

Combined with the stability and activity analysis above, it can be found that SACs exhibit a strong structure dependence in terms of stability, CO₂RR activity and selectivity (vs. the HER). Raised or planar metal centers serve as typical active sites in SACs, in which the stability of a given metal center follows a volcano-shaped trend as a function of the radius of the ligand heteroatom. The metal center with a large atomic radius and the ligand atom with either too large or too small a radius are not conducive to stabilizing SACs. Too large a radius of the metal center or the ligand atoms tends to push the metal center out of the anchoring graphene plane, easily leading to cluster formation and thus poor stability; too small a radius of the ligand atom can weaken the bond between the metal center and ligand heteroatom, diminishing the stability. Although raised M-XN₃ SACs generally exhibit worse stability than the planar ones, they show a superior ability to activate CO₂, due to the tip effect resulting from the directional aggregation of electrons in the raised metal center. However, this also strengthens the CO desorption and H⁺/e⁻ adsorption, resulting in relatively worse CO₂RR activity and selectivity (vs. the HER) compared to the planar M-XN₃ SACs. In a comprehensive comparison, the planar M-XN₃ could be more suitable for designing SACs for CO₂RR to CO, where however promoting the rate-determining CO₂ activation step is necessary.

4. Conclusions

In summary, we conducted a theoretical study toward the structure–performance relation of SACs after introducing different heteroatom ligands. We discovered that SACs generally exhibit two types of geometric structures: raised and planar metal centers. Interestingly, the stabilities of all these SACs follow a volcano-shaped trend with the change of the radius of the ligand. The ligands with too large or too small atomic radii are not conducive to the stability of SACs. The planar SACs exhibit a better stability compared with the raised ones. In terms of catalytic activity, although the raised SACs possess superior ability to activate CO₂ for the tip effect, they limit the CO desorption and strengthen H⁺/e⁻ adsorption, thus leading to their worse CO₂RR activity and selectivity (vs. the HER). In comparison, the planar SACs generally exhibit better CO₂RR activity and selectivity. Planar M-XN₃ could be more suitably used to design SACs for CO₂RR to CO, on which the rate-determining CO₂ activation is necessarily promoted. We believe that this work can provide a fundamental theoretical understanding of the structure dependence of SACs and give some guidance on the design or modification of M-XN₃ SACs for the CO₂RR or even other reactions.

Data availability

All relevant data are within the manuscript and its ESI†

Author contributions

X. Y. S. performed the DFT calculations and data analysis and wrote the manuscript. Y. H. and H. Y. directed the whole research. All the authors participated in editing the manuscript and contributed their efforts to the discussion.

Conflicts of interest

There are no conflicts to declare.

Acknowledgements

This work was financially supported by the National Ten Thousand Talent Program for Young Top-notch Talent, the National Natural Science Fund for Excellent Young Scholars (52022030), the National Natural Science Foundation of China (22472053 and 51972111), the Shanghai Municipal Natural Science Foundation (23ZR1416800 and 22ZR1418000), the “Dawn” Program of the Shanghai Education Commission (22SG28), Shanghai Fundamental Research Special Zone Projects (22TQ1400100-5), the Innovation Program of Shanghai Municipal Education Commission (E00014), the Fundamental Research Funds for the Central Universities (JKM01221621 and JKM01221678) and the Shanghai Engineering Research Center of Hierarchical Nanomaterials (18DZ2252400).

Notes and references

1. Z. W. Seh, J. Kibsgaard, C. F. Dickens, I. Chorkendorff, J. K. Nørskov and T. F. Jaramillo, Science, 2017, 355, eaad4998.
2. W. Liu, P. Zhai, A. Li, B. Wei, K. Si, Y. Wei, X. Wang, G. Zhu, Q. Chen, X. Gu, R. Zhang, W. Zhou and Y. Gong, Nat. Commun., 2022, 13, 1877.
3. P. Wang, S. Meng, B. Zhang, M. He, P. Li, C. Yang, G. Li and Z. Li, J. Am. Chem. Soc., 2023, 145, 26133–26143.
4. C. Xu, Y. Dong, H. Zhao and Y. Lei, Adv. Funct. Mater., 2023, 33, 2300926.
5. N. Han, P. Ding, L. He, Y. Li and Y. Li, Adv. Energy Mater., 2020, 10, 1902338.
6. A. S. Varela, N. R. Sahraie, J. Steinberg, W. Ju, H.-S. Oh and P. Strasser, Angew. Chem., Int. Ed., 2015, 54, 10758–10762.
7. T. Möller, W. Ju, A. Bagger, X. Wang, F. Luo, T. N. Thanh, A. S. Varela, J. Rossmeisl and P. Strasser, Energy Environ. Sci., 2019, 12, 640–647.
8. K. R. Phillips, Y. Katayama, J. Hwang and Y. Shao-Horn, J. Phys. Chem. Lett., 2018, 9, 4407–4412.
9. Y. Chen, Z. Fan, J. Wang, C. Ling, W. Niu, Z. Huang, G. Liu, B. Chen, Z. Lai, X. Liu, B. Li, Y. Zong, L. Gu, J. Wang, X. Wang and H. Zhang, J. Am. Chem. Soc., 2020, 142, 12760–12766.
10. W. Xia, Y. Xie, S. Jia, S. Han, R. Qi, T. Chen, X. Xing, T. Yao, D. Zhou, X. Dong, J. Zhai, J. Li, J. He, D. Jiang, Y. Yamauchi, M. He, H. Wu and B. Han, J. Am. Chem. Soc., 2023, 145, 17253–17264.
11. H.-Y. Jeong, M. Balamurugan, V. S. K. Choutipalli, E.-s. Jeong, V. Subramanian, U. Sim and K. T. Nam, J. Mater. Chem. A, 2019, 7, 10651–10661.
12. N. Zhang, X. Zhang, L. Tao, P. Jiang, C. Ye, R. Lin, Z. Huang, A. Li, D. Pang, H. Yan, Y. Wang, P. Xu, S. An, Q. Zhang, L. Liu, S. Du, X. Han, D. Wang and Y. Li, Angew. Chem., Int. Ed., 2021, 60, 6170–6176.
13. X. Wang, P. Ou, J. Wicks, Y. Xie, Y. Wang, J. Li, J. Tam, D. Ren, J. Y. Howe, Z. Wang, A. Ozden, Y. Z. Finfrock, Y. Xu, Y. Li, A. S. Rasouli, K. Bertens, A. H. Ip, M. Graetzel, D. Sinton and E. H. Sargent, Nat. Commun., 2021, 12, 3387.
14. Q. Lei, L. Huang, J. Yin, B. Davaasuren, Y. Yuan, X. Dong, Z.-P. Wu, X. Wang, K. X. Yao, X. Lu and Y. Han, Nat. Commun., 2022, 13, 4857.
15. K. Jiang, S. Siahrostami, T. Zheng, Y. Hu, S. Hwang, E. Stavitski, Y. Peng, J. Dynes, M. Gangisetty, D. Su, K. Attenkofer and H. Wang, Energy Environ. Sci., 2018, 11, 893–903.
16. H. B. Yang, S.-F. Hung, S. Liu, K. Yuan, S. Miao, L. Zhang, X. Huang, H.-Y. Wang, W. Cai, R. Chen, J. Gao, X. Yang, W. Chen, Y. Huang, H. M. Chen, C. M. Li, T. Zhang and B. Liu, Nat. Energy, 2018, 3, 140–147.
17. M. Li, H. Wang, W. Luo, P. C. Sherrell, J. Chen and J. Yang, Adv. Mater., 2020, 32, 2001848.
18. J. Leverett, R. Daiyan, L. Gong, K. Iputera, Z. Tong, J. Qu, Z. Ma, Q. Zhang, S. Cheong, J. Cairney, R. S. Liu, X. Lu, Z. Xia, L. Dai and R. Amal, ACS Nano, 2021, 15, 12006–12018.
19. L. Jiao, J. Li, L. L. Richard, Q. Sun, T. Stracensky, E. Liu, M. T. Sougrati, Z. Zhao, F. Yang, S. Zhong, H. Xu, S. Mukerjee, Y. Huang, D. A. Cullen, J. H. Park, M. Ferrandon, D. J. Myers, F. Jaouen and Q. Jia, Nat. Mater., 2021, 20, 1385–1391.
20. J. N. Tiwari, S. Sultan, C. W. Myung, T. Yoon, N. Li, M. Ha, A. M. Harzandi, H. J. Park, D. Y. Kim, S. S. Chandrasekaran, W. G. Lee, V. Vij, H. Kang, T. J. Shin, H. S. Shin, G. Lee, Z. Lee and K. S. Kim, Nat. Energy, 2018, 3, 773–782.
21. J. Su, L. Yu, B. Han, F. Li, Z. Chen and X. C. Zeng, J. Phys. Chem. Lett., 2024, 15, 8600–8607.
22. L. Yu, F. Li, J. Huang, B. G. Sumpter, W. E. Mustain and Z. Chen, ACS Catal., 2023, 13, 9616–9628.
23. Z. Li, R. Wu, S. Xiao, Y. Yang, L. Lai, J. S. Chen and Y. Chen, Chem. Eng. J., 2022, 430, 132882.
24. S. Liu, H. B. Yang, S.-F. Hung, J. Ding, W. Cai, L. Liu, J. Gao, X. Li, X. Ren, Z. Kuang, Y. Huang, T. Zhang and B. Liu, Angew. Chem., Int. Ed., 2020, 59, 798–803.
25. A. S. Varela, W. Ju, A. Bagger, P. Franco, J. Rossmeisl and P. Strasser, ACS Catal., 2019, 9, 7270–7284.
26. Y. Dai, H. Li, C. Wang, W. Xue, M. Zhang, D. Zhao, J. Xue, J. Li, L. Luo, C. Liu, X. Li, P. Cui, Q. Jiang, T. Zheng, S. Gu, Y. Zhang, J. Xiao, C. Xia and J. Zeng, Nat. Commun., 2023, 14, 3382.
27. Z. Chang, W. Zhao, M. Liu, M. Fan, B. Wang and R. Zhang, Appl. Surf. Sci., 2022, 597, 153755.
28. K. Li, S. Zhang, X. Zhang, S. Liu, H. Jiang, T. Jiang, C. Shen, Y. Yu and W. Chen, Nano Lett., 2022, 22, 1557–1565.
29. Z. Qiao, R. Jiang, H. Xu, D. Cao and X. C. Zeng, Angew. Chem., Int. Ed., 2024, 40, e202407812.
30. Z. Lou, W. Li, H. Yuan, Y. Hou, H. Yang and H. Wang, J. Mater. Chem. A, 2022, 10, 3585–3594.
31. Z. Jin, D. Jiao, Y. Dong, L. Liu, J. Fan, M. Gong, X. Ma, Y. Wang, W. Zhang, L. Zhang, Z. Gen Yu, D. Voiry, W. Zheng and X. Cui, Angew. Chem., Int. Ed., 2024, 63, e202318246.
32. T. Tang, Z. Wang and J. Guan, Adv. Funct. Mater., 2022, 32, 2111504.
33. G. Kresse and J. Hafner, Phys. Rev. B:Condens. Matter Mater. Phys., 1993, 47, 558–561.
34. G. Kresse and J. Hafner, Phys. Rev. B:Condens. Matter Mater. Phys., 1994, 49, 14251–14269.
35. G. Kresse and J. Furthmiiller, Comput. Mater. Sci., 1996, 6, 15–50.
36. J. P. Perdew, K. Burke and M. Ernzerhof, Phys. Rev. Lett., 1996, 77, 3865–3868.
37. Q. Fan, P. Hou, C. Choi, T. S. Wu, S. Hong, F. Li, Y. L. Soo, P. Kang, Y. Jung and Z. Sun, Adv. Energy Mater., 2019, 10, 1903068.
38. H.-Y. Tan, S.-C. Lin, J. Wang, C.-J. Chang, S.-C. Haw, K.-H. Lin, L. D. Tsai, H.-C. Chen and H. M. Chen, ACS Appl. Mater. Interfaces, 2021, 13, 52134–52143.
39. W. Ni, Z. Liu, Y. Zhang, C. Ma, H. Deng, S. Zhang and S. Wang, Adv. Mater., 2021, 33, 2003238.
40. P. Yin, T. Yao, Y. Wu, L. Zheng, Y. Lin, W. Liu, H. Ju, J. Zhu, X. Hong, Z. Deng, G. Zhou, S. Wei and Y. Li, Angew. Chem., Int. Ed., 2016, 55, 10800–10805.
41. Y. Liu, D. Wang, B. Yang, Z. Li, X. Peng, Z. Liu, L. Zeng, T. Zhang, R. D. Rodriguez, L. Lei and Y. Hou, Chem. Eng. Sci., 2023, 273, 118638.
42. Z. Lian, Y. Lu, C. Wang, X. Zhu, S. Ma, Z. Li, Q. Liu and S. Zang, Adv. Sci., 2021, 8, 2102550.
43. Z. Chen, W. Gong, Z. Liu, S. Cong, Z. Zheng, Z. Wang, W. Zhang, J. Ma, H. Yu, G. Li, W. Lu, W. Ren and Z. Zhao, Nanomater. Energy, 2019, 60, 394–403.
44. S.-L. Li, Y. Song, G. Tian, Q. Liu, L. Qiao, Y. Zhao and L.-Y. Gan, Fuel Process. Technol., 2024, 261, 108106.
45. Y. Feng, C. Wang, P. Cui, C. Li, B. Zhang, L. Gan, S. Zhang, X. Zhang, X. Zhou, Z. Sun, K. Wang, Y. Duan, H. Li, K. Zhou, H. Huang, A. Li, C. Zhuang, L. Wang, Z. Zhang and X. Han, Adv. Mater., 2022, 34, 2109074.
46. B. Cordero, V. Gómez, A. E. Platero-Prats, M. Revés, J. Echeverría, E. Cremades, F. Barragán and S. Alvarez, Dalton Trans., 2008, 2832–2838.
47. J. Wan, W. Chen, C. Jia, L. Zheng, J. Dong, X. Zheng, Y. Wang, W. Yan, C. Chen, Q. Peng, D. Wang and Y. Li, Adv. Mater., 2018, 30, 1705369.
48. R. Dronskowski and P. E. Bloechl, J. Phys. Chem. C, 1993, 97, 8617–8624.
49. H. Hu, J. Wang, P. Tao, C. Song, W. Shang, T. Deng and J. Wu, J. Mater. Chem. A, 2022, 10, 5835–5849.
50. F. Pan, H. Zhang, K. Liu, D. Cullen, K. More, M. Wang, Z. Feng, G. Wang, G. Wu and Y. Li, ACS Catal., 2018, 8, 3116–3122.
51. A. Álvarez, M. Borges, J. J. Corral-Pérez, J. G. Olcina, L. Hu, D. Cornu, R. Huang, D. Stoian and A. Urakawa, ChemPhysChem, 2017, 18, 3135–3141.
52. B. Yang, K. Liu, H. Li, C. Liu, J. Fu, H. Li, J. E. Huang, P. Ou, T. Alkayyali, C. Cai, Y. Duan, H. Liu, P. An, N. Zhang, W. Li, X. Qiu, C. Jia, J. Hu, L. Chai, Z. Lin, Y. Gao, M. Miyauchi, E. Cortes, S. A. Maier and M. Liu, J. Am. Chem. Soc., 2022, 144, 3039–3049.
53. J. K. Nørskov, J. Rossmeisl, A. Logadottir, L. Lindqvist, J. R. Kitchin, T. Bligaard and H. Jónsson, J. Phys. Chem. B, 2004, 108, 17886–17892.
54. S. Jin, Z. Hao, K. Zhang, Z. Yan and J. Chen, Angew. Chem., Int. Ed., 2021, 60, 20627–20648.
55. J. Greeley, T. F. Jaramillo, J. Bonde, I. B. Chorkendorff and J. K. Nørskov, Nat. Mater., 2006, 5, 909–913.
56. J. K. Nørskov, T. Bligaard, A. Logadottir, J. R. Kitchin, J. G. Chen, S. Pandelov and U. Stimming, J. Electrochem. Soc., 2005, 152, J23.
57. A. Tripathi and R. Thapa, Carbon, 2023, 208, 330–337.

---

Footnote

† Electronic supplementary information (ESI) available. See DOI: https://doi.org/10.1039/d4ta06604a

---